
Instructions: In all exercises, include software results (e.g., from Excel, MegaStat, or Minitab) to support your calculations. State the hypotheses, show how the degrees of freedom are calculated, find the critical value of chi-square from Appendix E or from Excel’s
Pick one Excel data set (A through F) and investigate whether the data could have come from a normal population using α = .01. Use any test you wish, including a histogram, or MegaStat’s
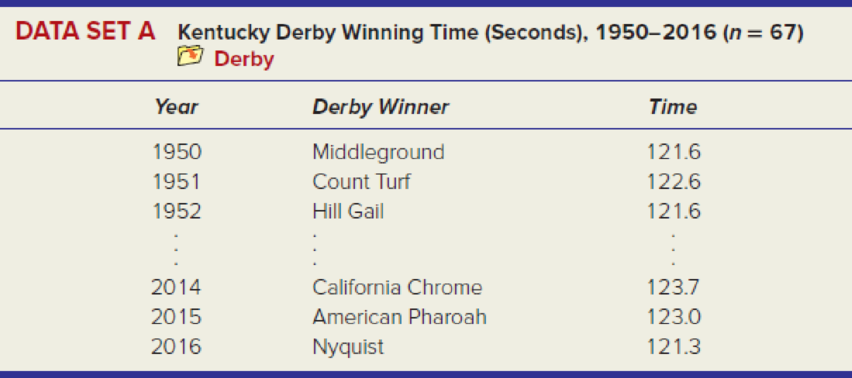
Source: www.wikipedia.org.
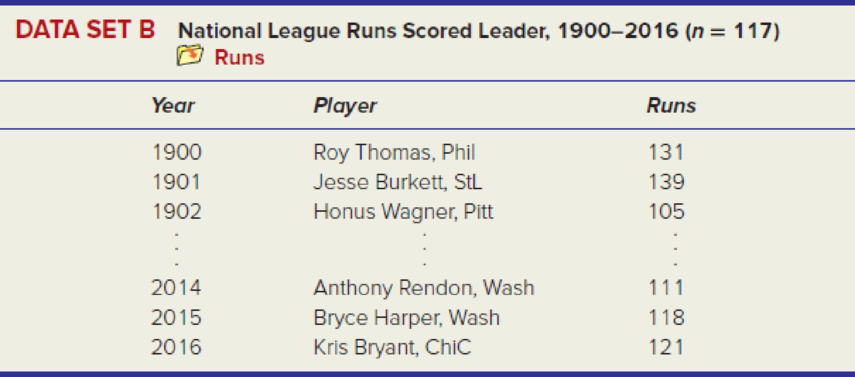
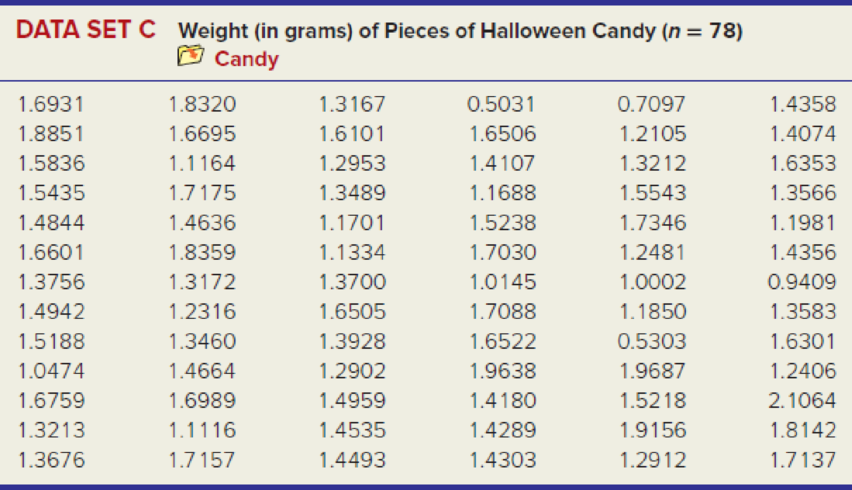
Source: Independent project by statistics student Frances Williams. Weighed on an American Scientific Model S/P 120 analytical balance, accurate to 0.0001 gram.

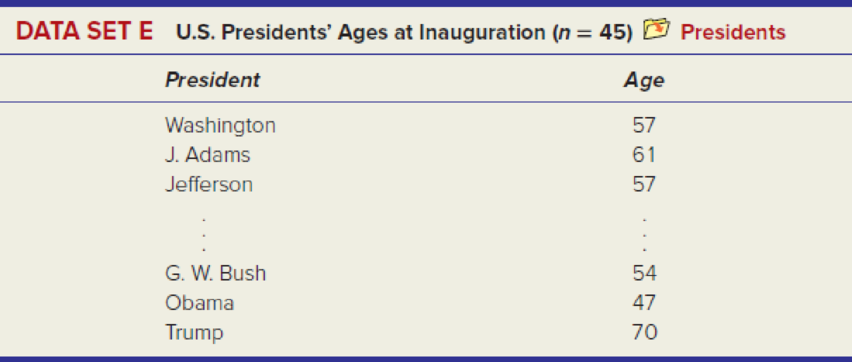
Source: www.wikipedia.org

State the null and alternative hypothesis.
Find the degrees of freedom.
Find the critical value of chi-square from Appendix E or from Excel’s function.
Calculate the chi-square test statistics at 0.01 level of significance.
Interpret the p-value.
Check whether the conclusion is sensitive to the level of significance chosen, identify the cells that contribute to the chi-square test statistic and check for the small expected frequencies.
Draw histogram.
Test to obtain a probability plot with the Anderson-Darling statistic.
Interpret p-values.
Answer to Problem 40CE
The null hypothesis is:
And the alternative hypothesis is:
The degrees of freedom is 1.
The critical-value using EXCEL is 2.705543.
The chi-square test statistics at 0.1 level of significance is 0.0486.
The p-value for the hypothesis test is 0.825518.
There is enough evidence to conclude that the Circulated nickels come from a Normal population.
The conclusion is not sensitive to the level of significance chosen.
The chi-square test statistic has highest chi-square value for zero appointments.
There is no expected frequencies that are too small.
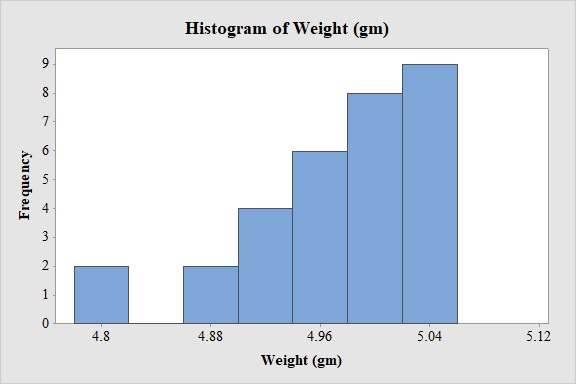
The histogram is:
The probability plot is:
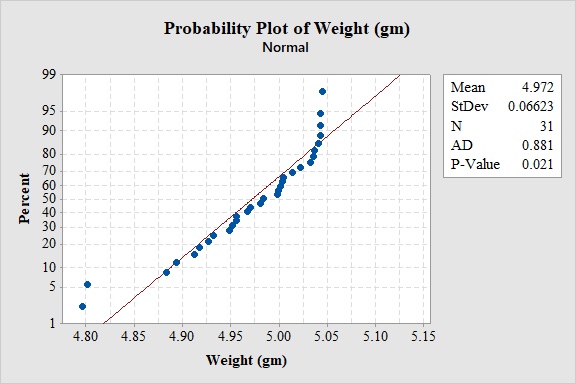
The Anderson-Darling statistics is 0.881.
The p-value is 0.021.
There is enough evidence to conclude that the Circulated nickels come from a Normal population.
Explanation of Solution
Calculation:
Results will vary.
There are 6 data set and pick any of them. The given information is the 31 randomly chosen circulated nickels.
The claim is to test whether the data provide sufficient evidence to conclude that the Circulated nickels come from a Normal population. If the claim is rejected, then the Circulated nickels do not come from a Normal population.
The test hypotheses are given below:
Null hypothesis:
Alternative hypothesis:
Software procedure:
- Step by step procedure to obtain the mean and standard deviations using the MINITAB software.
- Choose Stat > Basic Statistics>Display Descriptive Statistics.
- Under Variables, choose 'Weight'
- Choose Statistics, Select mean and Standard deviation.
- Click OK.
Output obtained from MINITAB software for the data is:
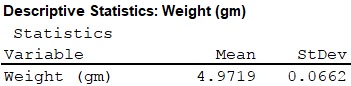
Thus, the mean and the standard deviation of the data is 4.9719 and 0.0662 respectively.
For a normal distribution the first and last class must be open-ended. The upper limit of bin j can be is obtained by the Excel’s function.
Procedure for upper limit using EXCEL:
Step-by-step software procedure to obtain upper limit for first class using EXCEL software is as follows:
- Open an EXCEL file.
- In cell A1, enter the formula “=NORM.INV(1/6,75.38,8.94)”
- Output using EXCEL software is given below:

Thus, the upper limit for first class using EXCEL is 4.908.
Similarly the remaining limits can be obtained as shown below:
| Weights |
| Under 4.908 |
| 4.908–4.943 |
| 4.943–4.972 |
| 4.972–5.000 |
| 5.000–5.036 |
| 5.036 or more |
The expected frequency can be obtained by the following formula:
Where c is the number of bins and n is the sample size.
Substitute
Then the expected frequency for each bin can be obtained as shown in the table:
| Weights | Expected Frequency |
| Under 4.908 | 5.17 |
| 4.908–4.943 | 5.17 |
| 4.943–4.972 | 5.17 |
| 4.972–5.000 | 5.17 |
| 5.000–5.036 | 5.17 |
| 5.036 or more | 5.17 |
| Total | 31 |
Frequency:
The frequencies are calculated by using the tally mark and the range of the data is from 4.796 to 5.045.
- Based on the given information, the class intervals are under 4.908, 4.908–4.943, and so on, 5.036 or more.
- Make a tally mark for each value in the corresponding class and continue for all values in the data.
- The number of tally marks in each class represents the frequency, f of that class.
Similarly, the frequency of remaining classes for the emission is given below:
| Weights | Tally | Observed Frequency |
| Under 4.908 | 4 | |
| 4.908–4.943 | 4 | |
| 4.943–4.972 | 6 | |
| 4.972–5.000 | 4 | |
| 5.000–5.036 | 8 | |
| 5.036 or more | 5 |
Let
The chi-square test statistics can be obtained by the formula:
Then the chi-square test statistics can be obtained as shown in the table:
| Weights | Frequency | Expected Frequency | ||
| Under 4.908 | 4 | 5.17 | –1.17 | 0.265 |
| 4.908–4.943 | 4 | 5.17 | –1.17 | 0.265 |
| 4.943–4.972 | 6 | 5.17 | 0.83 | 0.133 |
| 4.972–5.000 | 4 | 5.17 | –1.17 | 0.265 |
| 5.000–5.036 | 8 | 5.17 | 2.83 | 1.55 |
| 5.036 or more | 5 | 5.17 | –0.17 | 0.005 |
| Total | 31 | 31 | 0 | 2.483 |
Therefore, the chi-square test statistic is 2.483.
Degrees of freedom:
The degrees of freedom can be obtained as follows:
Where c is the number of classes and m is the number of parameters estimated. Here only 2 parameters are estimated.
Substitute 6 for c and 2 for m.
Thus, the degrees of freedom for the test is 3.
Procedure for p-value using EXCEL:
Step-by-step software procedure to obtain p-value using EXCEL software is as follows:
- Open an EXCEL file.
- In cell A1, enter the formula “=CHISQ.DIST.RT(2.483,3)”
- Output using EXCEL software is given below:
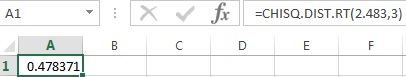
Thus, the p-value using EXCEL is 0.478.
Rejection rule:
If the p-value is less than or equal to the significance level, then reject the null hypothesis
Conclusion:
Here, the p-value is greater than the 0.01 level of significance.
That is,
Therefore, the null hypothesis is not rejected.
Thus, the data provide sufficient evidence to conclude that the Circulated nickels come from a Normal population.
Take
Here, the p-value is less than the 0.05 level of significance.
That is,
Therefore, the null hypothesis is not rejected.
Thus, the data provide sufficient evidence to conclude that the Circulated nickels come from a Normal population.
Thus, the conclusion is same for both the significance levels.
Hence, the conclusion is not sensitive to the level of significance chosen.
The class 5.000-5.036 contribute most to the chi-square test statistic.
Since all
Frequency Histogram:
Software procedure:
- Step by step procedure to draw the relative frequency histogram for mileage for 2000 using MINITAB software.
- Choose Graph > Histogram.
- Choose Simple.
- Click OK.
- In Graph variables, enter the column of 'Weight'.
- In Scale, Choose Y-scale Type as Frequency.
- Click OK.
- Select Edit Scale, Enter 4.8, 4.88, 4.96, 5.04, 5.12 in Positions of ticks.
- In Labels, Enter 4.8, 4.88, 4.96, 5.04, 5.12 41 in Specified.
- Click OK.
Observation:
It is clear from the histogram that the histogram is not symmetric. The left hand tail is little larger than the right hand tail from the maximum frequency value. Thus, there is little negative skewness on the histogram.
Software procedure:
Step by step procedure to obtain the probability plot using the MINITAB software:
- Choose Stat > Basic Statistics > Normality Test.
- In Variable, enter the column of 'Weights'.
- Under Test for Normality, select the column of Anderson-Darling.
- Click OK.
From the probability plot, it can be observed that most of the observations lies near to the straight line. Therefore, the data is from a normal distribution.
From the MINITAB output the Anderson-Darling statistics is 0.881.
From the MINITAB output the p-value is 0.021.
Conclusion:
Here, the p-value is greater than the 0.01 level of significance.
That is,
Therefore, the null hypothesis is not rejected.
Thus, the data provide sufficient evidence to conclude that the Circulated nickels come from a Normal population.
Want to see more full solutions like this?
Chapter 15 Solutions
APPLIED STAT.IN BUS.+ECONOMICS
- Why researchers are interested in describing measures of the center and measures of variation of a data set?arrow_forwardWHAT IS THE SOLUTION?arrow_forwardThe following ordered data list shows the data speeds for cell phones used by a telephone company at an airport: A. Calculate the Measures of Central Tendency from the ungrouped data list. B. Group the data in an appropriate frequency table. C. Calculate the Measures of Central Tendency using the table in point B. 0.8 1.4 1.8 1.9 3.2 3.6 4.5 4.5 4.6 6.2 6.5 7.7 7.9 9.9 10.2 10.3 10.9 11.1 11.1 11.6 11.8 12.0 13.1 13.5 13.7 14.1 14.2 14.7 15.0 15.1 15.5 15.8 16.0 17.5 18.2 20.2 21.1 21.5 22.2 22.4 23.1 24.5 25.7 28.5 34.6 38.5 43.0 55.6 71.3 77.8arrow_forward
- II Consider the following data matrix X: X1 X2 0.5 0.4 0.2 0.5 0.5 0.5 10.3 10 10.1 10.4 10.1 10.5 What will the resulting clusters be when using the k-Means method with k = 2. In your own words, explain why this result is indeed expected, i.e. why this clustering minimises the ESS map.arrow_forwardwhy the answer is 3 and 10?arrow_forwardPS 9 Two films are shown on screen A and screen B at a cinema each evening. The numbers of people viewing the films on 12 consecutive evenings are shown in the back-to-back stem-and-leaf diagram. Screen A (12) Screen B (12) 8 037 34 7 6 4 0 534 74 1645678 92 71689 Key: 116|4 represents 61 viewers for A and 64 viewers for B A second stem-and-leaf diagram (with rows of the same width as the previous diagram) is drawn showing the total number of people viewing films at the cinema on each of these 12 evenings. Find the least and greatest possible number of rows that this second diagram could have. TIP On the evening when 30 people viewed films on screen A, there could have been as few as 37 or as many as 79 people viewing films on screen B.arrow_forward
- Q.2.4 There are twelve (12) teams participating in a pub quiz. What is the probability of correctly predicting the top three teams at the end of the competition, in the correct order? Give your final answer as a fraction in its simplest form.arrow_forwardThe table below indicates the number of years of experience of a sample of employees who work on a particular production line and the corresponding number of units of a good that each employee produced last month. Years of Experience (x) Number of Goods (y) 11 63 5 57 1 48 4 54 5 45 3 51 Q.1.1 By completing the table below and then applying the relevant formulae, determine the line of best fit for this bivariate data set. Do NOT change the units for the variables. X y X2 xy Ex= Ey= EX2 EXY= Q.1.2 Estimate the number of units of the good that would have been produced last month by an employee with 8 years of experience. Q.1.3 Using your calculator, determine the coefficient of correlation for the data set. Interpret your answer. Q.1.4 Compute the coefficient of determination for the data set. Interpret your answer.arrow_forwardCan you answer this question for mearrow_forward
- Techniques QUAT6221 2025 PT B... TM Tabudi Maphoru Activities Assessments Class Progress lIE Library • Help v The table below shows the prices (R) and quantities (kg) of rice, meat and potatoes items bought during 2013 and 2014: 2013 2014 P1Qo PoQo Q1Po P1Q1 Price Ро Quantity Qo Price P1 Quantity Q1 Rice 7 80 6 70 480 560 490 420 Meat 30 50 35 60 1 750 1 500 1 800 2 100 Potatoes 3 100 3 100 300 300 300 300 TOTAL 40 230 44 230 2 530 2 360 2 590 2 820 Instructions: 1 Corall dawn to tha bottom of thir ceraan urina se se tha haca nariad in archerca antarand cubmit Q Search ENG US 口X 2025/05arrow_forwardThe table below indicates the number of years of experience of a sample of employees who work on a particular production line and the corresponding number of units of a good that each employee produced last month. Years of Experience (x) Number of Goods (y) 11 63 5 57 1 48 4 54 45 3 51 Q.1.1 By completing the table below and then applying the relevant formulae, determine the line of best fit for this bivariate data set. Do NOT change the units for the variables. X y X2 xy Ex= Ey= EX2 EXY= Q.1.2 Estimate the number of units of the good that would have been produced last month by an employee with 8 years of experience. Q.1.3 Using your calculator, determine the coefficient of correlation for the data set. Interpret your answer. Q.1.4 Compute the coefficient of determination for the data set. Interpret your answer.arrow_forwardQ.3.2 A sample of consumers was asked to name their favourite fruit. The results regarding the popularity of the different fruits are given in the following table. Type of Fruit Number of Consumers Banana 25 Apple 20 Orange 5 TOTAL 50 Draw a bar chart to graphically illustrate the results given in the table.arrow_forward
 Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL


