
Concept explainers
Find the member end moments and reaction for the frames.
Answer to Problem 31P
The end moments at the member AC
Explanation of Solution
Calculation:
Consider the elastic modulus E of the frame is constant.
Show the free body diagram of the entire frame as in Figure 1.
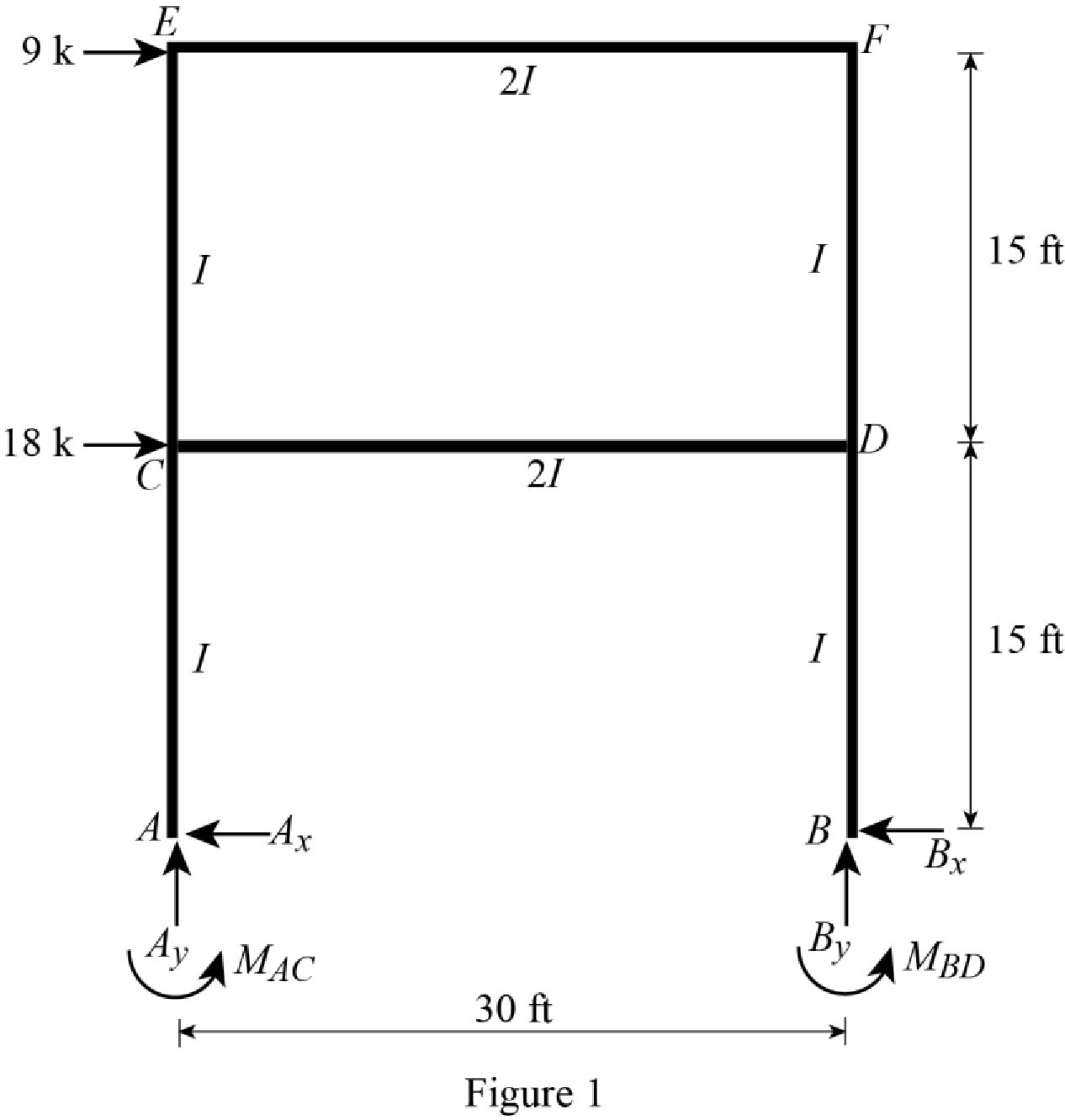
Refer Figure 1,
Calculate the fixed end moment for AC.
Calculate the fixed end moment for CA.
Calculate the fixed end moment for CD.
Calculate the fixed end moment for DC.
Calculate the fixed end moment for DB.
Calculate the fixed end moment for BD.
Calculate the fixed end moment for CE.
Calculate the fixed end moment for EC.
Calculate the fixed end moment for EF.
Calculate the fixed end moment for FE.
Calculate the fixed end moment for FD.
Calculate the fixed end moment for DF.
Chord rotations:
Show the free body diagram of the chord rotation of the frame as in Figure 2.
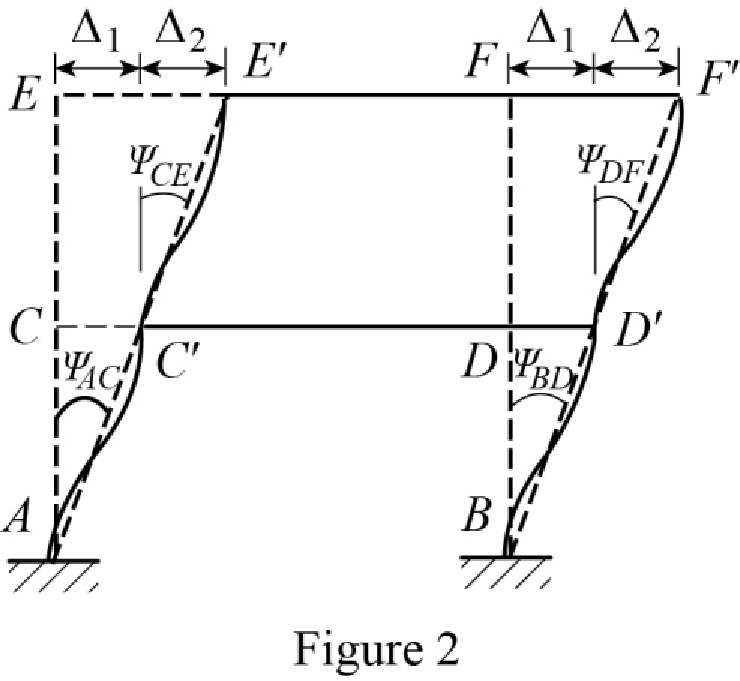
Refer Figure 2,
Calculate the chord rotation of the frame AC and BD.
Calculate the chord rotation of the frame CE and DF.
Calculate the chord rotation of the frame CD and EF.
Calculate the slope deflection equation for the member AC.
Substitute 15 ft for L, 0 for
Calculate the slope deflection equation for the member CA.
Substitute 15 ft for L, 0 for
Calculate the slope deflection equation for the member CD.
Substitute 30 ft for L, 0 for
Calculate the slope deflection equation for the member DC.
Substitute 30 ft for L, 0 for
Calculate the slope deflection equation for the member DB.
Substitute 15 ft for L, 0 for
Calculate the slope deflection equation for the member BD.
Substitute 15 ft for L, 0 for
Calculate the slope deflection equation for the member CE.
Substitute 15 ft for L,
Calculate the slope deflection equation for the member EC.
Substitute 15 ft for L,
Calculate the slope deflection equation for the member EF.
Substitute 30 ft for L, 0 for
Calculate the slope deflection equation for the member FE.
Substitute 30 ft for L, 0 for
Calculate the slope deflection equation for the member FD.
Substitute 15 ft for L,
Calculate the slope deflection equation for the member DF.
Substitute 15 ft for L,
Write the equilibrium equation as below.
Substitute equation (2), equation (3), and equation (7) in above equation.
Write the equilibrium equation as below.
Substitute equation (4), equation (5) and equation (12) in above equation.
Write the equilibrium equation as below.
Substitute equation (8) and equation (9) in above equation.
Write the equilibrium equation as below.
Substitute equation (10) and equation (11) in above equation.
Show the free body diagram of the joint E and F due to sway force as in Figure 3.
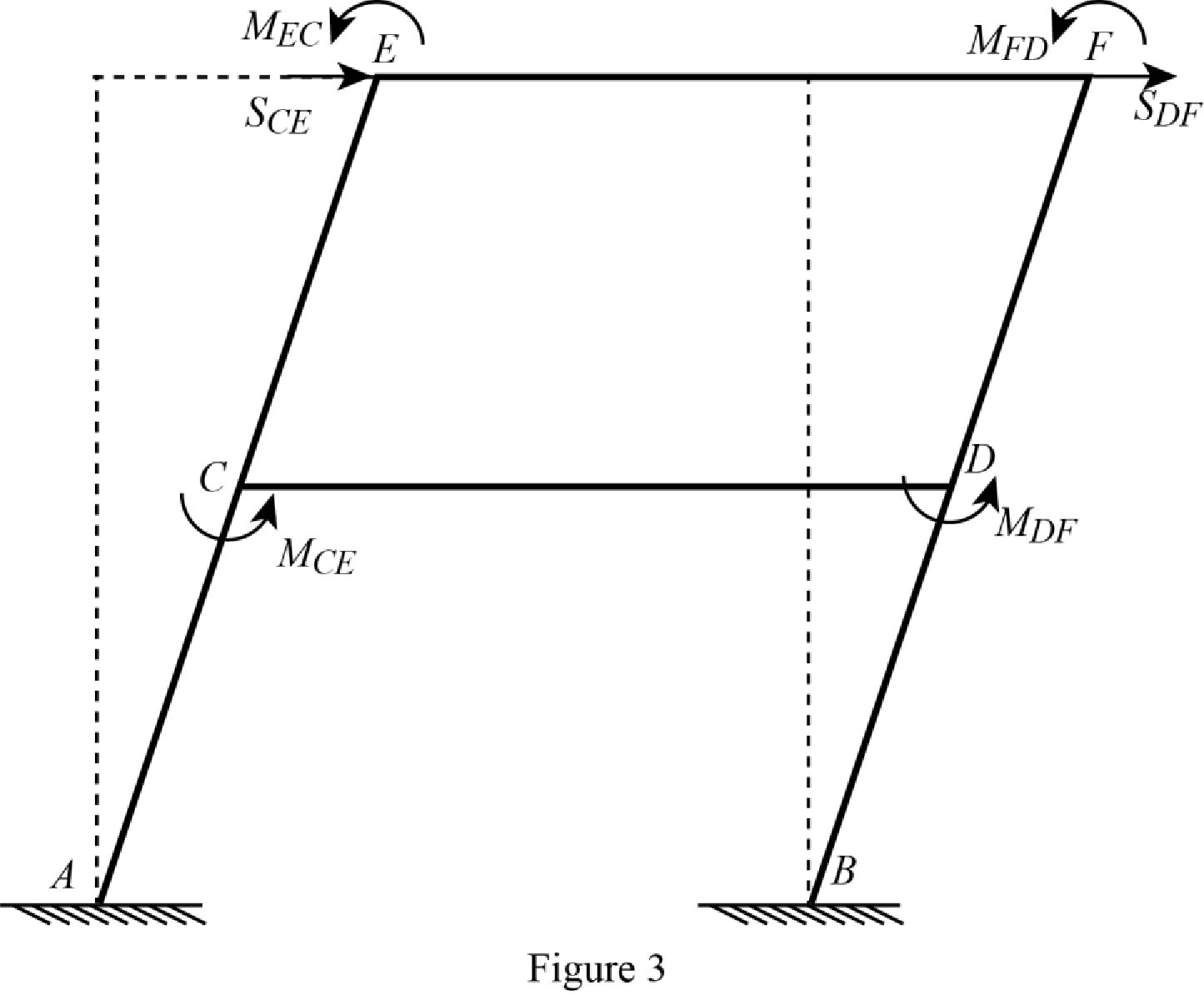
Calculate the horizontal reaction at the member CE due to sway force by taking moment about point C.
Calculate the horizontal reaction at the member DF due to sway force by taking moment about point D.
Calculate the reaction of the support E and support F due to sway force by considering horizontal equilibrium.
Substitute equation (7), (8), (11) and (12).
Show the free body diagram of the joint C and D due to sway force as in Figure 4.
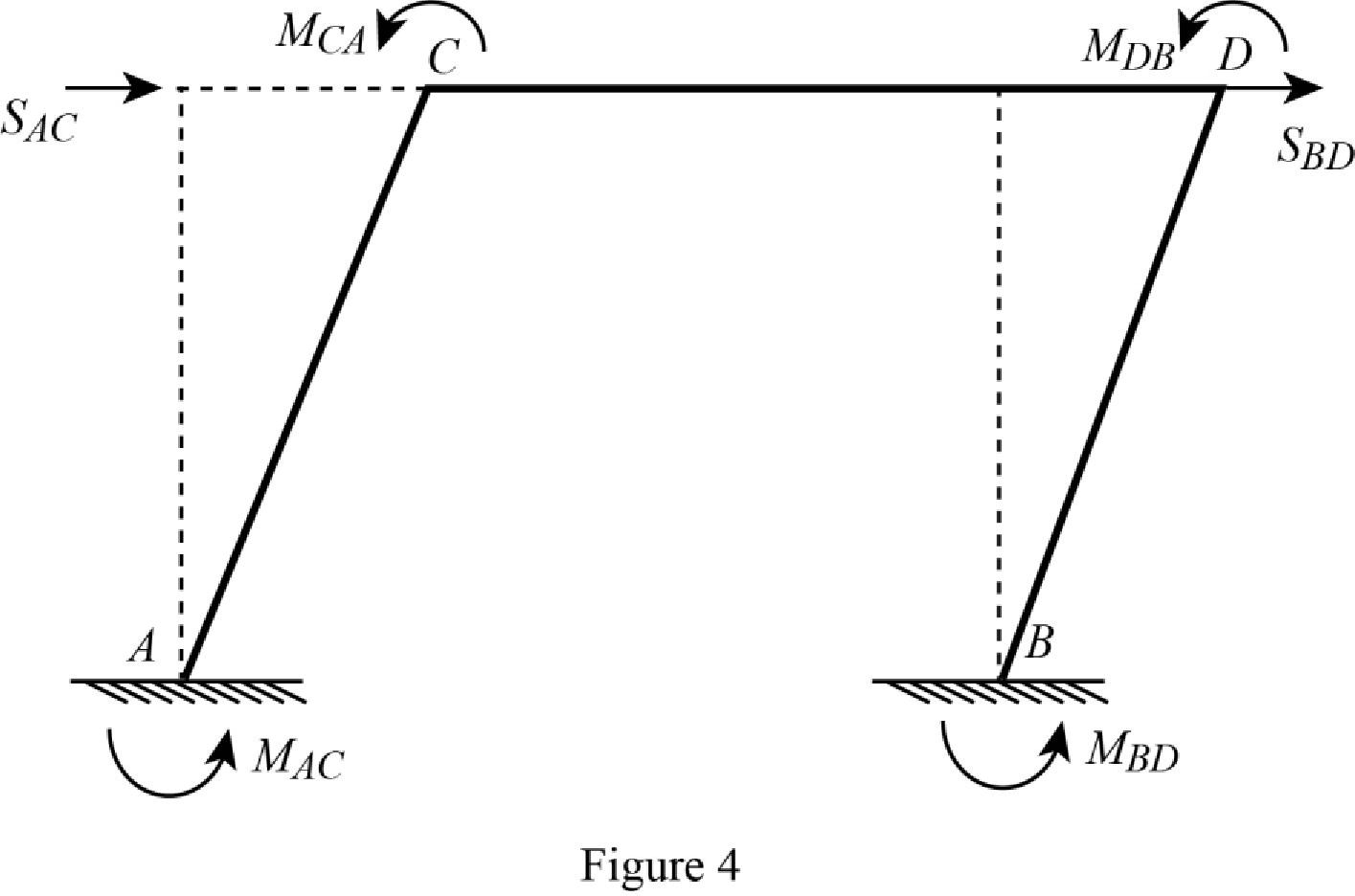
Calculate the horizontal reaction at the member AC due to sway force by taking moment about point A.
Calculate the horizontal reaction at the member BD due to sway force by taking moment about point B.
Calculate the reaction of the support C and support D due to sway force by considering horizontal equilibrium.
Substitute equation (1), equation (2), equation (5), and equation (6).
Solve the equation (13), equation (14), equation (15), equation (16), equation (17) and equation (18).
Calculate the moment about AC.
Substitute
Calculate the moment about CA.
Substitute
Calculate the moment about CD.
Substitute
Calculate the moment about DC.
Substitute
Calculate the moment about DB.
Substitute
Calculate the moment about BD.
Substitute
Calculate the moment about CE.
Substitute
Calculate the moment about EC.
Substitute
Calculate the moment about EF.
Substitute
Calculate the moment about FE.
Substitute
Calculate the moment about FD.
Substitute
Calculate the moment about DF.
Substitute
Show the section free body diagram of the member EF as in Figure 5.

Consider member EF:
Calculate the vertical reaction at the joint E by taking moment about point F.
Calculate the vertical reaction at joint F by resolving the horizontal equilibrium.
Show the section free body diagram of the member CD as in Figure 6.
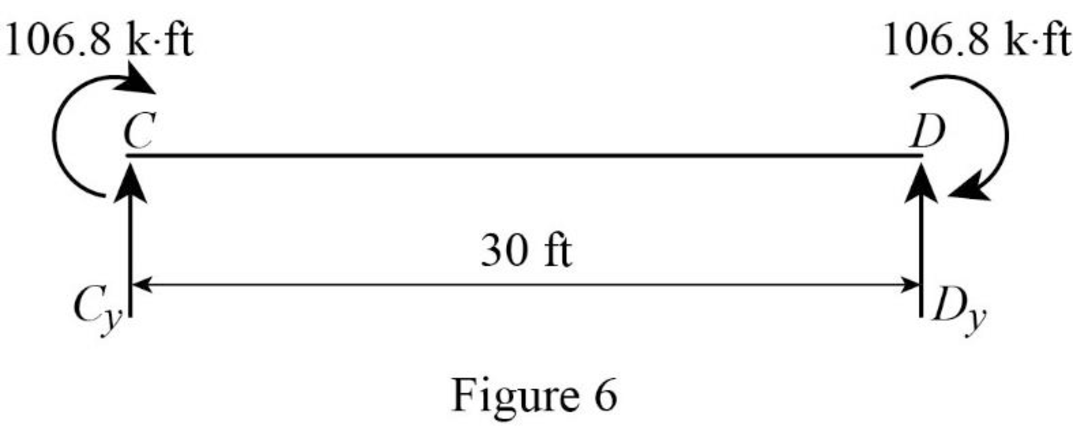
Consider member CD:
Calculate the vertical reaction at the joint C by taking moment about point D.
Calculate the vertical reaction at joint D by resolving the horizontal equilibrium.
Show the section free body diagram of the member AC, CE, DB and FD as in Figure 7.
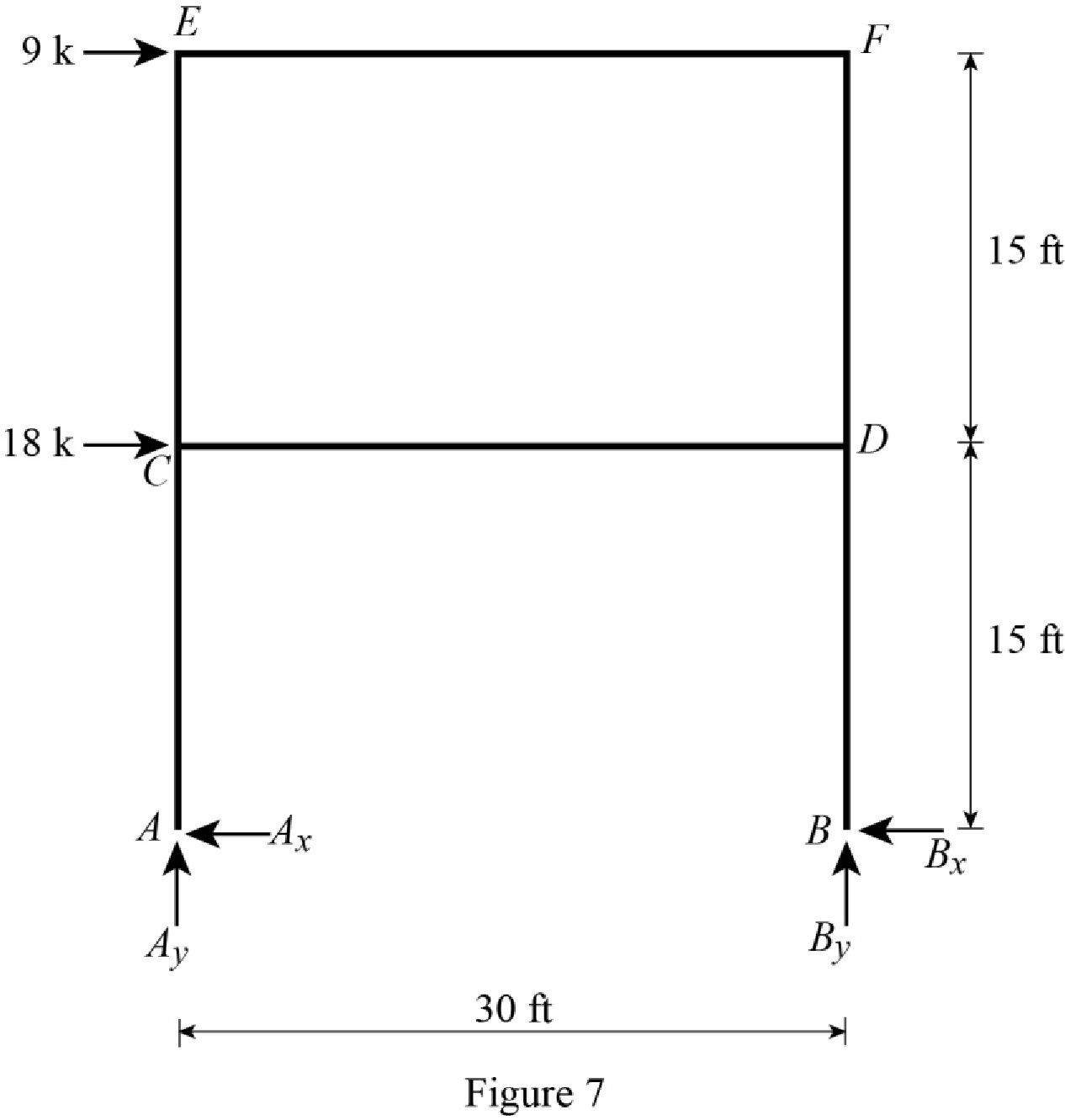
Calculate the reaction at joint A:
Calculate the reaction at joint B:
Consider member AC:
Calculate the horizontal reaction at the joint A by taking moment about point C.
Consider member BD:
Calculate the horizontal reaction at the joint B by taking moment about point D.
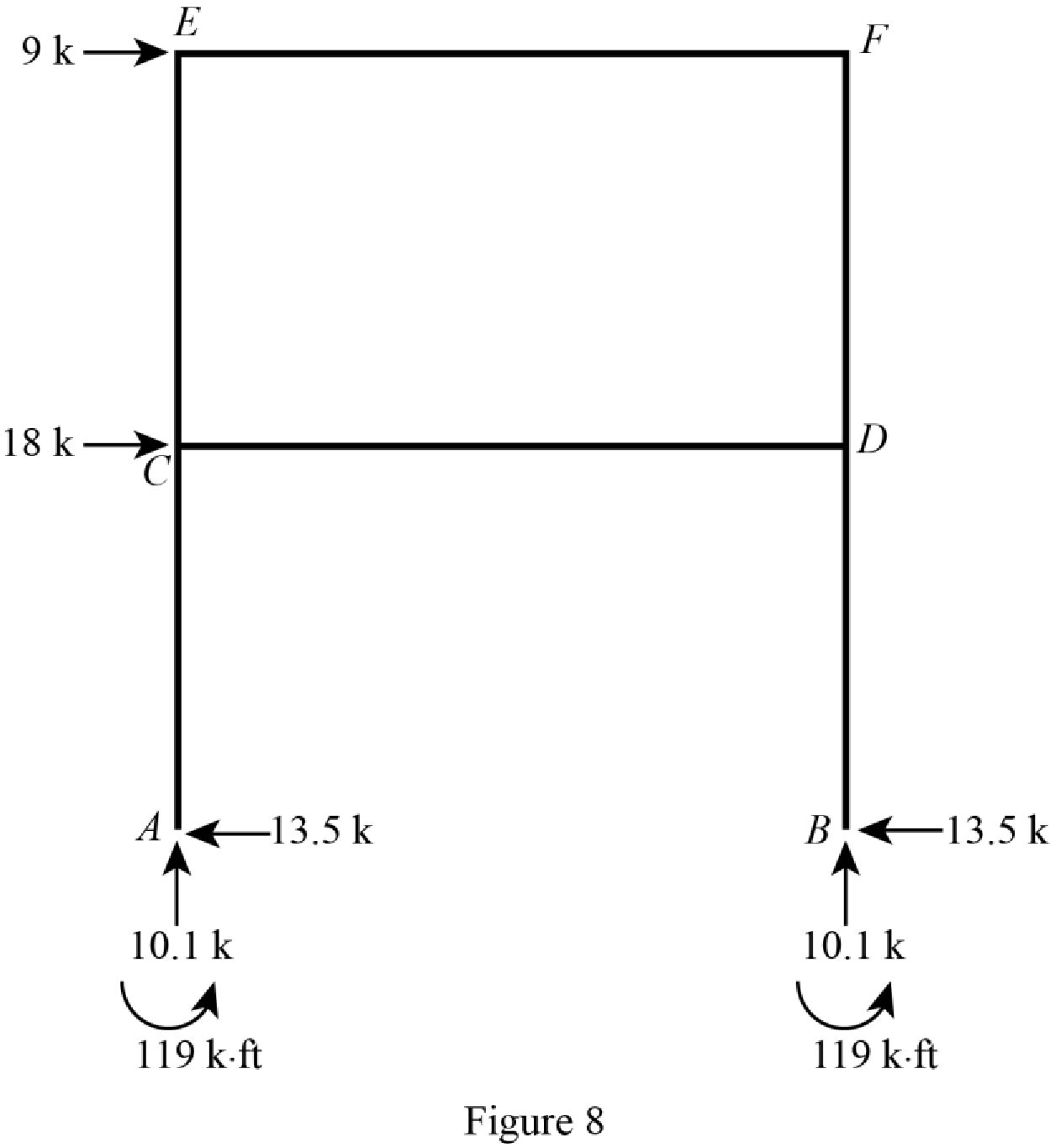
Show the reactions of the frame as in Figure 8.
Want to see more full solutions like this?
Chapter 15 Solutions
Structural Analysis
- 6. A lake with no outlet is fed by a river with a constant flow of 1200 ft3/s. Water evaporates from the surface at a constant rate of 13 ft3/s per square mile of surface area. The surface area varies with the depth h (in feet) as A (square miles) = 4.5 + 5.5h. What is the equilibrium depth of the lake? Below what river discharge (volume flow rate) will the lake dry up?arrow_forwardProblem 5 (A, B, C and D are fixed). Find the reactions at A and D 8 k B 15 ft A -20 ft C 10 ft Darrow_forwardProblem 4 (A, B, E, D and F are all pin connected and C is fixed) Find the reactions at A, D and F 8 m B 6m E 12 kN D F 4 marrow_forward
- Problem 1 (A, C and D are pins) Find the reactions and A, C and D. D 6 m B 12 kN/m 8 m A C 6 marrow_forwardUniform Grade of Pipe Station of Point A is 9+50.00. Elevation Point A = 250.75.Station of Point B is 13+75.00. Elevation Point B = 244.10 1) Calculate flowline of pipe elevations at every 50 ft. interval (Half Station). 2) Tabulate station and elevation for each station like shown on example 3) Draw Sketcharrow_forward40m 150N B 40marrow_forward
- Note: Please accurately answer it!. I'll give it a thumbs up or down based on the answer quality and precision. Question: What is the group name of Sample B in problem 3 from the image?. By also using the ASTM flow chart!. This unit is soil mechanics btwarrow_forwardPick the rural location of a project site in Victoria, and its catchment area-not bigger than 25 sqkm, and given the below information, determine the rainfall intensity for ARI = 5, 50, 100 year storm event. Show all the details of the procedure. Each student must propose different length of streams and elevations. Use fig below as a sample only. Pt. E-ht. 95.0 200m 600m PLD-M. 91.0 300m Pt. C-93.0 300m PL.B-ht. 92.0 PL.F-ht. 96.0 500m Pt. A-M. 91.00 To be deemed satisfactory the solution must include: Q.F1.1.Choice of catchment location Q.F1.2. A sketch displaying length of stream and elevation Q.F1.3. Catchment's IFD obtained from the Buro of Metheorology for specified ARI Q.F1.4.Calculation of the time of concentration-this must include a detailed determination of the equivalent slope. Q.F1.5.Use must be made of the Bransby-Williams method for the determination of the equivalent slope. Q.F1.6.The graphical display of the estimation of intensities for ARI 5,50, 100 must be shown.arrow_forwardQUANTITY SURVEYINGarrow_forward
