Electronics Fundamentals: Circuits, Devices & Applications
8th Edition
ISBN: 9780135072950
Author: Thomas L. Floyd, David Buchla
Publisher: Prentice Hall
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 15, Problem 1TFQ
Transient time for an RC circuit is the same as the time constant.
Expert Solution & Answer
To determine
To check: Whether the given statement is true or false.
Answer to Problem 1TFQ
The given statement is
Explanation of Solution
A given circuit is a RC integrator circuit. Consider the circuit of an RC integrator shown below.
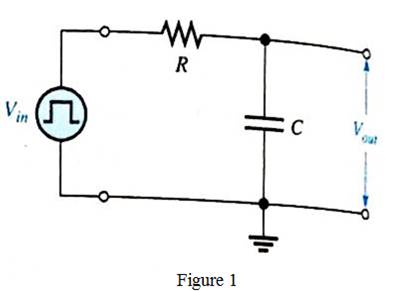
Here, input
In an RC circuit, the time taken for a capacitor to get fully charged or discharged is called transient time and it is expressed as 5 times the time constant of the circuit. The time constant of the circuit for a given value of resistance and capacitance is given by,
Hence, the transient time,
Thus, transient time and time constant are not the same.
Want to see more full solutions like this?
Subscribe now to access step-by-step solutions to millions of textbook problems written by subject matter experts!
Students have asked these similar questions
8.24 In the circuit of Fig. P8.24, is(t) = 0.2sin105t A,R = 20 W, L = 0.1 mH, and C = 2 μF. Show that the sum ofthe complex powers for the three passive elements is equal to thecomplex power of the source.
3. VEB (on) 0.7 V, VEC (sat) = 0.2 V, and ẞ = 150. RB = 50 kQ, Rc = 2 kQ, and Vcc =
5 V.
a) Find the range of V₁ for the cut-off. Forward active, and saturation regions. (20
points)
b) Draw the voltage transfer characteristic (VTC) graph. (10 points)
Vcc
VEB
V₁
RB
www
。 Vo
Rc
Figure 3
2-1) Lathi & Ding prob. 2.5-2 For the signals y(t) and x(t) shown below, find the component of the form
y(t) contained in x(t). In other words, find the optimum value of c in the approximation x(t) = cy(t) so that
the error signal energy is minimum. Also compute the error signal energy.
y(t)
x(t)
0
1
0
1
Chapter 15 Solutions
Electronics Fundamentals: Circuits, Devices & Applications
Ch. 15 - Transient time for an RC circuit is the same as...Ch. 15 - Prob. 2TFQCh. 15 - If the source voltage is increased in a pulsed RC...Ch. 15 - Prob. 4TFQCh. 15 - Prob. 5TFQCh. 15 - Prob. 6TFQCh. 15 - Prob. 7TFQCh. 15 - Prob. 8TFQCh. 15 - Prob. 9TFQCh. 15 - Prob. 10TFQ
Ch. 15 - Prob. 1STCh. 15 - Prob. 2STCh. 15 - Prob. 3STCh. 15 - Prob. 4STCh. 15 - In an RC differentiator, the output pulse closely...Ch. 15 - Prob. 6STCh. 15 - Prob. 7STCh. 15 - Prob. 8STCh. 15 - Prob. 9STCh. 15 - Prob. 10STCh. 15 - Prob. 1PCh. 15 - Determine how long it takes the capacitor in an...Ch. 15 - Prob. 3PCh. 15 - Prob. 4PCh. 15 - Prob. 5PCh. 15 - Prob. 6PCh. 15 - Show the approximate shape of an integrator output...Ch. 15 - Prob. 8PCh. 15 - Prob. 9PCh. 15 - Prob. 10PCh. 15 - Prob. 11PCh. 15 - Prob. 12PCh. 15 - Prob. 13PCh. 15 - Draw the differentiator output in Figure 15-59....Ch. 15 - Prob. 15PCh. 15 - Prob. 16PCh. 15 - Prob. 17PCh. 15 - Prob. 18PCh. 15 - Prob. 19PCh. 15 - Prob. 20PCh. 15 - Ideally, what is the output of an RC integrator...Ch. 15 - Prob. 22PCh. 15 - Prob. 23PCh. 15 - Prob. 24PCh. 15 - Prob. 25PCh. 15 - Prob. 26PCh. 15 - Prob. 27PCh. 15 - Draw the schematic for the circuit in Figure 15-69...Ch. 15 - Prob. 29PCh. 15 - Prob. 30PCh. 15 - Prob. 31PCh. 15 - Prob. 32P
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, electrical-engineering and related others by exploring similar questions and additional content below.Similar questions
- 1. Is1 = 2ls2 = 4 × 10-16 A, B₁ = ẞ2 = 100, and R₁ = 5 kQ. Find the VB such that lx = 1 mA. (30 points) R1 ww Q2 + VB Figure 1arrow_forward2-2) Lathi & Ding prob. 2.6-1 2.6-1 Find the correlation coefficient p between of signal x(t) and each of the four pulses g1(1), 82(1), 83(1), and g4(f) shown in Fig. P2.6-1. To provide maximum margin against the noise along the transmission path, which pair of pulses would you select for a binary communication? Figure P.2.6-1 x(f) (a) 8(1) (b) 82(1) (c) 1 1 sin 2πt sin 4πt -sin 2 0 0.707 83(1) 0 1 (d) 0 M P 0.707 84(1) (e) 0 0.5 -0.707arrow_forward2. Determine the operation point and the small-signal model of Q₁ for each of the circuits shown in Fig. 2. Assume Is = 8 × 10-16 A, B = 100 and VA = ∞. a) 20 points b) 20 points 0.8 V RC 50 Ω + Vcc = 2.5 V 4A" Figure 2-a Rc1kQ + Vcc = 2.5 V Figure 2-barrow_forward
- Please explain in detail how to solve this question. Include steps with calculations and theory. thank youarrow_forwardFinding crystallographic direction Z pt. 2 head pt. 1: ៩ Example 2: pt. 1 x₁ = a, y₁ = b/2, z₁ = 0 pt. 2 x2=-a, y₂ = b, Z₂ = c -a-a b-b/2 c-0 a b c tail => -2, 1/2, 1 Multiplying by 2 to eliminate the fraction -4,1,2 => [412] where the overbar represents a negative index families of directionsarrow_forwardCrystallographic planes Crystallographic planes are denoted by Miller indices. 5b Algorithm for Miller indices 1. Read off intercepts of plane with axes in terms of a, b, c 2. Take reciprocals of intercepts 3. Reduce to smallest integer values 4. Enclose in parentheses, no commas. 353 1/3 1/5 1/3 535 (535) In the cubic system, a plane and a direction with the same indices are orthogonal. E.g. [100] direction is perpendicular to (100) plane. Correspondingly, [123] direction is perpendicular to (123) plane. [2,3,3] Plane intercepts axes at 3a, 2b, 2c 2 11 1 Reciprocal numbers are: 3'2'2 b. Indices of the plane (Miller): (2,3,3) 2 a Indices of the direction: [2,3,3] X (200) (100) (110) (111) (100) Indices of crystallographic plane can be found from cross product of indices of any two non-parallel directions in this plane.arrow_forward
- Crystallographic positions Crystallographic position is denoted by three numbers, which are coefficients of the position vector, e.g. ½½½ for the red atom. Here the 'new' atom is at a/2 + b/2 + c/2 Silicon crystal has so-called "diamond type lattice". Each Si atom has 4 nearest neighbors. Diamond lattice starts with a FCC lattice and then adds four additional INTERNAL atoms at locations r = a/4+b/4+c/4 away from each of the atoms. In other words, diamond lattice is formed by two FCC lattices sifted by the vector r.arrow_forwardfind the answers for this prelabarrow_forwardQ2: (30 Marks) Design a DC/DC converter that produce output waveforms that shown in figures below from a fixed DC source of 20 volts. Vo (Volt) 14.1 IL (Amp) 13.9 2.25 1.75 † (msec) Output voltage 0.18 0.2 t (msec) L 0.214 0.22 Output currentarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Electricity for Refrigeration, Heating, and Air C...Mechanical EngineeringISBN:9781337399128Author:Russell E. SmithPublisher:Cengage Learning
Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning
Electricity for Refrigeration, Heating, and Air C...
Mechanical Engineering
ISBN:9781337399128
Author:Russell E. Smith
Publisher:Cengage Learning
Delmar's Standard Textbook Of Electricity
Electrical Engineering
ISBN:9781337900348
Author:Stephen L. Herman
Publisher:Cengage Learning
02 - Sinusoidal AC Voltage Sources in Circuits, Part 1; Author: Math and Science;https://www.youtube.com/watch?v=8zMiIHVMfaw;License: Standard Youtube License