
Concept explainers
Find the reaction and plot the shear and bending moment diagram.
Explanation of Solution
Fixed end moment:
Formula to calculate the fixed moment for point load with equal length are
Formula to calculate the fixed moment for point load with unequal length are
Formula to calculate the fixed moment for UDL is
Calculation:
Consider flexural rigidity EI of the beam is constant.
Show the free body diagram of the entire beam as in Figure 1.
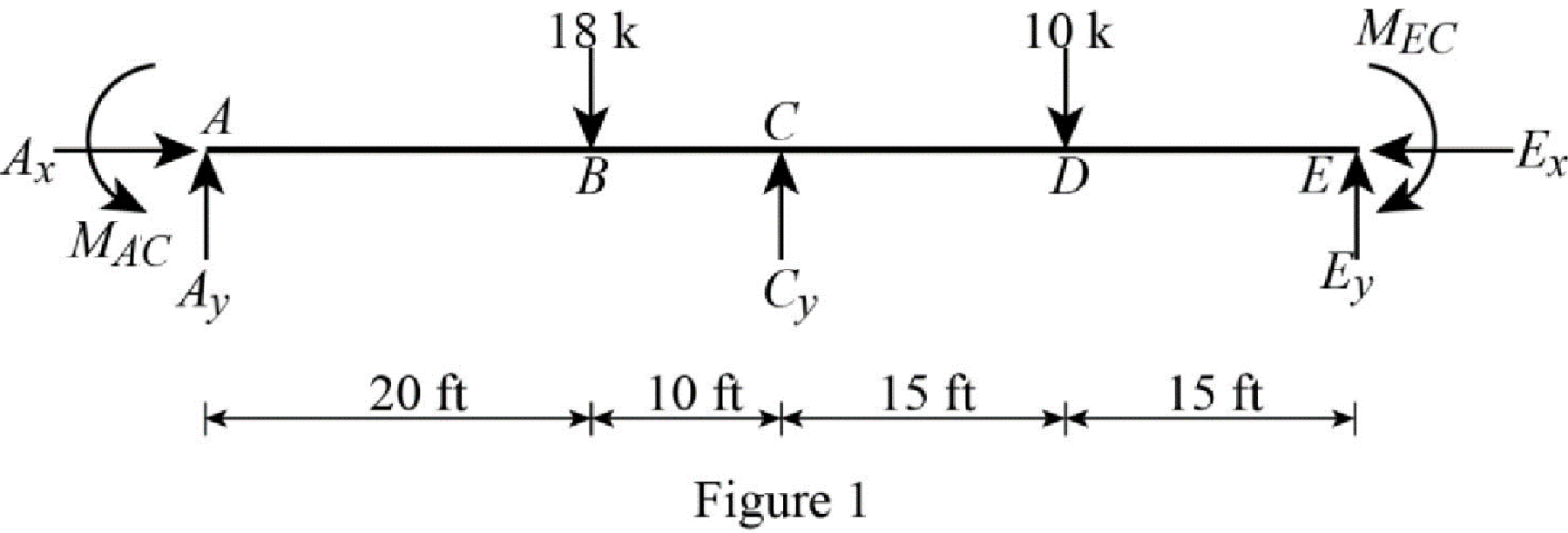
Refer Figure 1,
Calculate the fixed end moment for AC.
Calculate the fixed end moment for CA.
Calculate the fixed end moment for CE.
Calculate the fixed end moment for EC.
Calculate the slope deflection equation for the member AC.
Here,
Substitute 0 for
Calculate the slope deflection equation for the member CA.
Substitute 0 for
Calculate the slope deflection equation for the member CE.
Substitute 0 for
Calculate the slope deflection equation for the member EC.
Substitute 0 for
Write the equilibrium equation as below.
Substitute equation (2) and equation (3) in above equation.
Calculate the moment about AC.
Substitute
Calculate the moment about CA.
Substitute
Calculate the moment about CE.
Substitute
Calculate the moment about EC.
Substitute
Consider the member AC of the beam:
Show the free body diagram of the member AC as in Figure 2.
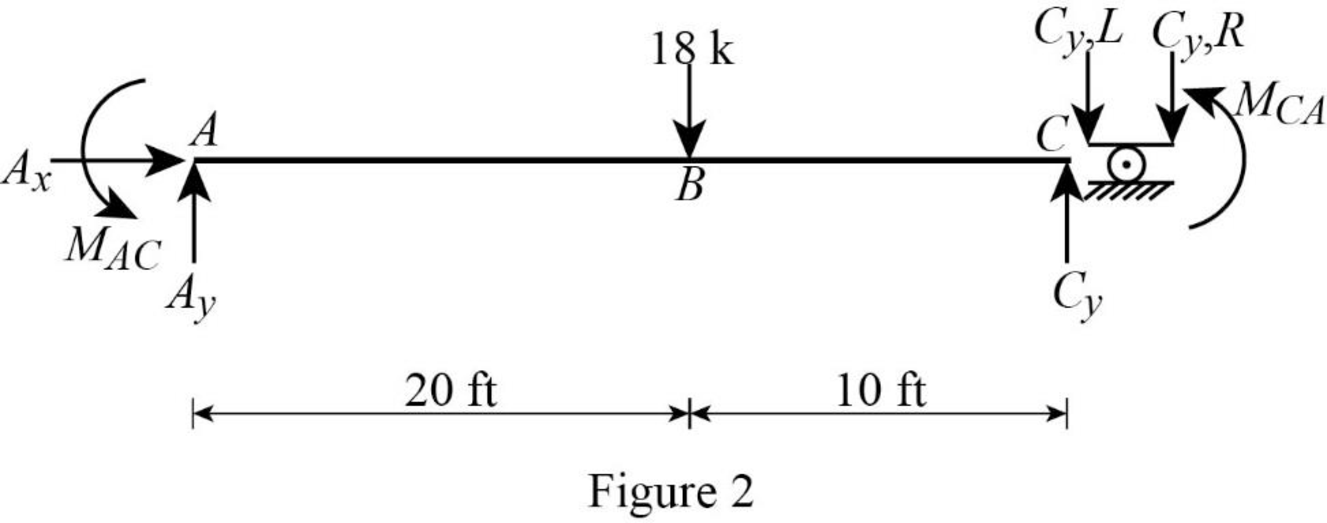
Calculate the vertical reaction at the left end of the joint C by taking moment about point A.
Calculate the horizontal reaction at point A by resolving the horizontal equilibrium.
Calculate the vertical reaction at point A by resolving the vertical equilibrium.
Consider the member CE of the beam:
Show the free body diagram of the member CE as in Figure 2.
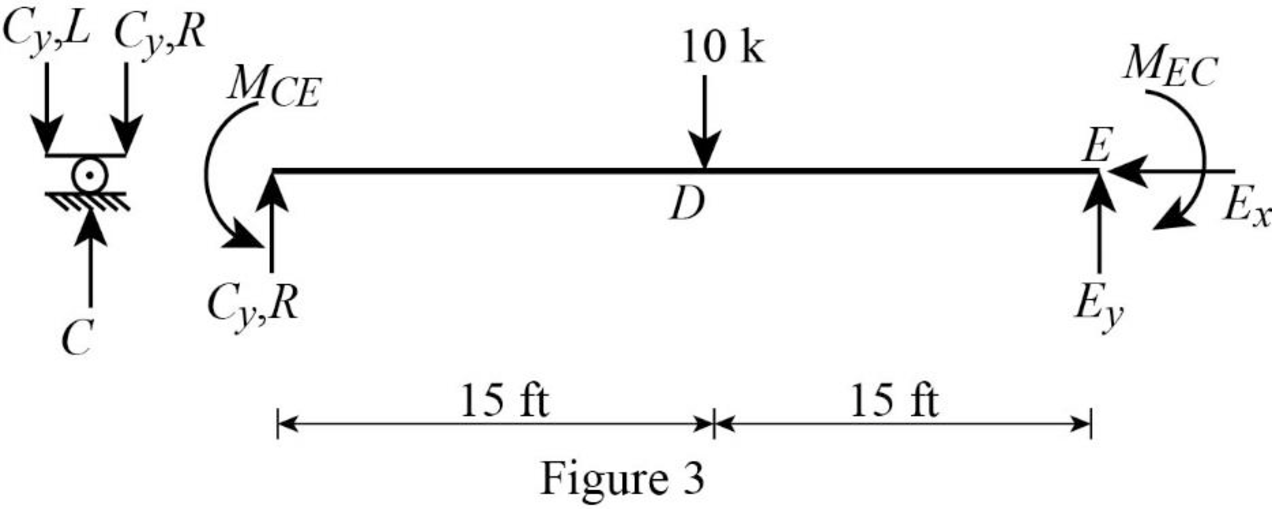
Calculate the vertical reaction at the right end of the joint C by taking moment about point E.
Calculate the horizontal reaction at point E by resolving the horizontal equilibrium.
Calculate the vertical reaction at point E by resolving the vertical equilibrium.
Calculate the total reaction at point C .
Substitute
Show the reaction of the beam in Figure 4.
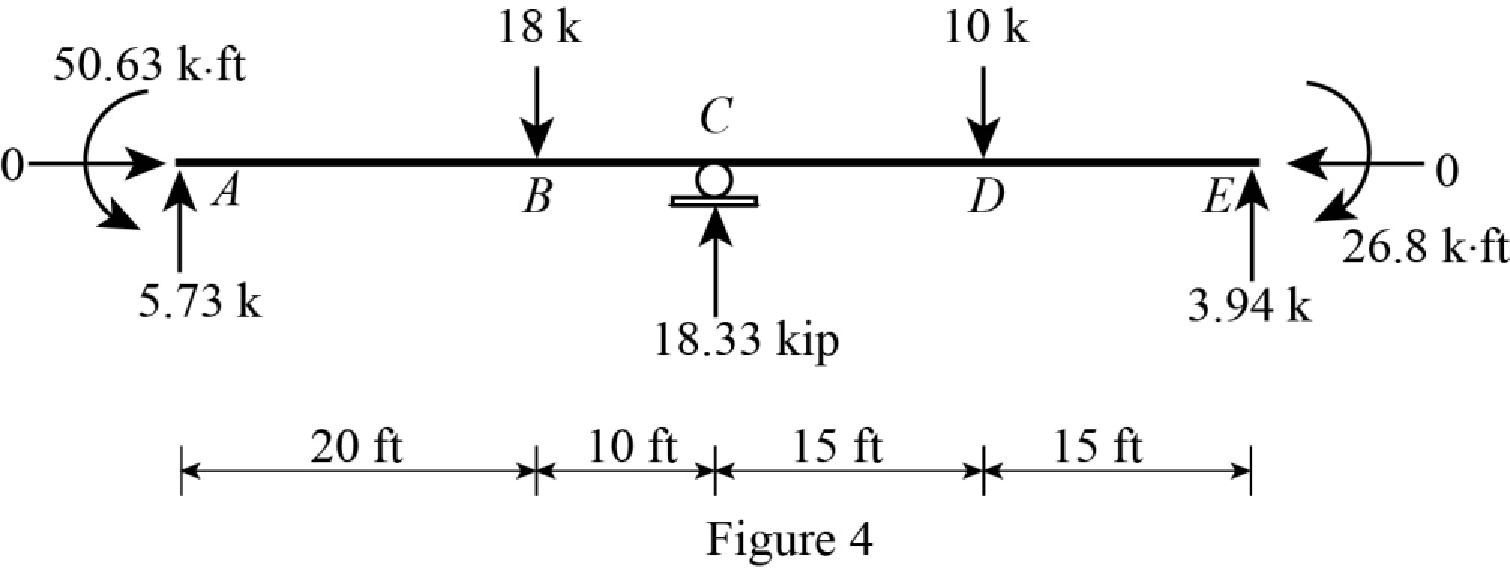
Refer Figure 4,
Shear diagram:
Point A:
Point B:
Point C:
Point D:
Point E:
Plot the shear force diagram of the beam as in Figure 5.
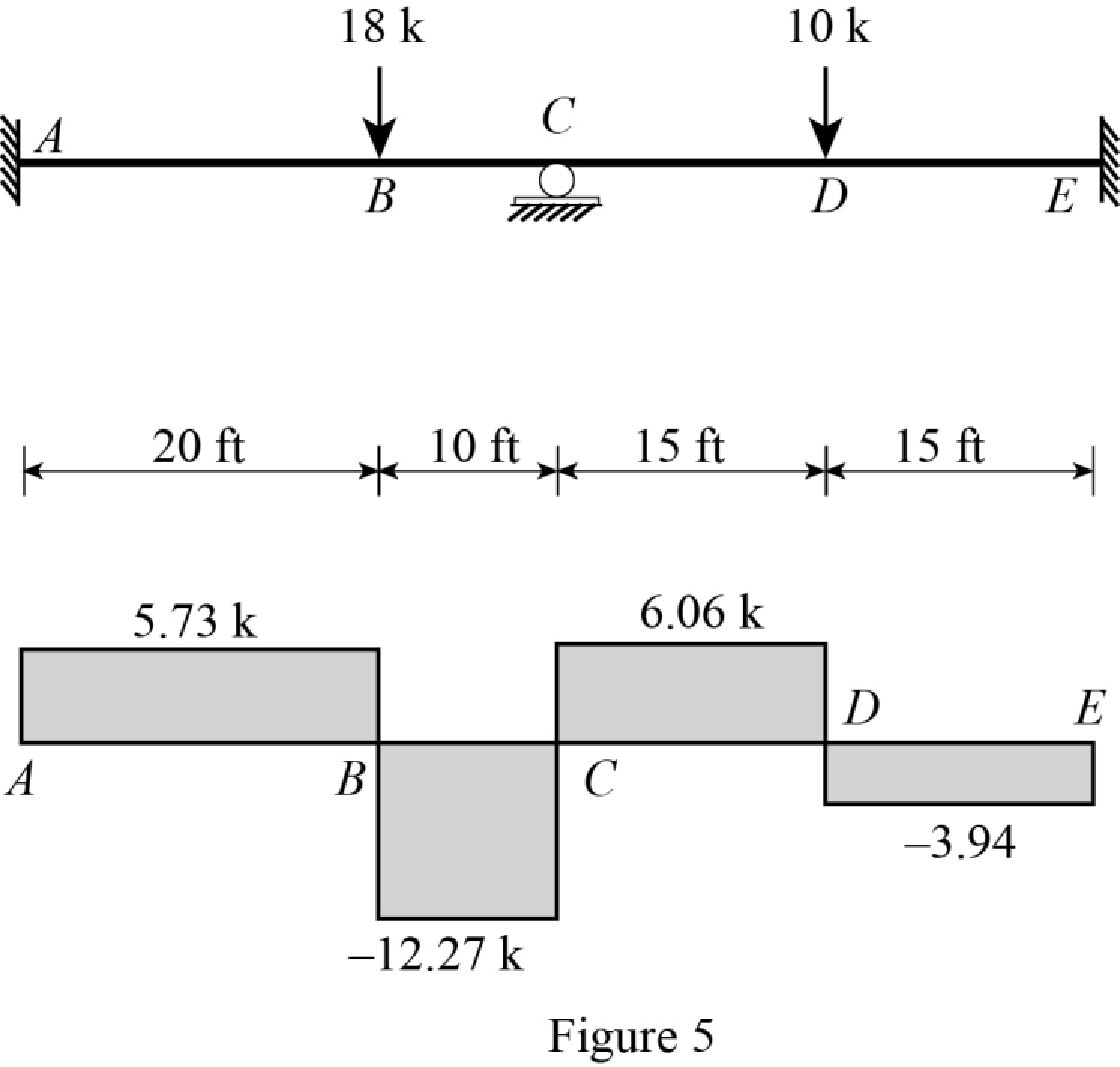
Refer Figure 4,
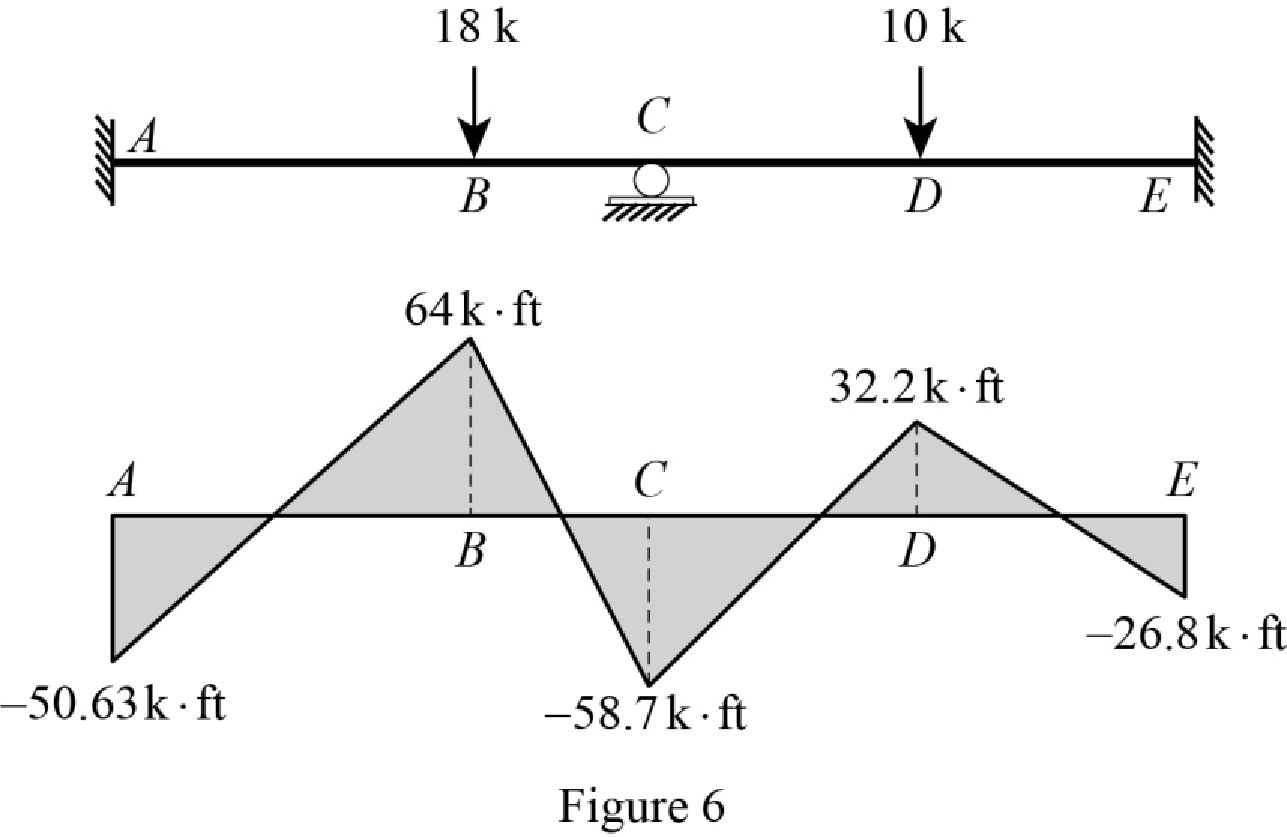
Bending moment diagram:
Point A:
Point B:
Point C:
Point D:
Point E:
Plot the bending moment diagram of the beam as in Figure 6.
Want to see more full solutions like this?
Chapter 15 Solutions
Structural Analysis, SI Edition
- For the beam shown, where should a uniformly distributed downward live load, wl, be placed to cause the maximum upward reaction at support A? (D, E, and F are the supports, from left to right, to the right of C.) B C a. From A to B and D to E b. From A to D and E to F c. From B to D and E to F ☑ d. From B to C and D to E L Larrow_forwardQuestion 1 1. A solid shaft of 1.55 m is used as a tractor propeller (prop) shaft. The shaft twists through 1.8 while rotating at 900 rpm. The diameter of the shaft is 60 mm and the modulus of rigidity is 85 GPa. Calculate 1.1 The maximum shear stress in the shaft 1.2 The power transmitted by the shaft. 2. The tractor has undergone minor modifications to increase the transmitted power by 20%. The solid shaft is replaced by a lighter hollow shaft of the same material, with a dimeter ratio of 2:1. Calculate the suitable dimeters of the hollow shaft.arrow_forwardUse Area Moment Method of Beam Deflectionsarrow_forward
- Determine rotations at all the nodes of the beam and reactions at the supports. Assume support 1 and 3 are roller and support 2 is pinned, L1=1.25m, L2=3.75m and w=60kN/m. Please show all working and FBD's where relevant.arrow_forwardspecific gravity of the soil is 1.41 percentage of water content by weight at field capacity and wilting point are 15% and 7% respectively calculate the equivalent moisture content as equivalent depth for 1.2m root zone: A. At permanent wilting point B. At field capacity C. For ready available water Ex 4-3: If the crop consumptive use in Ex(4-2) is 18 m³/donum/day,calculate: A. Irrigation interval in days B. Field water duty assuming 40% losses during irrigation C. Amount of added water to irrigate one donum. n: CFc- IWC) * As *D Lg dn - Q.te A date. A d9 Q IWC- 20% FC-271 Qg-40e D=100cm sec area of the field. Ed=65% 1 hectare As 1.3arrow_forward۰۹:۲۵ ZV9 HW2-C.pdf Dept: Civil Eng. Kerbala HW2 3rd Year (C) Ex 4-1: The field capacity and wilting point as depth equivalent of soil are 178mm and 102 mm respectively. PAD-60% Calculate the irrigation interval if the water consumptive use is 4.5 mm/day. Ex 4-2: The specific gravity of the soil is 1.41 percentage of water content by weight at field capacity and wilting point are 15% and 7% respectively. a) calculate the equivalent depth of moisture content for 1.2m root zone and PAD 70% at permanent wilting point, at field capacity and for readily available water b) If the crop consumptive use is 18 m/donum/day, calculate: Irrigation interval in days, field water duty assuming 40% losses during irrigation and amount of added water to irrigate one hectare. Ex4-3: Using Blaney-Criddle formula, calculate the irrigation interval through specific interval of plant growth according to the following data: . Mean air temperature 25 C percentage of day light hour through the month 8.4% Crop…arrow_forward
- Determine the global stiffness matrix of the beam shown in Fig. 3. Assume supports at 1 and 3 are rollers and the support at 2 is a pinned support. Indicate the degrees of freedom in all the stiffness matrices. EI is constant, w=60kN/m, L1=1.25m and L2=3.75m please explain how the code numbers for global matrix are determined in detailarrow_forwardFor the truss shown in Fig 2, determine the nodal displacement and member forces for all elements of the truss. Assume for each member A = 0.0015 m2 and E = 200 GPa please show all working, relevant FBD's and use ID's indicated in the diagram, if using an alpha numerical in equations please indicate where it is being applied to in the truss.arrow_forwardDetermine the global stiffness matrix of the beam shown in Fig. 3. Assume supports at 1 and 3 are rollers and the support at 2 is a pinned support. Indicate the degrees of freedom in all the stiffness matrices. EI is constant, w=60kN/m, L1=1.25m and L2=3.45m please explain how the code numbers for global matrix are determined in detailarrow_forward
