Mathematical Methods in the Physical Sciences
3rd Edition
ISBN: 9780471198260
Author: Mary L. Boas
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 14.3, Problem 6P
Evaluate the following line integrals in the complex plane by direct
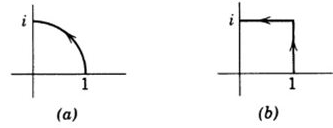
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Refer to page 145 for problems on constrained optimization.
Instructions:
•
Solve an optimization problem with constraints using the method of Lagrange multipliers.
•
•
Interpret the significance of the Lagrange multipliers in the given context.
Discuss the applications of this method in machine learning or operations research.
Link: [https://drive.google.com/file/d/1wKSrun-GlxirS31Z9qo Hazb9tC440 AZF/view?usp=sharing]
Only 100% sure experts solve it correct complete solutions ok
Give an example of a graph with at least 3 vertices that has exactly 2 automorphisms(one of which is necessarily the identity automorphism). Prove that your example iscorrect.
Chapter 14 Solutions
Mathematical Methods in the Physical Sciences
Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...
Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.1 - Find the real and imaginary parts u(x,y) and...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21 . Use the Cauchy-Riemann conditions to...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - 1 to 21. Use the Cauchy-Riemann conditions to find...Ch. 14.2 - Using the definition (2.1) of (d/dz)f(z), show...Ch. 14.2 - Using the definition (2.1) of (d/dz)f(z), show...Ch. 14.2 - Prob. 27PCh. 14.2 - Using the definition (2.1) of (d/dz)f(z), show...Ch. 14.2 - Problem 28 is the chain rule for the derivative of...Ch. 14.2 - Problem 28 is the chain rule for the derivative of...Ch. 14.2 - Problem 28 is the chain rule for the derivative of...Ch. 14.2 - Using the definition of ez by its power series...Ch. 14.2 - Using the definitions of sin...Ch. 14.2 - Using series you know from Chapter 1, write the...Ch. 14.2 - Using series you know from Chapter 1, write the...Ch. 14.2 - Using series you know from Chapter 1, write the...Ch. 14.2 - Using series you know from Chapter 1, write the...Ch. 14.2 - Using series you know from Chapter 1, write the...Ch. 14.2 - Using series you know from Chapter 1, write the...Ch. 14.2 - Using series you know from Chapter 1, write the...Ch. 14.2 - Using series you know from Chapter 1, write the...Ch. 14.2 - Using series you know from Chapter 1, write the...Ch. 14.2 - In Chapter 12, equations (5.1) and (5.2), we...Ch. 14.2 - Prob. 44PCh. 14.2 - Prob. 45PCh. 14.2 - Prob. 46PCh. 14.2 - Prob. 47PCh. 14.2 - Using polar coordinates (Problem 46), find out...Ch. 14.2 - Prob. 49PCh. 14.2 - Using polar coordinates (Problem 46), find out...Ch. 14.2 - Prob. 51PCh. 14.2 - Prob. 52PCh. 14.2 - Using polar coordinates (Problem 46), find out...Ch. 14.2 - Show that the following functions are harmonic,...Ch. 14.2 - Show that the following functions are harmonic,...Ch. 14.2 - Show that the following functions are harmonic,...Ch. 14.2 - Show that the following functions are harmonic,...Ch. 14.2 - Show that the following functions are harmonic,...Ch. 14.2 - Show that the following functions are harmonic,...Ch. 14.2 - Show that the following functions are harmonic,...Ch. 14.2 - Show that the following functions are harmonic,...Ch. 14.2 - Show that the following functions are harmonic,...Ch. 14.2 - Show that the following functions are harmonic,...Ch. 14.2 - It can be shown that, if u(x,y) is a harmonic...Ch. 14.3 - Evaluate the following line integrals in the...Ch. 14.3 - Evaluate the following line integrals in the...Ch. 14.3 - Evaluate the following line integrals in the...Ch. 14.3 - Evaluate the following line integrals in the...Ch. 14.3 - Evaluate the following line integrals in the...Ch. 14.3 - Evaluate the following line integrals in the...Ch. 14.3 - Evaluate the following line integrals in the...Ch. 14.3 - Evaluate the following line integrals in the...Ch. 14.3 - Evaluate the following line integrals in the...Ch. 14.3 - Evaluate the following line integrals in the...Ch. 14.3 - Evaluate C(z3)dz where C is the indicated closed...Ch. 14.3 - 01+2iz2dz along the indicated paths:Ch. 14.3 - In Chapter 6, Section 11, we showed that a...Ch. 14.3 - In finding complex Fourier series in Chapter 7, we...Ch. 14.3 - If f(z) is analytic on and inside the circle z=1,...Ch. 14.3 - If f(z) is analytic in the disk z2, evaluate...Ch. 14.3 - Use Cauchys theorem or integral formula to...Ch. 14.3 - Use Cauchys theorem or integral formula to...Ch. 14.3 - Use Cauchys theorem or integral formula to...Ch. 14.3 - Use Cauchys theorem or integral formula to...Ch. 14.3 - Differentiate Cauchys formula (3.9) or (3.10) to...Ch. 14.3 - Use Problem 21 to evaluate the following...Ch. 14.3 - Use Problem 21 to evaluate the following...Ch. 14.3 - Use Problem 21 to evaluate the following...Ch. 14.4 - Show that the sum of a power series which...Ch. 14.4 - Show that equation ( 4.4 ) can be written as...Ch. 14.4 - For each of the following functions find the first...Ch. 14.4 - For each of the following functions find the first...Ch. 14.4 - For each of the following functions find the first...Ch. 14.4 - For each of the following functions find the first...Ch. 14.4 - For each of the following functions find the first...Ch. 14.4 - For each of the following functions find the first...Ch. 14.4 - For each of the following functions, say whether...Ch. 14.4 - For each of the following functions, say whether...Ch. 14.4 - For each of the following functions, say whether...Ch. 14.4 - For each of the following functions, say whether...Ch. 14.5 - If C is a circle of radius about z0, show that...Ch. 14.5 - Verify the formulas (4.3) for the coefficients in...Ch. 14.5 - Obtain Cauchys integral formula ( 3.9 ) from the...Ch. 14.6 - Find the Laurent series for the following...Ch. 14.6 - Find the Laurent series for the following...Ch. 14.6 - Find the Laurent series for the following...Ch. 14.6 - Find the Laurent series for the following...Ch. 14.6 - Find the Laurent series for the following...Ch. 14.6 - Find the Laurent series for the following...Ch. 14.6 - Find the Laurent series for the following...Ch. 14.6 - Find the Laurent series for the following...Ch. 14.6 - Find the Laurent series for the following...Ch. 14.6 - Show that rule B is correct by applying it to...Ch. 14.6 - Derive (6.2) by using the limit definition of the...Ch. 14.6 - Prove rule C for finding the residue at a multiple...Ch. 14.6 - Prove rule C by using (3.9). Hints: If f(z) has a...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Prob. 33PCh. 14.6 - Find the residues of the following functions at...Ch. 14.6 - Find the residues of the following functions at...Ch. 14.6 - For complex z,Jp(z) can be defined by the series...Ch. 14.6 - The gamma function (z) is analytic except for...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - The values of the following integrals are known...Ch. 14.7 - In Example 4 we stated a rule for evaluating a...Ch. 14.7 - Using the rule of Example 4 (also see problem 21),...Ch. 14.7 - Using the rule of Example 4 (also see problem 21),...Ch. 14.7 - Using the rule of Example 4 (also see problem 21),...Ch. 14.7 - Using the rule of Example 4 (also see problem 21),...Ch. 14.7 - Using the rule of Example 4 (also see problem 21),...Ch. 14.7 - Using the rule of Example 4 (also see problem 21),...Ch. 14.7 - Using the rule of Example 4 (also see problem 21),...Ch. 14.7 - Using the rule of Example 4 (also see problem 21),...Ch. 14.7 - (a) By the method of Example 2 evaluate 0dx1+x4....Ch. 14.7 - Use the method of Problem 30(c) to evaluate...Ch. 14.7 - Use the method of Problem 30(c) and the contour...Ch. 14.7 - Evaluate the following integrals by the method of...Ch. 14.7 - Evaluate the following integrals by the method of...Ch. 14.7 - Evaluate the following integrals by the method of...Ch. 14.7 - Evaluate the following integrals by the method of...Ch. 14.7 - (a) Show that epx1+exdx=sinp for 0p1. Hint: Find...Ch. 14.7 - Using the same contour and method as in Problem...Ch. 14.7 - Evaluate e2x/3coshxdx. Hint: Use a rectangle as in...Ch. 14.7 - Evaluate 0xdxsinhx. Hint: First find the to ...Ch. 14.7 - The Fresnel integrals, 0usinu2du and 0ucosu2du,...Ch. 14.7 - If F(z)=f(z)/f(z) (a) show that the residue of...Ch. 14.7 - By using theorem (7.8), show that z3+z2+9=0 has...Ch. 14.7 - The fundamental theorem of algebra says that every...Ch. 14.7 - As in Problem 43 find out in which quadrants the...Ch. 14.7 - As in Problem 43 find out in which quadrants the...Ch. 14.7 - As in Problem 43 find out in which quadrants the...Ch. 14.7 - As in Problem 43 find out in which quadrants the...Ch. 14.7 - As in Problem 43 find out in which quadrants the...Ch. 14.7 - As in Problem 43 find out in which quadrants the...Ch. 14.7 - Use (7.8) to evaluate...Ch. 14.7 - Use (7.8) to evaluate z3dz1+2z4 around z=1.Ch. 14.7 - Use (7.8) to evaluate z3+4zz4+8z2+16dz around the...Ch. 14.7 - Use (7.8) to evaluate Csec2(z/4)dz1tan(z/4), where...Ch. 14.7 - Find the inverse Laplace transform of the...Ch. 14.7 - Find the inverse Laplace transform of the...Ch. 14.7 - Find the inverse Laplace transform of the...Ch. 14.7 - Find the inverse Laplace transform of the...Ch. 14.7 - Find the inverse Laplace transform of the...Ch. 14.7 - Find the inverse Laplace transform of the...Ch. 14.7 - Find the inverse Laplace transform of the...Ch. 14.7 - Find the inverse Laplace transform of the...Ch. 14.7 - Find the inverse Laplace transform of the...Ch. 14.7 - Find the inverse Laplace transform of the...Ch. 14.7 - Find the inverse Laplace transform of the...Ch. 14.7 - In equation (7.18), let u(x) be an even function...Ch. 14.8 - Let f(z) be expanded in the Laurent series that is...Ch. 14.8 - (a) Show that if f(z) tends to a finite limit as z...Ch. 14.8 - Find out whether infinity is a regular point, an...Ch. 14.8 - Find out whether infinity is a regular point, an...Ch. 14.8 - Find out whether infinity is a regular point, an...Ch. 14.8 - Find out whether infinity is a regular point, an...Ch. 14.8 - Find out whether infinity is a regular point, an...Ch. 14.8 - Find out whether infinity is a regular point, an...Ch. 14.8 - Find out whether infinity is a regular point, an...Ch. 14.8 - Find out whether infinity is a regular point, an...Ch. 14.8 - Find out whether infinity is a regular point, an...Ch. 14.8 - Find out whether infinity is a regular point, an...Ch. 14.8 - Prob. 13PCh. 14.8 - Evaluate the following integrals by computing...Ch. 14.8 - Evaluate the following integrals by computing...Ch. 14.8 - Observe that in Problems 14 and 15 the sum of the...Ch. 14.9 - In these problems you should be able to make rough...Ch. 14.9 - For each of the following functions w=f(z)=u+iv,...Ch. 14.9 - For each of the following functions w=f(z)=u+iv,...Ch. 14.9 - For each of the following functions w=f(z)=u+iv,...Ch. 14.9 - For each of the following functions w=f(z)=u+iv,...Ch. 14.9 - For each of the following functions w=f(z)=u+iv,...Ch. 14.9 - For each of the following functions w=f(z)=u+iv,...Ch. 14.9 - For each of the following functions w=f(z)=u+iv,...Ch. 14.9 - Describe the Riemann surface for w=z3Ch. 14.9 - Describe the Riemann surface for w=zCh. 14.9 - Describe the Riemann surface for w=lnzCh. 14.9 - If w=f(z)=u(x,y)+iv(x,y),f(z) analytic, defines a...Ch. 14.9 - Verify the matrix equation dudv=Jdxdy, where J is...Ch. 14.9 - We have discussed the fact that a conformal...Ch. 14.9 - Compare the directional derivative...Ch. 14.10 - Prove the theorem stated just after (10.2) as...Ch. 14.10 - Assuming from electricity the equations...Ch. 14.10 - A fluid flow is called irrotational if V=0 where...Ch. 14.10 - Let a flat plate in the shape of a quarter-circle,...Ch. 14.10 - Consider a capacitor made of two very large...Ch. 14.10 - Prob. 6PCh. 14.10 - Use the mapping function w=z2 to find the...Ch. 14.10 - Prob. 8PCh. 14.10 - Find and sketch the streamlines for the flow of...Ch. 14.10 - Find and sketch the streamlines for the indicated...Ch. 14.10 - For w=ln[(z+1)/(z1)], show that the images of u=...Ch. 14.10 - Use the results of Problem 11 to solve the...Ch. 14.10 - Let the figure in Problem 12 represent (the cross...Ch. 14.10 - In the figure in Problem 12, let z=1 be a source...Ch. 14.10 - In Problem 14, the streamlines were the images of...Ch. 14.10 - Two long parallel cylinders form a capacitor. (Let...Ch. 14.11 - In Problems 1 and 2, verify that the given...Ch. 14.11 - In Problems 1 and 2, verify that the given...Ch. 14.11 - Liouvilles theorem: Suppose f(z) is analytic for...Ch. 14.11 - Use Liouvilles theorem (Problem 3 ) to prove the...Ch. 14.11 - In Problems 5 to 8, find the residues of the given...Ch. 14.11 - In Problems 5 to $8,$ find the residues of the...Ch. 14.11 - In Problems 5 to 8, find the residues of the given...Ch. 14.11 - In Problems 5 to $8,$ find the residues of the...Ch. 14.11 - In Problems 9 to 10, use Laurent series to find...Ch. 14.11 - In Problems 9 to $10,$ use Laurent series to find...Ch. 14.11 - Find the Laurent series of f(z)=ez/(1z) for z1 and...Ch. 14.11 - Let f(z) be the branch of z21 which is positive...Ch. 14.11 - In Problems 13 and $14,$ find the residues at the...Ch. 14.11 - In Problems 13 and 14, find the residues at the...Ch. 14.11 - In Problem 15 to 20, evaluate the integrals by...Ch. 14.11 - In Problem 15 to 20, evaluate the integrals by...Ch. 14.11 - In Problem 15 to 20, evaluate the integrals by...Ch. 14.11 - In Problem 15 to $20,$ evaluate the integrals by...Ch. 14.11 - In Problem 15 to 20, evaluate the integrals by...Ch. 14.11 - In Problem 15 to $20,$ evaluate the integrals by...Ch. 14.11 - Verify the formulas in Problem 21 to 27 by contour...Ch. 14.11 - Verify the formulas in Problem 21 to 27 by contour...Ch. 14.11 - Verify the formulas in Problem 21 to 27 by contour...Ch. 14.11 - Verify the formulas in Problem 21 to 27 by contour...Ch. 14.11 - Verify the formulas in Problem 21 to 27 by contour...Ch. 14.11 - Verify the formulas in Problem 21 to 27 by contour...Ch. 14.11 - Verify the formulas in Problem 21 to 27 by contour...Ch. 14.11 - Evaluate 0xlnxdx(1+x)2 by using the contour of...Ch. 14.11 - Evaluate 0(lnx)21+x2dx by using the contour of...Ch. 14.11 - Show that PV0cos(lnx)x2+1dx=2cosh(/2) by...Ch. 14.11 - As in Section 7, find out how many roots the...Ch. 14.11 - As in Section 7, find out how many roots the...Ch. 14.11 - As in Section 7, find out how many roots the...Ch. 14.11 - As in Section 7, find out how many roots the...Ch. 14.11 - Show that the Cauchy-Riemann equations [see (2.2)...Ch. 14.11 - Show that a harmonic function u(x,y) is equal at...Ch. 14.11 - A (nonconstant) harmonic function takes its...Ch. 14.11 - Show that a Dirichlet problem (see Chapter 13,...Ch. 14.11 - Use the following sequence of mappings to find the...Ch. 14.11 - Use L13 of the Laplace transform table to find the...Ch. 14.11 - Evaluate by contour integration 0cos2(/2)122d....
Additional Math Textbook Solutions
Find more solutions based on key concepts
In Exercises 21-24, use these results from the “1-Panel-THC” test for marijuana use, which is provided by the c...
Elementary Statistics (13th Edition)
Derivatives involving ln x Find the following derivatives. 17. ddx((x2+1)lnx)
Calculus: Early Transcendentals (2nd Edition)
Consider two investments, one earning simple interest and one earning compound interest. If both start with the...
Using and Understanding Mathematics: A Quantitative Reasoning Approach (6th Edition)
The equivalent expression of x(y+z) by using the commutative property.
Calculus for Business, Economics, Life Sciences, and Social Sciences (14th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- 3. [10 marks] Let Go (Vo, Eo) and G₁ = (V1, E1) be two graphs that ⚫ have at least 2 vertices each, ⚫are disjoint (i.e., Von V₁ = 0), ⚫ and are both Eulerian. Consider connecting Go and G₁ by adding a set of new edges F, where each new edge has one end in Vo and the other end in V₁. (a) Is it possible to add a set of edges F of the form (x, y) with x € Vo and y = V₁ so that the resulting graph (VUV₁, Eo UE₁ UF) is Eulerian? (b) If so, what is the size of the smallest possible F? Prove that your answers are correct.arrow_forwardLet T be a tree. Prove that if T has a vertex of degree k, then T has at least k leaves.arrow_forwardHomework Let X1, X2, Xn be a random sample from f(x;0) where f(x; 0) = (-), 0 < x < ∞,0 € R Using Basu's theorem, show that Y = min{X} and Z =Σ(XY) are indep. -arrow_forward
- Homework Let X1, X2, Xn be a random sample from f(x; 0) where f(x; 0) = e−(2-0), 0 < x < ∞,0 € R Using Basu's theorem, show that Y = min{X} and Z =Σ(XY) are indep.arrow_forwardrmine the immediate settlement for points A and B shown in figure below knowing that Aq,-200kN/m², E-20000kN/m², u=0.5, Depth of foundation (DF-0), thickness of layer below footing (H)=20m. 4m B 2m 2m A 2m + 2m 4marrow_forwardSolve this pleasearrow_forward
- 13.4. Let f(z) =y-x-3ir² and y be given by the line segment z = 0 to z 1+i. Evaluate. L f(z)dz.arrow_forwardenter | Infinite Camp ilc 8.3 End-of-Unit Assessment, Op x Pride is the Devil - Google Drive x + 2 sdphiladelphia.ilclassroom.com/assignments/7FQ5923/lesson?card=806642 3 Problem 2 A successful music app tracked the number of song downloads each day for a month for 4 music artists, represented by lines l, j, m, and d over the course of a month. Which line represents an artist whose downloads remained constant over the month? Select the correct choice. = Sidebar Tools M 45 song downloads days d 1 2 3 4 5 6 7 8 00 8 m l RA 9 > КУ Fullscreen G Save & Exit De ☆arrow_forwardQ/Determine the set of points at which - f(z) = 622 2≥ - 4i/z12 i and differentiable analytice is:arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Definite Integral Calculus Examples, Integration - Basic Introduction, Practice Problems; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=rCWOdfQ3cwQ;License: Standard YouTube License, CC-BY