Precalculus Enhanced with Graphing Utilities (7th Edition)
7th Edition
ISBN: 9780134119281
Author: Michael Sullivan, Michael Sullivan III
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 14.1, Problem 17SB
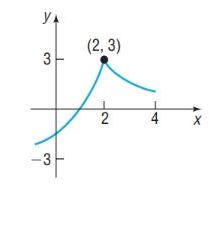
In Problems 17-22, use the graph shown to determine if the limit exists. If it does, find its value.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
The graph below is the function f(x)
-4
-1
lim
x-2+
5-
4
3
2
+
Determine the following values. Enter "DNE" if a value does not exist, enter "oo" (lower case "o") if the limit
approaches positive infinity, or "-oo" if the limit approaches negative infinity.
f(-2)
+
lim f(x) =
x-2-
lim f(x)
x--2
-2
f(x) =
Consider the follovwing graph of the function g.
From the given graph of g, state the numbers at which g is discontinuous. (Enter your answers as a comma-separated list.)
The graph below is the function f(x)
-5 -4 -3 -2
-1
3
2
1
lim f(x) =
$2
f(2)=
lim f(x)
lim f(x) =
1
Determine the following values. Enter "DNE" if a value does not exist, enter "oo" (lower case "o") if the limit
approaches positive infinity, or "-oo" if the limit approaches negative infinity.
200
2 3 4
Question Help: Message instructor D Post to forum
Submit Question
Q Search
Chapter 14 Solutions
Precalculus Enhanced with Graphing Utilities (7th Edition)
Ch. 14.1 - Graph f( x )={ 3x2ifx2 3ifx=2 (pp.100-102)Ch. 14.1 - If f( x )={ xifx0 1ifx0 what is f( 0 ) ?...Ch. 14.1 - The limit of a function f( x ) as x approaches c...Ch. 14.1 - If a function f has no limit as x approaches c ,...Ch. 14.1 - True or False lim xc f( x )=N may be described by...Ch. 14.1 - True or False lim xc f( x ) exists and equals some...Ch. 14.1 - lim x2 ( 4 x 3 )Ch. 14.1 - lim x3 ( 2 x 2 +1 )Ch. 14.1 - lim x0 x+1 x 2 +1Ch. 14.1 - lim x0 2x x 2 +4
Ch. 14.1 - lim x4 x 2 4x x4Ch. 14.1 - lim x3 x 2 9 x 2 3xCh. 14.1 - lim x0 ( e x +1 )Ch. 14.1 - Prob. 14SBCh. 14.1 - lim x0 cosx1 x , x in radiansCh. 14.1 - lim x0 tanx x , x in radiansCh. 14.1 - In Problems 17-22, use the graph shown to...Ch. 14.1 - In Problems 17-22, use the graph shown to...Ch. 14.1 - In Problems 17-22, use the graph shown to...Ch. 14.1 - In Problems 17-22, use the graph shown to...Ch. 14.1 - In Problems 17-22, use the graph shown to...Ch. 14.1 - In Problems 17-22, use the graph shown to...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 23-42, graph each function. Use the...Ch. 14.1 - In Problems 43-48, use a graphing utility to find...Ch. 14.1 - In Problems 43-48, use a graphing utility to find...Ch. 14.1 - In Problems 43-48, use a graphing utility to find...Ch. 14.1 - In Problems 43-48, use a graphing utility to find...Ch. 14.1 - In Problems 43-48, use a graphing utility to find...Ch. 14.1 - In Problems 43-48, use a graphing utility to find...Ch. 14.1 - Problems 49-52 are based on material learned...Ch. 14.1 - Problems 49-52 are based on material learned...Ch. 14.1 - Problems 49-52 are based on material learned...Ch. 14.1 - Problems 49-52 are based on material learned...Ch. 14.2 - The limit of the product of two functions equals...Ch. 14.2 - lim xc b= _____Ch. 14.2 - lim xc x= a. x b. c c. cx d. x cCh. 14.2 - True or False The limit of a polynomial function...Ch. 14.2 - True or False The limit of a rational function at...Ch. 14.2 - True or false The limit of a quotient equals the...Ch. 14.2 - In Problems 7- 42, find each limit algebraically....Ch. 14.2 - In Problems 7- 42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 7-42, find each limit algebraically....Ch. 14.2 - In Problems 43-52, find the limit as x approaches...Ch. 14.2 - In Problems 43-52, find the limit as x approaches...Ch. 14.2 - In Problems 43-52, find the limit as x approaches...Ch. 14.2 - In Problems 43-52, find the limit as x approaches...Ch. 14.2 - In Problems 43-52, find the limit as x approaches...Ch. 14.2 - In Problems 43-52, find the limit as x approaches...Ch. 14.2 - In Problems 43-52, find the limit as x approaches...Ch. 14.2 - In Problems 43-52, find the limit as x approaches...Ch. 14.2 - In Problems 43-52, find the limit as x approaches...Ch. 14.2 - In Problems 43-52, find the limit as x approaches...Ch. 14.2 - In problems 53-56, use the properties of limits...Ch. 14.2 - In problems 53-56, use the properties of limits...Ch. 14.2 - In problems 53-56, use the properties of limits...Ch. 14.2 - In problems 53-56, use the properties of limits...Ch. 14.2 - Problems 57-60 are based on material learned...Ch. 14.2 - Problems 57-60 are based on material learned...Ch. 14.2 - Problems 57-60 are based on material learned...Ch. 14.2 - Problems 57-60 are based on material learned...Ch. 14.3 - For the function f( x )={ x 2 ifx0 x+1if0x2...Ch. 14.3 - What are the domain and range of f( x )=lnx ?Ch. 14.3 - True or False The exponential function f( x )= e x...Ch. 14.3 - Name the trigonometric functions that have...Ch. 14.3 - True or False Some rational functions have holes...Ch. 14.3 - True or False Every polynomial function has a...Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - In Problems 7-42, find each limit algebraically....Ch. 14.3 - Find lim x 4 f( x ) .Ch. 14.3 - Find lim x 4 + f( x ) .Ch. 14.3 - Find lim x 2 f( x ) .Ch. 14.3 - Find lim x 2 + f( x ) .Ch. 14.3 - Does lim x4 f( x ) exist? If it does, what is it?Ch. 14.3 - Does lim x0 f( x ) exist? If it does, what is it?Ch. 14.3 - Is f continuous at 4 ?Ch. 14.3 - Is f continuous at 6 ?Ch. 14.3 - Is f continuous at 0?Ch. 14.3 - Is f continuous at 2?Ch. 14.3 - Is f continuous at 4?Ch. 14.3 - Is f continuous at 5?Ch. 14.3 - lim x 1 + ( 2x+3 )Ch. 14.3 - lim x 2 ( 42x )Ch. 14.3 - lim x 1 ( 2 x 3 +5x )Ch. 14.3 - lim x 2 + ( 3 x 2 8 )Ch. 14.3 - lim x/ 2 + sinxCh. 14.3 - lim x ( 3cosx )Ch. 14.3 - lim x 2 + x 2 4 x2Ch. 14.3 - lim x 1 x 3 x x1Ch. 14.3 - lim x 1 x 2 1 x 3 +1Ch. 14.3 - lim x 0 + x 3 x 2 x 4 + x 2Ch. 14.3 - lim x 2 + x 2 +x2 x 2 +2xCh. 14.3 - lim x 4 x 2 +x12 x 2 +4xCh. 14.3 - f( x )= x 3 3 x 2 +2x6c=2Ch. 14.3 - f( x )=3 x 2 6x+5c=3Ch. 14.3 - f( x )= x 2 +5 x6 c=3Ch. 14.3 - f( x )= x 3 8 x 2 +4 c=2Ch. 14.3 - f( x )= x+3 x3 c=3Ch. 14.3 - f( x )= x6 x+6 c=6Ch. 14.3 - f( x )= x 3 +3x x 2 3x c=0Ch. 14.3 - f( x )= x 2 6x x 2 +6x c=0Ch. 14.3 - f( x )={ x 3 +3x x 2 3x ifx0 1ifx=0 c=0Ch. 14.3 - f( x )={ x 2 6x x 2 +6x ifx0 2ifx=0 c=0Ch. 14.3 - f( x )={ x 3 +3x x 2 3x ifx0 1ifx=0 c=0Ch. 14.3 - f( x )={ x 2 6x x 2 +6x ifx0 1ifx=0 c=0Ch. 14.3 - f( x )={ x 3 1 x 2 1 ifx1 2ifx=1 3 x+1 ifx1 c=1Ch. 14.3 - f( x )={ x 2 2x x2 ifx2 2ifx=2 x4 x1 ifx2 c=2Ch. 14.3 - f( x )={ 2 e x ifx0 2ifx=0 x 3 +2 x 2 x 2 ifx0 c=0Ch. 14.3 - f( x )={ 3cosxifx0 3ifx=0 x 3 +3 x 2 x 2 ifx0 c=0Ch. 14.3 - f( x )=2x+3Ch. 14.3 - f( x )=43xCh. 14.3 - f( x )=3 x 2 +xCh. 14.3 - f( x )=3 x 3 +7Ch. 14.3 - f( x )=4sinxCh. 14.3 - f( x )=2cosxCh. 14.3 - f( x )=2tanxCh. 14.3 - f( x )=4cscxCh. 14.3 - f( x )= 2x+5 x 2 4Ch. 14.3 - f( x )= x 2 4 x 2 9Ch. 14.3 - f( x )= x3 InxCh. 14.3 - f( x )= lnx x3Ch. 14.3 - R( x )= x1 x 2 1 , c=1 and c=1Ch. 14.3 - R( x )= 3x+6 x 2 4 , c=2 and c=2Ch. 14.3 - R( x )= x 2 +x x 2 1 , c=1 and c=1Ch. 14.3 - R( x )= x 2 +4x x 2 16 , c=4 and c=4Ch. 14.3 - R( x )= x 3 x 2 +x1 x 4 x 3 +2x2Ch. 14.3 - R( x )= x 3 + x 2 +3x+3 x 4 + x 3 +2x+2Ch. 14.3 - R( x )= x 3 2 x 2 +4x8 x 2 +x6Ch. 14.3 - R( x )= x 3 x 2 +3x3 x 2 +3x4Ch. 14.3 - R( x )= x 3 +2 x 2 +x x 4 + x 3 +2x+2Ch. 14.3 - R( x )= x 3 3 x 2 +4x12 x 4 3 x 3 +x3Ch. 14.3 - R( x )= x 3 x 2 +x1 x 4 x 3 +2x2 Graph R(x) .Ch. 14.3 - R( x )= x 3 + x 2 +3x+3 x 4 + x 3 +2x+2 Graph R( x...Ch. 14.3 - R(x)= ( x 3 2 x 2 +4x8) ( x 2 +x6) Graph R( x ) .Ch. 14.3 - Prob. 86SBCh. 14.3 - Prob. 87SBCh. 14.3 - Prob. 88SBCh. 14.3 - Prob. 89DWCh. 14.3 - Prob. 90DWCh. 14.3 - Prob. 91RYKCh. 14.3 - Evaluate the permutation P( 5,3 ) .Ch. 14.3 - Prob. 93RYKCh. 14.3 - Prob. 94RYKCh. 14.4 - Find an equation of the line with slope 5...Ch. 14.4 - Prob. 2AYPCh. 14.4 - Prob. 3CVCh. 14.4 - Prob. 4CVCh. 14.4 - Prob. 5CVCh. 14.4 - Prob. 6CVCh. 14.4 - Prob. 7CVCh. 14.4 - Prob. 8CVCh. 14.4 - Prob. 9SBCh. 14.4 - Prob. 10SBCh. 14.4 - Prob. 11SBCh. 14.4 - Prob. 12SBCh. 14.4 - Prob. 13SBCh. 14.4 - Prob. 14SBCh. 14.4 - Prob. 15SBCh. 14.4 - Prob. 16SBCh. 14.4 - Prob. 17SBCh. 14.4 - Prob. 18SBCh. 14.4 - Prob. 19SBCh. 14.4 - Prob. 20SBCh. 14.4 - Prob. 21SBCh. 14.4 - Prob. 22SBCh. 14.4 - Prob. 23SBCh. 14.4 - Prob. 24SBCh. 14.4 - Prob. 25SBCh. 14.4 - Prob. 26SBCh. 14.4 - Prob. 27SBCh. 14.4 - Prob. 28SBCh. 14.4 - Prob. 29SBCh. 14.4 - Prob. 30SBCh. 14.4 - Prob. 31SBCh. 14.4 - f( x )=cosx at 0Ch. 14.4 - Prob. 33SBCh. 14.4 - Prob. 34SBCh. 14.4 - Prob. 35SBCh. 14.4 - Prob. 36SBCh. 14.4 - Prob. 37SBCh. 14.4 - Prob. 38SBCh. 14.4 - Prob. 39SBCh. 14.4 - Prob. 40SBCh. 14.4 - Prob. 41SBCh. 14.4 - Prob. 42SBCh. 14.4 - Prob. 43AECh. 14.4 - Prob. 44AECh. 14.4 - Prob. 45AECh. 14.4 - Prob. 46AECh. 14.4 - Prob. 47AECh. 14.4 - Instantaneous Velocity of a Ball In physics it is...Ch. 14.4 - Instantaneous Velocity on the Moon Neil Armstrong...Ch. 14.4 - Instantaneous Rate of Change The following data...Ch. 14.4 - Prob. 51RYKCh. 14.4 - Prob. 52RYKCh. 14.4 - Prob. 53RYKCh. 14.4 - Prob. 54RYKCh. 14.5 - In Problems 29-32, find the first five terms in...Ch. 14.5 - Prob. 2AYPCh. 14.5 - Prob. 3CVCh. 14.5 - Prob. 4CVCh. 14.5 - Prob. 5SBCh. 14.5 - Prob. 6SBCh. 14.5 - Prob. 7SBCh. 14.5 - Prob. 8SBCh. 14.5 - Prob. 9SBCh. 14.5 - Repeat Problem 9 for f( x )=4x .Ch. 14.5 - Prob. 11SBCh. 14.5 - Prob. 12SBCh. 14.5 - Prob. 13SBCh. 14.5 - Prob. 14SBCh. 14.5 - Prob. 15SBCh. 14.5 - Prob. 16SBCh. 14.5 - Prob. 17SBCh. 14.5 - Prob. 18SBCh. 14.5 - Prob. 19SBCh. 14.5 - Prob. 20SBCh. 14.5 - Prob. 21SBCh. 14.5 - Prob. 22SBCh. 14.5 - Prob. 23SBCh. 14.5 - Prob. 24SBCh. 14.5 - Prob. 25SBCh. 14.5 - Prob. 26SBCh. 14.5 - Prob. 27SBCh. 14.5 - Prob. 28SBCh. 14.5 - Prob. 29SBCh. 14.5 - Prob. 30SBCh. 14.5 - Prob. 31SBCh. 14.5 - Consider the function f( x )= 1 x 2 whose domain...Ch. 14.5 - Graph the function f( x )= log 2 x .Ch. 14.5 - If A=[ 1 2 3 4 ] and B=[ 5 6 0 7 8 1 ] , find AB .Ch. 14.5 - If f( x )=2 x 2 +3x+1 , find f( x+h )f( x ) h and...Ch. 14.5 - Prob. 36RYK
Additional Math Textbook Solutions
Find more solutions based on key concepts
Fill in each blank so that the resulting statement is true.
1. A combination of numbers, variables, and opera...
College Algebra (7th Edition)
Derivatives of inverse sine Evaluate the derivatives of the following functions. 7. f(x) = sin1 2x
Calculus: Early Transcendentals (2nd Edition)
Assessment 1-1A The following is a magic square all rows, columns, and diagonals sum to the same number. Find t...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
True or False? In Exercises 5–8, determine whether the statement is true or false. If it is false, rewrite it a...
Elementary Statistics: Picturing the World (7th Edition)
Finding Critical Values. In Exercises 5–8, find the critical value z?/2 that corresponds to the given confidenc...
Elementary Statistics (13th Edition)
The table for ordered pairs (x,y)
Pre-Algebra Student Edition
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- 2. Find the following limits without using a graphing calculator or tables. x-5 x-5 2x²-9x-5 a)lim b) lim x→1 -X c) lim h→0 5xh-4x³h² harrow_forwardGiven the graph of f(x) at the left, determine the following: a. f(6) = b. lim f(x) = C. x-2 lim f(x) = x-6 d. lim f(x) = x-2- =arrow_forwardThe graph below is the function f (x) -5 -4 -3 -2 -1 +-+ -3 -4 -S lim f(x) = x-3- lim f(x) = x-3 f(3) = lim f(x) = x 3+ 5 4 3 2 + Determine the following values. Enter "DNE" if a value does not exist, enter "oo" (lower case "o") if the limit approaches positive infinity, or "-oo" if the limit approaches negative infinity. Ā 2. Determine if the function is continuous or discontinuous at the limit value. If it is discontinuous, indicate if the discontinuity is removable or non-removable. O The function is continuous at x = 3 O The function has a removable discontinuity at x = 3 O The function has a non-removable discontinuity at x = 3 If the function has a discontinuity at the limit value, check all the boxes that indicate why the function is discontinuous there.arrow_forward
- Graph A Graph B Graph C Graph D Graph E Graph F 1. fi(x) = - + 8 matches graph ? and has limits: a. lim fi(x) = b. lim f1(x) = 2. f2(x) = + 8 matches graph ? 0 and has limits: a. lim f2(x) = b. lim f2(x) = 3. f3(x) = - 8 matches graph ? 0 and has limits: a. lim f3(x) = b. lim f3(x) = 4. fa(x) = - 8 matches graph ? and has limits: a. lim f4(x) = b. lim f4(x) =arrow_forward2. The drawing to the right shows the graph of y = f(x). Use the information in the graph to calculate each of the following values. If a value is undefined or a limit does not exist, explain why. (a) 3ƒ(2) (b) lim f(x) + 5 x-2 (c) lim f(x) (d) lim (r² f(x)) 1 x+2 f(x)-f(2) — 4 (e) lim (f(x))² - 9 (g) limf(x) - 3 (h) lim g(x), if f(x) ≤ g(x) ≤ 3 on the intervals [1, 2) and (2,3] (f) lim √4f(x) + 2x − 5ƒ(2) x-2 Y 3 2 1 -1 -2 1 2 ● 3 y = f(x) 4 xarrow_forwardSOLVE STEP BY STEP IN DIGITAL FORMAT Calculate the limit of the following functions at the indicated value. 2x - 20 lim x 10 √5x-1-7 x² - 144 lim x 12 √x + 135 lim X-8 x3 +5x2 − x +1 5x2 + 5x - 3arrow_forward
- The graph below is the function f(x) -5 -4 -3 -2 -1 x→-2- 5 4 3 lim_f(x) = -1 -2 -3 -4 -5 Determine the following values. Enter "DNE" if a value does not exist, enter "oo" (lower case "o") if the limit approaches positive infinity, or "-oo" if the limit approaches negative infinity. lim f(x) x→-2 lim f(x) = x→ 2+ f(-2) = 2 3 4 5arrow_forwardProblem : Evaluate the fallasing limit, if avit, Lim X-31. x²-3 X→3 8.26 X 11.69 inch 口arrow_forwardUse the graph to find the following limits and function value. a. lim f(x) x-3 b. lim f(x) x-3* c. lim f(x) 2 X-3 d. f(3)arrow_forward
- 2. For the function g(t) whose graph is given, state the value of each quantity, if it exists. If it does not exist, explain why. (a) lim g(t) (b) lim g(t) (c) lim g(1) (d) lim g(t) (e) lim g(t) (f) lim g(t) 2 (g) g(2) (h) lim g(t) 4 2 4arrow_forward4. Given the graph of the function f below, evaluate lim f(x).arrow_forwardGiven the graph of f(x) at the left, determine the following: a. f(6)= b. lim f(x) = x+2 C. lim f(x) = x-6 d. lim f(x) = x-2- 6- y = f(x) 4- 2- ΟΙ 2 -~ x 2 6 8arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendentals
Calculus
ISBN:9781285741550
Author:James Stewart
Publisher:Cengage Learning
Thomas' Calculus (14th Edition)
Calculus
ISBN:9780134438986
Author:Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:9780134763644
Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:PEARSON
Calculus: Early Transcendentals
Calculus
ISBN:9781319050740
Author:Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:W. H. Freeman
Calculus: Early Transcendental Functions
Calculus
ISBN:9781337552516
Author:Ron Larson, Bruce H. Edwards
Publisher:Cengage Learning
Limits and Continuity; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=9brk313DjV8;License: Standard YouTube License, CC-BY