
NOW TRY THIS
To derive the formula for area of a triangle from the formula for the area of a rectangle, cut out a triangle as in Figure 23(a); fold a perpendicular to the base with measurement b and containing point B to find the height h; and fold the altitude in half, as shown in Figure23(b). Next fold along the colored segments in the trapezoid in Figure23(c) to obtain rectangle in Figure 23(d).
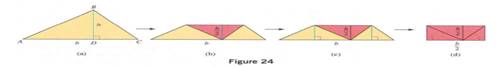
a. What is the area of the rectangle in Figure 23(d)?
b. How can the formula for the area of a triangle be developed from your answer in part (a)?
(a)
To find:
The area of rectangle of Figure 23(d).
Answer to Problem 4NT
Solution:
Area of rectangle be
Explanation of Solution
Given:
A rectangle with dimension
Approach:
Calculation:
(b)
To find:
The area of triangle from rectangle of figure 23(d).
Answer to Problem 4NT
Solution:
Explanation of Solution
Given:
A rectangle of dimension
Approach:
Calculation:
Here rectangle, Figure
So,
Thus
Want to see more full solutions like this?
Chapter 14 Solutions
Problem Solving Approach to Mathematics for Elementary School Teachers, A, Plus MyLab Math -- Access Card Package (12th Edition)
Additional Math Textbook Solutions
Elementary Statistics Using The Ti-83/84 Plus Calculator, Books A La Carte Edition (5th Edition)
Precalculus: A Unit Circle Approach (3rd Edition)
Finite Mathematics for Business, Economics, Life Sciences and Social Sciences
Elementary Statistics: Picturing the World (7th Edition)
Elementary Statistics: A Step By Step Approach
- solve the question based on hw 1, 1.41arrow_forwardالتمرين الأول: 08) نقاط) نرمي رباعي وجوه مرقم من ا إلى 4 بحيث إحتمال وجوهه يحقق العلاقة التالية: - 24 = (3)P(1) = ) = 4P -1 أحسب احتمال كل وجه. -2 (١ أحسب احتمال الحادثة : الحصول على عدد زوجي). ب استنتج احتمال الحادثة ة. -3 أحسب احتمال الحادثة B الحصول على عدد د أكبر أو يساوي (2)arrow_forwardA homeware company has been approached to manufacture a cake tin in the shape of a "ghost" from the Pac-Man video game to celebrate the 45th Anniversary of the games launch. The base of the cake tin has a characteristic dimension / and is illustrated in Figure 1 below, you should assume the top and bottom of the shape can be represented by semi-circles. The vertical sides of the cake tin have a height of h. As the company's resident mathematician, you need to find the values of r and h that minimise the internal surface area of the cake tin given that the volume of the tin is Vfixed- 2r Figure 1 - Plan view of the "ghost" cake tin base. (a) Show that the Volume (V) of the cake tin as a function of r and his 2(+1)²h V = 2arrow_forward
- 15. Please solve this and show each and every step please. PLEASE no chatgpt can I have a real person solve it please!! I am stuck. I am doing pratice problems and I do not even know where to start with this. The question is Please compute the indicated functional value.arrow_forwardSolve the system of equation for y using Cramer's rule. Hint: The determinant of the coefficient matrix is -23. - 5x + y − z = −7 2x-y-2z = 6 3x+2z-7arrow_forward◆ Switch To Light Mode HOMEWORK: 18, 19, 24, 27, 29 ***Please refer to the HOMEWORK sheet from Thursday, 9/14, for the problems ****Please text or email me if you have any questions 18. Figure 5-35 is a map of downtown Royalton, showing the Royalton River running through the downtown area and the three islands (A, B, and C) connected to each other and both banks by eight bridges. The Down- town Athletic Club wants to design the route for a marathon through the downtown area. Draw a graph that models the layout of Royalton. FIGURE 5-35 North Royalton Royalton River South Royption 19. A night watchman must walk the streets of the Green Hills subdivision shown in Fig. 5-36. The night watch- man needs to walk only once along each block. Draw a graph that models this situation.arrow_forward
- L 16.8. For each of the following functions f, describe the domain of ana- lyticity and apply the Cauchy-Goursat Theorem to show that f(z)dz = 0, where is the circle |2|=1:1 (a). f(z) = 1 z 2 + 2x + 2 (b). f(z) = ze*. What about (c). f(z) = (2z-i)-2?arrow_forward16.3. Evaluate each of the following integrals where the path is an arbitrary contour between the limits of integrations (a). [1 ri/2 edz, (b). (b). La cos COS (2) d dz, (c). (z−3)³dz. 0arrow_forwardQ/ prove that:- If Vis a finite dimensional vector space, then this equivalence relation has only a single equivalence class.arrow_forward

 Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,





