
Concept explainers
The 2-lb ball at A is suspended by an inextensible cord and given an initial horizontal velocity of v0. If l = 2 ft, xB = 0.3 ft, and yB = 0.4 ft, determine the initial velocity v0 so that the ball will enter the basket. (Hint: Use a computer to solve the resulting set of equations.)
Fig. P13.201
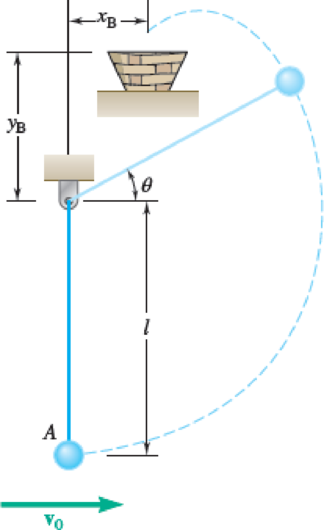
Find the initial velocity
Answer to Problem 13.201RP
The initial velocity
Explanation of Solution
Given information:
The weight of the ball (m) is
The length of the cord (l) is
The horizontal distance between the basket and point of suspension of the ball
The vertical distance between basket and point of suspension of the ball
The acceleration due to gravity (g) is
Calculation:
Show the diagram of the suspended ball by an inextensible cord as in Figure (1).
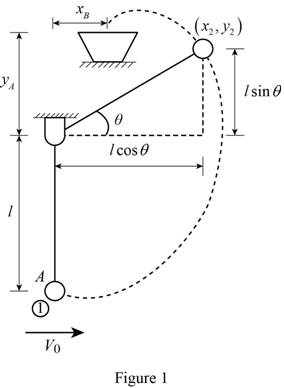
Assume that position ‘1’ be at A and position ‘2’ be at the point described by the angle where the path of the ball changes from circular to parabolic.
The tension in the cord at position ‘2’, becomes slack
Refer Figure (1),
The expression for the x-coordinate of the ball at position ‘2’
The expression for the y-coordinate of the ball at position ‘2’
Show the free body diagram of the ball at position ‘2’ as in Figure (2).
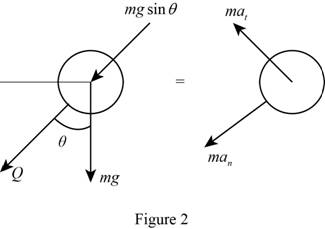
The expression for the normal acceleration of the ball
Since, the cord becomes slack at position ‘2’, so the tension (Q) will be zero.
Calculate the velocity of the ball by applying Newton’s second law and resolve the forces acting on the ball at position ‘2’ using the relation:
Substitute
Substitute 0 for
The expression for the kinetic energy of the ball at position ‘1’
Calculate the potential energy of the ball at position ‘1’
Here, negative sign is used as the ball is located below the datum level and h is the vertical distance of the ball from the datum level.
Substitute
The expression for the kinetic energy of the ball at position ‘2’
The expression for the vertical distance of the ball by referring the Figure 1 as follows:
Calculate the potential energy of the ball at position ‘2’
Substitute
The expression for principle of conservation of energy at position ‘1’ and position ‘2’ for the ball, to calculate the angle swept by the ball
Substitute
Find the velocity at position 2:
Substitute
Show the parabolic motion of the ball after it reaches position ‘2’ as in Figure (3).
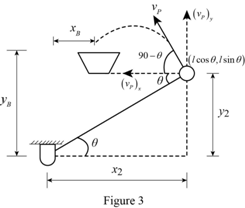
The expression for the velocity of the projectile ball after reaching the position ‘2’
Here,
The expression for the horizontal velocity component of the projectile ball along the negative X-axis as follows:
The expression for the horizontal distance between the basket and point of suspension of the ball
Here,
Substitute
Substitute
The expression for the vertical velocity component of the projectile ball along the negative X-axis as follows:
The expression for the vertical distance between the basket and point of suspension of the ball
Substitute
Substitute
Use trial and error method to calculate the value of
Case (1):
Try
Find the velocity at position 2:
Substitute
Find the time difference between basket and ball:
Substitute
Find the vertical distance between basket and ball:
Substitute
Case (2):
Try
Find the velocity at position 2:
Substitute
Find the time difference between basket and ball:
Substitute
Find the vertical distance between basket and ball:
Substitute
Case (3):
Try
Find the velocity at position 2:
Substitute
Find the time difference between basket and ball:
Substitute
Find the vertical distance between basket and ball:
Substitute
The expression for the data point as follows:
From above calculations, the following set of data points is obtained.
Calculate the general form of quadratic Equation.
Here, a, b, c are constants.
Substitute 0 for
Substitute
Substitute
Solve the equation (7) and equation (8).
Substitute
Substitute
Solve the above equation.
Calculate the angle
Substitute
Find the velocity at position 2:
Substitute
Find the initial velocity
Substitute
Therefore, the initial velocity
Want to see more full solutions like this?
Chapter 13 Solutions
VECTOR MECH. FOR EGR: STATS & DYNAM (LL
- (read image)arrow_forwardProblem 3.30 A piston-cylinder device contains 0.85 kg of refrigerant- 134a at -10°C. The piston that is free to move has a mass of 12 kg and a diameter of 25 cm. The local atmospheric pressure is 100 kPa. Now, heat is transferred to refrigerant-134a until the temperature is 15°C. Determine (a) the final pressure, (b) the change in the volume of the refrigerant, and (c) the change in the enthalpy of the refrigerant-134a. please show Al work step by steparrow_forwardPart 1 The storage tank contains lubricating oil of specific gravity 0.86 In one inclined side of the tank, there is a 0.48 m diameter circular inspection door, mounted on a horizontal shaft along the centre line of the gate. The oil level in the tank rests 8.8 m above the mounted shaft. (Please refer table 01 for relevant SG, D and h values). Describe the hydrostatic force and centre of pressure with the aid of a free body diagram of the inspection door. Calculate the magnitude of the hydrostatic force and locate the centre of pressure. 45° Estimate the moment that would have to be applied to the shaft to open the gate. Stop B If the oil level raised by 2 m from the current level, calculate the new moment required to open the gate. Figure 01arrow_forward
- From thermodynamics please fill in the table show all work step by steparrow_forwardThe 150-lb skater passes point A with a speed of 6 ft/s. (Figure 1) Determine his speed when he reaches point B. Neglect friction. Determine the normal force exerted on him by the track at this point. 25 ft B = 4x A 20 ft xarrow_forwardA virtual experiment is designed to determine the effect of friction on the timing and speed of packages being delivered to a conveyor belt and the normal force applied to the tube. A package is held and then let go at the edge of a circular shaped tube of radius R = 5m. The particle at the bottom will transfer to the conveyor belt, as shown below. Run the simulations for μ = 0, 0.1, 0.2, 0.3, 0.4, 0.5, 0.6 and determine the time and speed at which the package is delivered to the conveyor belt. In addition, determine the maximum normal force and its location along the path as measured by angle 0. Submit in hardcopy form: (0) Free Body Diagram, equations underneath, derivations (a) Your MATLAB mfile (b) A table listing the values in 5 columns: μ, T (time of transfer), V (speed of transfer), 0 (angle of max N), Nmax (max N) (c) Based on your results, explain in one sentence what you think will happen to the package if the friction is increased even further, e.g. μ = 0.8. NOTE: The ODE is…arrow_forward
- Patm = 1 bar Piston m = 50 kg 5 g of Air T₁ = 600 K P₁ = 3 bar Stops A 9.75 x 10-3 m² FIGURE P3.88arrow_forwardAssume a Space Launch System (Figure 1(a)) that is approximated as a cantilever undamped single degree of freedom (SDOF) system with a mass at its free end (Figure 1(b)). The cantilever is assumed to be massless. Assume a wind load that is approximated with a concentrated harmonic forcing function p(t) = posin(ωt) acting on the mass. The known properties of the SDOF and the applied forcing function are given below. • Mass of SDOF: m =120 kip/g • Acceleration of gravity: g = 386 in/sec2 • Bending sectional stiffness of SDOF: EI = 1015 lbf×in2 • Height of SDOF: h = 2000 inches • Amplitude of forcing function: po = 6 kip • Forcing frequency: f = 8 Harrow_forwardAssume a Space Launch System (Figure 1(a)) that is approximated as a cantilever undamped single degree of freedom (SDOF) system with a mass at its free end (Figure 1(b)). The cantilever is assumed to be massless. Assume a wind load that is approximated with a concentrated harmonic forcing function p(t) = posin(ωt) acting on the mass. The known properties of the SDOF and the applied forcing function are given below. • Mass of SDOF: m =120 kip/g • Acceleration of gravity: g = 386 in/sec2 • Bending sectional stiffness of SDOF: EI = 1015 lbf×in2 • Height of SDOF: h = 2000 inches • Amplitude of forcing function: po = 6 kip • Forcing frequency: f = 8 Hz Figure 1: Single-degree-of-freedom system in Problem 1. Please compute the following considering the steady-state response of the SDOF system. Do not consider the transient response unless it is explicitly stated in the question. (a) The natural circular frequency and the natural period of the SDOF. (10 points) (b) The maximum displacement of…arrow_forward
- Assume a Space Launch System (Figure 1(a)) that is approximated as a cantilever undamped single degree of freedom (SDOF) system with a mass at its free end (Figure 1(b)). The cantilever is assumed to be massless. Assume a wind load that is approximated with a concentrated harmonic forcing function p(t) = posin(ωt) acting on the mass. The known properties of the SDOF and the applied forcing function are given below. • Mass of SDOF: m =120 kip/g • Acceleration of gravity: g = 386 in/sec2 • Bending sectional stiffness of SDOF: EI = 1015 lbf×in2 • Height of SDOF: h = 2000 inches • Amplitude of forcing function: po = 6 kip • Forcing frequency: f = 8 Hz Figure 1: Single-degree-of-freedom system in Problem 1. Please compute the following considering the steady-state response of the SDOF system. Do not consider the transient response unless it is explicitly stated in the question. (a) The natural circular frequency and the natural period of the SDOF. (10 points) (b) The maximum displacement of…arrow_forwardPlease solve 13 * √(2675.16)² + (63.72 + 2255,03)² = 175x106 can you explain the process for getting d seperate thank youarrow_forwardIf the 300-kg drum has a center of mass at point G, determine the horizontal and vertical components of force acting at pin A and the reactions on the smooth pads C and D. The grip at B on member DAB resists both horizontal and vertical components of force at the rim of the drum. P 60 mm; 60 mm: 600 mm A E 30° B C 390 mm 100 mm D Garrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





