
To determine the shaded region the given figure.
Answer to Problem 52SR
Explanation of Solution
Given:
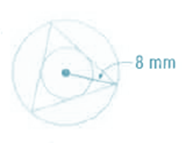
Radius of the outer
Formula used:
Area of a circle
where
Area of an equilateral
Calculation:
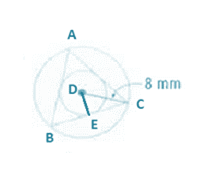
As
In
So,
On cross-multiplying,
As
So,
Area of
Putting the value of a,
So, area of
Now,
On cross-multiplying,
Area of smaller circle,
Radius
Area =
Putting the value of
So, area of small circle
Now,
Area of outer circle,
Putting the value of R,
So, area of the outer circle
Hence, Area of the shaded region = Area of outer circle
Now, putting the values,
Conclusion:
Therefore, the area of the shaded region is
Chapter 12 Solutions
Glencoe Geometry
Additional Math Textbook Solutions
Thinking Mathematically (6th Edition)
Basic Business Statistics, Student Value Edition
Elementary Statistics: Picturing the World (7th Edition)
Algebra and Trigonometry (6th Edition)
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
College Algebra with Modeling & Visualization (5th Edition)
- 39 Two sides of one triangle are congruent to two sides of a second triangle, and the included angles are supplementary. The area of one triangle is 41. Can the area of the second triangle be found?arrow_forwardA parallelogram with an area of 211.41 m^2 hast a base Thatcher measures 24.3m. Find ist height.arrow_forwardBH is tangent to circle A and DF is a diameter. I don't know where to go from here. May you help please?arrow_forward
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning

