
Concept explainers
(a)
Draw the influence line for the positive moment at section B using moment distribution method.
(a)
Explanation of Solution
Influence line for the moment at support B.
Influence lines will be constructed by placing the unit load at every 20 ft interval from A along the span of the beam. The points are indicated by the circled numbers.
To establish the influence line ordinate at the left end (point 1), the unit load is placed on the beam directly over support A. Since the entire load passes directly into the support, the beam is unstressed. Therefore,
Similarly, if the unit load is moved at C and D,
Case 1:
Apply unit load at 20 ft from A. (point 2).
Draw the beam with points of applying unit load at B (point 2) as in Figure 1.
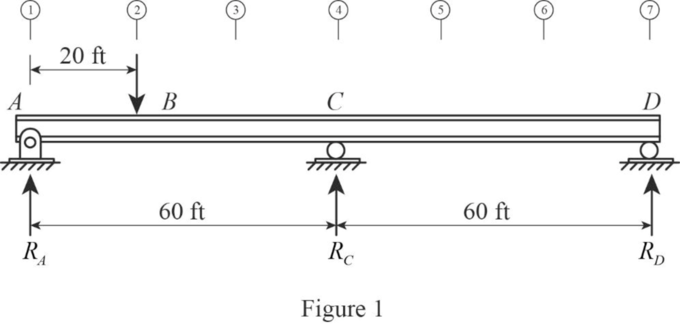
Refer Figure 1.
Find the fixed end moment at each end of the member CD as follows;
Find the fixed end moment at each end of the member CA as follows;
Find the distribution factor.
Show the computation of distribution factor as in Table 1.
| Joints | Member | Stiffness, K | ||
| C | CA | |||
| CD |
Show the moment distribution computations as in Table 2.
| Joint | A | C | D | ||
| Member | AC | CA | CD | DC | |
| DF | 0.5 | 0.5 | |||
| FEM | ‑8.89 | 4.44 | 0 | 0 | |
| Released moment | +8.89 | 0 | 0 | 0 | |
| COM | 0 | 4.44 | 0 | ||
| Total | 0 | 8.88 | 0 | 0 | |
| DEM | ‑4.44 | ‑4.44 | |||
| COM | |||||
| Final | 0 | 4.44 | ‑4.44 | 0 | |
Find the reaction at A using section AC.
Draw the free body diagram of section AC as in Figure 2.
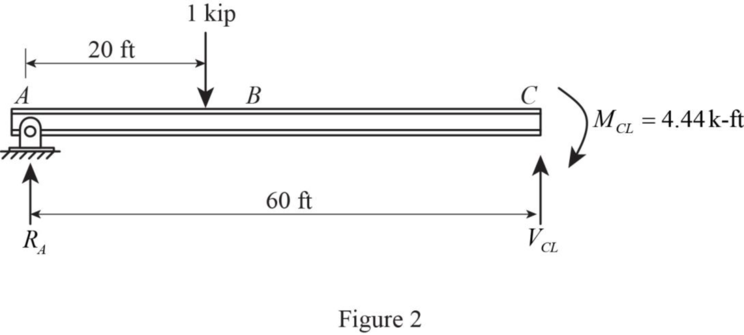
Refer Figure 1.
Find the reaction at A.
Consider moment at C
Find the moment at B.
Consider moment at B
Thus, the influence line ordinate of moment at B when 1 kip applied at B is 11.86.
Case 2:
Apply unit load at 20 ft from C. (point 5).
Draw the beam with points of applying at point 5 as in Figure 3.
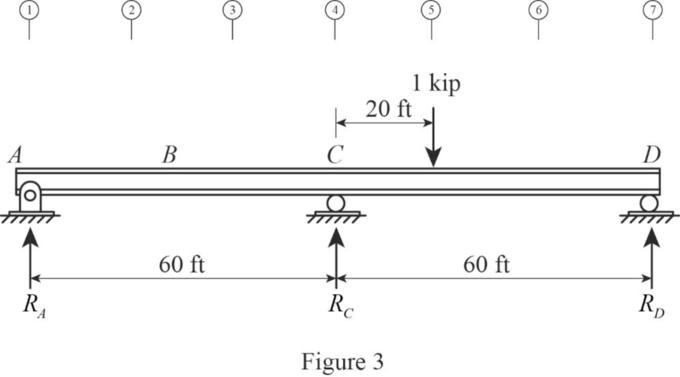
Refer Figure 3.
Find the fixed end moment at each end of the member CD as follows;
Find the fixed end moment at each end of the member DC as follows;
Show the moment distribution computations as in Table 3.
| Joint | A | C | D | ||
| Member | AC | CA | CD | DC | |
| DF | 0.5 | 0.5 | |||
| FEM | 0 | 0 | ‑8.89 | 4.440 | |
| Released moment | 0 | 0 | 0 | ‑4.44 | |
| COM | 0 | 0 | ‑2.22 | ||
| Total | 0 | 0 | ‑11.11 | 0 | |
| DEM | +5.56 | +5.56 | |||
| COM | |||||
| Final | 0 | 5.56 | ‑5.56 | 0 | |
Find the reaction at A using section AC.
Draw the free body diagram of section AC as in Figure 4.
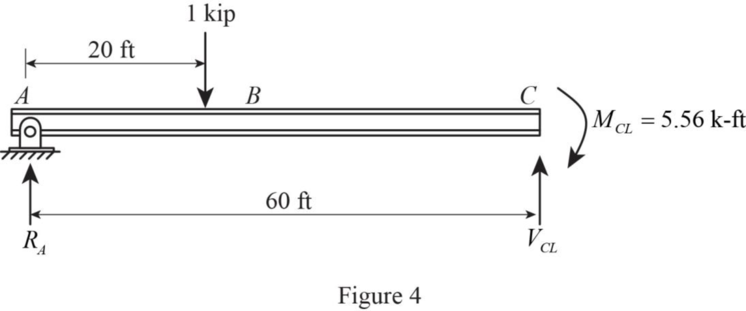
Refer Figure 4.
Find the reaction at A.
Consider moment at C
Find the moment at B.
Consider moment at B
Thus, the influence line ordinate of moment at B when 1 kip applied at point 5 is ‑1.876.
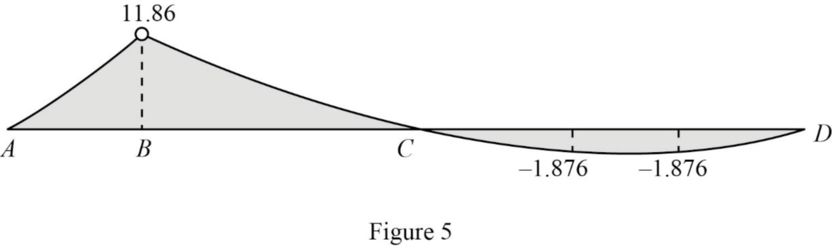
Similarly calculate the influence line ordinate by applying the points 3 and 5 and tabulate the values as in Table 4.
| Apply 1 k at point | Influence line ordinate of moment at B. |
| 1 | 0 |
| 2 | 11.86 |
| 4 | 0 |
| 5 | ‑1.876 |
| 6 | ‑1.876 |
| 7 | 0 |
Draw the influence line diagram of influence line ordinate of moment at B using Table 4 as in Figure 5.
(b)
Find the maximum moment at B for the given loading condition.
(b)
Answer to Problem 53P
The maximum moment at B for the given loading condition is
Explanation of Solution
Consider the uniformly distributed load of 2 kips/ft act on the span AC only and the concentrated live load of 20 kips act at B.
Draw the free body diagram of the beam for the given loading as in Figure 6.
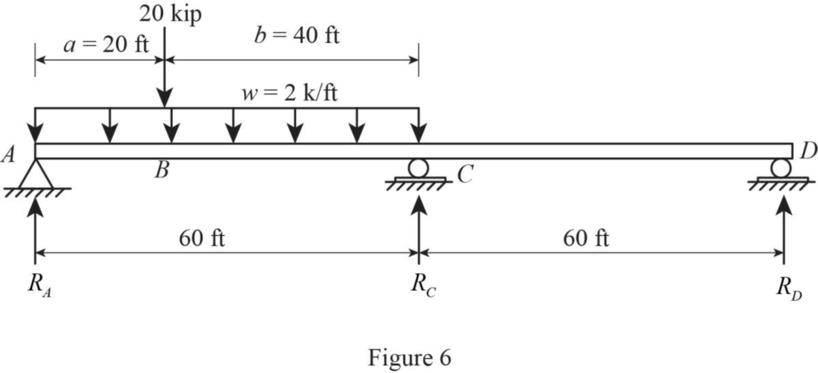
Refer Figure 6.
Find the fixed end moment at each end of the member AC as follows;
Find the fixed end moment at each end of the member CA as follows;
Show the moment distribution computations as in Table 5.
| Joint | A | C | D | ||
| Member | AC | CA | CD | DC | |
| DF | 0.5 | 0.5 | |||
| FEM | ‑777.78 | 688.89 | 0 | 0 | |
| Released moment | +777.78 | 00 | 0 | 0 | |
| COM | 388.89 | ||||
| Total | 0 | 1,077.78 | 0 | 0 | |
| DEM | ‑538.89 | ‑538.89 | |||
| COM | |||||
| Final | 0 | 538.89 | ‑538.89 | 0 | |
Find the reaction at A using section AC.
Draw the free body diagram of section AC as in Figure 7.
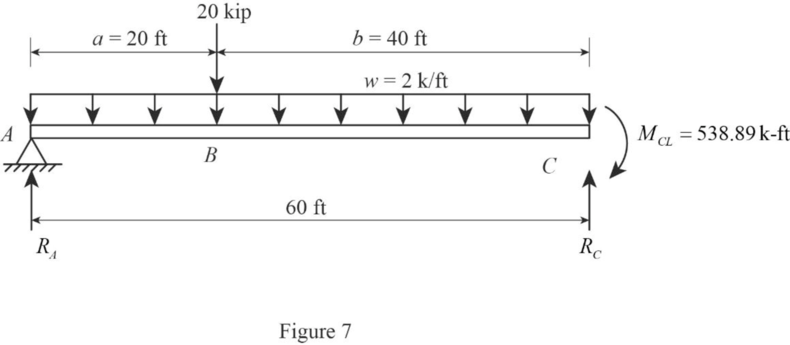
Refer Figure 7.
Find the reaction at A.
Consider moment at C
Find the moment at B.
Consider moment at B
Therefore, the maximum moment at B for the given loading condition is
(c)
Find the maximum moment at B due to wheel loads using moment distribution method.
(c)
Answer to Problem 53P
The maximum moment at B due to wheel loads using moment distribution method is
Explanation of Solution
The maximum moment at B is produced when wheel load is placed at B.
Draw the free body diagram of the beam for the wheel loading as in Figure 8.
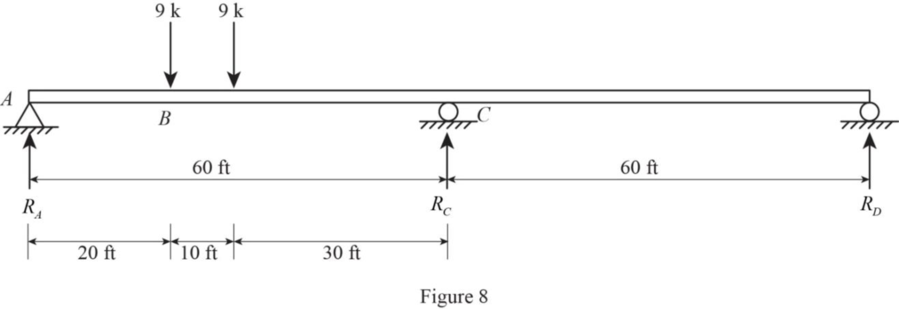
Refer Figure 8.
Find the fixed end moment at each end of the member AC as follows;
Find the fixed end moment at each end of the member CA as follows;
Show the moment distribution computations as in Table 6.
| Joint | A | C | D | ||
| Member | AC | CA | CD | DC | |
| DF | 0.5 | 0.5 | |||
| FEM | ‑147.5 | 107.5 | 0 | 0 | |
| Released moment | +147.5 | 0 | 0 | 0 | |
| COM | 73.75 | ||||
| Total | 0 | 181.25 | 0 | 0 | |
| DEM | ‑90.63 | 90.63 | |||
| COM | |||||
| Final | 0 | ‑90.63 | 90.63 | 0 | |
Find the reaction at A using section AC.
Draw the free body diagram of section AC as in Figure 9.
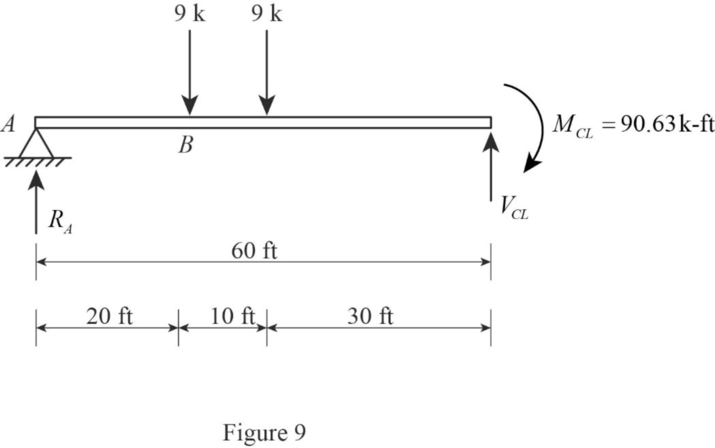
Refer Figure 7.
Find the reaction at A.
Consider moment at C
Find the moment at B.
Consider moment at B
Therefore, the maximum moment at B for the given loading condition is
Want to see more full solutions like this?
Chapter 12 Solutions
UCD FUND OF STRUCTURAL ANALYSIS 5E
- In testing a certain kind or truck tire over rugged terrain, it is found that 20% of the trucks fail to complete the test run without a blowout. Of the next 13 trucks tested, find the probability that (a) from 2 to 6 have blowouts, (b) fewer than 4 have blowouts, and (c) more than 5 have blowouts. Click here to view page 1 of the table of binomial probability sums. Click here to view page 2 of the table of binomial probability sums. (a) The probability that from 2 to 6 trucks have blowouts is (Round to four decimal places as needed.)arrow_forwardA project requires 125 cubic yards of concrete sidewalk to be placed, for which 165 workhours have been budgeted. The latest weekly progress report shows that 78 cubic yards have been placed and 103 workhours have been expended to date. What is the status of the concrete placement? Significantly under budget. On budget. Significantly over budget. Status cannot be determined with information supplied.arrow_forwardRefer to exhibit #098. At what depth was water encountered?arrow_forward
- What is the reaction moment at A for the frame shown? a. 222.1 k-ft b. 107.8 k-ft c. 20.8 k-ft d. 23.25 k-ftarrow_forward“When a conflict exists between the project floor plans and detailed material schedule relative to size or number, which of the following usually governs in typical order of precedence?arrow_forwardWhat are the critical activitiesarrow_forward
- Approximately how many pounds of water are necessary to hydrate 100 pounds of type I Portland cement? 30 50 75 94arrow_forward7:05 3.1 Trabajo en clase.pptx .III LTE 8 Trabajo en clases 3.1 C9 X 20 W8 X 21 5-15. PL¹× 12 Fy = 50 klb/plg² KL = 16 pies KL 21 pies 2 plg MC 13 × 50 PL × 12 Fy = 42 klb/plg2 Fy = 36 klb/plg² 8 plg K k MC8 × 21.4 KL = 20 piesarrow_forwardThe steel frameword below is used to support the reinforced concrete slab used for an office area above the first storey. The slab is 210 mm thick. Sketch the loading that acts along members BE and FED. Use a = 2.15 m and b = 5.25 m. Refer to the 2024 OBC live load table. The unit weight for the concrete is 24.15 kN/m3.find:Loading for member BE Loading for member FED Live and Dead Loadsarrow_forward
- For the simply supported beam below, draw both the shear force (VFD) and ending moment (BDM) diagrams. Please show all equations and free body diagrams (FBD). Note: I want a cut through each of the three sections of the beam, with all related forces calculated and shown on the VFD and BMD.Reaction Forces Shear Force DiagramMaximum Shear ForceEquation for cut 1, 2, 3 respectively.Confirmation of Reaction ForcesBending Moment DiagramMaximum Bending Momentarrow_forwardFor the structural frame below, draw the shear force (VFD) and bending moment (BMD) diagrams for each of the three members of the frame. The frame is pin connected at A, C and D and fixed at joint B.Find:VFD & BMD for segment AB VFD & BMD for segment BCVFD & BMD for segment CD Reaction Forces VFD Equations BMD EquationsFree Body Diagramsarrow_forwardDetermine the horizontal and vertical reactions at A and C for the two member frame below. Use P1 = 3.2 kN, P2 = 14.5 kN/m, L1 = 3.3 m, and L2 = 2.3 m. Free Body DiagramsTriangular Load Use of Pin Reaction Forcesarrow_forward

 Structural Analysis (10th Edition)Civil EngineeringISBN:9780134610672Author:Russell C. HibbelerPublisher:PEARSON
Structural Analysis (10th Edition)Civil EngineeringISBN:9780134610672Author:Russell C. HibbelerPublisher:PEARSON Principles of Foundation Engineering (MindTap Cou...Civil EngineeringISBN:9781337705028Author:Braja M. Das, Nagaratnam SivakuganPublisher:Cengage Learning
Principles of Foundation Engineering (MindTap Cou...Civil EngineeringISBN:9781337705028Author:Braja M. Das, Nagaratnam SivakuganPublisher:Cengage Learning Fundamentals of Structural AnalysisCivil EngineeringISBN:9780073398006Author:Kenneth M. Leet Emeritus, Chia-Ming Uang, Joel LanningPublisher:McGraw-Hill Education
Fundamentals of Structural AnalysisCivil EngineeringISBN:9780073398006Author:Kenneth M. Leet Emeritus, Chia-Ming Uang, Joel LanningPublisher:McGraw-Hill Education
 Traffic and Highway EngineeringCivil EngineeringISBN:9781305156241Author:Garber, Nicholas J.Publisher:Cengage Learning
Traffic and Highway EngineeringCivil EngineeringISBN:9781305156241Author:Garber, Nicholas J.Publisher:Cengage Learning





