
Concept explainers
Find whether the sample data indicate that the
Perform a hypothesis test to see whether the mean number of transactions per customer is more than 10 per month.
Check whether the mean number of transactions per customer is more than 9 per month.
Find whether there is any difference in the mean checking account balances among the four branches. Also find the pair of branches where these differences occur.
Check whether there is a difference in ATM usage among the four branches.
Find whether there is a difference in ATM usage between the customers who have debit cards and who do not have debit cards.
Find whether there is a difference in ATM usage between the customers who pay interest verses those who do not pay.
Explanation of Solution
Hypothesis test for mean checking account balance:
Denote
The null and alternative hypotheses are stated below:
That is, the mean account balance is $1,600.
That is, the mean account balance is less than $1,600.
Step-by-step procedure to obtain the test statistic using Excel MegaStat is as follows:
- In EXCEL, Select Add-Ins > Mega Stat > Hypothesis Tests.
- Choose Mean Vs Hypothesized Value.
- Choose Data Input.
- Enter A1:A61 Under Input
Range . - Enter 1,600 Under Hypothesized mean.
- Check t-test.
- Choose less than in alternative.
- Click OK.
Output obtained using Excel MegaStat is as follows:
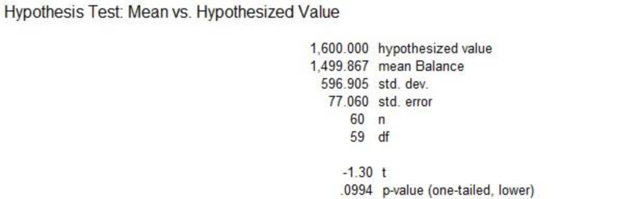
From the output, the t-test statistic value is –1.30 and the p-value is 0.0994.
Decision Rule:
If the p-value is less than the level of significance, reject the null hypothesis. Otherwise, fail to reject the null hypothesis.
Conclusion:
Consider that the level of significance is 0.05.
Here, the p-value is 0.0994. Since the p-value is greater than the level of significance, by the rejection rule, fail to reject the null hypothesis at the 0.05 significance level.
Thus, the sample data do not indicate that the mean account balance has declined from $1,600.
Hypothesis test for the mean number of transaction10 per customer per month:
Denote
The null and alternative hypotheses are stated below:
The mean number of transaction per customer is less than or equal to 10 per month.
The mean number of transaction per customer is more than 10 per month.
Step-by-step procedure to obtain the test statistic using Excel MegaStat is as follows:
- In EXCEL, Select Add-Ins > Mega Stat > Hypothesis Tests.
- Choose Mean Vs Hypothesized Value.
- Choose Data Input.
- Enter A1:A61 Under Input Range.
- Enter 10 Under Hypothesized mean.
- Check t-test.
- Choose greater than in alternative.
- Click OK.
Output obtained using Excel MegaStat is as follows:
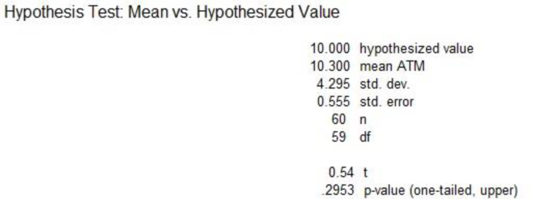
From the above output, the t-test statistic value is 0.54 and the p-value is 0.2953.
Conclusion:
Since the p-value is greater than the level of significance, by the rejection rule, fail to reject the null at the 0.05 significance level. Therefore, there is no sufficient evidence to conclude that the mean number of transactions per customer is more than 10 per month.
Hypothesis test for mean number of transaction 9 per customer per month:
The null and alternative hypotheses are stated below:
The mean number of transactions per customer is less than or equal to 9 per month.
The mean number of transaction per customer is more than 9 per month.
Follow the same procedure mentioned above to obtain the test statistic.
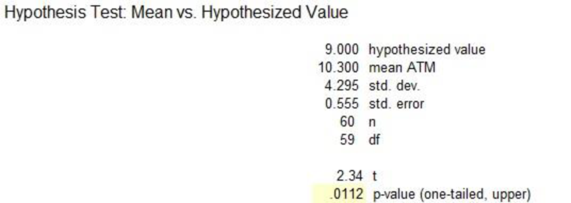
From the above output, the test statistic value is 2.34 and the p-value is 0.0112.
Conclusion:
Here, the p-value is less than the significance level 0.05. Therefore, the advertising agency can be concluded that the mean number of transactions per customer is more than 9 per month.
Hypothesis test for mean checking account balance among the four branches:
The null and alternative hypotheses are given below:
Null hypothesis:
The mean checking account balance among the four branches is equal.
Alternative hypothesis:
The mean checking account balance among the four branches is different.
Step-by-step procedure to obtain the test statistic using Excel MegaStat is as follows:
- In EXCEL, Select Add-Ins > Mega Stat > Analysis of Variance.
- Choose One-Factor ANOVA.
- In Input Range, select thedata range.
- In Post-Hoc Analysis, Choose When p < 0.05.
- Click OK.
Output obtained using Excel MegaStat is as follows:
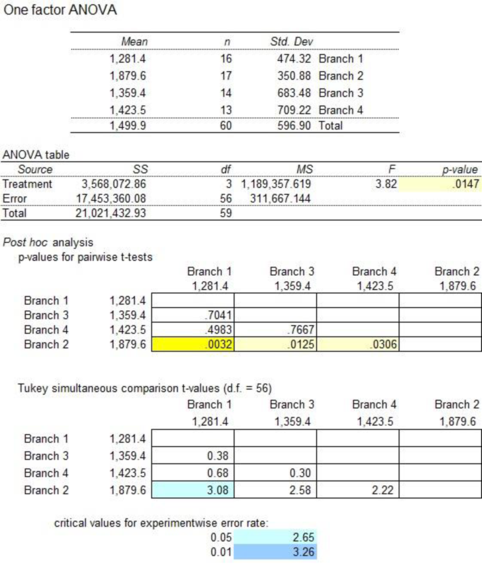
From the above output, the F-test statistic is 3.82 and the p-value is 0.0147.
Conclusion:
The p-value is less than the significance level 0.05. By the rejection rule, reject the null hypothesis at the 0.05 significance level. Therefore, there is a difference in the mean checking account balances among the four branches.
Post hoc test reveals that the differences occur between the pair of branches. The p-values for branches1–2, branches 2–3, and branches 2–4 are less than the significance level 0.05.
Thus, the branches 1–2, branches 2–3, and branches 2–4 are significantly different in the mean account balance.
Test of hypothesis for ATM usage among the branches:
The null and alternative hypotheses are stated below:
Null hypothesis: There is no difference in ATM use among the branches.
Alternative hypothesis: There is a difference in ATM use among the branches.
Step-by-step procedure to obtain the test statistic using Excel MegaStat is as follows:
- In EXCEL, Select Add-Ins > Mega Stat > Analysis of Variance.
- Choose One-Factor ANOVA.
- In Input Range, select thedata range.
- In Post-Hoc Analysis, Choose When p < 0.05.
- Click OK.
Output obtained using Excel MegaStat is as follows:
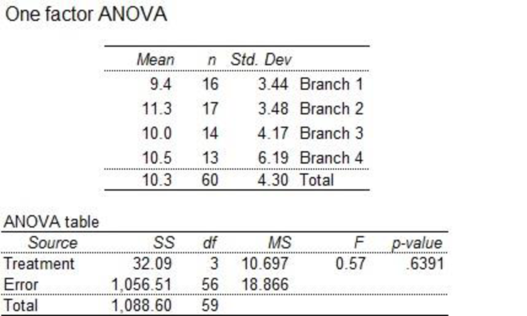
From the above output, the F-test statistic is 0.57 and the p-value is 0.6391.
Conclusion:
Here, the p-value is greater than the significance level. By the rejection rule, one fails to reject the null at the 0.05 significance level. Therefore, it can be concluded that there is no difference in ATM use among the four branches.
Hypothesis test for the customers who have debit cards:
The null and alternative hypotheses are stated below:
Null hypothesis: There is no difference in ATM use between customers who have debit cards and who do not have.
Alternative hypothesis: There is a difference in ATM use by customers who have debit cards and who do not have.
Step-by-step procedure to obtain the test statistic using Excel MegaStat is as follows:
- In EXCEL, Select Add-Ins > Mega Stat > Hypothesis Tests.
- Choose Compare Two Independent Groups.
- Choose Data Input.
- In Group 1, enter the column of without debit cards.
- In Group 2, enter the column of debit cards.
- Enter 0 Under Hypothesized difference.
- Check t-test (pooled variance).
- Choose not equal in alternative.
- Click OK.
Output obtained is represented as follows:
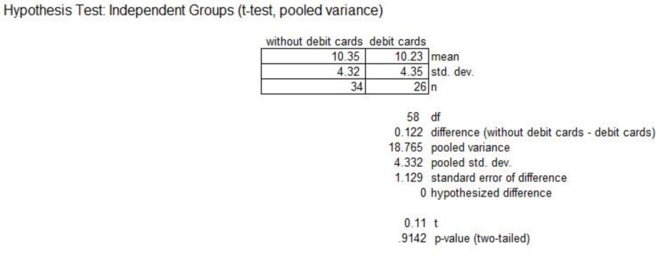
From the above output, the t-test statistic is 0.11 and the p-value is 0.9142.
Conclusion:
Here, the p-value is greater than the significance level. By the rejection rule, one fails to reject the null at the 0.05 significance level. Therefore, there is no difference in ATM use between the customers who have debit cards and who do not have.
Hypothesis test for the customers who pay interest verses those who do not:
The null and alternative hypotheses are stated below:
Null hypothesis: There is no difference in ATM use between customers who pay interest and who do not.
Alternative hypothesis: There is a difference in ATM use by customers who pay interest and who do not.
Step-by-step procedure to obtain the test statistic using Excel MegaStat is as follows:
- In EXCEL, Select Add-Ins > Mega Stat > Hypothesis Tests.
- Choose Compare Two Independent Groups.
- Choose Data Input.
- In Group 1, enter the column of Pay interest.
- In Group 2, enter the column of don’t pay interest.
- Enter 0 Under Hypothesized difference.
- Check t-test (pooled variance).
- Choose not equal in alternative.
- Click OK.
Output obtained is represented as follows:
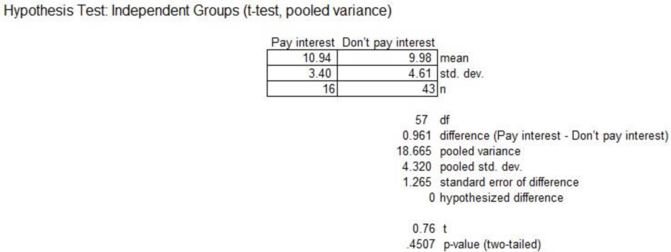
From the above output, the t-test statistic is 0.76 and the p-value is 0.4507.
Conclusion:
Here, the p-value is greater than the significance level. By the rejection rule, one fails to reject the null at the 0.05 significance level. Therefore, there is no difference in ATM use between the customers who pay interest and who do not pay.
Want to see more full solutions like this?
Chapter 12 Solutions
Statistical Techniques in Business and Economics
- 1. If a firm spends more on advertising, is it likely to increase sales? Data on annual sales (in $100,000s) and advertising expenditures (in $10,000s) were collected for 20 firms in order to estimate the model Sales = Po + B₁Advertising + ε. A portion of the regression results is shown in the accompanying table. Intercept Advertising Standard Coefficients Error t Stat p-value -7.42 1.46 -5.09 7.66E-05 0.42 0.05 8.70 7.26E-08 a. Interpret the estimated slope coefficient. b. What is the sample regression equation? C. Predict the sales for a firm that spends $500,000 annually on advertising.arrow_forwardCan you help me solve problem 38 with steps im stuck.arrow_forwardHow do the samples hold up to the efficiency test? What percentages of the samples pass or fail the test? What would be the likelihood of having the following specific number of efficiency test failures in the next 300 processors tested? 1 failures, 5 failures, 10 failures and 20 failures.arrow_forward
- The battery temperatures are a major concern for us. Can you analyze and describe the sample data? What are the average and median temperatures? How much variability is there in the temperatures? Is there anything that stands out? Our engineers’ assumption is that the temperature data is normally distributed. If that is the case, what would be the likelihood that the Safety Zone temperature will exceed 5.15 degrees? What is the probability that the Safety Zone temperature will be less than 4.65 degrees? What is the actual percentage of samples that exceed 5.25 degrees or are less than 4.75 degrees? Is the manufacturing process producing units with stable Safety Zone temperatures? Can you check if there are any apparent changes in the temperature pattern? Are there any outliers? A closer look at the Z-scores should help you in this regard.arrow_forwardNeed help pleasearrow_forwardPlease conduct a step by step of these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points. 4. One-Way ANOVA: Analyze the customer satisfaction scores across four different product categories to determine if there is a significant difference in means. (Hints: The null can be about maintaining status-quo or no difference among groups) H0 = H1=arrow_forward
- Please conduct a step by step of these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points 2. Two-Sample T-Test: Compare the average sales revenue of two different regions to determine if there is a significant difference. (Hints: The null can be about maintaining status-quo or no difference among groups; if alternative hypothesis is non-directional use the two-tailed p-value from excel file to make a decision about rejecting or not rejecting null) H0 = H1=arrow_forwardPlease conduct a step by step of these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points 3. Paired T-Test: A company implemented a training program to improve employee performance. To evaluate the effectiveness of the program, the company recorded the test scores of 25 employees before and after the training. Determine if the training program is effective in terms of scores of participants before and after the training. (Hints: The null can be about maintaining status-quo or no difference among groups; if alternative hypothesis is non-directional, use the two-tailed p-value from excel file to make a decision about rejecting or not rejecting the null) H0 = H1= Conclusion:arrow_forwardPlease conduct a step by step of these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points. The data for the following questions is provided in Microsoft Excel file on 4 separate sheets. Please conduct these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points. 1. One Sample T-Test: Determine whether the average satisfaction rating of customers for a product is significantly different from a hypothetical mean of 75. (Hints: The null can be about maintaining status-quo or no difference; If your alternative hypothesis is non-directional (e.g., μ≠75), you should use the two-tailed p-value from excel file to…arrow_forward
- Please conduct a step by step of these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points. 1. One Sample T-Test: Determine whether the average satisfaction rating of customers for a product is significantly different from a hypothetical mean of 75. (Hints: The null can be about maintaining status-quo or no difference; If your alternative hypothesis is non-directional (e.g., μ≠75), you should use the two-tailed p-value from excel file to make a decision about rejecting or not rejecting null. If alternative is directional (e.g., μ < 75), you should use the lower-tailed p-value. For alternative hypothesis μ > 75, you should use the upper-tailed p-value.) H0 = H1= Conclusion: The p value from one sample t-test is _______. Since the two-tailed p-value is _______ 2. Two-Sample T-Test:…arrow_forwardPlease conduct a step by step of these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points. What is one sample T-test? Give an example of business application of this test? What is Two-Sample T-Test. Give an example of business application of this test? .What is paired T-test. Give an example of business application of this test? What is one way ANOVA test. Give an example of business application of this test? 1. One Sample T-Test: Determine whether the average satisfaction rating of customers for a product is significantly different from a hypothetical mean of 75. (Hints: The null can be about maintaining status-quo or no difference; If your alternative hypothesis is non-directional (e.g., μ≠75), you should use the two-tailed p-value from excel file to make a decision about rejecting or not…arrow_forwardThe data for the following questions is provided in Microsoft Excel file on 4 separate sheets. Please conduct a step by step of these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points. What is one sample T-test? Give an example of business application of this test? What is Two-Sample T-Test. Give an example of business application of this test? .What is paired T-test. Give an example of business application of this test? What is one way ANOVA test. Give an example of business application of this test? 1. One Sample T-Test: Determine whether the average satisfaction rating of customers for a product is significantly different from a hypothetical mean of 75. (Hints: The null can be about maintaining status-quo or no difference; If your alternative hypothesis is non-directional (e.g., μ≠75), you…arrow_forward
 Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt


