
INTERNATIONAL EDITION---Engineering Mechanics: Statics, 14th edition (SI unit)
14th Edition
ISBN: 9780133918922
Author: Russell C. Hibbeler
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Textbook Question
Chapter 11.3, Problem 8P
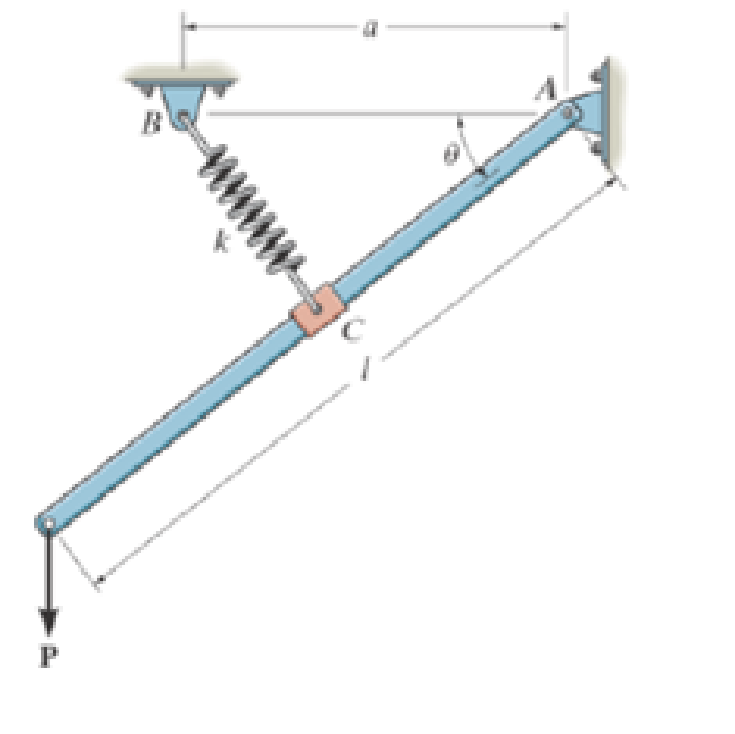
If the unstretched length of the spring is I0, determine the force P needed to hold the bar in the equilibrium position θ. Neglect the weight of the bar.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
ә
レ
Figure below shows a link mechanism in which the link OA rotates uniformly in an
anticlockwise direction at 10 rad/s. the lengths of the various links are OA=75 mm, OB-150 mm,
BC=150 mm, CD-300 mm. Determine for the position shown, the sliding velocity of D.
A
A
B
#
Space Diagram
o NTS (Not-to-Scale)
C
10
=--20125
735)
750 x2.01
اه
2
レ
Tanism in which the link OA mm. O
anticlockwise direction at 10 rad/s, the lengths of the various links are OA=75mm, OB=150mm,
BC=150mm,CD=300mm. Determine for the position shown, the sliding velocity of D.
A
A
Space Diagram
o NT$ (Not-to-Scale)
B
#
C
か
750 x2.01
165
79622
Ashaft fitted with a flywheel rotates at 300 rpm. and drives a machine. The torque
required to drive the machine varies in a cyclic manner over a period of 2 revolutions. The torque drops
from 20,000 Nm to 10,000 Nm uniformly during 90 degrees and remains constant for the following 180
degrees. It then rises uniformly to 35,000 Nm during the next 225 degrees and after that it drops to
20,000 in a uniform manner for 225 degrees, the cycle being repeated thereafter.
Determine the power required to drive the machine and percentage fluctuation in speed, if the driving
torque applied to the shaft is constant and the mass of the flywheel is 12 tonnes with radius of gyration of
500 mm. What is the maximum angular acceleration of the flywheel.
35,000
TNM
20,000
10,000
0
90
270
495
Crank angle 8 degrees
720
Chapter 11 Solutions
INTERNATIONAL EDITION---Engineering Mechanics: Statics, 14th edition (SI unit)
Ch. 11.3 - Each link has a mass of 20 kg.Ch. 11.3 - Determine the magnitude of force P required to...Ch. 11.3 - Determine the angle for equilibrium. The spring...Ch. 11.3 - Determine the angle for equilibrium. The spring...Ch. 11.3 - Prob. 5FPCh. 11.3 - Determine the angle for equilibrium. The spring...Ch. 11.3 - The lamp weighs 10 lb.Ch. 11.3 - Each of the four links has a length L and is pin...Ch. 11.3 - Determine the force screw exerts on the cork of...Ch. 11.3 - Determine the disks rotation if the end of the...
Ch. 11.3 - Prob. 5PCh. 11.3 - Prob. 6PCh. 11.3 - if the uniform inks AB and CD each weigh 10 lb....Ch. 11.3 - If the unstretched length of the spring is I0,...Ch. 11.3 - It vertical forces P1 = P2 = 30 lb act at C and E...Ch. 11.3 - Prob. 10PCh. 11.3 - The spring which always remains vertical. Is...Ch. 11.3 - Prob. 12PCh. 11.3 - Prob. 13PCh. 11.3 - Prob. 14PCh. 11.3 - Prob. 15PCh. 11.3 - Prob. 16PCh. 11.3 - Prob. 17PCh. 11.3 - Determine the angle for equilibrium. The spring...Ch. 11.3 - Determine the stillness k of the spring for...Ch. 11.3 - Determine the horizontal compressive force F...Ch. 11.3 - Prob. 21PCh. 11.3 - Prob. 22PCh. 11.3 - The lever is in balance when the load and block...Ch. 11.3 - If the load F weighs 20 lb and the block G weighs...Ch. 11.3 - Determine the force in the hydraulic cylinder...Ch. 11.7 - Determine the equilibrium positions and...Ch. 11.7 - Prob. 27PCh. 11.7 - If the potential function for a conservative...Ch. 11.7 - Prob. 29PCh. 11.7 - Prob. 30PCh. 11.7 - The rod BD, having negligible weight, passes...Ch. 11.7 - Determine the angle for equilibrium when a weight...Ch. 11.7 - Determine the angle for equilibrium and...Ch. 11.7 - Prob. 34PCh. 11.7 - Prob. 35PCh. 11.7 - The bars each have a mass of 3 Kg one the...Ch. 11.7 - The bars each have a mass of 10 kg and the spring...Ch. 11.7 - Determine the required stiffness k of the spring...Ch. 11.7 - It is unstretched when the rod assembly is in the...Ch. 11.7 - Determine the minimum distance d in order for it...Ch. 11.7 - If the spring is unstretched when = 60. Determine...Ch. 11.7 - The contact at A is smooth, end both are pm...Ch. 11.7 - Determine the steepest grade along which it can...Ch. 11.7 - Determine the weight W2, that is on the pan in...Ch. 11.7 - If the rod is supported by a smooth slider block...Ch. 11.7 - Point C is coincident with B when OA is...Ch. 11.7 - Prob. 47PCh. 11.7 - Prob. 48PCh. 11.7 - If the block has three equal sides of length d,...Ch. 11.7 - Prob. 1RPCh. 11.7 - Determine the horizontal force P required to hold...Ch. 11.7 - Prob. 3RPCh. 11.7 - Prob. 4RPCh. 11.7 - Prob. 5RPCh. 11.7 - Prob. 6RPCh. 11.7 - If both spring DE and BC are unstretched when =...Ch. 11.7 - Prob. 8RP
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- Figure below shows a link mechanism in which the link OA rotates uniformly in an anticlockwise direction at 10 rad/s. the lengths of the various links are OA=75 mm, OB-150 mm, BC=150 mm, CD-300 mm. Determine for the position shown, the sliding velocity of D. A 45 B Space Diagram o NTS (Not-to-Scale) C Darrow_forwardmotion is as follows; 1- Dwell 45°. Plot the displacement diagram for a cam with flat follower of width 14 mm. The required 2- Rising 60 mm in 90° with Simple Harmonic Motion. 3- Dwell 90°. 4- Falling 60 mm for 90° with Simple Harmonic Motion. 5- Dwell 45°. Then design the cam profile to give the above displacement diagram if the minimum circle diameter of the cam is 50 mm.arrow_forwardAn ideal gas, occupying a volume of 0.02 m3 , has a temperature of 25 0C and is at 1.2 bar. The gas is compressed reversibly and adiabatically to a final pressure of 8 bar. Assuming the gas has an adiabatic index of γ = 1.4, calculate (a) the final temperature, (b) the final volume, (c) the work performed during the compression and (d) the heat transferred.arrow_forward
- attached is a past paper question in which we werent given the solution. a solution with clear steps and justification would be massively appreciated thankyou.arrow_forwardin this scenario, when it comes to matrix iterations it states this system is assumed out of phase. why is this?arrow_forwardQ1. A curved beam of a circular cross section of diameter "d" is fixed at one end and subjected to a concentrated load P at the free end (Fig. 1). Calculate stresses at points A and C. Given: P = 800 N, d = 30 mm, a 25 mm, and b = 15 mm. Fig.1 P b B (10 Marks)arrow_forward
- You are working as an engineer in a bearing systems design company. The flow of lubricant inside a hydrodynamic bearing (p = 0.001 kg m-1 s-1) can be approximated as a parallel, steady, two-dimensional, incompressible flow between two parallel plates. The top plate, representing the moving part of the bearing, travels at a constant speed, U, while the bottom plate remains stationary (Figure Q1). The plates are separated by a distance of 2h = 1 cm and are W = 20 cm wide. Their length is L = 10 cm. By applying the above approximations to the Navier-Stokes equations and assuming that end effects can be neglected, the horizontal velocity profile can be shown to be y = +h I 2h = 1 cm x1 y = -h u(y) 1 dP 2μ dx -y² + Ay + B moving plate stationary plate U 2 I2 L = 10 cm Figure Q1: Flow in a hydrodynamic bearing. The plates extend a width, W = 20 cm, into the page.arrow_forwardQuestion 1 You are working as an engineer in a bearing systems design company. The flow of lubricant inside a hydrodynamic bearing (µ = 0.001 kg m¯¹ s¯¹) can be approximated as a parallel, steady, two-dimensional, incompressible flow between two parallel plates. The top plate, representing the moving part of the bearing, travels at a constant speed, U, while the bottom plate remains stationary (Figure Q1). The plates are separated by a distance of 2h = 1 cm and are W = 20 cm wide. Their length is L = 10 cm. By applying the above approximations to the Navier-Stokes equations and assuming that end effects can be neglected, the horizontal velocity profile can be shown to be 1 dP u(y) = 2μ dx -y² + Ay + B y= +h Ꮖ 2h=1 cm 1 x1 y = −h moving plate stationary plate 2 X2 L = 10 cm Figure Q1: Flow in a hydrodynamic bearing. The plates extend a width, W = 20 cm, into the page. (a) By considering the appropriate boundary conditions, show that the constants take the following forms: U U 1 dP A =…arrow_forwardQuestion 2 You are an engineer working in the propulsion team for a supersonic civil transport aircraft driven by a turbojet engine, where you have oversight of the design for the engine intake and the exhaust nozzle, indicated in Figure Q2a. The turbojet engine can operate when provided with air flow in the Mach number range, 0.60 to 0.80. You are asked to analyse a condition where the aircraft is flying at 472 m/s at an altitude of 14,000 m. For all parts of the question, you can assume that the flow path of air through the engine has a circular cross section. (a) ← intake normal shock 472 m/s A B (b) 50 m/s H 472 m/s B engine altitude: 14,000 m exhaust nozzle E F exit to atmosphere diameter: DE = 0.30 m E F diameter: DF = 0.66 m Figure Q2: Propulsion system for a supersonic aircraft. a) When the aircraft is at an altitude of 14,000 m, use the International Standard Atmosphere in the Module Data Book to state the local air pressure and tempera- ture. Thus show that the aircraft speed…arrow_forward
- يكا - put 96** I need a detailed drawing with explanation or in wake, and the top edge of im below the free surface of the water. Determine the hydrothed if hydrostatic on the Plot the displacement diagram for a cam with roller follower of diameter 10 mm. The required motion is as follows; 1- Rising 60 mm in 135° with uniform acceleration and retardation motion. 2- Dwell 90° 3- Falling 60 mm for 135° with Uniform acceleration-retardation motion. Then design the cam profile to give the above displacement diagram if the minimum circle diameter of the cam is 50 mm. =--20125 7357 750 X 2.01arrow_forwardYou are working as an engineer in a bearing systems design company. The flow of lubricant inside a hydrodynamic bearing (µ = 0.001 kg m¯¹ s¯¹) can be approximated as a parallel, steady, two-dimensional, incompressible flow between two parallel plates. The top plate, representing the moving part of the bearing, travels at a constant speed, U, while the bottom plate remains stationary (Figure Q1). The plates are separated by a distance of 2h = 1 cm and are W = 20 cm wide. Their length is L = 10 cm. By applying the above approximations to the Navier-Stokes equations and assuming that end effects can be neglected, the horizontal velocity profile can be shown to be U y = +h У 2h = 1 cm 1 x1 y=-h u(y) = 1 dP 2μ dx -y² + Ay + B moving plate - U stationary plate 2 I2 L = 10 cm Figure Q1: Flow in a hydrodynamic bearing. The plates extend a width, W = 20 cm, into the page. (a) By considering the appropriate boundary conditions, show that the constants take the following forms: A = U 2h U 1 dP…arrow_forwardQuestion 2 You are an engineer working in the propulsion team for a supersonic civil transport aircraft driven by a turbojet engine, where you have oversight of the design for the engine intake and the exhaust nozzle, indicated in Figure Q2a. The turbojet engine can operate when provided with air flow in the Mach number range, 0.60 to 0.80. You are asked to analyse a condition where the aircraft is flying at 472 m/s at an altitude of 14,000 m. For all parts of the question, you can assume that the flow path of air through the engine has a circular cross section. (a) normal shock 472 m/s A B (b) intake engine altitude: 14,000 m D exhaust nozzle→ exit to atmosphere 472 m/s 50 m/s B diameter: DE = 0.30 m EX diameter: DF = 0.66 m Figure Q2: Propulsion system for a supersonic aircraft. F a) When the aircraft is at an altitude of 14,000 m, use the International Standard Atmosphere in the Module Data Book to state the local air pressure and tempera- ture. Thus show that the aircraft speed of…arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L

International Edition---engineering Mechanics: St...
Mechanical Engineering
ISBN:9781305501607
Author:Andrew Pytel And Jaan Kiusalaas
Publisher:CENGAGE L
Mechanical SPRING DESIGN Strategy and Restrictions in Under 15 Minutes!; Author: Less Boring Lectures;https://www.youtube.com/watch?v=dsWQrzfQt3s;License: Standard Youtube License