
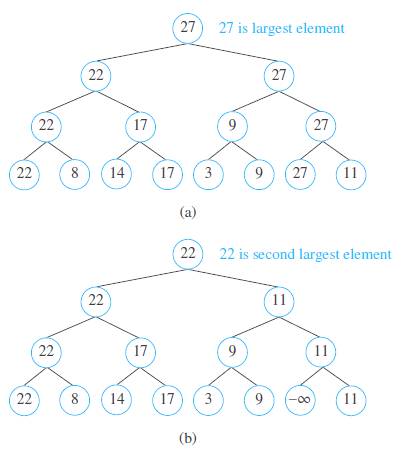
The tournament sort is a sorting algorithm that works by building an ordered binary tree. We represent the elements to be sorted by vertices that sill become the leaves. We build up the tree one level at a time we would construct the tree representing the winners of matches in a tournament Working left to right, we compare pairs of consecutive elements, adding a parent vertex labeled with the larger of the two elements under comparison. We make similar comparisons between labels of vertices at each level until we reach the root of the tree that is labeled with the largest element. The tree constructed by the tournament sort of , 8.14,17,3,9,27,11 is ilinstrated in part(a)ef the figure. Once the argestelementhbeendetermined. The leaf with this labelisrelabeled by -s,which is definedtobelessthanevery element The labels of all vertices on the path from this vertex up to the root of the tree are recalculated, as shown in part (b) of the figure.
This produces the second largest element This process continues until the entire list has been sorted.
20. Construct the binary tree with prefix codes representing these coding schemes.
a) a: 11, e: o, t: 101, s: 100
b) a: 1, e: 01, t: 001, s: 0001, n: 00001
c) a: 1010, e: o, t: 11, s: 1011, n: 1001, 1: 10001
Want to see the full answer?
Check out a sample textbook solution
Chapter 11 Solutions
DISCRETE MATH CONNECT ACCESS
- Answer question S8 stepwisearrow_forwardAnswer questions 8.2.11 and 8.2.12 respectivelyarrow_forward8.4.2 An article in Knee Surgery, Sports Traumatology, Arthroscopy [“Arthroscopic Meniscal Repair with an Absorbable Screw: Results and Surgical Technique” (2005, Vol. 13, pp. 273–279)] showed that only 25 out of 37 tears (67.6%) located between 3 and 6 mm from the meniscus rim were healed. a. Calculate a two-sided 95% confidence interval on the proportion of such tears that will heal. b. Calculate a 95% lower confidence bound on the proportion of such tears that will heal. 8.4.3 An article in the Journal of the American Statistical Association [“Illustration of Bayesian Inference in Normal Data Models Using Gibbs Sampling” (1990, Vol. 85, pp. 972–985)] measured the weight of 30 rats under experiment controls. Suppose that 12 were underweight rats. a. Calculate a 95% two-sided confidence interval on the true proportion of rats that would show underweight from the experiment. b. Using the point estimate of p obtained from the preliminary sample, what sample size is needed to be 95%…arrow_forward
- 8.4.8 Use the data from Exercise 8.4.2 to compute the two-sided Agresti-Coull CI on the proportion of tears that heal. Compare and discuss the relationship of this interval to the one computed in Exercise 8.4.2.arrow_forwardAnswer questions 8.3.7 and 8.4.1 respectivelyarrow_forwardDon't do 14. Please solve 19arrow_forward
- 8.4.7 Use the data from Exercise 8.4.5 to compute the two-sided Agresti-Coull CI on the proportion of digits read correctly. Compare and discuss the relationship of this interval to the one computed in Exercise 8.4.5.arrow_forward8.6.5 Consider the fuel rod enrichment data described in Exercise 8.2.11. Compute a 90% prediction interval on the enrichment of the next rod tested. Compare the length of the prediction interval with the length of the 99% CI on the population mean.arrow_forward8.4.4 The Arizona Department of Transportation wishes to survey state residents to determine what proportion of the population would like to increase statewide highway speed limits from 65 mph to 75 mph. How many residents does the department need to survey if it wants to be at least 99% confident that the sample proportion is within 0.05 of the true proportion? 8.4.5 The U.S. Postal Service (USPS) has used optical character recognition (OCR) since the mid-1960s. In 1983, USPS began deploying the technology to major post offices throughout the country (www.britannica.com). Suppose that in a random sample of 500 handwritten zip code digits, 466 were read correctly. a. Construct a 95% confidence interval for the true proportion of correct digits that can be automatically read. b. What sample size is needed to reduce the margin of error to 1%? c. How would the answer to part (b) change if you had to assume that the machine read only one-half of the digits correctly?arrow_forward
 Discrete Mathematics and Its Applications ( 8th I...MathISBN:9781259676512Author:Kenneth H RosenPublisher:McGraw-Hill Education
Discrete Mathematics and Its Applications ( 8th I...MathISBN:9781259676512Author:Kenneth H RosenPublisher:McGraw-Hill Education Mathematics for Elementary Teachers with Activiti...MathISBN:9780134392790Author:Beckmann, SybillaPublisher:PEARSON
Mathematics for Elementary Teachers with Activiti...MathISBN:9780134392790Author:Beckmann, SybillaPublisher:PEARSON
 Thinking Mathematically (7th Edition)MathISBN:9780134683713Author:Robert F. BlitzerPublisher:PEARSON
Thinking Mathematically (7th Edition)MathISBN:9780134683713Author:Robert F. BlitzerPublisher:PEARSON Discrete Mathematics With ApplicationsMathISBN:9781337694193Author:EPP, Susanna S.Publisher:Cengage Learning,
Discrete Mathematics With ApplicationsMathISBN:9781337694193Author:EPP, Susanna S.Publisher:Cengage Learning, Pathways To Math Literacy (looseleaf)MathISBN:9781259985607Author:David Sobecki Professor, Brian A. MercerPublisher:McGraw-Hill Education
Pathways To Math Literacy (looseleaf)MathISBN:9781259985607Author:David Sobecki Professor, Brian A. MercerPublisher:McGraw-Hill Education





