INTRODUCTORY STATISTICS (LOOSELEAF)
3rd Edition
ISBN: 9780135163146
Author: Gould
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 11, Problem 57CRE
Triglycerides and Gender Using the NHANES data, we performed an ANOVA to test whether gender is associated with level of triglycerides, a form of fat, in the blood. ANOVA and t-test output from a two-sample t-test is shown. In both cases, we are testing the hypothesis that the mean triglyceride levels for men and women are different. Assume that conditions for both tests have been met.
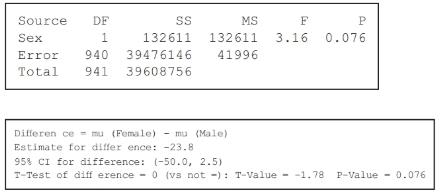
Compare the output by comparing p-values. Square the t-value to see what you get for comparison. Explain.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
A program administrator at a high school wanted to see if there was a significant association between experiencing verbal
abuse and anxiety in teenage women. She wasn't sure what to expect. Perform the correct statistical procedure to assess the
degree of association between these variables and interpret your findings. Set alpha = .05 for your statistical test. YOU CAN
EITHER TYPE YOUR WORK OUT HERE OR TAKE A PICTURE OF HANDWRITTEN WORK AND EMAIL IT TO ME.
%3D
Anxiety
Verbal Abuse
5.
4.
4.
Factor V is a protein involved in the forming of blood clots. The higher the level of factor V, the faster the blood clots. A Blood Transfusions Service is interested in the effects of sterilization of blood plasma because factor V is unstable and may break down during sterilization. The table below gives measured levels of factor V in blood samples from nine donors. Both pre- and post-sterilization measurements are given for each person. Test whether the mean factor V levels are different post-sterilization. Run the test at a 5% level of significance. Give each of the following 1) the appropriate null and alternative hypotheses; 2) the appropriate test; 3) the decision rule; 4) the calculation of the test statistic; and 5) your conclusion including a comparison to alpha or the critical value. Factor V measurements
Donor number
Pre-Sterilization
Post-Sterilization
1
1025
985
2
1060
1030
3
953
923
4
847
892
5
750
650
6
855
759…
hi, if you could help me with this problem that would be very helpful. thank you.
Chapter 11 Solutions
INTRODUCTORY STATISTICS (LOOSELEAF)
Ch. 11 - In Exercises 11.1 and 11.2, for each situation,...Ch. 11 - In Exercises 11.1 and 11.2, for each situation,...Ch. 11 - Bonferroni Correction (Example 1) Suppose you have...Ch. 11 - Prob. 4SECh. 11 - Apartment Rents Random samples of rents for...Ch. 11 - Prob. 6SECh. 11 - Gas Prices The website Gasbuddy.com reports the...Ch. 11 - More Gas Prices The following table shows the...Ch. 11 - Prob. 9SECh. 11 - Prob. 10SE
Ch. 11 - Gas Price Intervals Use the data from exercise...Ch. 11 - Gas Price Intervals Use the data from exercise...Ch. 11 - Prob. 13SECh. 11 - Baseball Position and Hits Use the data in the...Ch. 11 - Comparing F -Values from Boxplots (Example 3)...Ch. 11 - Comparing F -Values from Boxplots Refer to the...Ch. 11 - Marital Status and Cholesterol (Example 4) Refer...Ch. 11 - Marital Status and Blood Pressure Test the...Ch. 11 - Schoolwork and Class (Example 5) A random survey...Ch. 11 - TV Hours A random survey was done at a small...Ch. 11 - Schoolwork and Class Use the information for...Ch. 11 - TV Hours Use the information for exercise 11.20....Ch. 11 - Schoolwork Again Go back to the information in...Ch. 11 - TV Hours Again Go back to the information in...Ch. 11 - Pulse Rates (Example 6) Pulse rates were taken for...Ch. 11 - UCLA Music Survey The figure shows side-by-side...Ch. 11 - Commute Times by Method A survey was given to...Ch. 11 - Gas Price ANOVA Based on the following output,...Ch. 11 - Apartment Rents (Example 7) Samples of rents for...Ch. 11 - Study Hours by Major Three independent random...Ch. 11 - Salary by Type of College Information was gathered...Ch. 11 - Draft Lottery When the draft lottery for military...Ch. 11 - Reaction Times for Athletes A random sample of...Ch. 11 - Tomato Plants and Colored Light Jennifer Brogan, a...Ch. 11 - GPAs by Seating Choice A random sample of students...Ch. 11 - Reading Comprehension Sixty-six reading students...Ch. 11 - Hours of Steep and Health Status In a study done...Ch. 11 - Happiness and Age Category StatCrunch surveyed...Ch. 11 - Prob. 41SECh. 11 - House Prices Tukey HSD confidence intervals (with...Ch. 11 - GPA and Row (Example 8) A random sample of...Ch. 11 - Reading Scores by Teaching Method Refer to...Ch. 11 - Reaction Distances Use the data given in exercise...Ch. 11 - Study Hours Use the data given in exercise 11.32....Ch. 11 - Prob. 47SECh. 11 - Tomatoes Use the data given in exercise 11.36....Ch. 11 - Concern over Nuclear Power Following the...Ch. 11 - Social Media Use A StatCrunch survey asked...Ch. 11 - Happiness and Age Consider the data from the...Ch. 11 - GPA and Row Number Suppose you collect data on...Ch. 11 - Contacting Mother Professors of ethics (Eth),...Ch. 11 - Ideal Percentage to Charity Professors of ethics...Ch. 11 - Actual Percentage to Charity Professors of ethics...Ch. 11 - Hours of Television by Age Group The StatCrunch...Ch. 11 - Triglycerides and Gender Using the NHANES data, we...Ch. 11 - Cholesterol and Gender Using NHANES data, we...
Additional Math Textbook Solutions
Find more solutions based on key concepts
Provide an example of a qualitative variable and an example of a quantitative variable.
Elementary Statistics ( 3rd International Edition ) Isbn:9781260092561
For Problems 23-28, write in simpler form, as in Example 4. logbFG
Finite Mathematics for Business, Economics, Life Sciences and Social Sciences
(a) Make a stem-and-leaf plot for these 24 observations on the number of customers who used a down-town CitiBan...
APPLIED STAT.IN BUS.+ECONOMICS
Fill in each blank so that the resulting statement is true.
1. The degree of the polynomial function is _____....
Algebra and Trigonometry (6th Edition)
1. How is a sample related to a population?
Elementary Statistics: Picturing the World (7th Edition)
NOTE: Write your answers using interval notation when appropriate.
CHECKING ANALYTIC SKILLS Fill in each blank ...
Graphical Approach To College Algebra
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.Similar questions
- Ankle Brachial Index. The ankle brachial index (ABI) compares the blood pressure of a patient’s arm to the blood pressure of the patient’s leg. The ABI can be an indicator of different diseases, including arterial diseases. A healthy (or normal) ABI is 0.9 or greater. In a study by M. McDermott et al. titled “Sex Differences in Peripheral Arterial Disease: Leg Symptoms and Physical Functioning” (Journal of the American Geriatrics Society, Vol. 51, No. 2, pp. 222–228), the researchers obtained the ABI of 187 women with peripheral arterial disease. The results were a mean ABI of 0.64 with a standard deviation of 0.15. At the 1% significance level, do the data provide sufficient evidence to conclude that, on average, women with peripheral arterial disease have an unhealthy ABI?arrow_forwardAs an occupational health epidemiologist you are required to measure the effect of stress on the workers in your manufacturing plant. Two different tests previously developed to measure stress in industrial workers are selected: stress test alpha and stress test delta. The sensitivity and specificity of each test are shown below. Which test generates the greatest proportion of false negatives? Stress Test Alpha Stress Test Delta Sensitivity = 60% 75% M Specificity = 95% 90% Stress Test Alpha Stress Test Deltaarrow_forwardA test was conducted of two overnight mail delivery services. Two samples of identical deliveries were set up so that both delivery services were notified of the need for a delivery at the same time. The hours required to make each delivery follow. Do the data shown suggest a difference in the delivery times for the two services? Use a .05 le of significance for the test. Use Table 1 of Appendix B. Click on the datafile logo to reference the data. DATA file Service Delivery 1 2 24.5 26.0 28.0 21.0 18.0 36.0 25.0 21.0 24.0 26.0 31.0 28.0 25.5 32.0 20.0 19.5 28.0 29.0 22.0 23.5 29.5 30.0 3 4 6. 9. 10 11 What is the z-statistic? If required, round your answer to two decimal places. Enter negative values as negative number, if necessary. .30 What is the p-value? If required, round your answer to two decimal places. .7642 Conclude:arrow_forward
- A researcher hypothesizes that different colors of cars result in different average speeds. To test this claim, she took a random sample of 20 people who own 4 different colors of colors of cars (n = 20, N = 80, G = 4), and she then tracks their average speed on the highway for a week of driving. The following ANOVA table has some of her results. Please help her answer her research questions by completing the following ANOVA table below and answering the follow-up questions. Be sure to label your answers with the appropriate letter and show all your work! Source Sums of Squares df Mean Square F Effect (between) Error (within) 100.90 ------ Total 170.10 ------- ------ a) What is the Sums of Squares (SS) between (effect)? b) What are the Mean Square (MS) between (effect) and the MS within (error)? c) What are the degrees of freedom (df) between, the df within, and the df total? d) What is the overall F-statistic? e) Based on the…arrow_forwardA test was conducted of two overnight mail delivery services. Two samples of identical deliveries were set up so that both delivery services were notified of the need for a delivery at the same time. The hours required to make each delivery follow. Do the data shown suggest a difference in the delivery times for the two services? Use a .05 level of significance for the test. Use Table 1 of Appendix B. Click on the datafile logo to reference the data. DATA file Service Delivery 1 2 24.5 26.0 28.0 21.0 18.0 36.0 25.0 21.0 24.0 26.0 31.0 28.0 25.5 32.0 20.0 19.5 28.0 29.0 22.0 23.5 29.5 30.0 What is the z-statistic? If required, round your answer to two decimal places. Enter negative values as negative number, if necessary. -.31 What is the p-value? If required, round your answer to two decimal places. .76 Conclude. 12345 7890:=arrow_forwardWe will be using stress dataset to compare the stress level of college students and the general population. Stress level score is based on three metrics, 1. Responsiveness, 2. Exertion Balance, and 3. Sleep patterns. The scale is from 0 to 30, the lower the score the less stress. Use ANOVA to answer the following questions: Question Generate a boxplot of stress score by the group using proc boxplot in SAS. Describe your observations. Compare the means and variances of stress score by group. Explain if any of the assumptions of ANOVA is violated. Write out the ANOVA model. Assuming you want to compare the stress level of college students and the general population, write out the null and the alternative hypotheses. Fit a one-way ANOVA to compare the stress score for the college students and the general population. Using the result in question 5, test the hypothesis and make a statistical decision. What further statistical analysis might you perform if the null hypothesis is rejected.arrow_forward
- A study examined the link between sodium intake and blood pressure inchildren and adolescents between the ages of 8 and 18. The mean systolicblood pressure (SBP) was 112.8 mm Hg among the 636 study participants whowere overweight or obese and consumed high levels of sodium. Based on thisdata the researchers need to decide if they believe that the mean systolicblood pressure for the group was higher than for other groups. a. What would be a Type I error in this context? Your answer shouldconsist of both the researchers’ decision and what the reality is. b. What would be a Type II error in this context? Your answer shouldconsist of both the researchers’ decision and what the reality is.arrow_forwardplease do the full problem!arrow_forwardHow is my work? Should anything be changed? A researcher wanted to examine the effect of a new math study skills program on second graders. Fifteen students took the new study skills program for six weeks and then took a standardized math exam, which is known to have a population mean score of µ = 80. The scores on the exam for the 15 students (who took the study skills program) are reported below. Scores on the standardized math exam for the 15 students:78, 85, 83, 75, 81, 85, 84, 82, 78, 83, 78, 90, 85, 83, 81 Test to see whether the students who took the new study skills program have significantly higher test scores than the national average. Perform the appropriate test in SPSS using α = .05 a. State the null and alternative hypotheses below. Null hypothesis: H0: μ = 80. Alternative hypothesis: H1: μ > 80 b. What type of test should be run on the data (be specific with the exact name of the test)? For example, don’t just state “t test,” but the specific t-test used. One sample…arrow_forward
- Social functioning is the ability for an individual to interact or adapt in a normal or usual way in social environments. Albert et al. (2007) asked if relatives of patients with OCD are as healthy as those in general healthy populations. They measured the social functioning of relatives using a 36-item survey with scores ranging from 0 (worst possible health) to 100 (best possible health). The mean score for the general healthy population was 77.43. Using the sample data, test whether there was a difference. Scores: 20, 60, 48, 92, 50, 82, 48, 90, 30, 68, 43, 54, 60, 62, 94, 67, 63, 85 State hypotheses 2. Set the criteria using α= 0.05 3. Calculate t 4. What should you conclude?arrow_forwardWhen nicotine is absorbed by the body, cotinine is produced. A measurement of cotinine is therefore a good indicator of how much a person smokes. In the table below are the number of cigarettes smoked per day and the subjects’ cotinine level (ng/mL). Use a significance level of 0.05 and Spearman’s Rank correlation to determine if there is an association between the number of cigarettes smoked and the subjects’ cotinine level. State the null and alternative hypothesis Calculate the test statistic The critical value, and your decision and conclusion. Cigarettes (per day) 60 10 4 15 10 1 20 8 7 10 10 20 Cotinine 179 283 75.6 174 209 9.51 350 1.85 43.4 25.1 408 344arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Big Ideas Math A Bridge To Success Algebra 1: Stu...
Algebra
ISBN:9781680331141
Author:HOUGHTON MIFFLIN HARCOURT
Publisher:Houghton Mifflin Harcourt
Statistics 4.1 Point Estimators; Author: Dr. Jack L. Jackson II;https://www.youtube.com/watch?v=2MrI0J8XCEE;License: Standard YouTube License, CC-BY
Statistics 101: Point Estimators; Author: Brandon Foltz;https://www.youtube.com/watch?v=4v41z3HwLaM;License: Standard YouTube License, CC-BY
Central limit theorem; Author: 365 Data Science;https://www.youtube.com/watch?v=b5xQmk9veZ4;License: Standard YouTube License, CC-BY
Point Estimate Definition & Example; Author: Prof. Essa;https://www.youtube.com/watch?v=OTVwtvQmSn0;License: Standard Youtube License
Point Estimation; Author: Vamsidhar Ambatipudi;https://www.youtube.com/watch?v=flqhlM2bZWc;License: Standard Youtube License