
It is often useful for companies to know who their customers are and how they became customers. A credit card company is interested in whether the owner of the card applied for the card on his or her own or was contacted by a telemarketer. The company obtained the following sample information regarding end-of-the-month balances for the two groups.
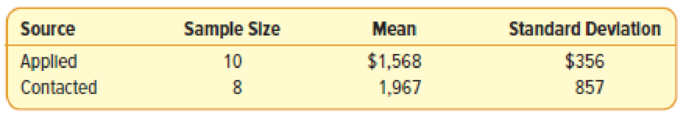
Is it reasonable to conclude the
- (a) State the null hypothesis and the alternate hypothesis.
- (b) How many degrees of freedom are there?
- (c) What is the decision rule?
- (d) What is the value of the test statistic?
- (e) What is your decision regarding the null hypothesis?
- (f) Interpret the result.
a.
State the null and the alternative hypothesis.
Answer to Problem 3SR
The null hypothesis is
The alternative hypothesis is
Explanation of Solution
In this context,
The hypotheses are given below:
In this context,
Null hypothesis:
Alternative hypothesis:
Thus, the null hypothesis is
b.
Find the number of degrees of freedom.
Answer to Problem 3SR
Thus, the number of degrees of freedom is 8.
Explanation of Solution
Calculation:
For t distribution with the two independent random samples, the degrees of freedom is as follows:
Substitute
Thus, the number of degrees of freedom is 8.
c.
Determine the decision rule.
Explanation of Solution
Step-by-step procedure to obtain the critical value using MINITAB software:
- Choose Graph > Probability Distribution Plot choose View Probability > OK.
- From Distribution, choose ‘t’ distribution.
- In Degrees of freedom, enter 8.
- Click the Shaded Area tab.
- Choose Probability and left Tail for the region of the curve to shade.
- Enter the Probability value as 0.05.
- Click OK.
Output obtained using MINITAB software is given below:
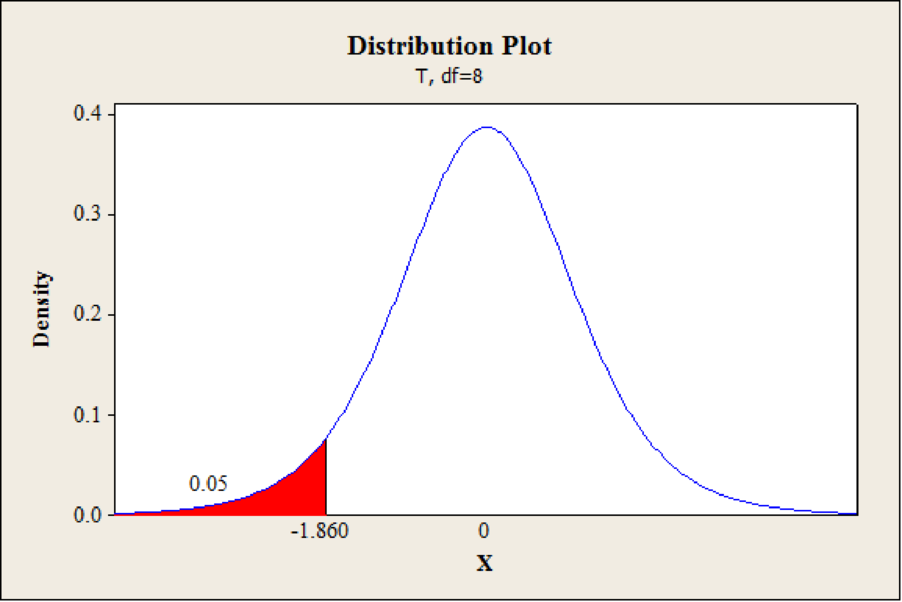
From the MINITAB output, the critical value is –1.860.
The decision rule is as follows:
If
d.
Find the value of test statistic.
Answer to Problem 3SR
Thus, the value of the test statistic is –1.234.
Explanation of Solution
Calculation:
Test statistic:
The test statistics is obtained as follows:
Thus, the test statistic is –1.234.
e.
Determine the decision regarding
Answer to Problem 3SR
The decision is that fail to reject the null hypothesis
Explanation of Solution
Decision:
The critical value is –1.860 and the value of test statistic is –1.234.
The value of test statistic is greater than the critical value.
That is,
From the decision rule, fail to reject the null hypothesis.
f.
Determine the interpretation of the study.
Answer to Problem 3SR
The interpretation is that there is no evidence that the mean balance is larger for the credit card holders contacted by telemarketers than those who applied on their won for the card.
Explanation of Solution
Interpretation:
From the decision rule, fail to reject the null hypothesis.
Therefore, there is no evidence that the mean balance is larger for the credit card holders contacted by telemarketers than those who applied on their won for the card.
Want to see more full solutions like this?
Chapter 11 Solutions
Loose Leaf for Statistical Techniques in Business and Economics
- 2. Hypothesis Testing - Two Sample Means A nutritionist is investigating the effect of two different diet programs, A and B, on weight loss. Two independent samples of adults were randomly assigned to each diet for 12 weeks. The weight losses (in kg) are normally distributed. Sample A: n = 35, 4.8, s = 1.2 Sample B: n=40, 4.3, 8 = 1.0 Questions: a) State the null and alternative hypotheses to test whether there is a significant difference in mean weight loss between the two diet programs. b) Perform a hypothesis test at the 5% significance level and interpret the result. c) Compute a 95% confidence interval for the difference in means and interpret it. d) Discuss assumptions of this test and explain how violations of these assumptions could impact the results.arrow_forward1. Sampling Distribution and the Central Limit Theorem A company produces batteries with a mean lifetime of 300 hours and a standard deviation of 50 hours. The lifetimes are not normally distributed—they are right-skewed due to some batteries lasting unusually long. Suppose a quality control analyst selects a random sample of 64 batteries from a large production batch. Questions: a) Explain whether the distribution of sample means will be approximately normal. Justify your answer using the Central Limit Theorem. b) Compute the mean and standard deviation of the sampling distribution of the sample mean. c) What is the probability that the sample mean lifetime of the 64 batteries exceeds 310 hours? d) Discuss how the sample size affects the shape and variability of the sampling distribution.arrow_forwardA biologist is investigating the effect of potential plant hormones by treating 20 stem segments. At the end of the observation period he computes the following length averages: Compound X = 1.18 Compound Y = 1.17 Based on these mean values he concludes that there are no treatment differences. 1) Are you satisfied with his conclusion? Why or why not? 2) If he asked you for help in analyzing these data, what statistical method would you suggest that he use to come to a meaningful conclusion about his data and why? 3) Are there any other questions you would ask him regarding his experiment, data collection, and analysis methods?arrow_forward
- Businessarrow_forwardWhat is the solution and answer to question?arrow_forwardTo: [Boss's Name] From: Nathaniel D Sain Date: 4/5/2025 Subject: Decision Analysis for Business Scenario Introduction to the Business Scenario Our delivery services business has been experiencing steady growth, leading to an increased demand for faster and more efficient deliveries. To meet this demand, we must decide on the best strategy to expand our fleet. The three possible alternatives under consideration are purchasing new delivery vehicles, leasing vehicles, or partnering with third-party drivers. The decision must account for various external factors, including fuel price fluctuations, demand stability, and competition growth, which we categorize as the states of nature. Each alternative presents unique advantages and challenges, and our goal is to select the most viable option using a structured decision-making approach. Alternatives and States of Nature The three alternatives for fleet expansion were chosen based on their cost implications, operational efficiency, and…arrow_forward
- The following ordered data list shows the data speeds for cell phones used by a telephone company at an airport: A. Calculate the Measures of Central Tendency from the ungrouped data list. B. Group the data in an appropriate frequency table. C. Calculate the Measures of Central Tendency using the table in point B. 0.8 1.4 1.8 1.9 3.2 3.6 4.5 4.5 4.6 6.2 6.5 7.7 7.9 9.9 10.2 10.3 10.9 11.1 11.1 11.6 11.8 12.0 13.1 13.5 13.7 14.1 14.2 14.7 15.0 15.1 15.5 15.8 16.0 17.5 18.2 20.2 21.1 21.5 22.2 22.4 23.1 24.5 25.7 28.5 34.6 38.5 43.0 55.6 71.3 77.8arrow_forwardII Consider the following data matrix X: X1 X2 0.5 0.4 0.2 0.5 0.5 0.5 10.3 10 10.1 10.4 10.1 10.5 What will the resulting clusters be when using the k-Means method with k = 2. In your own words, explain why this result is indeed expected, i.e. why this clustering minimises the ESS map.arrow_forwardwhy the answer is 3 and 10?arrow_forward
 Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning



