
(a)
Interpretation:
The moment of inertia of a linear molecule of the form ABC has to be derived.
Concept Introduction:
Moment of inertia:
The moment of inertia of a molecule is the mass of each atom multiplied by the square of its perpendicular distance from the axis of rotation.
(a)
Explanation of Solution
Consider a triatomic linear molecule of the form ABC. Let,
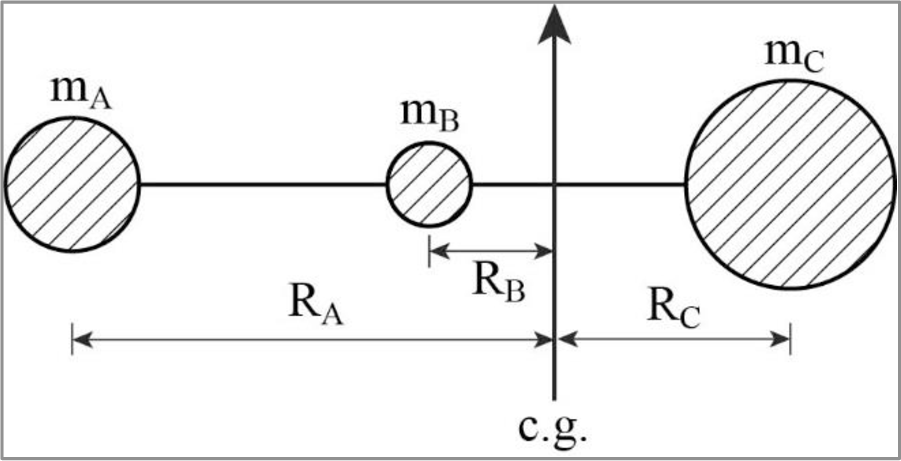
Figure.1
Now,
Moment around the center of gravity,
Then,
Now substitute the value of equation 3 in equation 2.
Now substituting the equation 4 in equation 1,
Now substituting the value of
It can be rearranged as
This is the expression for the moment of inertia of a triatomic linear molecule of the form ABC.
(b)
Interpretation:
The rotational constants and moment of inertia of
Concept Introduction:
The gap between two adjacent spectral lines is
(b)
Answer to Problem 11.5P
The rotational constants of
Explanation of Solution
Given data:

Figure.2
Calculation of rotational constants:
For
The gap between the two spectral lines,
Now
For
The gap between the two spectral lines,
Now
Therefore, the rotational constants for
Calculation of moment of inertia:
For
As
So for
So for
Therefore, the moment of inertia of
(c)
Interpretation:
The
Concept Introduction:
The bond length of a given molecule can be found out from its moment of inertia as moment of inertia is the product of mass and bond length.
(c)
Answer to Problem 11.5P
The
Explanation of Solution
The moment of inertia of
It can be arranged further,
Now
Where,
After putting the value of moment of inertia into the above equation,
After solving this complex equations, the outcome is
Want to see more full solutions like this?
Chapter 11 Solutions
Elements Of Physical Chemistry
- Identify and provide a concise explanation of a specific analytical instrument capable of detecting and quantifying trace compounds in food samples. Emphasise the instrumental capabilities relevant to trace compound analysis in the nominated food. Include the specific application name (eg: identification and quantification of mercury in salmon), outline a brief description of sample preparation procedures, and provide a summary of the obtained results from the analytical process.arrow_forwardIdentify and provide an explanation of what 'Seperation Science' is. Also describe its importance with the respect to the chemical analysis of food. Provide specific examples.arrow_forward5. Propose a Synthesis for the molecule below. You may use any starting materials containing 6 carbons or less (reagents that aren't incorporated into the final molecule such as PhзP do not count towards this total, and the starting material can have whatever non-carbon functional groups you want), and any of the reactions you have learned so far in organic chemistry I, II, and III. Your final answer should show each step separately, with intermediates and conditions clearly drawn. H3C CH3arrow_forward
- State the name and condensed formula of isooxazole obtained by reacting acetylacetone and hydroxylamine.arrow_forwardState the name and condensed formula of the isothiazole obtained by reacting acetylacetone and thiosemicarbazide.arrow_forwardProvide the semi-developed formula of isooxazole obtained by reacting acetylacetone and hydroxylamine.arrow_forward
- Given a 1,3-dicarbonyl compound (R1-CO-CH2-CO-R2), indicate the formula of the compound obtaineda) if I add hydroxylamine (NH2OH) to give an isooxazole.b) if I add thiosemicarbazide (NH2-CO-NH-NH2) to give an isothiazole.arrow_forwardAn orange laser has a wavelength of 610 nm. What is the energy of this light?arrow_forwardThe molar absorptivity of a protein in water at 280 nm can be estimated within ~5-10% from its content of the amino acids tyrosine and tryptophan and from the number of disulfide linkages (R-S-S-R) between cysteine residues: Ε280 nm (M-1 cm-1) ≈ 5500 nTrp + 1490 nTyr + 125 nS-S where nTrp is the number of tryptophans, nTyr is the number of tyrosines, and nS-S is the number of disulfide linkages. The protein human serum transferrin has 678 amino acids including 8 tryptophans, 26 tyrosines, and 19 disulfide linkages. The molecular mass of the most dominant for is 79550. Predict the molar absorptivity of transferrin. Predict the absorbance of a solution that’s 1.000 g/L transferrin in a 1.000-cm-pathlength cuvet. Estimate the g/L of a transferrin solution with an absorbance of 1.50 at 280 nm.arrow_forward
- In GC, what order will the following molecules elute from the column? CH3OCH3, CH3CH2OH, C3H8, C4H10arrow_forwardBeer’s Law is A = εbc, where A is absorbance, ε is the molar absorptivity (which is specific to the compound and wavelength in the measurement), and c is concentration. The absorbance of a 2.31 × 10-5 M solution of a compound is 0.822 at a wavelength of 266 nm in a 1.00-cm cell. Calculate the molar absorptivity at 266 nm.arrow_forwardHow to calculate % of unknown solution using line of best fit y=0.1227x + 0.0292 (y=2.244)arrow_forward
 ChemistryChemistryISBN:9781305957404Author:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCostePublisher:Cengage Learning
ChemistryChemistryISBN:9781305957404Author:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCostePublisher:Cengage Learning ChemistryChemistryISBN:9781259911156Author:Raymond Chang Dr., Jason Overby ProfessorPublisher:McGraw-Hill Education
ChemistryChemistryISBN:9781259911156Author:Raymond Chang Dr., Jason Overby ProfessorPublisher:McGraw-Hill Education Principles of Instrumental AnalysisChemistryISBN:9781305577213Author:Douglas A. Skoog, F. James Holler, Stanley R. CrouchPublisher:Cengage Learning
Principles of Instrumental AnalysisChemistryISBN:9781305577213Author:Douglas A. Skoog, F. James Holler, Stanley R. CrouchPublisher:Cengage Learning Organic ChemistryChemistryISBN:9780078021558Author:Janice Gorzynski Smith Dr.Publisher:McGraw-Hill Education
Organic ChemistryChemistryISBN:9780078021558Author:Janice Gorzynski Smith Dr.Publisher:McGraw-Hill Education Chemistry: Principles and ReactionsChemistryISBN:9781305079373Author:William L. Masterton, Cecile N. HurleyPublisher:Cengage Learning
Chemistry: Principles and ReactionsChemistryISBN:9781305079373Author:William L. Masterton, Cecile N. HurleyPublisher:Cengage Learning Elementary Principles of Chemical Processes, Bind...ChemistryISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY
Elementary Principles of Chemical Processes, Bind...ChemistryISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY





