
MML PRECALCULUS ENHANCED
7th Edition
ISBN: 9780134119250
Author: Sullivan
Publisher: INTER PEAR
expand_more
expand_more
format_list_bulleted
Textbook Question
Chapter 10.3, Problem 83AE
†In Problems 79-83, use the fact that the orbit of a planet about the Sun is an ellipse, with the Sun at one focus. The aphelion of a planet is its greatest distance from the Sun, and the perihelion is its shortest distance. The mean distance of a planet from the Sun is the length of the semimajor axis of the elliptical orbit. See the illustration.
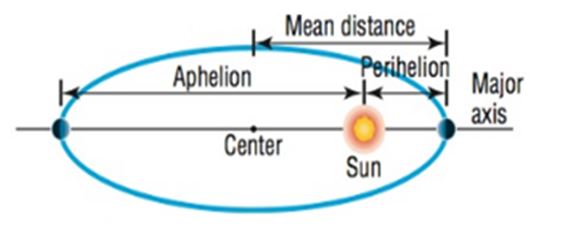
Elliptical Orbit A planet orbits a star in an elliptical orbit with the star located at one focus. The perihelion of the planet is 5 million miles. The eccentricity e of a
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
A circle of radius r centered at the point (0,r) in the plane will intersect the y-axis at the origin and the point A=(0,2r), as pictured below. A line passes through the point A and the point C=(11/2,0) on the x-axis. In this problem, we will investigate the coordinates of the intersection point B between the circle and the
line, as 1 → ∞
A=(0,2r)
B
(0,0)
(a) The line through A and C has equation:
y=
2
117
x+27
(b) The x-coordinate of the point B is
4472
121,2
+4
40
(c) The y-coordinate of the point B is
+27
121
44
(d) The limit as r→ ∞ of the x-coordinate of B is
121
(if your answer is oo, write infinity).
1. Show that the vector field
F(x, y, z)
=
(2x sin ye³)ix² cos yj + (3xe³ +5)k
satisfies the necessary conditions for a conservative vector field, and find a potential function for
F.
i need help please
Chapter 10 Solutions
MML PRECALCULUS ENHANCED
Ch. 10.2 - The formula for the distance d from P 1 =( x 1 , y...Ch. 10.2 - To complete the square of x 2 4x , add_______...Ch. 10.2 - Use the Square Root Method to find the real...Ch. 10.2 - The point that is symmetric with respect to the...Ch. 10.2 - To graph y= ( x3 ) 2 +1 , shift the graph of y= x...Ch. 10.2 - A( n ) _____ is the collection of all points in a...Ch. 10.2 - The line through the focus and perpendicular to...Ch. 10.2 - For the parabola y 2 =4ax , the line segment...Ch. 10.2 - Answer Problems 9-12 using the figure. If a0 , the...Ch. 10.2 - Answer Problems 9-12 using the figure. The...
Ch. 10.2 - Answer Problems 9-12 using the figure. If a=4 ,...Ch. 10.2 - Answer Problems 9-12 using the figure. If a=4 ,...Ch. 10.2 - In Problems 13-20, the graph of a parabola is...Ch. 10.2 - In Problems 13-20, the graph of a parabola is...Ch. 10.2 - In Problems 13-20, the graph of a parabola is...Ch. 10.2 - In Problems 13-20, the graph of a parabola is...Ch. 10.2 - In Problems 13-20, the graph of a parabola is...Ch. 10.2 - In Problems 13-20, the graph of a parabola is...Ch. 10.2 - In Problems 13-20, the graph of a parabola is...Ch. 10.2 - In Problems 13-20, the graph of a parabola is...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 21-38, find the equation of the...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 39-56, find the vertex, focus, and...Ch. 10.2 - In Problems 57-64, write an equation for each...Ch. 10.2 - In Problems 57-64, write an equation for each...Ch. 10.2 - In Problems 57-64, write an equation for each...Ch. 10.2 - In Problems 57-64, write an equation for each...Ch. 10.2 - In Problems 57-64, write an equation for each...Ch. 10.2 - In Problems 57-64, write an equation for each...Ch. 10.2 - In Problems 57-64, write an equation for each...Ch. 10.2 - In Problems 57-64, write an equation for each...Ch. 10.2 - Satellite Dish A satellite dish is shaped like a...Ch. 10.2 - Constructing a TV Dish A cable TV receiving dish...Ch. 10.2 - Constructing a Flashlight The reflector of a...Ch. 10.2 - Constructing a Headlight A sealed-beam headlight...Ch. 10.2 - Suspension Bridge The cables of a suspension...Ch. 10.2 - Suspension Bridge The cables of a suspension...Ch. 10.2 - Searchlight A searchlight is shaped like a...Ch. 10.2 - Searchlight A searchlight is shaped like a...Ch. 10.2 - Solar Heat A mirror is shaped like a paraboloid of...Ch. 10.2 - Reflecting Telescope A reflecting telescope...Ch. 10.2 - Parabolic Arch Bridge A bridge is built in the...Ch. 10.2 - Parabolic Arch Bridge A bridge is to be built in...Ch. 10.2 - Gateway Arch The Gateway Arch in St. Louis is...Ch. 10.2 - Show that an equation of the form A x 2 +Ey=0 A0...Ch. 10.2 - Show that an equation of the form C y 2 +Dx=0 C0...Ch. 10.2 - Show that the graph of an equation of the form A x...Ch. 10.2 - Show that the graph of an equation of the form C y...Ch. 10.2 - Problems 82-85 are based on material learned...Ch. 10.2 - Problems 82-85 are based on material learned...Ch. 10.2 - Problems 82-85 are based on material learned...Ch. 10.2 - Problems 82-85 are based on material learned...Ch. 10.3 - The distance d from P 1 =( 2,5 ) to P 2 =( 4,2 )...Ch. 10.3 - To complete the square of x 2 3x , Add _____. (p....Ch. 10.3 - Find the intercepts of the equation y 2 =164 x 2 ....Ch. 10.3 - The point that is symmetric with respect to the...Ch. 10.3 - The point that is symmetric with respect to the...Ch. 10.3 - Prob. 6AYPCh. 10.3 - A(n) _______ is the collection of all points in a...Ch. 10.3 - For an ellipse, the foci lie on a line called the...Ch. 10.3 - For the ellipse x 2 4 + y 2 25 =1 , the vertices...Ch. 10.3 - For the ellipse x 2 25 + y 2 9 =1 , the value of a...Ch. 10.3 - If the center of an ellipse is ( 2,3 ) , the major...Ch. 10.3 - If the foci of an ellipse are ( 4,4 ) and ( 6,4 )...Ch. 10.3 - In problems 13-16, the graph of an ellipse is...Ch. 10.3 - In problems 13-16, the graph of an ellipse is...Ch. 10.3 - In problems 13-16, the graph of an ellipse is...Ch. 10.3 - In problems 13-16, the graph of an ellipse is...Ch. 10.3 - In problems 17-26, find the vertices and foci of...Ch. 10.3 - In problems 17-26, find the vertices and foci of...Ch. 10.3 - In problems 17-26, find the vertices and foci of...Ch. 10.3 - In problems 17-26, find the vertices and foci of...Ch. 10.3 - In problems 17-26, find the vertices and foci of...Ch. 10.3 - In problems 17-26, find the vertices and foci of...Ch. 10.3 - In problems 17-26, find the vertices and foci of...Ch. 10.3 - In problems 17-26, find the vertices and foci of...Ch. 10.3 - In problems 17-26, find the vertices and foci of...Ch. 10.3 - In problems 17-26, find the vertices and foci of...Ch. 10.3 - In Problems 27-38, find an equation for each...Ch. 10.3 - In Problems 27-38, find an equation for each...Ch. 10.3 - In Problems 27-38, find an equation for each...Ch. 10.3 - In Problems 27-38, find an equation for each...Ch. 10.3 - In Problems 27-38, find an equation for each...Ch. 10.3 - In Problems 27-38, find an equation for each...Ch. 10.3 - In Problems 27-38, find an equation for each...Ch. 10.3 - In Problems 27-38, find an equation for each...Ch. 10.3 - In Problems 27-38, find an equation for each...Ch. 10.3 - In Problems 27-38, find an equation for each...Ch. 10.3 - In Problems 27-38, find an equation for each...Ch. 10.3 - In Problems 27-38, find an equation for each...Ch. 10.3 - In Problems 39-42, write an equation for each...Ch. 10.3 - In Problems 39-42, write an equation for each...Ch. 10.3 - In Problems 39-42, write an equation for each...Ch. 10.3 - In Problems 39-42, write an equation for each...Ch. 10.3 - In Problems 43-54, analyze each equation; that is,...Ch. 10.3 - In Problems 43-54, analyze each equation; that is,...Ch. 10.3 - In Problems 43-54, analyze each equation; that is,...Ch. 10.3 - In Problems 43-54, analyze each equation; that is,...Ch. 10.3 - In Problems 43-54, analyze each equation; that is,...Ch. 10.3 - In Problems 43-54, analyze each equation; that is,...Ch. 10.3 - In Problems 43-54, analyze each equation; that is,...Ch. 10.3 - In Problems 43-54, analyze each equation; that is,...Ch. 10.3 - In Problems 43-54, analyze each equation; that is,...Ch. 10.3 - In Problems 43-54, analyze each equation; that is,...Ch. 10.3 - In Problems 43-54, analyze each equation; that is,...Ch. 10.3 - In Problems 43-54, analyze each equation; that is,...Ch. 10.3 - In Problems 55-64, find an equation for each...Ch. 10.3 - In Problems 55-64, find an equation for each...Ch. 10.3 - In Problems 55-64, find an equation for each...Ch. 10.3 - In Problems 55-64, find an equation for each...Ch. 10.3 - In Problems 55-64, find an equation for each...Ch. 10.3 - In Problems 55-64, find an equation for each...Ch. 10.3 - In Problems 55-64, find an equation for each...Ch. 10.3 - In Problems 55-64, find an equation for each...Ch. 10.3 - In Problems 55-64, find an equation for each...Ch. 10.3 - In Problems 55-64, find an equation for each...Ch. 10.3 - In Problems 65-68, graph each function. Be sure to...Ch. 10.3 - In Problems 65-68, graph each function. Be sure to...Ch. 10.3 - In Problems 65-68, graph each function. Be sure to...Ch. 10.3 - In Problems 65-68, graph each function. Be sure to...Ch. 10.3 - Semielliptical Arch Bridge An arch in the shape of...Ch. 10.3 - Semielliptical Arch Bridge The arch of a bridge is...Ch. 10.3 - Whispering Gallery A hall 100 feet in length is to...Ch. 10.3 - Whispering Gallery Jim, standing at one focus of a...Ch. 10.3 - Semielliptical Arch Bridge A bridge is built in...Ch. 10.3 - Semielliptical Arch Bridge A bridge is to be built...Ch. 10.3 - Racetrack Design Consult the figure. A racetrack...Ch. 10.3 - Semielliptical Arch Bridge An arch for a bridge...Ch. 10.3 - Installing a Vent Pipe A homeowner is putting in a...Ch. 10.3 - Semielliptical Arch Bridge An arch for a bridge...Ch. 10.3 - In Problems 79-83, use the fact that the orbit of...Ch. 10.3 - In Problems 79-83, use the fact that the orbit of...Ch. 10.3 - In Problems 79-83, use the fact that the orbit of...Ch. 10.3 - In Problems 79-83, use the fact that the orbit of...Ch. 10.3 - †In Problems 79-83, use the fact that the orbit...Ch. 10.3 - A rectangle is inscribed in an ellipse with major...Ch. 10.3 - Let D be the line given by the equation x+5=0 ....Ch. 10.3 - Show that an equation of the form A x 2 +C y 2...Ch. 10.3 - Show that the graph of an equation of the form A x...Ch. 10.3 - The eccentricity e of an ellipse is defined as the...Ch. 10.3 - Problems 89-92 are based on material learned...Ch. 10.3 - Problems 89-92 are based on material learned...Ch. 10.3 - Problems 89-92 are based on material learned...Ch. 10.3 - Prob. 92RYKCh. 10.4 - The distance d from P 1 =( 2,5 ) to P 2 =( 4,2 )...Ch. 10.4 - To complete the square of x 2 +5x , add ______....Ch. 10.4 - Find the intercepts of the equation y 2 =9+4 x 2 ....Ch. 10.4 - True or False The equation y 2 =9+ x 2 is...Ch. 10.4 - To graph y= ( x5 ) 3 4 , shift the graph of y= x 3...Ch. 10.4 - Find the vertical asymptotes, if any, and the...Ch. 10.4 - A(n) _______ is the collection of points in a...Ch. 10.4 - For a hyperbola, the foci lie on a line called the...Ch. 10.4 - Answer Problems 9-11 using the figure to the...Ch. 10.4 - Answer Problems 9-11 using the figure to the...Ch. 10.4 - Answer Problems 9-11 using the figure to the...Ch. 10.4 - In a hyperbola, if a=3 and c=5 , then b= ________....Ch. 10.4 - Prob. 13CVCh. 10.4 - Prob. 14CVCh. 10.4 - Prob. 15SBCh. 10.4 - In Problems 15-18, the graph of a hyperbola is...Ch. 10.4 - Prob. 17SBCh. 10.4 - Prob. 18SBCh. 10.4 - In Problems 19-28, find an equation for the...Ch. 10.4 - In Problems 19-28, find an equation for the...Ch. 10.4 - In Problems 19-28, find an equation for the...Ch. 10.4 - In Problems 19-28, find an equation for the...Ch. 10.4 - In Problems 19-28, find an equation for the...Ch. 10.4 - In Problems 19-28, find an equation for the...Ch. 10.4 - In Problems 19-28, find an equation for the...Ch. 10.4 - In Problems 19-28, find an equation for the...Ch. 10.4 - In Problems 19-28, find an equation for the...Ch. 10.4 - In Problems 19-28, find an equation for the...Ch. 10.4 - Prob. 29SBCh. 10.4 - In Problems 29-36, find the center, transverse...Ch. 10.4 - In Problems 29-36, find the center, transverse...Ch. 10.4 - Prob. 32SBCh. 10.4 - Prob. 33SBCh. 10.4 - Prob. 34SBCh. 10.4 - Prob. 35SBCh. 10.4 - Prob. 36SBCh. 10.4 - Prob. 37SBCh. 10.4 - In Problems 37-40, write an equation for each...Ch. 10.4 - Prob. 39SBCh. 10.4 - In Problems 37-40, write an equation for each...Ch. 10.4 - Prob. 41SBCh. 10.4 - Prob. 42SBCh. 10.4 - In Problems 41-48, find an equation for the...Ch. 10.4 - Prob. 44SBCh. 10.4 - Prob. 45SBCh. 10.4 - Prob. 46SBCh. 10.4 - Prob. 47SBCh. 10.4 - Prob. 48SBCh. 10.4 - Prob. 49SBCh. 10.4 - In Problems 49-62, find the center, transverse...Ch. 10.4 - Prob. 51SBCh. 10.4 - Prob. 52SBCh. 10.4 - Prob. 53SBCh. 10.4 - In Problems 49-62, find the center, transverse...Ch. 10.4 - Prob. 55SBCh. 10.4 - Prob. 56SBCh. 10.4 - Prob. 57SBCh. 10.4 - Prob. 58SBCh. 10.4 - Prob. 59SBCh. 10.4 - In Problems 49-62, find the center, transverse...Ch. 10.4 - Prob. 61SBCh. 10.4 - In Problems 49-62, find the center, transverse...Ch. 10.4 - Prob. 63SBCh. 10.4 - Prob. 64SBCh. 10.4 - Prob. 65SBCh. 10.4 - Prob. 66SBCh. 10.4 - Prob. 67MPCh. 10.4 - In Problems 67-74, analyze each equation. ( y+2 )...Ch. 10.4 - Prob. 69MPCh. 10.4 - Prob. 70MPCh. 10.4 - In Problems 67-74, analyze each equation. 25 x 2...Ch. 10.4 - In Problems 67-74, analyze each equation. x 2 +36...Ch. 10.4 - In Problems 67-74, analyze each equation. x 2...Ch. 10.4 - In Problems 67-74, analyze each equation. 9 x 2 y...Ch. 10.4 - Fireworks Display Suppose that two people standing...Ch. 10.4 - Lightning Strikes Suppose that two people standing...Ch. 10.4 - Nuclear Power Plaut Some nuclear power plants...Ch. 10.4 - An Explosion Two recording devices are set 2400...Ch. 10.4 - Rutherford’s Experiment In May 1911, Ernest...Ch. 10.4 - Hyperbolic Mirrors Hyperbolas have interesting...Ch. 10.4 - The eccentricity e of a hyperbola is defined as...Ch. 10.4 - A hyperbola for which a=b is called an equilateral...Ch. 10.4 - Two hyperbolas that have the same set of...Ch. 10.4 - Prove that the hyperbola y 2 a 2 x 2 b 2 =1 has...Ch. 10.4 - Show that the graph of an equation of the form A x...Ch. 10.4 - Show that the graph of an equation of the form A x...Ch. 10.4 - Prob. 87RYKCh. 10.4 - Prob. 88RYKCh. 10.4 - Prob. 89RYKCh. 10.4 - Prob. 90RYKCh. 10.5 - The sum formula for the sine function is sin( A+B...Ch. 10.5 - Prob. 2AYPCh. 10.5 - Prob. 3AYPCh. 10.5 - Prob. 4AYPCh. 10.5 - Prob. 5CVCh. 10.5 - Prob. 6CVCh. 10.5 - Prob. 7CVCh. 10.5 - Prob. 8CVCh. 10.5 - Prob. 9CVCh. 10.5 - Prob. 10CVCh. 10.5 - Prob. 11SBCh. 10.5 - Prob. 12SBCh. 10.5 - Prob. 13SBCh. 10.5 - Prob. 14SBCh. 10.5 - Prob. 15SBCh. 10.5 - Prob. 16SBCh. 10.5 - Prob. 17SBCh. 10.5 - Prob. 18SBCh. 10.5 - Prob. 19SBCh. 10.5 - Prob. 20SBCh. 10.5 - Prob. 21SBCh. 10.5 - Prob. 22SBCh. 10.5 - Prob. 23SBCh. 10.5 - Prob. 24SBCh. 10.5 - Prob. 25SBCh. 10.5 - Prob. 26SBCh. 10.5 - Prob. 27SBCh. 10.5 - Prob. 28SBCh. 10.5 - Prob. 29SBCh. 10.5 - Prob. 30SBCh. 10.5 - In Problems 31-42, rotate the axes so that the new...Ch. 10.5 - In Problems 31-42, rotate the axes so that the new...Ch. 10.5 - In Problems 31-42, rotate the axes so that the new...Ch. 10.5 - In Problems 31-42, rotate the axes so that the new...Ch. 10.5 - Prob. 35SBCh. 10.5 - In Problems 31-42, rotate the axes so that the new...Ch. 10.5 - In Problems 31-42, rotate the axes so that the new...Ch. 10.5 - In Problems 31-42, rotate the axes so that the new...Ch. 10.5 - In Problems 31-42, rotate the axes so that the new...Ch. 10.5 - In Problems 31-42, rotate the axes so that the new...Ch. 10.5 - In Problems 31-42, rotate the axes so that the new...Ch. 10.5 - In Problems 31-42, rotate the axes so that the new...Ch. 10.5 - In Problems 43-52, identify the graph of each...Ch. 10.5 - In Problems 43-52, identify the graph of each...Ch. 10.5 - In Problems 43-52, identify the graph of each...Ch. 10.5 - In Problems 43-52, identify the graph of each...Ch. 10.5 - In Problems 43-52, identify the graph of each...Ch. 10.5 - In Problems 43-52, identify the graph of each...Ch. 10.5 - In Problems 43-52, identify the graph of each...Ch. 10.5 - In Problems 43-52, identify the graph of each...Ch. 10.5 - In Problems 43-52, identify the graph of each...Ch. 10.5 - In Problems 43-52, identify the graph of each...Ch. 10.5 - Prob. 53AECh. 10.5 - Prob. 54AECh. 10.5 - Prob. 55AECh. 10.5 - Prob. 56AECh. 10.5 - Use the rotation formulas ( 5 ) to show that...Ch. 10.5 - Show that the graph of the equation x 1/2 + y 1/2...Ch. 10.5 - Formulate a strategy for analyzing and graphing an...Ch. 10.5 - Explain how your strategy presented in Problem 59...Ch. 10.5 - Problems 61-64 are based on material learned...Ch. 10.5 - Problems 61-64 are based on material learned...Ch. 10.5 - Problems 61-64 are based on material learned...Ch. 10.5 - Problems 61-64 are based on material learned...Ch. 10.6 - Prob. 1AYPCh. 10.6 - Transform the equation r=6cos from polar...Ch. 10.6 - A _______ is the set of points P in a plane such...Ch. 10.6 - The eccentricity e of a parabola is ________, of...Ch. 10.6 - True or False If ( r, ) are polar coordinates, the...Ch. 10.6 - Prob. 6CVCh. 10.6 - Prob. 7SBCh. 10.6 - Prob. 8SBCh. 10.6 - Prob. 9SBCh. 10.6 - Prob. 10SBCh. 10.6 - Prob. 11SBCh. 10.6 - Prob. 12SBCh. 10.6 - In Problems 13-24, analyze each equation and graph...Ch. 10.6 - In Problems 13-24, analyze each equation and graph...Ch. 10.6 - In Problems 13-24, analyze each equation and graph...Ch. 10.6 - In Problems 13-24, analyze each equation and graph...Ch. 10.6 - In Problems 13-24, analyze each equation and graph...Ch. 10.6 - In Problems 13-24, analyze each equation and graph...Ch. 10.6 - In Problems 13-24, analyze each equation and graph...Ch. 10.6 - In Problems 13-24, analyze each equation and graph...Ch. 10.6 - In Problems 13-24, analyze each equation and graph...Ch. 10.6 - In Problems 13-24, analyze each equation and graph...Ch. 10.6 - Prob. 23SBCh. 10.6 - Prob. 24SBCh. 10.6 - In Problems 25-36, convert each polar equation to...Ch. 10.6 - In Problems 25-36, convert each polar equation to...Ch. 10.6 - In Problems 25-36, convert each polar equation to...Ch. 10.6 - In Problems 25-36, convert each polar equation to...Ch. 10.6 - In Problems 25-36, convert each polar equation to...Ch. 10.6 - In Problems 25-36, convert each polar equation to...Ch. 10.6 - In Problems 25-36, convert each polar equation to...Ch. 10.6 - In Problems 25-36, convert each polar equation to...Ch. 10.6 - In Problems 25-36, convert each polar equation to...Ch. 10.6 - In Problems 25-36, convert each polar equation to...Ch. 10.6 - In Problems 25-36, convert each polar equation to...Ch. 10.6 - In Problems 25-36, convert each polar equation to...Ch. 10.6 - Prob. 37SBCh. 10.6 - Prob. 38SBCh. 10.6 - Prob. 39SBCh. 10.6 - Prob. 40SBCh. 10.6 - Prob. 41SBCh. 10.6 - Prob. 42SBCh. 10.6 - Prob. 43AECh. 10.6 - Prob. 44AECh. 10.6 - Derive equation (d) in Table 5: r= ep 1esinCh. 10.6 - Orbit of Mercury The planet Mercury travels around...Ch. 10.6 - Prob. 47RYKCh. 10.6 - Problems 47-50 are based on material learned...Ch. 10.6 - Problems 47-50 are based on material learned...Ch. 10.6 - Problems 47-50 are based on material learned...Ch. 10.7 - The function f( x )=3sin( 4x ) has amplitude...Ch. 10.7 - Let x=f( t ) and y=g( t ) , where f and g are two...Ch. 10.7 - The parametric equations x=2sint , y=3cost define...Ch. 10.7 - Prob. 4CVCh. 10.7 - True or False Parametric equations defining a...Ch. 10.7 - True or False Curves defined using parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - In Problems 7-26, graph the curve whose parametric...Ch. 10.7 - y=4x1Ch. 10.7 - y=8x+3Ch. 10.7 - y= x 2 +1Ch. 10.7 - y= x 3Ch. 10.7 - y= x 3Ch. 10.7 - y= x 4 +1Ch. 10.7 - x= y 3/2Ch. 10.7 - x= yCh. 10.7 - In Problems 35-38, find parametric equations that...Ch. 10.7 - In Problems 35-38, find parametric equations that...Ch. 10.7 - In Problems 35-38, find parametric equations that...Ch. 10.7 - In Problems 35-38, find parametric equations that...Ch. 10.7 - In Problems 39-42, find parametric equations for...Ch. 10.7 - In Problems 39-42, find parametric equations for...Ch. 10.7 - In Problems 39-42, find parametric equations for...Ch. 10.7 - In Problems 39-42, find parametric equations for...Ch. 10.7 - In Problems 43 and 44, the parametric equations of...Ch. 10.7 - In Problems 43 and 44, the parametric equations of...Ch. 10.7 - In Problems 45-48, use a graphing utility to graph...Ch. 10.7 - In Problems 45-48, use a graphing utility to graph...Ch. 10.7 - In Problems 45-48, use a graphing utility to graph...Ch. 10.7 - In Problems 45-48, use a graphing utility to graph...Ch. 10.7 - Projectile Motion Bob throws a ball straight up...Ch. 10.7 - Projectile Motion Alice throws a ball straight up...Ch. 10.7 - Catching a Train Bill’s train leaves at 8:06 AM...Ch. 10.7 - Catching a Bus Jodi’s bus leaves at 5:30 PM and...Ch. 10.7 - Projectile Motion Ichiro throws a baseball with an...Ch. 10.7 - Projectile Motion Mark Texeira hit a baseball with...Ch. 10.7 - Projectile Motion Suppose that Adam hits a golf...Ch. 10.7 - Projectile Motion Suppose that Karla hits a golf...Ch. 10.7 - Uniform Motion AToyota Camry (traveling east at 40...Ch. 10.7 - Uniform Motion A Cessna (heading south at 120 mph)...Ch. 10.7 - The Green Monster The left field wall at Fenway...Ch. 10.7 - Projectile Motion The position of a projectile...Ch. 10.7 - Show that the parametric equations for a line...Ch. 10.7 - Hypocycloid The hypocycloid is a curve defined by...Ch. 10.7 - In Problem 62, we graphed the hypocycloid. Now...Ch. 10.7 - Problems 65-68 are based on material learned...Ch. 10.7 - Problems 65-68 are based on material learned...Ch. 10.7 - Problems 65-68 are based on material learned...Ch. 10.7 - Problems 65-68 are based on material learned...Ch. 10.R - In Problems 1-10, identify each equation. If it is...Ch. 10.R - In Problems 1-10, identify each equation. If it is...Ch. 10.R - In Problems 1-10, identify each equation. If it is...Ch. 10.R - Prob. 4RECh. 10.R - In Problems 1-10, identify each equation. If it is...Ch. 10.R - In Problems 1-10, identify each equation. If it is...Ch. 10.R - In Problems 1-10, identify each equation. If it is...Ch. 10.R - In Problems 1-10, identify each equation. If it is...Ch. 10.R - Prob. 9RECh. 10.R - In Problems 1-10, identify each equation. If it is...Ch. 10.R - In Problems 11-18, find an equation of the conic...Ch. 10.R - In Problems 11-18, find an equation of the conic...Ch. 10.R - In Problems 11-18, find an equation of the conic...Ch. 10.R - In Problems 11-18, find an equation of the conic...Ch. 10.R - In Problems 11-18, find an equation of the conic...Ch. 10.R - In Problems 11-18, find an equation of the conic...Ch. 10.R - In Problems 11-18, find an equation of the conic...Ch. 10.R - In Problems 11-18, find an equation of the conic...Ch. 10.R - In Problems 19-23, identify each conic without...Ch. 10.R - Prob. 20RECh. 10.R - Prob. 21RECh. 10.R - Prob. 22RECh. 10.R - Prob. 23RECh. 10.R - In Problems 24-26, rotate the axes so that the new...Ch. 10.R - In Problems 24-26, rotate the axes so that the new...Ch. 10.R - In Problems 24-26, rotate the axes so that the new...Ch. 10.R - Prob. 27RECh. 10.R - Prob. 28RECh. 10.R - Prob. 29RECh. 10.R - Prob. 30RECh. 10.R - Prob. 31RECh. 10.R - In Problems 32-34, graph the curve whose...Ch. 10.R - Prob. 33RECh. 10.R - In Problems 32-34, graph the curve whose...Ch. 10.R - Prob. 35RECh. 10.R - Prob. 36RECh. 10.R - Prob. 37RECh. 10.R - Prob. 38RECh. 10.R - Prob. 39RECh. 10.R - Prob. 40RECh. 10.R - Prob. 41RECh. 10.R - Prob. 42RECh. 10.R - Prob. 43RECh. 10.R - Prob. 44RE
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- 6. (i) Sketch the trace of the following curve on R², (t) = (sin(t), 3 sin(t)), tЄ [0, π]. [3 Marks] Total marks 10 (ii) Find the length of this curve. [7 Marks]arrow_forwardhelppparrow_forward7. Let F(x1, x2) (F₁(x1, x2), F2(x1, x2)), where = X2 F1(x1, x2) X1 F2(x1, x2) x+x (i) Using the definition, calculate the integral LF.dy, where (t) = (cos(t), sin(t)) and t = [0,2]. [5 Marks] (ii) Explain why Green's Theorem cannot be used to find the integral in part (i). [5 Marks]arrow_forward
- 6. Sketch the trace of the following curve on R², п 3п (t) = (t2 sin(t), t2 cos(t)), tЄ 22 [3 Marks] Find the length of this curve. [7 Marks]arrow_forwardTotal marks 10 Total marks on naner: 80 7. Let DCR2 be a bounded domain with the boundary OD which can be represented as a smooth closed curve : [a, b] R2, oriented in the anticlock- wise direction. Use Green's Theorem to justify that the area of the domain D can be computed by the formula 1 Area(D) = ½ (−y, x) · dy. [5 Marks] (ii) Use the area formula in (i) to find the area of the domain D enclosed by the ellipse y(t) = (10 cos(t), 5 sin(t)), t = [0,2π]. [5 Marks]arrow_forwardTotal marks 15 Total marks on paper: 80 6. Let DCR2 be a bounded domain with the boundary ǝD which can be represented as a smooth closed curve : [a, b] → R², oriented in the anticlockwise direction. (i) Use Green's Theorem to justify that the area of the domain D can be computed by the formula 1 Area(D) = . [5 Marks] (ii) Use the area formula in (i) to find the area of the domain D enclosed by the ellipse (t) = (5 cos(t), 10 sin(t)), t = [0,2π]. [5 Marks] (iii) Explain in your own words why Green's Theorem can not be applied to the vector field У x F(x,y) = ( - x² + y²²x² + y² ). [5 Marks]arrow_forward
- Total marks 15 པ་ (i) Sketch the trace of the following curve on R2, (t) = (t2 cos(t), t² sin(t)), t = [0,2π]. [3 Marks] (ii) Find the length of this curve. (iii) [7 Marks] Give a parametric representation of a curve : [0, that has initial point (1,0), final point (0, 1) and the length √2. → R² [5 Marks] Turn over. MA-201: Page 4 of 5arrow_forwardTotal marks 15 5. (i) Let f R2 R be defined by f(x1, x2) = x² - 4x1x2 + 2x3. Find all local minima of f on R². (ii) [10 Marks] Give an example of a function f: R2 R which is not bounded above and has exactly one critical point, which is a minimum. Justify briefly your answer. [5 Marks] 6. (i) Sketch the trace of the following curve on R2, y(t) = (sin(t), 3 sin(t)), t = [0,π]. [3 Marks]arrow_forwardA ladder 25 feet long is leaning against the wall of a building. Initially, the foot of the ladder is 7 feet from the wall. The foot of the ladder begins to slide at a rate of 2 ft/sec, causing the top of the ladder to slide down the wall. The location of the foot of the ladder, its x coordinate, at time t seconds is given by x(t)=7+2t. wall y(1) 25 ft. ladder x(1) ground (a) Find the formula for the location of the top of the ladder, the y coordinate, as a function of time t. The formula for y(t)= √ 25² - (7+2t)² (b) The domain of t values for y(t) ranges from 0 (c) Calculate the average velocity of the top of the ladder on each of these time intervals (correct to three decimal places): . (Put your cursor in the box, click and a palette will come up to help you enter your symbolic answer.) time interval ave velocity [0,2] -0.766 [6,8] -3.225 time interval ave velocity -1.224 -9.798 [2,4] [8,9] (d) Find a time interval [a,9] so that the average velocity of the top of the ladder on this…arrow_forward
- Total marks 15 3. (i) Let FRN Rm be a mapping and x = RN is a given point. Which of the following statements are true? Construct counterex- amples for any that are false. (a) If F is continuous at x then F is differentiable at x. (b) If F is differentiable at x then F is continuous at x. If F is differentiable at x then F has all 1st order partial (c) derivatives at x. (d) If all 1st order partial derivatives of F exist and are con- tinuous on RN then F is differentiable at x. [5 Marks] (ii) Let mappings F= (F1, F2) R³ → R² and G=(G1, G2) R² → R² : be defined by F₁ (x1, x2, x3) = x1 + x², G1(1, 2) = 31, F2(x1, x2, x3) = x² + x3, G2(1, 2)=sin(1+ y2). By using the chain rule, calculate the Jacobian matrix of the mapping GoF R3 R², i.e., JGoF(x1, x2, x3). What is JGOF(0, 0, 0)? (iii) [7 Marks] Give reasons why the mapping Go F is differentiable at (0, 0, 0) R³ and determine the derivative matrix D(GF)(0, 0, 0). [3 Marks]arrow_forward5. (i) Let f R2 R be defined by f(x1, x2) = x² - 4x1x2 + 2x3. Find all local minima of f on R². (ii) [10 Marks] Give an example of a function f: R2 R which is not bounded above and has exactly one critical point, which is a minimum. Justify briefly Total marks 15 your answer. [5 Marks]arrow_forwardTotal marks 15 4. : Let f R2 R be defined by f(x1, x2) = 2x²- 8x1x2+4x+2. Find all local minima of f on R². [10 Marks] (ii) Give an example of a function f R2 R which is neither bounded below nor bounded above, and has no critical point. Justify briefly your answer. [5 Marks]arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning, Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL  Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL

Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781305652224
Author:Charles P. McKeague, Mark D. Turner
Publisher:Cengage Learning

Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Graph Theory: Euler Paths and Euler Circuits; Author: Mathispower4u;https://www.youtube.com/watch?v=5M-m62qTR-s;License: Standard YouTube License, CC-BY
WALK,TRIAL,CIRCUIT,PATH,CYCLE IN GRAPH THEORY; Author: DIVVELA SRINIVASA RAO;https://www.youtube.com/watch?v=iYVltZtnAik;License: Standard YouTube License, CC-BY