Explanation This should be shown for the figure, given below if a route that crosses each edge is present just once. Explanation will also have to be given. An Eulerian circuit is a circuit that traces each edge of the graph and anEulerian trail passes through each edge once. If a graph has anEulerian circuit, it must also be a graph connected to the degree of the corner. AEulerian mark is present in a connected graph if two vertices are of odd degree and other corners are of equal degree. To be able to traverse all edges in a trail, the graph either consists of anEulean circuit or a Eulerian trail. In this problem we do not cross edges, but have to cross edges. So we label the six regions in this figure as shown below: A pseudograph is constructed where the vertices represent these regions and each edge indicates the crossing of an edge in the figure above when a passage passes between regions...

3rd Edition

ISBN: 9780131679955

Author: Edgar G. Goodaire

Publisher: Prentice Hall

See similar textbooks

Question

Chapter 10.1, Problem 14E

To determine

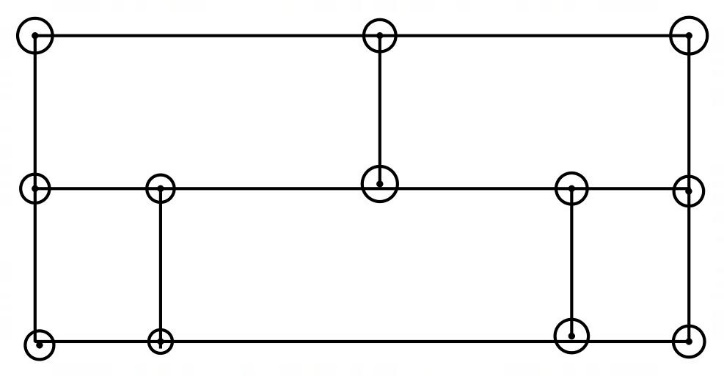
The existence of any sort route in and around the figure that crosses every edge exactly once.

Expert Solution & Answer

Want to see the full answer?

Check out a sample textbook solution

See solution

Chapter 10.1, Problem 13E

Chapter 10.1, Problem 15E

Students have asked these similar questions

Students were asked to simplify the expression (secØ - cosØ)/secØ Two students' work is given.Student A: step 1 secØ/secØ - cosØ/secØstep 2 cosØ/1 - (1/cosØ)step 3 1 - cos^2Østep 4 sin^2ØStudent B: step 1 (1/cosØ)-cosØ)/secØstep 2 (1 - cos^2Ø/cosØ)/secØstep 3 sin^2Ø/cos^2Østep 4 tan^2ØPart A: Which student simplified the expression incorrectly? Explain the errors that were made or the formulas that were misused.Part B: Complete the student's solution correctly, beginning with the location of the error.

Although 330° is a special angle on the unit circle, Amar wanted to determine its coordinates using the sum and difference formulas.Part A: Determine cos 330° using the cosine sum identity. Be sure to include all necessary work.Part B: Determine sin 330° using the sine difference identity. Be sure to include all necessary work.

A public health researcher is studying the impacts of nudge marketing techniques on shoppers vegetables

Chapter 10 Solutions

Discrete Mathematics with Graph Theory

Ch. 10.1 - Prob. 1TFQ Ch. 10.1 - A path is a walk in which all vertices are...Ch. 10.1 - 3. A trail is a path Ch. 10.1 - A path is trail.Ch. 10.1 - A cycle is a special type of circuit.Ch. 10.1 - 6. A cycle is a circuit with no repeated edges Ch. 10.1 - 7. An Eulerian circuit is a cycle. Ch. 10.1 - Prob. 8TFQ Ch. 10.1 - A sub graph of a connected graph must be...Ch. 10.1 - Prob. 10TFQ

Ch. 10.1 - K8,10 is Eulerian.Ch. 10.1 - Prob. 12TFQ Ch. 10.1 - 13. A graph with more than one component cannot be...Ch. 10.1 - Prob. 1E Ch. 10.1 - [BB] Answer the Konigsberg bridge Problem and...Ch. 10.1 - Prob. 3E Ch. 10.1 - Prob. 4E Ch. 10.1 - Prob. 5E Ch. 10.1 - 6. Suppose we modify the definition of Eulerian...Ch. 10.1 - 7. (a) Is there an Eulerian trail from A to B in...Ch. 10.1 - [BB] (Fictitious) A recently discovered map of the...Ch. 10.1 - 9. Euler’s original article about the Konigsberg...Ch. 10.1 - Prob. 10E Ch. 10.1 - Prob. 11E Ch. 10.1 - [BB] For which values of n1 , if any, is Kn...Ch. 10.1 - 13. (a) Find a necessary and sufficient condition...Ch. 10.1 - Prob. 14E Ch. 10.1 - 15.[BB] Prove that any circuit in the graph must...Ch. 10.1 - Prob. 16E Ch. 10.1 - Prob. 17E Ch. 10.1 - Prob. 18E Ch. 10.1 - Prob. 19E Ch. 10.1 - Prob. 20E Ch. 10.1 - Prob. 21E Ch. 10.1 - Prob. 22E Ch. 10.1 - Prob. 23E Ch. 10.1 - Prob. 24E Ch. 10.1 - 25. Prove that a graph is bipartite if and only if...Ch. 10.1 - Prob. 26E Ch. 10.1 - Prob. 27E Ch. 10.2 - A Hamiltonian cycle is a circuit. Ch. 10.2 - Prob. 2TFQ Ch. 10.2 - Prob. 3TFQ Ch. 10.2 - Prob. 4TFQ Ch. 10.2 - Prob. 5TFQ Ch. 10.2 - A graph that contains a proper cycle cannot be...Ch. 10.2 - Prob. 7TFQ Ch. 10.2 - Prob. 8TFQ Ch. 10.2 - Prob. 9TFQ Ch. 10.2 - Prob. 10TFQ Ch. 10.2 - Prob. 1E Ch. 10.2 - 2. Determine whether or not each of the graphs of...Ch. 10.2 - Determine whether each of the graph shown is...Ch. 10.2 - Prob. 4E Ch. 10.2 - Consider the graph shown. Is it Hamiltonian? Is...Ch. 10.2 - Prob. 6E Ch. 10.2 - Prob. 7E Ch. 10.2 - Does the graph have a Hamiltonian cycle that...Ch. 10.2 - Prob. 9E Ch. 10.2 - Prob. 10E Ch. 10.2 - How many edges must a Hamiltonian cycle is kn...Ch. 10.2 - 12. Draw a picture of a cube, by imagining that...Ch. 10.2 - Prob. 13E Ch. 10.2 - Prob. 14E Ch. 10.2 - Prob. 15E Ch. 10.2 - Prob. 16E Ch. 10.2 - Suppose G is a graph with n3 vertices and at least...Ch. 10.2 - 18.[BB] Suppose G is a graph with vertices such...Ch. 10.2 - Prob. 19E Ch. 10.2 - Prob. 20E Ch. 10.2 - Answer true of false and in each case either given...Ch. 10.2 - Prob. 22E Ch. 10.2 - Prob. 23E Ch. 10.2 - Find a necessary and sufficient condition on m and...Ch. 10.3 - Prob. 1TFQ Ch. 10.3 - Prob. 2TFQ Ch. 10.3 - Prob. 3TFQ Ch. 10.3 - Prob. 4TFQ Ch. 10.3 - Prob. 5TFQ Ch. 10.3 - Prob. 6TFQ Ch. 10.3 - Prob. 7TFQ Ch. 10.3 - Prob. 8TFQ Ch. 10.3 - Prob. 9TFQ Ch. 10.3 - Prob. 10TFQ Ch. 10.3 - Prob. 1E Ch. 10.3 - Prob. 2E Ch. 10.3 - Prob. 3E Ch. 10.3 - Prob. 4E Ch. 10.3 - Prob. 5E Ch. 10.3 - Prob. 6E Ch. 10.3 - Prob. 7E Ch. 10.3 - 8. (a) [BB] Find the adjacency matrices and of...Ch. 10.3 - 9. Repeat Exercise 8 for the graphs and shown....Ch. 10.3 - Prob. 10E Ch. 10.3 - Let A=[abcpqrxyz] and let P=[010001100]. Thus P is...Ch. 10.3 - Prob. 12E Ch. 10.3 - 13. For each pair of matrices shown, decide...Ch. 10.3 - 14. [BB] Let A be the adjacency matrix of a...Ch. 10.3 - Prob. 15E Ch. 10.3 - Prob. 16E Ch. 10.3 - Prob. 17E Ch. 10.3 - Prob. 18E Ch. 10.4 - Prob. 1TFQ Ch. 10.4 - Prob. 2TFQ Ch. 10.4 - It is an open question as to whether there exists...Ch. 10.4 - Prob. 4TFQ Ch. 10.4 - Prob. 5TFQ Ch. 10.4 - Prob. 6TFQ Ch. 10.4 - Prob. 7TFQ Ch. 10.4 - Prob. 8TFQ Ch. 10.4 - Prob. 9TFQ Ch. 10.4 - Prob. 10TFQ Ch. 10.4 - Prob. 1E Ch. 10.4 - Prob. 2E Ch. 10.4 - Prob. 3E Ch. 10.4 - Prob. 4E Ch. 10.4 - Prob. 5E Ch. 10.4 - Prob. 6E Ch. 10.4 - Prob. 7E Ch. 10.4 - Prob. 8E Ch. 10.4 - Prob. 9E Ch. 10.4 - Prob. 10E Ch. 10.4 - Prob. 11E Ch. 10.4 - 12. [BB] Could Dijkstra’s algorithm (original...Ch. 10.4 - Prob. 13E Ch. 10.4 - 14. (a) If weights were assigned to the edges of...Ch. 10.4 - Prob. 15E Ch. 10.4 - Prob. 16E Ch. 10.4 - Prob. 17E Ch. 10.4 - Prob. 18E Ch. 10.4 - Prob. 19E Ch. 10.4 - Prob. 20E Ch. 10.4 - Prob. 21E Ch. 10.4 - Prob. 22E Ch. 10.4 - Prob. 23E Ch. 10.4 - Prob. 24E Ch. 10 - In the Konigsberg Bringe Problem (see fig. 9.1),...Ch. 10 - Prob. 2RE Ch. 10 - Suppose G1 and G2 are graphs with no vertices in...Ch. 10 - Prob. 4RE Ch. 10 - Prob. 5RE Ch. 10 - Is the graph Hamiltonian? Is it Eulerian? Explain...Ch. 10 - Determine, with reason, whether each of the...Ch. 10 - Prob. 8RE Ch. 10 - Prob. 9RE Ch. 10 - Prob. 10RE Ch. 10 - Prob. 11RE Ch. 10 - Prob. 12RE Ch. 10 - Prob. 13RE Ch. 10 - Prob. 14RE Ch. 10 - 15. A connected graph G has 10 vertices and 41...Ch. 10 - Prob. 16RE Ch. 10 - Let v1,v2,........v8 and w1,w2,..........w12 be...Ch. 10 - Prob. 18RE Ch. 10 - Martha claims that a graph with adjacency...Ch. 10 - Prob. 20RE Ch. 10 - Which of the following three matrices (if any) is...Ch. 10 - Apply the first form of Dijkstras algorithm to the...Ch. 10 - Prob. 23RE Ch. 10 - 24. Apply the original form of Dijkstra’s...Ch. 10 - Apply the improved version of Dijkstras algorithm...Ch. 10 - Prob. 26RE Ch. 10 - 27. Apply the Floyd- Warshall algorithm apply to...Ch. 10 - Prob. 28RE

Knowledge Booster

The existence of any sort route in and around the figure that crosses every edge exactly once.

Solution Summary: The author explains that a pseudograph has 4 odd vertices and 2 even ones, so no route exists for the figure.

The existence of any sort route in and around the figure that crosses every edge exactly once.

Want to see the full answer?

Chapter 10 Solutions