
Concept explainers
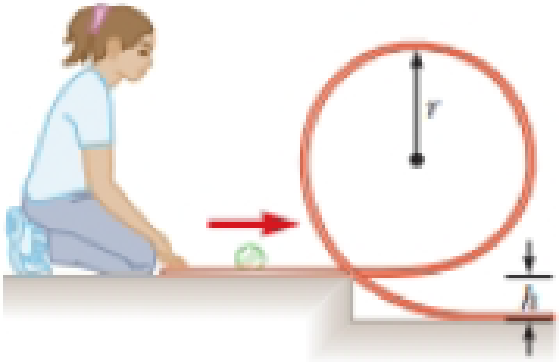
A tennis ball is a hollow sphere with a thin wall. It is set rolling without slipping at 4.03 m/s on a horizontal section of a track as shown in Figure P10.33. It rolls around the inside of a vertical circular loop of radius r = 45.0 cm. As the ball nears the bottom of the loop, the shape of the track deviates from a perfect circle so that the ball leaves the track at a point h = 20.0 cm below the horizontal section. (a) Find the ball’s speed at the top of the loop. (b) Demonstrate that the ball will not fall from the track at the top of the loop. (c) Find the ball’s speed as it leaves the track at the bottom. (d) What If? Suppose that static friction between ball and track were negligible so that the ball slid instead of rolling. Describe the speed of the ball at the top of the loop in this situation. (e) Explain your answer to part (d).
Figure P10.33
(a)
The speed of the ball at the top of the loop.
Answer to Problem 33P
The speed of the ball at the top of the loop is
Explanation of Solution
Given information: The radius of the circular loop is
Write the expression for the law of energy conservation between horizontal track and the top of the loop is,
Here,
Write the expression for the translational kinetic energy of the ball at horizontal track is,
Here,
Write the expression for the rotational kinetic energy of the ball at horizontal track is,
Here,
Write the expression for the moment of inertia of the ball is,
Write the expression for the initial angular speed of the ball is,
Substitute
Write the expression for the translational kinetic energy of the ball at top point of the loop is,
Here,
Write the expression for the rotational kinetic energy of the ball at top point of the loop is,
Here,
Write the expression for the moment of inertia of the ball is,
Write the expression for the initial angular speed of the ball is,
Substitute
Write the expression for the potential energy of the ball at top point of the loop is,
Here,
Substitute
Simplify the above equation for
Substitute
Thus, the speed of the ball at the top of the loop is
Conclusion:
Therefore, the speed of the ball at the top of the loop is
(b)
The reason that the ball will not fall from the track at the top of the loop.
Answer to Problem 33P
The ball will not fall because the value of the centripetal acceleration is more than the acceleration due to gravity at the top point of the circular loop.
Explanation of Solution
Given information: The radius of the circular loop is
Formula to calculate the centripetal acceleration on the ball at the top of the loop is,
Here,
Substitute
Thus, the centripetal acceleration act on the ball at the top of the loop is
Since the centripetal acceleration at the top of the loop is more than the acceleration due to gravity that is
Conclusion:
Therefore, the ball will not fall because the value of the centripetal acceleration is more than the acceleration due to gravity at the top point of the circular loop.
(c)
The speed of the ball as it leaves the track at the bottom.
Answer to Problem 33P
The speed of the ball as it leaves the track at the bottom is
Explanation of Solution
Given information: The radius of the circular loop is
Write the expression for the law of energy conservation between horizontal track and the bottom of the loop is,
Here,
Write the expression for the translational kinetic energy of the ball at bottom point of the loop is,
Here,
Write the expression for the rotational kinetic energy of the ball at bottom point of the loop is,
Here,
Write the expression for the initial angular speed of the ball is,
Substitute
Write the expression for the potential energy of the ball at top point of the loop is,
Here,
Substitute
Simplify the above equation for
Substitute
Thus, the speed of the ball as it leaves the track at the bottom is
Conclusion:
Therefore, the speed of the ball as it leaves the track at the bottom is
(d)
The speed of the ball at the top of the loop if ball slide instead of roll.
Answer to Problem 33P
The speed of the ball at the top of the loop is imaginary.
Explanation of Solution
Given information: The radius of the circular loop is
Write the expression for the law of energy conservation between horizontal track and the top of the loop is,
Here,
Write the expression for the rotational kinetic energy of the ball at horizontal track is,
Write the expression for the new translational kinetic energy of the ball at top point of the loop is,
Here,
Write the expression for the new potential energy of the ball at top point of the loop is,
Substitute
Simplify the above equation for
Substitute
Since the value inside the square root is negative that means the value is imaginary. This condition is impractical.
Thus, the speed of the ball at the top of the loop is imaginary.
Conclusion:
Therefore, the speed of the ball at the top of the loop is imaginary. It can’t be calculated.
(e)
The explanation of the solution of part (d).
Answer to Problem 33P
The ball has not sufficient energy to arrive at top of the circular loop.
Explanation of Solution
Given information: The radius of the circular loop is
The velocity comes out to be imaginary in part (d) that indicates the situation in impossible because as the boll slide instead of rolling, the ball have only translational kinetic energy which is insufficient for the ball to reach the top point on the circular loop.
Thus, the ball did not arrive at the top point of the loop.
Conclusion:
Therefore, the ball has not sufficient energy to arrive at top of the circular loop.
Want to see more full solutions like this?
Chapter 10 Solutions
Physics for Scientists and Engineers with Modern Physics
- below the horizontal, and land 55 m horizontally from the end of the jump. Your job is to specify the slope of the ground so skiers' trajectories make an angle of only 3.0° with the ground on land- ing, ensuring their safety. What slope do you specify? T 9.5° -55 marrow_forwardMake sure to draw a sketch and a free body diagram. DO NOT give me examples but ONLY the solutionarrow_forwardMake sure to draw a sketch AND draw a Free body diagramarrow_forward
- P -3 ft 3 ft. O A B 1.5 ft Do 1.5 ft ✓ For the frame and loading shown, determine the magnitude of the reaction at C (in lb) if P = 55 lb. (Hint: Use the special cases: Two-force body and Three-force body.)arrow_forwardA convex mirror (f.=-6.20cm) and a concave minor (f2=8.10 cm) distance of 15.5cm are facing each other and are separated by a An object is placed between the mirrors and is 7.8cm from each mirror. Consider the light from the object that reflects first from the convex mirror and then from the concave mirror. What is the distance of the image (dia) produced by the concave mirror? cm.arrow_forwardAn amusement park spherical mirror shows park spherical mirror shows anyone who stands 2.80m in front of it an upright image one and a half times the person's height. What is the focal length of the minor? m.arrow_forward
- An m = 69.0-kg person running at an initial speed of v = 4.50 m/s jumps onto an M = 138-kg cart initially at rest (figure below). The person slides on the cart's top surface and finally comes to rest relative to the cart. The coefficient of kinetic friction between the person and the cart is 0.440. Friction between the cart and ground can be ignored. (Let the positive direction be to the right.) m M (a) Find the final velocity of the person and cart relative to the ground. (Indicate the direction with the sign of your answer.) m/s (b) Find the friction force acting on the person while he is sliding across the top surface of the cart. (Indicate the direction with the sign of your answer.) N (c) How long does the friction force act on the person? S (d) Find the change in momentum of the person. (Indicate the direction with the sign of your answer.) N.S Find the change in momentum of the cart. (Indicate the direction with the sign of your answer.) N.S (e) Determine the displacement of the…arrow_forwardSmall ice cubes, each of mass 5.60 g, slide down a frictionless track in a steady stream, as shown in the figure below. Starting from rest, each cube moves down through a net vertical distance of h = 1.50 m and leaves the bottom end of the track at an angle of 40.0° above the horizontal. At the highest point of its subsequent trajectory, the cube strikes a vertical wall and rebounds with half the speed it had upon impact. If 10 cubes strike the wall per second, what average force is exerted upon the wall? N ---direction--- ▾ ---direction--- to the top to the bottom to the left to the right 1.50 m 40.0°arrow_forwardThe magnitude of the net force exerted in the x direction on a 3.00-kg particle varies in time as shown in the figure below. F(N) 4 3 A 2 t(s) 1 2 3 45 (a) Find the impulse of the force over the 5.00-s time interval. == N⚫s (b) Find the final velocity the particle attains if it is originally at rest. m/s (c) Find its final velocity if its original velocity is -3.50 î m/s. V₁ m/s (d) Find the average force exerted on the particle for the time interval between 0 and 5.00 s. = avg Narrow_forward
- ••63 SSM www In the circuit of Fig. 27-65, 8 = 1.2 kV, C = 6.5 µF, R₁ S R₂ R3 800 C H R₁ = R₂ = R3 = 0.73 MQ. With C completely uncharged, switch S is suddenly closed (at t = 0). At t = 0, what are (a) current i̟ in resistor 1, (b) current 2 in resistor 2, and (c) current i3 in resistor 3? At t = ∞o (that is, after many time constants), what are (d) i₁, (e) i₂, and (f) iz? What is the potential difference V2 across resistor 2 at (g) t = 0 and (h) t = ∞o? (i) Sketch V2 versus t between these two extreme times. Figure 27-65 Problem 63.arrow_forwardThor flies by spinning his hammer really fast from a leather strap at the end of the handle, letting go, then grabbing it and having it pull him. If Thor wants to reach escape velocity (velocity needed to leave Earth’s atmosphere), he will need the linear velocity of the center of mass of the hammer to be 11,200 m/s. Thor's escape velocity is 33532.9 rad/s, the angular velocity is 8055.5 rad/s^2. While the hammer is spinning at its maximum speed what impossibly large tension does the leather strap, which the hammer is spinning by, exert when the hammer is at its lowest point? the hammer has a total mass of 20.0kg.arrow_forwardIf the room’s radius is 16.2 m, at what minimum linear speed does Quicksilver need to run to stay on the walls without sliding down? Assume the coefficient of friction between Quicksilver and the wall is 0.236.arrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





