EBK NUMERICAL METHODS FOR ENGINEERS
7th Edition
ISBN: 8220100254147
Author: Chapra
Publisher: MCG
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 1, Problem 26P
Beyond fluids, Archimedes' principle has proven useful in geology when applied to solids on the earth's crust. Figure P1.26 depicts one such case where a lighter conical granite mountain “floats on” a denser basalt layer at the earth's surface. Note that the part of the cone below the surface is formally referred to as a frustum. Develop a steady-state force balance for this case in terms of the following parameters: basalt's density
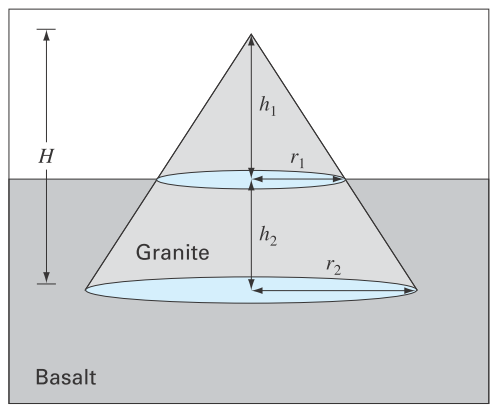
FIGURE P1.26
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
A force of 9 lb is required to keep a spring compressed to
ft shorter than its equilibrium length. What is its spring constant?
k =
lb/ft
Use the concept of Particular Antiderivatives and Rectilinear Motion to answer the problem below:
On the edge of an 8m building, Sam throws his toy vertically upwards at initial velocity = 28 m/s. If the only force that acts on the toy is accelerationdue to gravity (-32 ft/s),
a. How many seconds will it take for the toy to reach its maximum height?b. What is the toy's speed when it hits the ground?
After a mass weighing 8 pounds is attached to a 5-foot spring, the springmeasures 6.6 feet. The entire system is placed in a medium that offersa damping constant of one.Find the equation of motion if the mass is initially released from a point 6inches above the equilibrium position with a downward velocity of1 ft/sec
Chapter 1 Solutions
EBK NUMERICAL METHODS FOR ENGINEERS
Ch. 1 - Use calculus to solve Eq. (1.9) for the case where...Ch. 1 - 1.2 Repeat Example 1.2. Compute the velocity to ...Ch. 1 - Rather than the linear relationship of Eq. (1.7),...Ch. 1 - For the free-falling parachutist with linear drag,...Ch. 1 - Compute the velocity of a free-falling parachutist...Ch. 1 - 1.6 The following information is available for a...Ch. 1 - The amount of a uniformly distributed radioactive...Ch. 1 - A group of 35 students attend a class in a room...Ch. 1 - A storage tank contains a liquid at depth y, where...Ch. 1 - For the same storage tank described in Prob. 1.9,...
Ch. 1 - 1.11 Apply the conservation of volume (see Prob....Ch. 1 - In our example of the free-falling parachutist,...Ch. 1 - 1.13 Suppose that a spherical droplet of liquid...Ch. 1 - Newtons law of cooling says that the temperature...Ch. 1 - As depicted in Fig. P1.15, an RLC circuit consists...Ch. 1 - 1.16 Cancer cells grow exponentially with a...Ch. 1 - 1.17 A fluid is pumped into the network shown in...Ch. 1 - The velocity is equal to the rate of change of...Ch. 1 - You are working as a crime-scene investigator and...Ch. 1 - 1.21 As noted in Prob. 1.3, drag is more...Ch. 1 - 1.22 As depicted in Fig. P1.22, a spherical...Ch. 1 - As described in Prob. 1.22, in addition to the...Ch. 1 - As depicted in Fig. P1.24, the downward deflection...Ch. 1 - 1.25 Use Archimedes’ principle to develop a...Ch. 1 - Beyond fluids, Archimedes principle has proven...
Additional Engineering Textbook Solutions
Find more solutions based on key concepts
(a) Make a stem-and-leaf plot for these 24 observations on the number of customers who used a down-town CitiBan...
APPLIED STAT.IN BUS.+ECONOMICS
The largest polynomial that divides evenly into a list of polynomials is called the _______.
Elementary & Intermediate Algebra
Find all solutions of each equation in the interval .
Precalculus: A Unit Circle Approach (3rd Edition)
First Derivative Test a. Locale the critical points of f. b. Use the First Derivative Test to locale the local ...
Calculus: Early Transcendentals (2nd Edition)
In Exercises 5-36, express all probabilities as fractions.
23. Combination Lock The typical combination lock us...
Elementary Statistics
For Problems 23-28, write in simpler form, as in Example 4. logbFG
Finite Mathematics for Business, Economics, Life Sciences and Social Sciences
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- A spring is stretched 8 feet into equilibrium by an object weighing 64 pounds, with a damping force of 8 pounds per ft/sec and an ex ternal force of 24 pounds. If the object is pushed down with a speed of 4ft/sec from a position 1 ft above the equilibrium position, find its displacement.arrow_forwardRather than the linear relationship of Eq. (1.7), you might choose to model the upward force on the parachutist as a second-order relationship. FU = −c'v2, where c' = a second-order drag coefficient (kg/m). a. Using calculus, obtain the closed-form solution for the casewhere the jumper is initially at rest (v = 0 at t = 0).b. Repeat the numerical calculation in Example 1.2 with thesame initial condition and parameter values. Use a value of0.225 kg/m for c' .arrow_forwardA seasoned parachutist went for a skydiving trip where he performed freefall before deploying the parachute. According to Newton's Second Law of Motion, there are two forcës acting on the body of the parachutist, the forces of gravity (F,) and drag force due to air resistance (Fa) as shown in Figure 1. Fa = -cv ITM EUTM FUTM * UTM TM Fg= -mg x(t) UTM UT UTM /IM LTM UTM UTM TUIM UTM F UT GROUND Figure 1: Force acting on body of free-fall where x(t) is the position of the parachutist from the ground at given time, t is the time of fall calculated from the start of jump, m is the parachutist's mass, g is the gravitational acceleration, v is the velocity of the fall and c is the drag coefficient. The equation for the velocity and the position is given by the equations below: EUTM PUT v(t) = mg -et/m – 1) (Eq. 1.1) x(t) = x(0) – Where x(0) = 3200 m, m = 79.8 kg, g = 9.81m/s² and c = 6.6 kg/s. It was established that the critical position to deploy the parachutes is at 762 m from the ground…arrow_forward
- A spring has a natural length of 10 feet, and when an 64lb weight is attached, the spring measures 13.2 feet. The weight is initially displaced 4 feet above equilibrium and given a downward velocity of 3 ft/sec. Find its displacement for t>0 if the medium resists the motion with a force of two Ibs for each ft/sec of velocity.arrow_forwardA mass weighing 4 pounds is attached to a spring whose constant is 2 Ib/ft. The medium offers a damping force that is numerically equal to the instantaneous velocity. The mass is initially released from a point 1 foot above the equilibrium position with a downward velocity of 16 ft/s. Determine the time (in s) at which the mass passes through the equilibrium position. (Use g = 32 ft/s? for the acceleration due to gravity.) Find the time (in s) after the mass passes through the equilibrium position at which the mass attains its extreme displacement from the equilibrium position. What is the position (in ft) of the mass at this instant? ftarrow_forwardAfter a mass weighing 10 pounds is attached to a 5-foot spring,the spring measures 7 feet. This mass is removed and replacedwith another mass that weighs 8 pounds. The entire system isplaced in a medium that offers a damping force numericallyequal to the instantaneous velocity.(a) Find the equation of motion if the mass is initially releasedfrom a point 12 foot below the equilibrium position witha downward velocity of 1 ft/s.(b) Express the equation of motion in the form givenin (23).(c) Find the times at which the mass passes through the equilibrium position heading downward.(d) Graph the equation of motion.arrow_forward
- A mass weighing 4 pounds is attached to a spring whose constant is 2 Ib/ft. The medium offers a damping force that is numerically equal to the instantaneous velocity. The mass is initially released from a point 1 foot above the equilibrium position with a downward velocity of 16 ft/s. Determine the time (in s) at which the mass passes through the equilibrium position. (Useg = 32 ft/s? for the acceleration due to gravity.) Find the time (in s) after the mass passes through the equilibrium position at which the mass attains its extreme displacement from the equilibrium position. What is the position (in ft) of the mass at this instant? ftarrow_forwardA circular swimming pool has a diameter of 20 m. The circular side of the pool is 4 m high, and the depth of the water is 3.5 m. (The acceleration due to gravity is 9.8 m/s² and the density of water is 1000 kg/m³.) How much work (in Joules) is required to: (a) pump all of the water over the side? (1000-9.8-7-10²-3.5) [42-0.5²] 2 (b) pump all of the water out of an outlet 3 m over the side? (1000-9.8--102.3.5) 2 - [7²-0.5²]arrow_forwardThe density of air changes with height. Under some conditions density p, depends on height z, and temperature T according to the following equation where Po and A are both constants. A meteorological balloon ascends (i.e., starts at z = 1 and gains height) over the course of several hours. Complete parts (a) and (b) below. Az P(z,T) = Po e ..... dz v) and that the temperature changes over time (i.e., that T is given by a function T(t)), derive, using the chain rule, an expression for the rate of change of air density, (a) Assuming that the balloon ascends at a speed v (i.e., dt as measured by the weather balloon. Choose the correct answer below. dp Az dT O A. dt T2 dt dp %3D dt Az dT dp С. dt %3D + T dt dp Az) dT O D. dt T2) dt (b) Assume that v = 1, Po = 1, and A = 1 and that when t= 0, T= 1. Are there any conditions under which the density, as measured by the balloon will not change in time? That is, find a differential equation that T must satisfy, if dp = 0, and solve this…arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Trigonometry - Harmonic Motion - Equation Setup; Author: David Hays;https://www.youtube.com/watch?v=BPrZnn3DJ6Q;License: Standard YouTube License, CC-BY
Simple Harmonic Motion - An introduction : ExamSolutions Maths Revision; Author: ExamSolutions;https://www.youtube.com/watch?v=tH2vldyP5OE;License: Standard YouTube License, CC-BY