International Edition---engineering Mechanics: Statics, 4th Edition
4th Edition
ISBN: 9781305501607
Author: Andrew Pytel And Jaan Kiusalaas
Publisher: CENGAGE L
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 1, Problem 1.51P
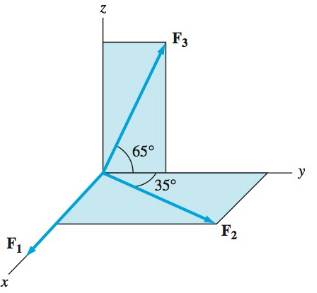
The magnitudes of the three forces are
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
Find the solution of the following Differential Equations
1) 4y+y=0,
y(0)=2,
y'(0) = 0.
2) y+y=0,
y(0) = A,
y'(0) = B.
3) "+2y'-8y=0,
y(0)=1,
y'(0)=8.
4) y"-2y-3y=0,
y(0)=1,
y'(0)=7.
5) y"-ky' =0,
y(0)=2,
y'(0) =k.
6) y+ky'-2k2y=0,
y(0)=2,
y'(0) = 2k.
7) y'+4y=0,
y(0)=2.8
y+y-17sin(21)
y(0)=-1.
9) y-y'-6y=0,
y(0)=6.
y'(0)=13.
10) y-y=0,
11) y"-4y+4y=0,
y(0)=4,
y'(0) = 0.
y(0) = 2.1,
y'(0)=3.9
12) y+2y+2y=0,
y(0)=1,
y'(0)=-3.
13)
"+7y+12y=21e",
y(0)=3.5,
y'(0)=-10.
14) "+9y=10e",
y(0)=0.
y'(0) = 0.
15) y+3y+2.25y=91³ +64.
y(0)=1,
y'(0) = 31.5
16) "-6y+5y= 29 cos(21),
y(0)=3.2,
y'(0) = 6.2
17) y+2y+2y=0,
y(0)=0,
y'(0)=1.
18) y+2y+17y=0,
y(0)=0,
y'(0)=12.
19) y-4y+5y=0,
y(0)-1,
y'(0) 2.
20) 9y-6y+y=0.
y(0)=3,
y'(0)=1.
21) -2y+10y=0,
y(0)=3,
y'(0)=3.
22) 4y-4y+37y=0,
(0) 3.
y(0) 1.5
23) 4y-8y+5y=0,
(0)-0,
y(0) 1.
24) y+y+1.25y=0,
y(0) 1.
y'(0) -0.5
25) y+y=2 cos(1).
y(0) 2.
y'(0) = 0.
26) -4y+3y=0,
(0)-3,
y'(0) = 7.
27) y+2y+y=e",
y(0)-0.
y'(0) = 0.
29)
28) y+2y-3y-10sinh(2),…
Note:
Please provide a clear, step-by-step simplified handwritten working out (no explanations!), ensuring it is done without any AI involvement. I require an expert-level answer, and I will assess and rate based on the quality and accuracy of your work and refer to the provided image for more clarity. Make sure to double-check everything for correctness before submitting appreciate your time and effort!.
Question:
4. Block A and B are two different pieces of wood. Determine the minimum dimension for
"a", if the shear stress of the wood is 50Mpa. The thickness of the wood is 30cm.
600N
A
Chapter 1 Solutions
International Edition---engineering Mechanics: Statics, 4th Edition
Ch. 1 - A person weighs 30 lb on the moon, where...Ch. 1 - The radius and length of a steel cylinder are 40...Ch. 1 - Convert the following: (a) 400lbft to knm; (b)...Ch. 1 - A compact car travels 30 mi on one gallon of gas....Ch. 1 - The kinetic energy of a car of mass m moving with...Ch. 1 - In a certain application, the coordinate a and the...Ch. 1 - When a force F acts on a linear spring, the...Ch. 1 - In some applications dealing with very high...Ch. 1 - A geometry textbook gives the equation of a...Ch. 1 - A differential equation is d2ydt2=Ay2+Byt where y...
Ch. 1 - The position coordinate x of a particle is...Ch. 1 - A differential equation encountered in the...Ch. 1 - Determine the dimensions of constants A and B far...Ch. 1 - The typical power output of a compact car engine...Ch. 1 - Two 12-kg spheres are placed 400 mm apart. Express...Ch. 1 - Two identical spheres of radius 8 in. and weighing...Ch. 1 - A man weighs 170 lb on the surface of the earth....Ch. 1 - Use Eq. (1.4) to show that the weight of an object...Ch. 1 - Plot the earths gravitational acceleration g(m/s2)...Ch. 1 - Find the elevation h (km) where the weight of an...Ch. 1 - Calculate the gravitational force between the...Ch. 1 - The magnitudes of the two velocity vectors are...Ch. 1 - Determine the magnitudes of vectors v1 and v2 so...Ch. 1 - The pole AB is held up by the rope attached to B....Ch. 1 - Resolve the 20-kN force into components along the...Ch. 1 - The velocity vector of the boat has two...Ch. 1 - Two members of a truss apply the forces shown to...Ch. 1 - Two members of a truss apply the forces shown to...Ch. 1 - Determine the resultant of the position vectors A...Ch. 1 - Resolve the position vector A of the car (measured...Ch. 1 - Resolve the 360-lb force into components along the...Ch. 1 - The supporting cables AB and AC are oriented so...Ch. 1 - The two forces shown act on the structural member...Ch. 1 - The resultant of the two forces has a magnitude of...Ch. 1 - The forces acting on the bob of the pendulum are...Ch. 1 - A surveyor sights a target at C from points A and...Ch. 1 - Knowing that the resultant of the two forces is...Ch. 1 - To move the oil drum, the resultant of the three...Ch. 1 - The resultant of the 50-Ib and 30-lb forces is R....Ch. 1 - Obtain the rectangular representation of the force...Ch. 1 - The length of the position vector r is 240 mm....Ch. 1 - Determine the rectangular components of the 560-lb...Ch. 1 - The coordinates of points A and B are (-3, 0, 2)...Ch. 1 - The slider travels along the guide rod AB with the...Ch. 1 - Find the rectangular representation of the force...Ch. 1 - The magnitude of the force F is 160 lb. Find its...Ch. 1 - A rifle at A is fired at a target at B. If the...Ch. 1 - The pole OB is subjected to the 6004b force at B....Ch. 1 - The cables AB and AC are attached to the frame...Ch. 1 - The two forces are applied to the end of the boom...Ch. 1 - The magnitudes of the three forces are...Ch. 1 - Given that P=120lb and Q=130lb, find the...Ch. 1 - Knowing that P=90lb and that the resultant of P...Ch. 1 - If R is the resultant of the forces P and Q, find...Ch. 1 - The force R is the resultant of P and 0. Determine...Ch. 1 - The vertical post is secured by three cables. The...Ch. 1 - Compute the dot product A - B for each of the...Ch. 1 - Compute the cross product C=AB for each of the...Ch. 1 - Given r=4i6j+2km (position vector) F=20i+40j30kN...Ch. 1 - Compute AB and CB for the position vectors shown.Ch. 1 - Use the dot product to find the angle between the...Ch. 1 - Use the dot product to find the angle between the...Ch. 1 - Let A and B be two nonparallel vectors that lie in...Ch. 1 - Determine (a) the angle between the position...Ch. 1 - Find a unit vector that is perpendicular to both...Ch. 1 - The three points A(0,2,2),B(1,4,1), and C(3,0,0)...Ch. 1 - For the position vectors P and Q shown, determine...Ch. 1 - Compute the orthogonal Component of F=6i+20j12klb...Ch. 1 - Compute the value of the scalar a for which the...Ch. 1 - Resolve A=3i+5j4k in. into two vector...Ch. 1 - The force F=5i+12j+4k lb is applied to the handle...Ch. 1 - Determine the value of the scalar a if the...Ch. 1 - Resolve the force F=20i+30j+50klb into two...Ch. 1 - It can be show that a plane area may he...Ch. 1 - The coordinates of the corners of a triangle ABC...Ch. 1 - Show that |abc| equals the volume of a...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- 1. Determine the reaction force at A. 60 kN 5 B 1 m 1 m- -1 m 4 3 m 30 kN marrow_forwardFind the Laplace Transform of the following functions 1) f() cos(ar) Ans. F(s)=7 2ws 2) f() sin(at) Ans. F(s)= s² + a² 3) f(r)-rcosh(at) Ans. F(s)= 2as 4)(t)=sin(at) Ans. F(s)= 2 5) f(1) = 2te' Ans. F(s)= (S-1) 5+2 6) (1) e cos() Ans. F(s) = (+2)+1 7) (1) (Acostẞr)+ Bsin(Br)) Ans. F(s)- A(s+a)+BB (s+a)+B 8) f()-(-)() Ans. F(s)= 9)(1)(1) Ans. F(s): 10) f(r),()sin() Ans. F(s): 11) 2 k 12) 0 13) 0 70 ㄷ.. a 2a 3a 4a 2 3 4 14) f(1)=1, 0<1<2 15) (1) Ksin(t) 0arrow_forward2. Determine the average normal stress developed in rod AB. The mass is 50kg and the diameter of the rod AB is 8mm. B 8 mmarrow_forward2.64 A 2.75-kN tensile load is applied to a test coupon made from 1.6-mm flat steel plate (E = 200 GPa, v = 0.30). Determine the resulting change in (a) the 50-mm gage length, (b) the width of portion AB of the test coupon, (c) the thickness of portion AB, (d) the cross-sectional area of portion AB. 2.75 kN A 12 mm 50 mm B 2.75 kNarrow_forwardProcedure:1- Cartesian system, 2(D)/(3)D,type of support2- Free body diagram3 - Find the support reactions4- If you find a negativenumber then flip the force5- Find the internal force3D\sum Fx=0\sum Fy=0\sum Fz=0\sum Mx=0\sum My=0\Sigma Mz=02D\Sigma Fx=0\Sigma Fy=0\Sigma Mz=05- Use method of sectionand cut the elementwhere you want to findthe internal force andkeep either side of thesectionarrow_forward3. The design of a pump and pipe system has been completed, except for the valves. The system is used to transpor10t water at 120°F through 2 nom sch 40 commercial steel pipe at a required flow rate of 85 gpm. Without the valves, the pump selected has the capability to overcome an additional 18 psi of pressure drop due to the valves and still provide the required flow rate. The pipe/valve joints are threaded. Determine how many 2-inch globe valves can be installed in this pump and pipe system.arrow_forward4. Figure 1 shows a pump and pipe network being used to transport heptane at 120°F to a large, elevated, closed storage tank. The tank is pressurized and maintained at 18 psia. The volumetric flow rate of the heptane is 500 gpm. a. Specify the nominal diameter of the check valve. b. Determine the pump discharge pressure required (psia) to move the heptane through the discharge pipe. Plank = 18 psia Liquid level Large pressurized storage tank 40 ft All pipes are 6-nom sch 40 commercial steel Standard 90° elbows and 180° bend Total length of straight pipe = 115 ft Class 300 swing check valve INH Pump Figure 1: Pressurized storage tank systemarrow_forward2. In a particular section of a fluid system, a 30% ethylene glycol mixture is flowing through a 6- nom xs cast iron pipe at a temperature of 0°C. In this section of piping, the velocity must be maintained in the range 1.5 m/sarrow_forward1. Steam leaves the boiler of a power plant at 5 MPa, 500°C as shown in the following figure. As the steam passes to the turbine, the temperature drops to 496°C before it enters the turbine due to a heat loss through the pipe's insulation. The pressure drop in the pipe connecting the boiler to the turbine is negligible. The steam then passes through an adiabatic turbine and exits at 10 kPa. The turbine has an isentropic efficiency of 85% and is delivering 1000 MW of power. Determine the following. P = 5 MPa T₁ = 500°C Boiler P₁₂ =5 MPa Τ =496°C 7 = 85% W = 1,000 MW P=1 atm To=25°C Turbine 3+ P = 10 kPa a. The heat transfer rate from the pipe connecting the boiler to the turbine (in MW) b. The change in flow exergy rate as the steam flows through the pipe (MW). This represents exergy that is lost to the environment and unavailable for power delivery. Comment on the magnitude of this exergy loss compared to the power delivered by the turbine. What factor(s) would warrant better…arrow_forwardAn aluminum rod of length L = 1m has mass density p = 2700 kg and Young's modulus E = 70 GPa. The rod is fixed at both ends. The exact natural eigenfrequencies of the rod are wexact E = √ ρ for n=1,2,3,. . . . 1. What is the minimum number of linear elements necessary to determine the fundamental frequency w₁ of the system? Discretize the rod in that many elements of equal length, assemble the global system of equations KU = w² MU, and find the fundamental frequency w₁. Compute the relative error e₁ = (w1 - wexact) /w exact Sketch the fundamental mode of vibration. 2. Use COMSOL to solve the same problem. Show the steps necessary to find the fundamental frequency and mode of the rod. What is the relative error using linear elements and a normal mesh?arrow_forwardA ball with a mass of 5.0 kg is hanging from a string and is initially at rest. A bullet with a mass of 10.0 g and a velocity of 200.0 m/s is fired at the ball. The bullet embeds itself inside the ball. How high (h) do the ball and the bullet rise? Gravitational acceleration: g=9.81g = 9.81g=9.81 m/s².arrow_forwardDon't use chatgpt. Need handwritten solution. Mechanical engineeringarrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_iosRecommended textbooks for you
International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
How to balance a see saw using moments example problem; Author: Engineer4Free;https://www.youtube.com/watch?v=d7tX37j-iHU;License: Standard Youtube License