The quantities A and φ (called the amplitude and the phase) are undetermined by the differential equation. They are determined by initial conditions -- specifically, the initial position and the initial velocity -- usually at t = 0, but sometimes at another time. In the oscillating part of the experiment, I measured only the time of 30 periods. I measured no position or velocity. Consequently, A and φ (and also y0) are irrelevant in the problem. We only compare the period T or the frequency ω with the theoretical prediction. You have (hopefully) derived (or maybe looked up) the relation between ω and k and m. This final question relates ω and T. If ω = 8.2*102 rad/s, calculate T in seconds. (Remember, that a radian equals one.) T might be a fraction of a second.
The quantities A and φ (called the amplitude and the phase) are undetermined by the differential equation. They are determined by initial conditions -- specifically, the initial position and the initial velocity -- usually at t = 0, but sometimes at another time.
In the oscillating part of the experiment, I measured only the time of 30 periods. I measured no position or velocity. Consequently, A and φ (and also y0) are irrelevant in the problem. We only compare the period T or the frequency ω with the theoretical prediction. You have (hopefully) derived (or maybe looked up) the relation between ω and k and m.
This final question relates ω and T. If ω = 8.2*102 rad/s, calculate T in seconds. (Remember, that a radian equals one.) T might be a fraction of a second.
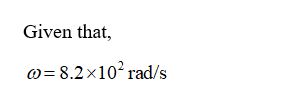
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 2 images
