The average salary for American college graduates is $45,100. You suspect that the average is less for graduates from your college. The 50 randomly selected graduates from your college had an average salary of $42,788 and a standard deviation of $5,940. What can be concluded at the αα = 0.05 level of significance?For this study, we should use Select an answer t-test for a population mean z-test for a population proportion The null and alternative hypotheses would be: H0:H0: ? μ p Select an answer > < = ≠ H1:H1: ? p μ Select an answer ≠ > = < The test statistic ? z t = (please show your answer to 3 decimal places.)The p-value = (Please show your answer to 4 decimal places.)The p-value is ? > ≤ ααBased on this, we should Select an answer fail to reject reject accept the null hypothesis.Thus, the final conclusion is that ...The data suggest that the population mean is not significantly less than 45,100 at αα = 0.05, so there is statistically insignificant evidence to conclude that the population mean salary for graduates from your college is less than 45,100.The data suggest that the sample mean is not significantly less than 45,100 at αα = 0.05, so there is statistically insignificant evidence to conclude that the sample mean salary for graduates from your college is less than 42,788.The data suggest that the populaton mean is significantly less than 45,100 at αα = 0.05, so there is statistically significant evidence to conclude that the population mean salary for graduates from your college is less than 45,100.

Name: The average salary for American college graduates is $45,100. You susp
Uploaded: 2024-03-20T07:07:12.000Z
Channel: Bartleby
Description: The average salary for American college graduates is $45,100. You suspect that the average is less for graduates from your college. The 50 randomly selected graduates from your college had an average salary of $42,788 and a standard deviation of $5,9

Answered: The null and alternative hypotheses…

MATLAB: An Introduction with Applications

6th Edition

ISBN:9781119256830

Author:Amos Gilat

Publisher:Amos Gilat

Chapter1: Starting With Matlab

Section: Chapter Questions

Problem 1P

See similar textbooks

Related questions

Topic Video

Question

The average salary for American college graduates is $45,100. You suspect that the average is less for graduates from your college. The 50 randomly selected graduates from your college had an average salary of $42,788 and a standard deviation of $5,940. What can be concluded at the αα = 0.05 level of significance?

For this study, we should use Select an answer t-test for a population mean z-test for a population proportion
The null and alternative hypotheses would be:

H0:H0: ? μ p Select an answer > < = ≠

H1:H1: ? p μ Select an answer ≠ > = <

The test statistic ? z t = (please show your answer to 3 decimal places.)
The p-value = (Please show your answer to 4 decimal places.)
The p-value is ? > ≤ αα
Based on this, we should Select an answer fail to reject reject accept the null hypothesis.
Thus, the final conclusion is that ...
- The data suggest that the population mean is not significantly less than 45,100 at αα = 0.05, so there is statistically insignificant evidence to conclude that the population mean salary for graduates from your college is less than 45,100.
- The data suggest that the sample mean is not significantly less than 45,100 at αα = 0.05, so there is statistically insignificant evidence to conclude that the sample mean salary for graduates from your college is less than 42,788.
- The data suggest that the populaton mean is significantly less than 45,100 at αα = 0.05, so there is statistically significant evidence to conclude that the population mean salary for graduates from your college is less than 45,100.

Definition Definition Measure of central tendency that is the average of a given data set. The mean value is evaluated as the quotient of the sum of all observations by the sample size. The mean, in contrast to a median, is affected by extreme values. Very large or very small values can distract the mean from the center of the data. Arithmetic mean: The most common type of mean is the arithmetic mean. It is evaluated using the formula: μ = 1 N ∑ i = 1 N x i Other types of means are the geometric mean, logarithmic mean, and harmonic mean. Geometric mean: The nth root of the product of n observations from a data set is defined as the geometric mean of the set: G = x 1 x 2 ... x n n Logarithmic mean: The difference of the natural logarithms of the two numbers, divided by the difference between the numbers is the logarithmic mean of the two numbers. The logarithmic mean is used particularly in heat transfer and mass transfer. ln x 2 − ln x 1 x 2 − x 1 Harmonic mean: The inverse of the arithmetic mean of the inverses of all the numbers in a data set is the harmonic mean of the data. 1 1 x 1 + 1 x 2 + ...

Expert Solution

Step 1

Concept:

The one-sample t-test is a statistical technique that used to define whether a sample of data could have been produced by a procedure with a precise mean. The one-sample t-test is used when the population standard deviation is not known.

As the population standard deviation is known, we will use a one-sample t-test.

For this study, we should use a t-test for a population mean
The null and alternative hypotheses would be:

H0: μ = 45,100

H1: μ < 45,100

The test statistic t can be calculated as:

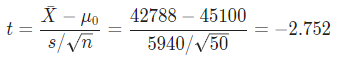
Step by step

Solved in 2 steps with 1 images

SEE SOLUTION Check out a sample Q&A here

Knowledge Booster

$Hypothesis Tests and Confidence Intervals for Means$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.