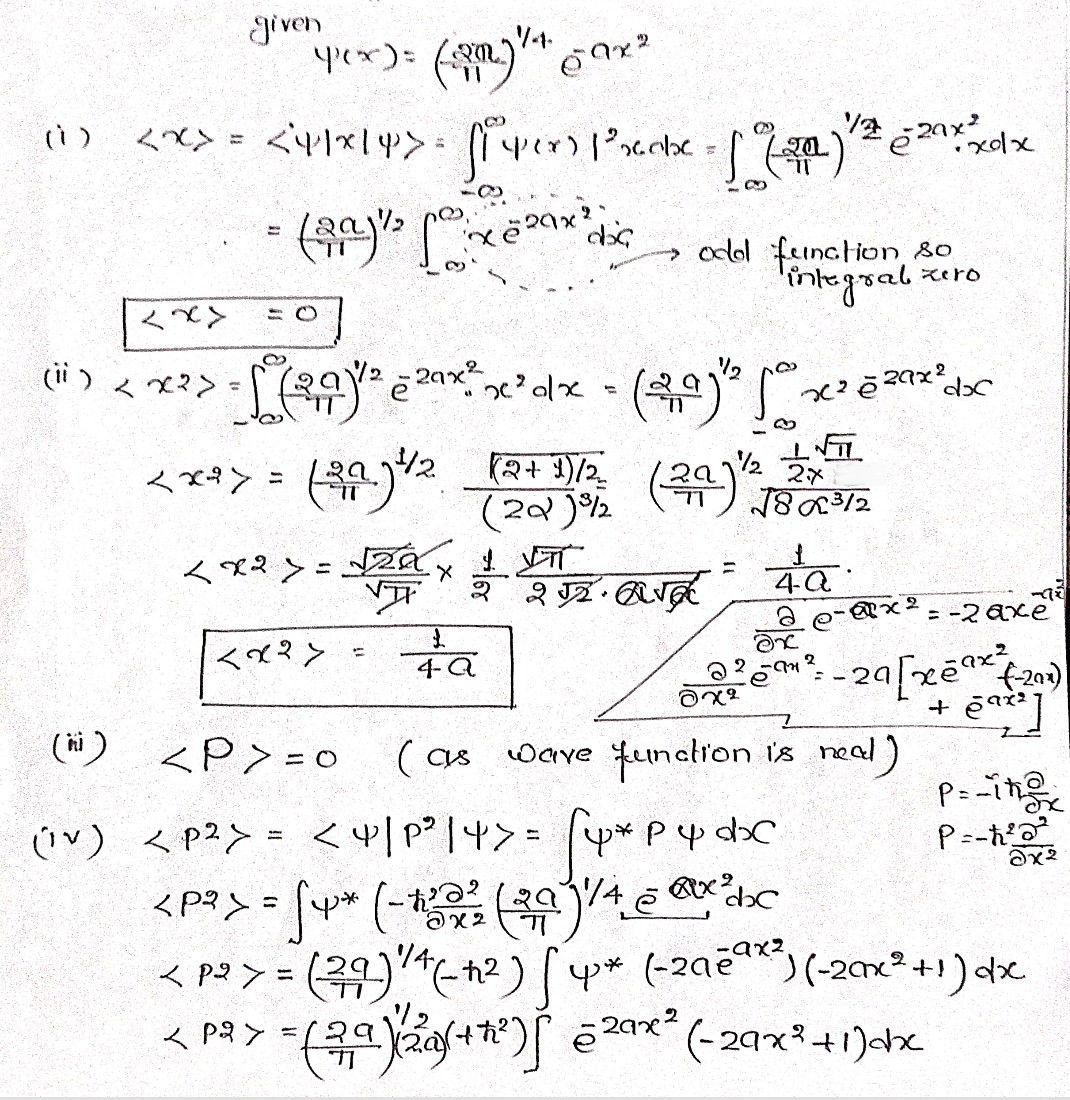
provided that a particle is described by the normalized wavefunction ψ(x)=(2a/π)1/4e-ax^2 with a=constant and -∞ ≤ x ≤ ∞. Calculate the expectation values (⟨x⟩,⟨x2⟩, ⟨px⟩, ⟨p2x⟩, then calculate Δpx= {⟨p2x⟩-⟨px⟩2}1/2 and Δx= {⟨x2⟩-⟨x⟩2}1/2. Finally, verify that the value of ΔpxΔx is consistent with Heisenberg's uncertainty principle. Show full and complete procedure in a clear way. DO NOT SKIP ANY STEP
provided that a particle is described by the normalized wavefunction ψ(x)=(2a/π)1/4e-ax^2 with a=constant and -∞ ≤ x ≤ ∞. Calculate the expectation values (⟨x⟩,⟨x2⟩, ⟨px⟩, ⟨p2x⟩, then calculate Δpx= {⟨p2x⟩-⟨px⟩2}1/2 and Δx= {⟨x2⟩-⟨x⟩2}1/2. Finally, verify that the value of ΔpxΔx is consistent with Heisenberg's uncertainty principle. Show full and complete procedure in a clear way. DO NOT SKIP ANY STEP
Related questions
Question
provided that a particle is described by the normalized wavefunction ψ(x)=(2a/π)1/4e-ax^2 with a=constant and -∞ ≤ x ≤ ∞. Calculate the expectation values (⟨x⟩,⟨x2⟩, ⟨px⟩, ⟨p2x⟩, then calculate Δpx= {⟨p2x⟩-⟨px⟩2}1/2 and Δx= {⟨x2⟩-⟨x⟩2}1/2. Finally, verify that the value of ΔpxΔx is consistent with Heisenberg's uncertainty principle. Show full and complete procedure in a clear way. DO NOT SKIP ANY STEP
Expert Solution
Step 1: Find the average values
Step by step
Solved in 3 steps with 2 images
