Problems 1 - 4 share the same summaries presented in the table below. Two groups of students, graduates and undergraduates, were subject to comparative studies. Their individual grades are assumed to be normal with unknown parameters. Respondents were selected independently and their sample summaries are presented in the table below. Group S2 = s² /n Size Mean Variance Graduate n1 = 10| (X)ı = 85.1 (S²)1 vi = 8 = 4 = 40 %3D %3D Undergraduate n2 = 10 (X)2 = 70.9 (S²)2 = 600 600 10 60 V2 For all hypothesis testing problems, you are supposed to show your conclusions in the standardized form as follows. 1. Test statistic value 2. Critical values required 3. State rejection rule explaining what you decide 4. The decision such as "yes, reject the null" or "not enough evidence for rejection"
Problems 1 - 4 share the same summaries presented in the table below. Two groups of students, graduates and undergraduates, were subject to comparative studies. Their individual grades are assumed to be normal with unknown parameters. Respondents were selected independently and their sample summaries are presented in the table below. Group S2 = s² /n Size Mean Variance Graduate n1 = 10| (X)ı = 85.1 (S²)1 vi = 8 = 4 = 40 %3D %3D Undergraduate n2 = 10 (X)2 = 70.9 (S²)2 = 600 600 10 60 V2 For all hypothesis testing problems, you are supposed to show your conclusions in the standardized form as follows. 1. Test statistic value 2. Critical values required 3. State rejection rule explaining what you decide 4. The decision such as "yes, reject the null" or "not enough evidence for rejection"
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
Related questions
Question

Transcribed Image Text:**Comparative Study of Graduate and Undergraduate Student Grades**
**Overview:**
This study involves comparing the grades of two independently selected groups of students: graduates and undergraduates. The grades are assumed to follow a normal distribution with unknown parameters.
**Data Summary Table:**
| Group | Size | Mean (\(\bar{X}\)) | Variance (\(S^2\)) | \(v = S^2/n\) |
|----------------|------|---------------------|--------------------|---------------|
| Graduate | \(n_1 = 10\) | \((\bar{X})_1 = 85.1\) | \((S^2)_1 = 40\) | \(v_1 = \frac{40}{10} = 4\) |
| Undergraduate | \(n_2 = 10\) | \((\bar{X})_2 = 70.9\) | \((S^2)_2 = 600\) | \(v_2 = \frac{600}{10} = 60\) |
**Hypothesis Testing Framework:**
For all hypothesis testing scenarios related to this study, follow the standardized conclusion format:
1. **Test Statistic Value:** Calculate the value of the test statistic.
2. **Critical Values Required:** Determine the critical values for the chosen level of significance.
3. **State Rejection Rule:** Clearly explain the decision-making criteria, whether to reject or not reject the null hypothesis.
4. **Decision:** Articulate the conclusion, such as "yes, reject the null" or "not enough evidence for rejection."
**Confidence Intervals:**
To derive confidence intervals, provide the critical values required and present the interval using the following format:
- Upper Confidence Limit (UCL) =
- Lower Confidence Limit (LCL) =
This structured approach ensures clarity and consistency in hypothesis testing and confidence interval derivation.

Transcribed Image Text:**Text Transcription for Educational Website:**
---
Continue with the same summaries and assumption as in Problem 3. Faculty assumed that two populations have **ENTIRELY UNKNOWN VARIANCES**, \((\sigma_1)^2\) and \((\sigma_2)^2\). Samples of size \( n_1 = n_2 = n = 10 \) were collected and summarized, with summaries presented in the table shown on page 2.
Estimate the difference, \( \mu = \mu_1 - \mu_2 \) between two population means with confidence \( C = 0.95 \).
1. Show critical value (or values) needed for this procedure.
2. Evaluate upper and lower confidence limits for the targeted parameter, \( \mu_1 - \mu_2 \).
---
*Note: Since no graphs or diagrams are provided in the image, there are none to describe.*
Expert Solution
Step 1
Hey, since there are multiple subparts posted, we will answer first three question. If you want any specific question to be answered then please submit that question only or specify the question number in your message.
1.
The test statistic is 1.775, which is obtained below:
2.
The degrees of freedom is,
The critical-value is obtained by using Excel function “=TINV(probability, degrees of freedom)”.
Output obtained from Excel is given below:
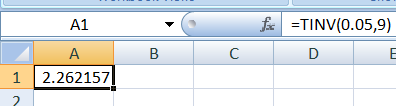
From the output, the critical value for two tailed test with 0.05 level is 2.262.
Step by step
Solved in 2 steps with 1 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.Recommended textbooks for you

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON

The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman

Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman