Let 3 and y denote the standard bases in the vector spaces M2x2(F) and P3 (F) respectively: 0 0 B = · ( ( )· ( ) ( ) ( :) ). 1 y = (1, x, x², x³). Find the matrices [T] and [U] for the operators defined as follows: (918). (a) For A € M2x2(F), T(A) = AB - BA, where B = (b) For p(x) = P3 (F), U(p(x)) = p(2) x³ – 2p'(x).
Let 3 and y denote the standard bases in the vector spaces M2x2(F) and P3 (F) respectively: 0 0 B = · ( ( )· ( ) ( ) ( :) ). 1 y = (1, x, x², x³). Find the matrices [T] and [U] for the operators defined as follows: (918). (a) For A € M2x2(F), T(A) = AB - BA, where B = (b) For p(x) = P3 (F), U(p(x)) = p(2) x³ – 2p'(x).
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
![Let \(\beta\) and \(\gamma\) denote the standard bases in the vector spaces \(M_{2 \times 2}(F)\) and \(P_3(F)\) respectively:
\[
\beta = \left( \left( \begin{array}{cc} 1 & 0 \\ 0 & 0 \end{array} \right), \left( \begin{array}{cc} 0 & 1 \\ 0 & 0 \end{array} \right), \left( \begin{array}{cc} 0 & 0 \\ 1 & 0 \end{array} \right), \left( \begin{array}{cc} 0 & 0 \\ 0 & 1 \end{array} \right) \right), \quad \gamma = (1, x, x^2, x^3).
\]
Find the matrices \([T]_\beta^\beta\) and \([U]_\gamma^\gamma\) for the operators defined as follows:
(a) For \(A \in M_{2 \times 2}(F)\), \(T(A) = AB - BA\), where \(B = \left( \begin{array}{cc} 0 & 1 \\ -1 & 0 \end{array} \right)\).
(b) For \(p(x) \in P_3(F)\), \(U(p(x)) = p(2) \, x^3 - 2p'(x)\).](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F32f77ee0-291c-46d0-b315-80fb2fd096d8%2F2631eff5-4f93-484e-81e1-53b47d66db4b%2F5d2cdbs_processed.jpeg&w=3840&q=75)
Transcribed Image Text:Let \(\beta\) and \(\gamma\) denote the standard bases in the vector spaces \(M_{2 \times 2}(F)\) and \(P_3(F)\) respectively:
\[
\beta = \left( \left( \begin{array}{cc} 1 & 0 \\ 0 & 0 \end{array} \right), \left( \begin{array}{cc} 0 & 1 \\ 0 & 0 \end{array} \right), \left( \begin{array}{cc} 0 & 0 \\ 1 & 0 \end{array} \right), \left( \begin{array}{cc} 0 & 0 \\ 0 & 1 \end{array} \right) \right), \quad \gamma = (1, x, x^2, x^3).
\]
Find the matrices \([T]_\beta^\beta\) and \([U]_\gamma^\gamma\) for the operators defined as follows:
(a) For \(A \in M_{2 \times 2}(F)\), \(T(A) = AB - BA\), where \(B = \left( \begin{array}{cc} 0 & 1 \\ -1 & 0 \end{array} \right)\).
(b) For \(p(x) \in P_3(F)\), \(U(p(x)) = p(2) \, x^3 - 2p'(x)\).
Expert Solution
Step 1
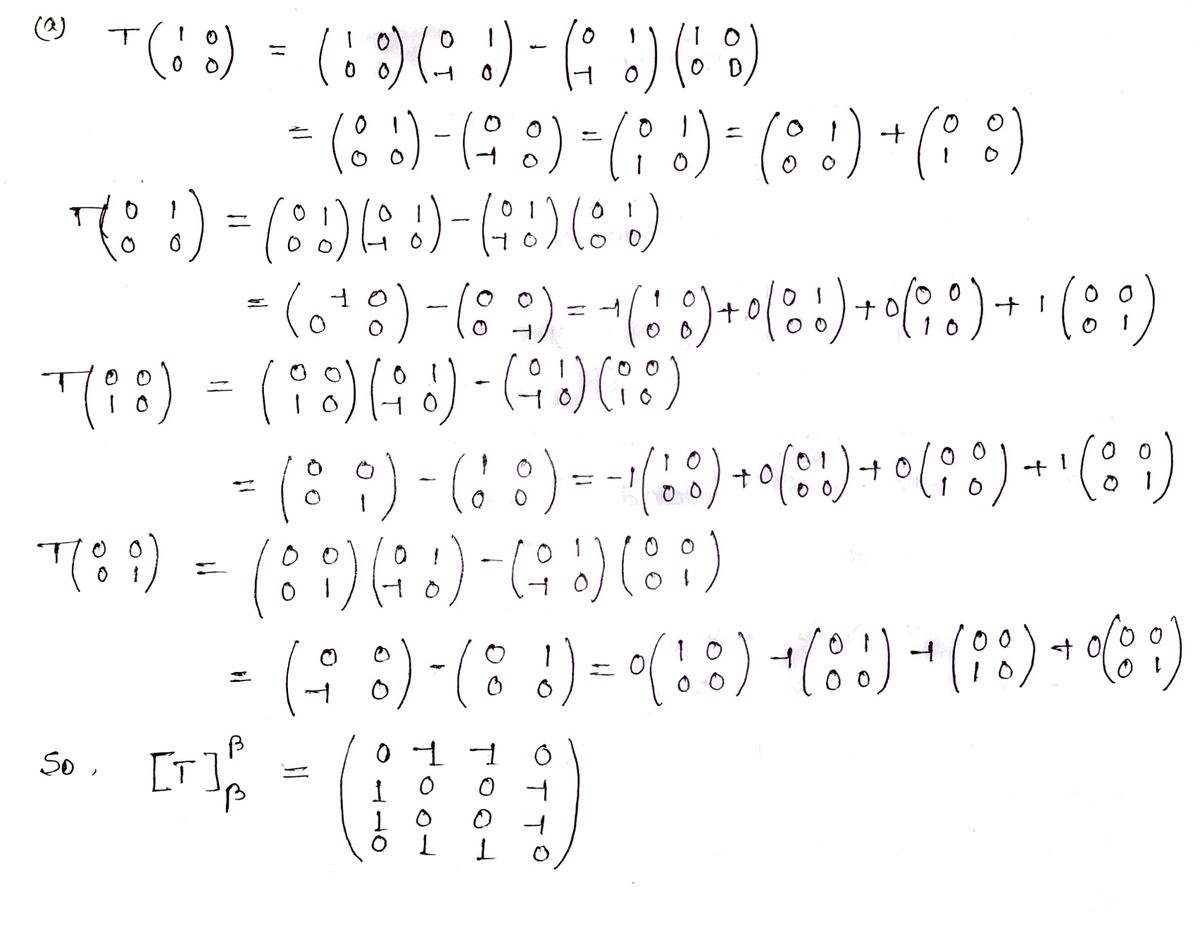
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

