itegral s Rdr y Fram Evaluate the where R2 = xt porial P(3,4 } fa) along the duied path op (b)alang the dinect path of P the path Ofg P tis the aigun. 6) alang P (3,4) 윤(3,0)
itegral s Rdr y Fram Evaluate the where R2 = xt porial P(3,4 } fa) along the duied path op (b)alang the dinect path of P the path Ofg P tis the aigun. 6) alang P (3,4) 윤(3,0)
Introductory Circuit Analysis (13th Edition)
13th Edition
ISBN:9780133923605
Author:Robert L. Boylestad
Publisher:Robert L. Boylestad
Chapter1: Introduction
Section: Chapter Questions
Problem 1P: Visit your local library (at school or home) and describe the extent to which it provides literature...
Related questions
Question
100%
U can use the second picture to derive part b and c I know how to do part a can u explain clearly how to do part b and c I am lost on the parameter of the integration and how to setup the integration operation ?
![**Example A (continued 3/4)**
(b) along the path \( OP_1P \)
\[ C = OP_1P = OP_1 + P_1P \]
\[
d\vec{\ell} = d\vec{y}; \quad [OP_1]
\]
\[
d\vec{\ell} = d\vec{x}; \quad [P_1P]
\]
\[
\int_C r^2 \, d\vec{\ell} = \int_0^{P_1} r^2 \, d\vec{y} + \int_{P_1}^P r^2 \, d\vec{x}
\]
\[
= \hat{a_y} \int_0^1 (0 + y^2) \, dy + \hat{a_x} \int_0^1 (x^2 + 1) \, dx
\]
\[
= \hat{a_y} (1/3) + \hat{a_x} (4/3)
\]
**Diagram Explanation:**
The diagram is a graph with points labeled on a coordinate plane. The path being evaluated is highlighted as follows:
- Point \( O \) is at the origin \((0,0)\).
- Point \( P_1 \) is at \( (0, 1) \).
- Point \( P_2 \) is at \( (1, 0) \).
- Point \( P \) is at \( (1, 1) \).
The path \( OP_1P \) is shown with lines indicating movement first along the \( y \)-axis from \( O \) to \( P_1 \), and then along the \( x \)-axis from \( P_1 \) to \( P \). The calculations are shown for integrating along these paths separately.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F6e3992ca-2280-40ba-b65a-68dc98c03d5d%2Fbf66b3be-c7d4-4d5e-a513-8ae1842e7dfd%2Fy5b8g4c_processed.jpeg&w=3840&q=75)
Transcribed Image Text:**Example A (continued 3/4)**
(b) along the path \( OP_1P \)
\[ C = OP_1P = OP_1 + P_1P \]
\[
d\vec{\ell} = d\vec{y}; \quad [OP_1]
\]
\[
d\vec{\ell} = d\vec{x}; \quad [P_1P]
\]
\[
\int_C r^2 \, d\vec{\ell} = \int_0^{P_1} r^2 \, d\vec{y} + \int_{P_1}^P r^2 \, d\vec{x}
\]
\[
= \hat{a_y} \int_0^1 (0 + y^2) \, dy + \hat{a_x} \int_0^1 (x^2 + 1) \, dx
\]
\[
= \hat{a_y} (1/3) + \hat{a_x} (4/3)
\]
**Diagram Explanation:**
The diagram is a graph with points labeled on a coordinate plane. The path being evaluated is highlighted as follows:
- Point \( O \) is at the origin \((0,0)\).
- Point \( P_1 \) is at \( (0, 1) \).
- Point \( P_2 \) is at \( (1, 0) \).
- Point \( P \) is at \( (1, 1) \).
The path \( OP_1P \) is shown with lines indicating movement first along the \( y \)-axis from \( O \) to \( P_1 \), and then along the \( x \)-axis from \( P_1 \) to \( P \). The calculations are shown for integrating along these paths separately.

Transcribed Image Text:**Title: Evaluating the Integral of \( R^2 \) Along Different Paths**
**Objective:**
Evaluate the integral \(\int R^2 \, dl\) where \(R^2 = x^2 + y^2\), from the origin \(O\) to the point \(P(3,4)\).
**Paths for Evaluation:**
1. **Path (a):** Along the direct path \(OP\)
2. **Path (b):** Along the direct path \(OAP\)
3. **Path (c):** Along the path \(OP_2P\)
**Diagram Explanation:**
- **Coordinates:**
- Origin \(O(0,0)\)
- Point \(A(0,4)\)
- Point \(P_2(3,0)\)
- Point \(P(3,4)\)
The diagram illustrates three paths:
- **Path \(OP\):** A diagonal line directly from \(O\) to \(P(3,4)\).
- **Path \(OAP\):** A two-segment path, first going vertically to \(A(0,4)\) and then horizontally to \(P(3,4)\).
- **Path \(OP_2P\):** A two-segment path, first going horizontally to \(P_2(3,0)\) and then vertically to \(P(3,4)\).
**Approach:**
This exercise requires evaluating an integral along different paths between defined points, exploring how the integral of \(R^2\) varies based on the path taken. This helps illustrate properties such as path independence in vector fields when applicable.
Expert Solution
Step 1
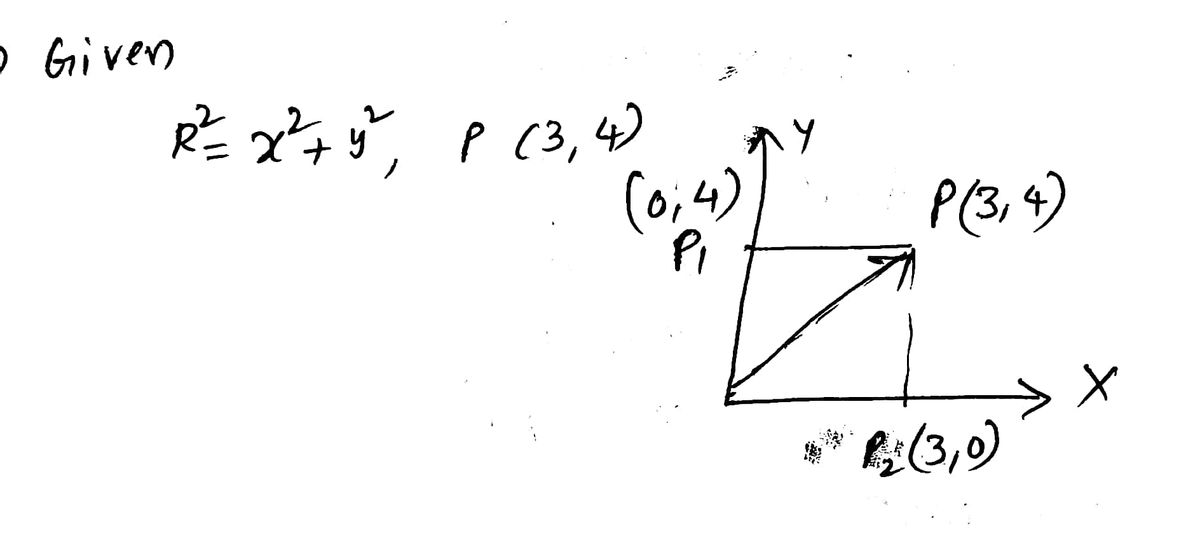
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Introductory Circuit Analysis (13th Edition)
Electrical Engineering
ISBN:
9780133923605
Author:
Robert L. Boylestad
Publisher:
PEARSON

Delmar's Standard Textbook Of Electricity
Electrical Engineering
ISBN:
9781337900348
Author:
Stephen L. Herman
Publisher:
Cengage Learning

Programmable Logic Controllers
Electrical Engineering
ISBN:
9780073373843
Author:
Frank D. Petruzella
Publisher:
McGraw-Hill Education

Introductory Circuit Analysis (13th Edition)
Electrical Engineering
ISBN:
9780133923605
Author:
Robert L. Boylestad
Publisher:
PEARSON

Delmar's Standard Textbook Of Electricity
Electrical Engineering
ISBN:
9781337900348
Author:
Stephen L. Herman
Publisher:
Cengage Learning

Programmable Logic Controllers
Electrical Engineering
ISBN:
9780073373843
Author:
Frank D. Petruzella
Publisher:
McGraw-Hill Education

Fundamentals of Electric Circuits
Electrical Engineering
ISBN:
9780078028229
Author:
Charles K Alexander, Matthew Sadiku
Publisher:
McGraw-Hill Education

Electric Circuits. (11th Edition)
Electrical Engineering
ISBN:
9780134746968
Author:
James W. Nilsson, Susan Riedel
Publisher:
PEARSON

Engineering Electromagnetics
Electrical Engineering
ISBN:
9780078028151
Author:
Hayt, William H. (william Hart), Jr, BUCK, John A.
Publisher:
Mcgraw-hill Education,