1:50Making conclusions in a z test for a pr...A business knew that 30% of its customerswere less than 25 years old. The businesswanted to increase this percentage, so theycreated a marketing campaign that targetedthis age group. 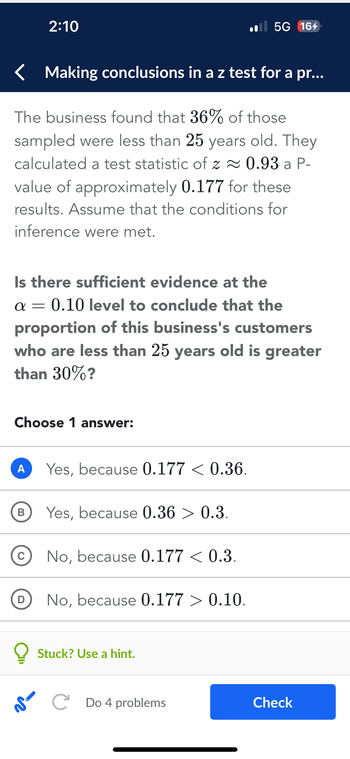After the campaign, thebusiness obtained a random sample of 50customers to test Ho: p = 0.3 versusHa : p > 0.3, where p is the proportion ofthis business's customers who are less than25 years old after the marketing campaign.The business found that 36% of thosesampled were less than 25 years old. Theycalculated a test statistic of z≈ 0.93 a P-value of approximately 0.177 for theseresults. Assume that the conditions forinference were met.αIs there sufficient evidence at the0.10 level to conclude that theproportion of this business's customerswho are less than 25 years old is greaterthan 30%?-5G 20Stuck? Use a hint.SC Do 4 problemsCheck

The given test statistic is 0.93 and P-value is 0.177.

Answered: Is there sufficient evidence at the a =…

MATLAB: An Introduction with Applications

6th Edition

ISBN:9781119256830

Author:Amos Gilat

Publisher:Amos Gilat

Chapter1: Starting With Matlab

Section: Chapter Questions

Problem 1P

See similar textbooks

Similar questions

Recall that Benford's Law claims that numbers chosen from very large data files tend to have "1" as the first nonzero digit disproportionately often. In fact, research has shown that if you randomly draw a number from a very large data file, the probability of getting a number with "1" as the leading digit is about 0.301. Now suppose you are the auditor for a very large corporation. The revenue file contains millions of numbers in a large computer data bank. You draw a random sample of n = 226 numbers from this file and r = 85 have a first nonzero digit of 1. Let p represent the population proportion of all numbers in the computer file that have a leading digit of 1.(i) Test the claim that p is more than 0.301. Use ? = 0.05. (a) What is the level of significance?State the null and alternate hypotheses. H0: p > 0.301; H1: p = 0.301 H0: p = 0.301; H1: p > 0.301 H0: p = 0.301; H1: p < 0.301 H0: p = 0.301; H1: p ≠ 0.301 (b) What sampling distribution will you use? The…
3. An assesses depreciated the machinery of his factory by 10% each in the first two years and by 40% in the third year and thereby claimed 21% average depreciation relief from taxation department, but the I.T.O. objected and allowed only 20%. Show which of the two is right. 4. The mean weight of 150 students in a certain class is 60 kilograms. The mean weight of boys in the class is 70 kilogram and that of the girls is 55 kilograms. Find the number of boys and number of girls in the class.
Recall that Benford's Law claims that numbers chosen from very large data files tend to have "1" as the first nonzero digit disproportionately often. In fact, research has shown that if you randomly draw a number from a very large data file, the probability of getting a number with "1" as the leading digit is about 0.301. Now suppose you are the auditor for a very large corporation. The revenue file contains millions of numbers in a large computer data bank. You draw a random sample of n = 228 numbers from this file and r = 85 have a first nonzero digit of 1. Let p represent the population proportion of all numbers in the computer file that have a leading digit of 1.(i) Test the claim that p is more than 0.301. Use ? = 0.10 (a) What is the value of the sample test statistic? (Round your answer to two decimal places.) (b) Find the P-value of the test statistic. (Round your answer to four decimal places.)
4. Along with interest rates, life expectancy is a component in pricing financial annuities. Suppose that you know that last year average life expectancy was 77 years for your annuity holders. Now you want to know if your clients this year have a longer life expectancy, on average, so you randomly sample n=20 of your recently deceased annuity holders to see actual age at death. Using a 5% level of significance, test whether or not the new data shows evidence of your annuity holders now live longer than 77 years, on average. The data below are the sample data (in years of life): (78,75,83,81,81,77,78,79,79,81,76,79,77,76,79,81,73,74,78,79) a) Does this sample indicate that life expectancy has increased? Test an appropriate hypothesis and state your conclusion (use a 5% level of significance). Be sure to check the necessary assumptions and conditions before conducting your test. b) Construct A 90% confidence interval for the true average age of death for the population of your annuity…
Recall that Benford's Law claims that numbers chosen from very large data files tend to have "1" as the first nonzero digit disproportionately often. In fact, research has shown that if you randomly draw a number from a very large data file, the probability of getting a number with "1" as the leading digit is about 0.301. Now suppose you are an auditor for a very large corporation. The revenue report involves millions of numbers in a large computer file. Let us say you took a random sample of n = 221 numerical entries from the file and r = 50 of the entries had a first nonzero digit of 1. Let p represent the population proportion of all numbers in the corporate file that have a first nonzero digit of 1. (i) Test the claim that p is less than 0.301. Use α = 0.05. (a) What is the level of significance? State the null and alternate hypotheses. Ho: P = 0.301; H₁: p = 0.301 Ho: P 0.301 Ho: P = 0.301; H₁: p 5 and nq > 5. O The Student's t, since np 5 and nq > 5. What is the value of the…
2. When you performed null hypothesis tests using a z-test for one sample proportion, what kinds of inferences can you draw about a past population's growth rate if you rejected the null hypothesis that the population proportion was 0.16?
1. For a certain year a study reports that the percentage of Americans aged 21 – 30 that had personal credit card debt was 72%. A researcher claims that this is too high for Americans aged 21 – 30 that have at least a Bachelor's degree. In a random sample of 320 Americans aged 21 – 30 with at least a Bachelor's degree, 208 of them had personal credit card debt. At a = 0.01, is the researcher correct %3D about her claim? а. State the null and alternative hypotheses AND identify which is the researcher's claim. b. Determine the critical value(s) that separate the rejection region(s) from the non-rejection region. c. Compute the appropriate test statistic and provide the corresponding p-value. d. Make your decision about the evidence and provide a justification.
Recall that Benford's Law claims that numbers chosen from very large data files tend to have "1" as the first nonzero digit disproportionately often. In fact, research has shown that if you randomly draw a number from a very large data file, the probability of getting a number with "1" as the leading digit is about 0.301. Now suppose you are the auditor for a very large corporation. The revenue file contains millions of numbers in a large computer data bank. You draw a random sample of n = 226 numbers from this file and r = 87 have a first nonzero digit of 1. Let p represent the population proportion of all numbers in the computer file that have a leading digit of 1. 1) Test the claim that p is more than 0.301. Use α = 0.10. 2) What is the value of the sample test statistic? (Round your answer to two decimal places.) 3) Find the P-value of the test statistic. (Round your answer to four decimal places.) 4) If p is in fact larger than 0.301, it would seem there are too many numbers in…
Recall that Benford's Law claims that numbers chosen from very large data files tend to have "1" as the first nonzero digit disproportionately often. In fact, research has shown that if you randomly draw a number from a very large data file, the probability of getting a number with "1" as the leading digit is about 0.301. Now suppose you are an auditor for a very large corporation. The revenue report involves millions of numbers in a large computer file. Let us say you took a random sample of n = 223 numerical entries from the file and r = 48 of the entries had a first nonzero digit of 1. Let p represent the population proportion of all numbers in the corporate file that have a first nonzero digit of 1.(i) Test the claim that p is less than 0.301. Use ? = 0.05. (a) What is the level of significance?State the null and alternate hypotheses. H0: p < 0.301; H1: p = 0.301 H0: p = 0.301; H1: p > 0.301 H0: p = 0.301; H1: p < 0.301 H0: p = 0.301; H1: p ≠ 0.301 (b) What sampling…
Recall that Benford's Law claims that numbers chosen from very large data files tend to have "1" as the first nonzero digit disproportionately often. In fact, research has shown that if you randomly draw a number from a very large data file, the probability of getting a number with "1" as the leading digit is about 0.301. Now suppose you are an auditor for a very large corporation. The revenue report involves millions of numbers in a large computer file. Let us say you took a random sample of n = 225 numerical entries from the file and r = 51 of the entries had a first nonzero digit of 1. Let p represent the population proportion of all numbers in the corporate file that have a first nonzero digit of 1.(i) Test the claim that p is less than 0.301. Use ? = 0.05.
Recall that Benford's Law claims that numbers chosen from very large data files tend to have "1" as the first nonzero digit disproportionately often. In fact, research has shown that if you randomly draw a number from a very large data file, the probability of getting a number with "1" as the leading digit is about 0.301. Now suppose you are an auditor for a very large corporation. The revenue report involves millions of numbers in a large computer file. Let us say you took a random sample of n = 220 numerical entries from the file and r = 49 of the entries had a first nonzero digit of 1. Let p represent the population proportion of all numbers in the corporate file that have a first nonzero digit of 1. A) What is the value of the sample test statistic? (Round your answer to two decimal places.)B) Find the P-value of the test statistic. (Round your answer to four decimal places.)
1. The data in Table 7–6 were collected in a clinical trial to evaluate a new compound designed to improve wound healing in trauma patients. The new compound was com- pared against a placebo. After treatment for 5 days with the new compound or placebo, the extent of wound healing was measured. Is there a difference in the extent of wound healing between the treatments? (Hint: Are treatment and the percent wound healing independent?) Run the appropriate test at a 5% level of significance. Use the data in Problem 1. Pool the data across the treatments into one sample of size n = 250. Use the pooled data to test whether the distribution of the percent wound healing is approximately normal. Specifically, use the following distribution 30%, 40%, 20%, and 10% and a = 0.05 to run the appropriate test. Percent Wound Healing Treatment 0-25% 26-50% 51-75% 76-100% New compound (n=125) 15 37 32 41 Placebo (n=125) 36 45 34 10

SEE MORE QUESTIONS

Recommended textbooks for you

MATLAB: An Introduction with Applications

Statistics

ISBN:

9781119256830

Author:

Amos Gilat

Publisher:

John Wiley & Sons Inc

Probability and Statistics for Engineering and th…

Statistics

ISBN:

9781305251809

Author:

Jay L. Devore

Publisher:

Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…

Statistics

ISBN:

9781305504912

Author:

Frederick J Gravetter, Larry B. Wallnau

Publisher:

Cengage Learning

MATLAB: An Introduction with Applications

Statistics

ISBN:

9781119256830

Author:

Amos Gilat

Publisher:

John Wiley & Sons Inc

Probability and Statistics for Engineering and th…

Statistics

ISBN:

9781305251809

Author:

Jay L. Devore

Publisher:

Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…

Statistics

ISBN:

9781305504912

Author:

Frederick J Gravetter, Larry B. Wallnau

Publisher:

Cengage Learning

Elementary Statistics: Picturing the World (7th E…

Statistics

ISBN:

9780134683416

Author:

Ron Larson, Betsy Farber

Publisher:

PEARSON

The Basic Practice of Statistics

Statistics

ISBN:

9781319042578

Author:

David S. Moore, William I. Notz, Michael A. Fligner

Publisher:

W. H. Freeman

Introduction to the Practice of Statistics

Statistics

ISBN:

9781319013387

Author:

David S. Moore, George P. McCabe, Bruce A. Craig

Publisher:

W. H. Freeman

SEE MORE TEXTBOOKS

Is there sufficient evidence at the a = 0.10 level to conclude that the proportion of this business's customers who are less than 25 years old is greater than 30%?