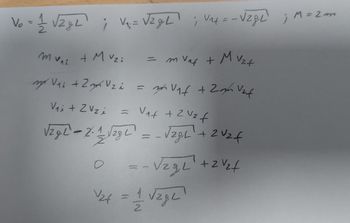Consider a pendulum of mass m and length L. The suspension point of the pendulum it’s on a height L. The pendulum is released from the horizontal position, on its lowest point collides with a block of mass M=2m, the block has a velocity of -V0 in the x axis. The pendulum bounces reaching the initial, horizontal position. If the collision is elastic, A) Find de speed v0 as a function of m, L and g. B) Find de velocity of the block M after the collision.
Hi, con you help me with this problem please?
Consider a pendulum of mass m and length L. The suspension point of the pendulum it’s on a height L. The pendulum is released from the horizontal position, on its lowest point collides with a block of mass M=2m, the block has a velocity of -V0 in the x axis. The pendulum bounces reaching the initial, horizontal position. If the collision is elastic,
A) Find de speed v0 as a function of m, L and g.
B) Find de velocity of the block M after the collision.
Thanks.

Step by step
Solved in 3 steps

in step 3, is there a minus ( - ) missing in the initial velocity of the block?
Knowing that v0= 1/2 sqrt (2gL) ; v1i = sqrt(2gL) ; v1f = -sqrt(2gL) ; M=2m
By the conservation of the momentum, wouldn't the velocity of the block after the collision be v0?
Thanks