College Physics
11th Edition
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Raymond A. Serway, Chris Vuille
Chapter1: Units, Trigonometry. And Vectors
Section: Chapter Questions
Problem 1CQ: Estimate the order of magnitude of the length, in meters, of each of the following; (a) a mouse, (b)...
Related questions
Question
100%
![**Problem:**
Find the total resistance (µΩ) of the combination of resistors shown in the figure below.
Given:
- \( R_1 = 1.0 \, \mu \Omega \)
- \( R_2 = 2.0 \, \mu \Omega \)
- \( R_3 = 3.0 \, \mu \Omega \)
**Diagram Explanation:**
The diagram consists of three resistors, \( R_1 \), \( R_2 \), and \( R_3 \), connected in a triangular configuration. Resistors \( R_1 \) and \( R_2 \) are in series along the top, and \( R_3 \) is connected in parallel below them, completing a triangular loop.
**Steps to Calculate Total Resistance:**
1. **Series Combination:**
- When resistors are in series, their resistances add up:
\[
R_{\text{series}} = R_1 + R_2
\]
Substitute the given values:
\[
R_{\text{series}} = 1.0 \, \mu\Omega + 2.0 \, \mu\Omega = 3.0 \, \mu\Omega
\]
2. **Parallel Combination:**
- The total resistance \( R_{\text{total}} \) for resistors in parallel is given by:
\[
\frac{1}{R_{\text{total}}} = \frac{1}{R_{\text{series}}} + \frac{1}{R_3}
\]
Substitute the values:
\[
\frac{1}{R_{\text{total}}} = \frac{1}{3.0 \, \mu\Omega} + \frac{1}{3.0 \, \mu\Omega}
\]
\[
\frac{1}{R_{\text{total}}} = \frac{2}{3.0 \, \mu\Omega}
\]
\[
R_{\text{total}} = \frac{3.0}{2} \, \mu\Omega = 1.5 \, \mu\Omega
\]
Thus, the total resistance of the circuit is \( R_{\text{total}} = 1.5 \, \mu\Omega \).](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fb98782ef-20ba-41ff-a405-9d515999d412%2F1fe335f8-3658-4e5f-9ea4-e57f63c20782%2Fzg7xay6_processed.png&w=3840&q=75)
Transcribed Image Text:**Problem:**
Find the total resistance (µΩ) of the combination of resistors shown in the figure below.
Given:
- \( R_1 = 1.0 \, \mu \Omega \)
- \( R_2 = 2.0 \, \mu \Omega \)
- \( R_3 = 3.0 \, \mu \Omega \)
**Diagram Explanation:**
The diagram consists of three resistors, \( R_1 \), \( R_2 \), and \( R_3 \), connected in a triangular configuration. Resistors \( R_1 \) and \( R_2 \) are in series along the top, and \( R_3 \) is connected in parallel below them, completing a triangular loop.
**Steps to Calculate Total Resistance:**
1. **Series Combination:**
- When resistors are in series, their resistances add up:
\[
R_{\text{series}} = R_1 + R_2
\]
Substitute the given values:
\[
R_{\text{series}} = 1.0 \, \mu\Omega + 2.0 \, \mu\Omega = 3.0 \, \mu\Omega
\]
2. **Parallel Combination:**
- The total resistance \( R_{\text{total}} \) for resistors in parallel is given by:
\[
\frac{1}{R_{\text{total}}} = \frac{1}{R_{\text{series}}} + \frac{1}{R_3}
\]
Substitute the values:
\[
\frac{1}{R_{\text{total}}} = \frac{1}{3.0 \, \mu\Omega} + \frac{1}{3.0 \, \mu\Omega}
\]
\[
\frac{1}{R_{\text{total}}} = \frac{2}{3.0 \, \mu\Omega}
\]
\[
R_{\text{total}} = \frac{3.0}{2} \, \mu\Omega = 1.5 \, \mu\Omega
\]
Thus, the total resistance of the circuit is \( R_{\text{total}} = 1.5 \, \mu\Omega \).
Expert Solution
Step 1
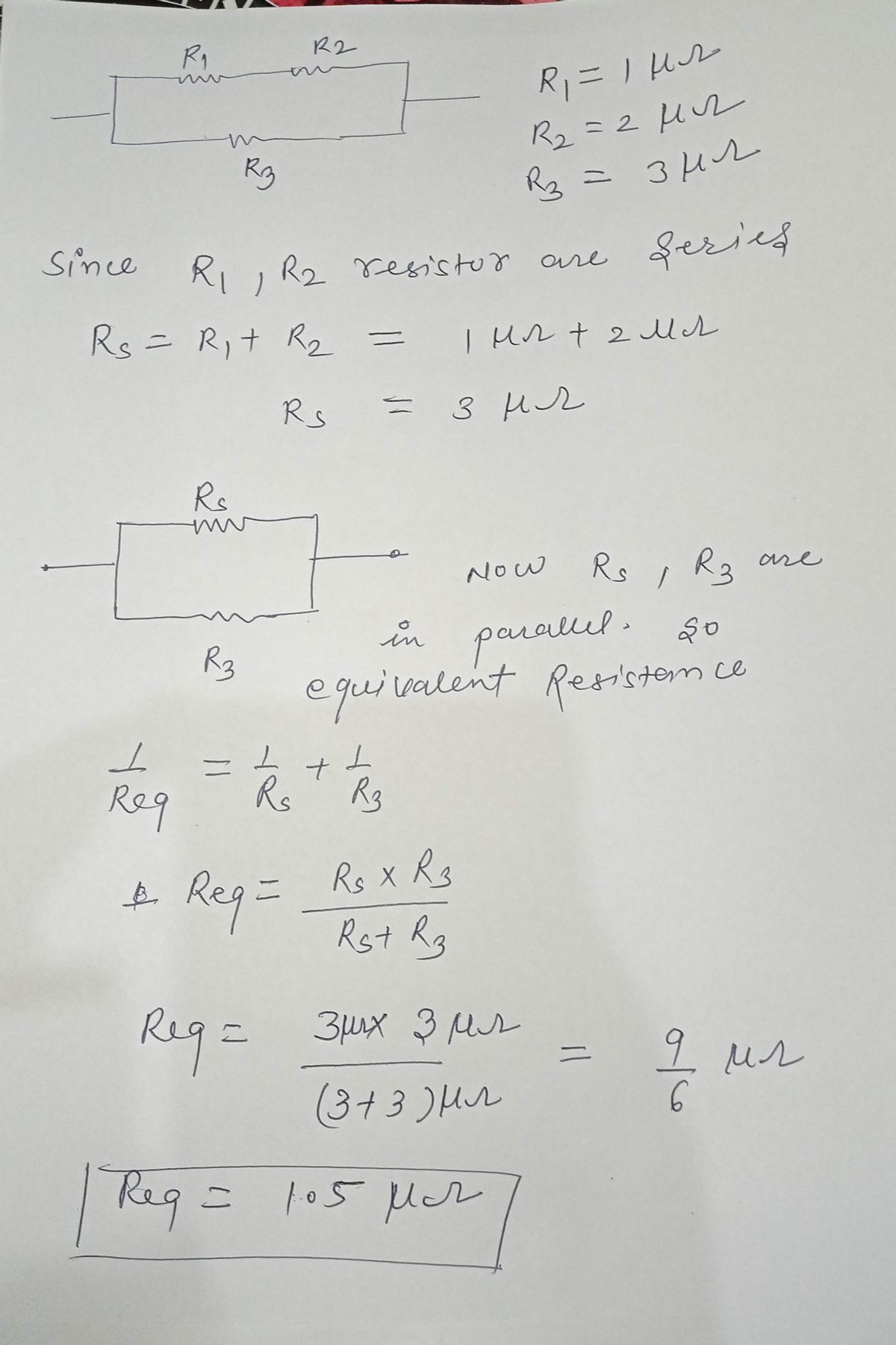
Step by step
Solved in 2 steps with 1 images

Recommended textbooks for you

College Physics
Physics
ISBN:
9781305952300
Author:
Raymond A. Serway, Chris Vuille
Publisher:
Cengage Learning

University Physics (14th Edition)
Physics
ISBN:
9780133969290
Author:
Hugh D. Young, Roger A. Freedman
Publisher:
PEARSON

Introduction To Quantum Mechanics
Physics
ISBN:
9781107189638
Author:
Griffiths, David J., Schroeter, Darrell F.
Publisher:
Cambridge University Press

College Physics
Physics
ISBN:
9781305952300
Author:
Raymond A. Serway, Chris Vuille
Publisher:
Cengage Learning

University Physics (14th Edition)
Physics
ISBN:
9780133969290
Author:
Hugh D. Young, Roger A. Freedman
Publisher:
PEARSON

Introduction To Quantum Mechanics
Physics
ISBN:
9781107189638
Author:
Griffiths, David J., Schroeter, Darrell F.
Publisher:
Cambridge University Press

Physics for Scientists and Engineers
Physics
ISBN:
9781337553278
Author:
Raymond A. Serway, John W. Jewett
Publisher:
Cengage Learning

Lecture- Tutorials for Introductory Astronomy
Physics
ISBN:
9780321820464
Author:
Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina Brissenden
Publisher:
Addison-Wesley

College Physics: A Strategic Approach (4th Editio…
Physics
ISBN:
9780134609034
Author:
Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field
Publisher:
PEARSON