Answered: Find the moment of inertia of a solid… | bartleby

Related questions

Question

Find the moment of inertia of a solid cylinder of mass m, length l and radius R about the following axis:

a. A

b. O (CM)

Expert Solution

Step 1

Step 2

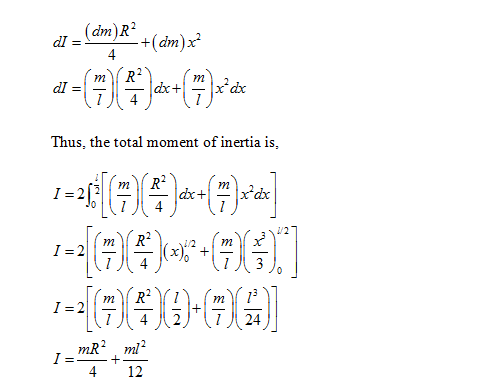
Step by step

Solved in 3 steps with 4 images

Blurred answer

Similar questions

Determine the angular momentum of a 78-g particle about the origin of coordinates when the particle is at x = 4.9 m, y = -6.3 m, and it has velocity v = (3.51-80k) m/s. Find the x-component. Express your answer using two significant figures. Lx = ΜΕ ΑΣΦ Submit Request Answer ▼ Part B Find the y-component. Express your answer using two significant figures. Ly= ΜΕ ΑΣΦ Submit Request Answer Part C Find the z-component. Express your answer using two significant figures. ? kg-m²/s ? kg-m²/s
24. o A car is traveling along a road, and its engine is turning over with an angular velocity of +220 rad/s. The driver steps on the accelerator, and in a time of 10.0 s the angular velocity increases to +280 rad/s. (a) What would have been the angular displacement of the engine if its angular velocity had remained constant at the initial value of +220 rad/s during the entire 10.0-s interval? (b) What would have been the angular displacement if the angular velocity had been equal to its final value of +280 rad/s during the entire 10.0-s interval? (c) Determine the actual value of the angular displacement during the 10.0-s interval.
В C P D The above diagram shows four objects, A, B, C, and D, all moving to the right in a straight line at the same constant speed. Which one of the following statements is correct about the angular momenta La, LB, Lc, and Lp of the objects, with respect to point P? O LA = LB = Lc = LD> 0 O LA > LB = Lc > Lp = 0 LA = LB = Lc = LD = 0 LA> LB > Lc> LD = 0 LA > Lc> LB > LD = 0
R M A solid sphere of uniform density starts from rest and rolls without slipping down an inclined plane with angle 0 = 30°. The sphere has mass M = 8 kg and radius R = 0.19 m . The coefficient of static friction between the sphere and the plane is u = 0.64. What is the magnitude of the frictional force on the sphere? Ff =
A uniform bar has two small balls glued to its ends. The bar is 1.9 m long and has mass 1.5 kg, while the balls each have mass 0.5 kg and can be treated as point masses. Find the moment of inertia of this combination about an axis perpendicular to the bar and through one of the balls. the answer's unit is. Needs Complete typed solution with 100 % accuracy.
A force F = 71 N i acts on an object at a point (x0, y0) = (2.8 m, 2.2 m) as shown in the figure. What is the magnitude of the torque generated by this force about the origin? What is the magnitude of the torque generated by this force about the point (x, y) = (1.1 m, 0.9 m)? Suppose the object is free to rotate about the z axis. If the object has a moment of inertia I = 61.0 kg*m2 for rotation about the z axis, what is the magnitude of the angular acceleration of the object due to the application of the force F?
A uniform thin rod of mass M = 3.37 kg pivots about an axis through its center and perpendicular to its length. Two small bodies, each of mass m = 0.219 kg, are attached to the ends of the rod. What must the length L of the rod be so that the moment of inertia of the three-body system with respect to the described axis is I = 0.975 kg-m? ? L = m M. m m L
A bicycle wheel of mass M (all at the edges) and radius r is fixed about an axis through its center and is initially at rest. You wrap the wheel with a cord and attach a hanging weight of mass m to the cord. You release the weight and allow it to fall a distance d. The weight falls smoothly, and the cord does not slip over the wheel as it unwinds. a. (Write an expression for the angular acceleration α of the wheel in terms of the tension T of the cord.b. Write an expression for the linear acceleration a of the weight in terms of the tension T of the cord.c. (Using parts (a) and (b) find the linear acceleration in terms of M, m, and g.d. Write an expression for the linear speed v of the weight after it falls a distance d.e. For M = 7.5 kg, r = 33 cm, m = 250 g, and d = 1.5 m, what is the rotational kinetic energy of the wheel afterthe weight has fallen?
P A 1 n 1. A uniform solid sphere rolls without slipping down a 27° inclined plane. What is the acceleration of the sphere's center of mass? The moment of inertia of a uniform solid sphere about an axis that passes through its center = mr²2, The moment of inertia of a uniform solid sphere about an axis that is tangent to its surface = 7/5mr², m/s2 N 2 W S #3 X H command E с D $ 4 C R FL % 5 V 1332 Search or type URL T G ^ MacBook Pro 6 B Y H & 7 N U J * 00 1332 8 M - + K ( 9 O < ) 0 L P ^ : ; command option { [ ? 1 + 11
a top with radius 10cm, mass 2 kg has a string around the edge and is initially at rest. assume the top can be reasonably approximated as a solid cylinder. the top is held in place as the string is pulled. the string applies a force of 10N tangential to the edge for 2s. what is the angular velocity (in radians per second) of the top after 3 seconds? what is the angular momentum of the top after the string is pulled? after the string is pulled, the top is released. it travels away from its initial position at a linear velocity of 10m/s. what is the rotational velocity (in radians per s) at this top? assume no friction
A string is wrapped around a uniform solid cylinder of radius r, as shown in The Picture. The cylinder can rotate freely about its axis. The loose end of the string is attached to a block. The block and cylinder each have mass m. Find the magnitude (alpha(α)) of the angular acceleration of the cylinder as the block descends.
A disk of radius R and thickness t has a mass density that increases from the center outward, given by p = po (r/R), where r is the distance from the axis of the disk. What is the moment of inertia about the disk axis in terms of M and R? O MR 2MR O sMP 3MR 5 O 2MR 3 5MR 3

SEE MORE QUESTIONS