Do larger universities tend to have more property crime? University crime statistics are affected by a variety of factors. The surrounding community, accessibility given to outside visitors, and many other factors influence crime rate. Let x be a variable that represents student enrollment (in thousands) on a university campus, and let ybe a variable that represents the number of burglaries in a year on the university campus. A random sample of n = 8 universities in California gave the following information about enrollments and annual burglary incidents. x 10.5 30.6 24.5 14.3 7.5 27.7 16.2 20.1 y 23 75 39 23 15 30 15 25 (a)Make a scatter diagram of the data. Then visualize the line you think best fits the data. (Submit a file with a maximum size of 1 MB.) (b)Use a calculator to verify that Σ(x) = 151.4, Σ(x2) = 3341.34, Σ(y) = 245,Σ(y2) = 10179 and Σ(x y) = 5509.9. Compute r. (Enter a number. Round to 3 decimal places.)As x increases, does the value of r imply that y should tend to increase or decrease? Explain your answer.Given our value of r, y should tend to increase as x increases.Given our value of r, we can not draw any conclusions for the behavior of y as x increases. Given our value of r, y should tend to decrease as x increases.Given our value of r, y should tend to remain constant as x increases.
Contingency Table
A contingency table can be defined as the visual representation of the relationship between two or more categorical variables that can be evaluated and registered. It is a categorical version of the scatterplot, which is used to investigate the linear relationship between two variables. A contingency table is indeed a type of frequency distribution table that displays two variables at the same time.
Binomial Distribution
Binomial is an algebraic expression of the sum or the difference of two terms. Before knowing about binomial distribution, we must know about the binomial theorem.
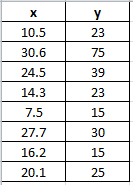
Do larger universities tend to have more property crime? University crime statistics are affected by a variety of factors. The surrounding community, accessibility given to outside visitors, and many other factors influence crime rate. Let x be a variable that represents student enrollment (in thousands) on a university campus, and let ybe a variable that represents the number of burglaries in a year on the university campus. A random sample of n = 8 universities in California gave the following information about enrollments and annual burglary incidents.
| x | 10.5 | 30.6 | 24.5 | 14.3 | 7.5 | 27.7 | 16.2 | 20.1 |
|---|---|---|---|---|---|---|---|---|
| y | 23 | 75 | 39 | 23 | 15 | 30 | 15 | 25 |
(a)Make a scatter diagram of the data. Then visualize the line you think best fits the data. (Submit a file with a maximum size of 1 MB.)
(b)Use a calculator to verify that Σ(x) = 151.4, Σ(x2) = 3341.34, Σ(y) = 245,Σ(y2) = 10179 and Σ(x y) = 5509.9.
Compute r. (Enter a number. Round to 3 decimal places.)As x increases, does the value of r imply that y should tend to increase or decrease? Explain your answer.Given our value of r, y should tend to increase as x increases.Given our value of r, we can not draw any conclusions for the behavior of y as x increases. Given our value of r, y should tend to decrease as x increases.Given our value of r, y should tend to remain constant as x increases.
The question is about correlation
Given :
To find :
a ) scatter diagram
b ) Verify given values
c ) Find r and interpret it
Step by step
Solved in 2 steps with 3 images









