According the April 12, 2017 Pew Research survey, 58% of Americans approve of U.S. missile strikes in Syria in response to reports of the use of chemical weapons by Bashar al-Assads government (the Syrian government). A sample of 50 Americans are surveyed. Let p be the sample proportion of Americans who approve the U.S. missile strikes. 1. What is the population proportion? 58 (decimal form) 2. What is the sample size? 50 3. Can the normal approximation be used with this distribution? Yes, the sample distribution meets the "rule of thumb
According the April 12, 2017 Pew Research survey, 58% of Americans approve of U.S. missile strikes in Syria in response to reports of the use of chemical weapons by Bashar al-Assads government (the Syrian government). A sample of 50 Americans are surveyed. Let p be the sample proportion of Americans who approve the U.S. missile strikes. 1. What is the population proportion? 58 (decimal form) 2. What is the sample size? 50 3. Can the normal approximation be used with this distribution? Yes, the sample distribution meets the "rule of thumb
A First Course in Probability (10th Edition)
10th Edition
ISBN:9780134753119
Author:Sheldon Ross
Publisher:Sheldon Ross
Chapter1: Combinatorial Analysis
Section: Chapter Questions
Problem 1.1P: a. How many different 7-place license plates are possible if the first 2 places are for letters and...
Related questions
Question

Transcribed Image Text:**Analyzing Public Opinion on U.S. Missile Strikes in Syria: A Statistical Approach**
**Background:**
According to a Pew Research survey conducted on April 12, 2017, 58% of Americans approve of U.S. missile strikes in Syria, responding to reports of chemical weapons usage by Bashar al-Assad's government (the Syrian government). In this scenario, a sample of 50 Americans is used to determine the proportion that approve of the missile strikes. The sample proportion is represented by \( \hat{p} \).
**Statistical Analysis:**
1. **Population Proportion (p):**
- Given as 0.58 (in decimal form).
2. **Sample Size (n):**
- Given as 50.
3. **Normal Approximation:**
- Yes, the sample distribution meets the "rule of thumb" criteria.
4. **Mean of the Sampling Proportion:**
- Equal to the population proportion, 0.58.
5. **Standard Deviation of the Sampling Proportion:**
- Needs to be calculated and rounded to four decimal places. (An incorrect value is indicated in the image.)
6. **Probability Calculations:**
a. **Probability that no more than 25 of the 50 Americans approve of the strikes:**
- Calculate the z-score and then find the probability.
- \( \hat{p} \) is 0.5.
- Calculated z-score: -0.83 (rounded to the nearest hundredth).
- Associated probability: P(\(\hat{p} \leq\) 0.5).
b. **Probability that more than 30 of the 50 Americans approve of the strikes:**
- Calculate the z-score and then find the probability.
- \( \hat{p} \) is 0.6.
- Calculated z-score: 0.67 (rounded to the nearest hundredth).
- Associated probability: P(\(\hat{p} > 0.6\)).
**Note:**
This educational exercise illustrates how statistical principles can be applied in real-world scenarios to understand public opinion. Calculating the z-scores and probabilities requires using standard statistical formulas and understanding the normal distribution.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 3 steps

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
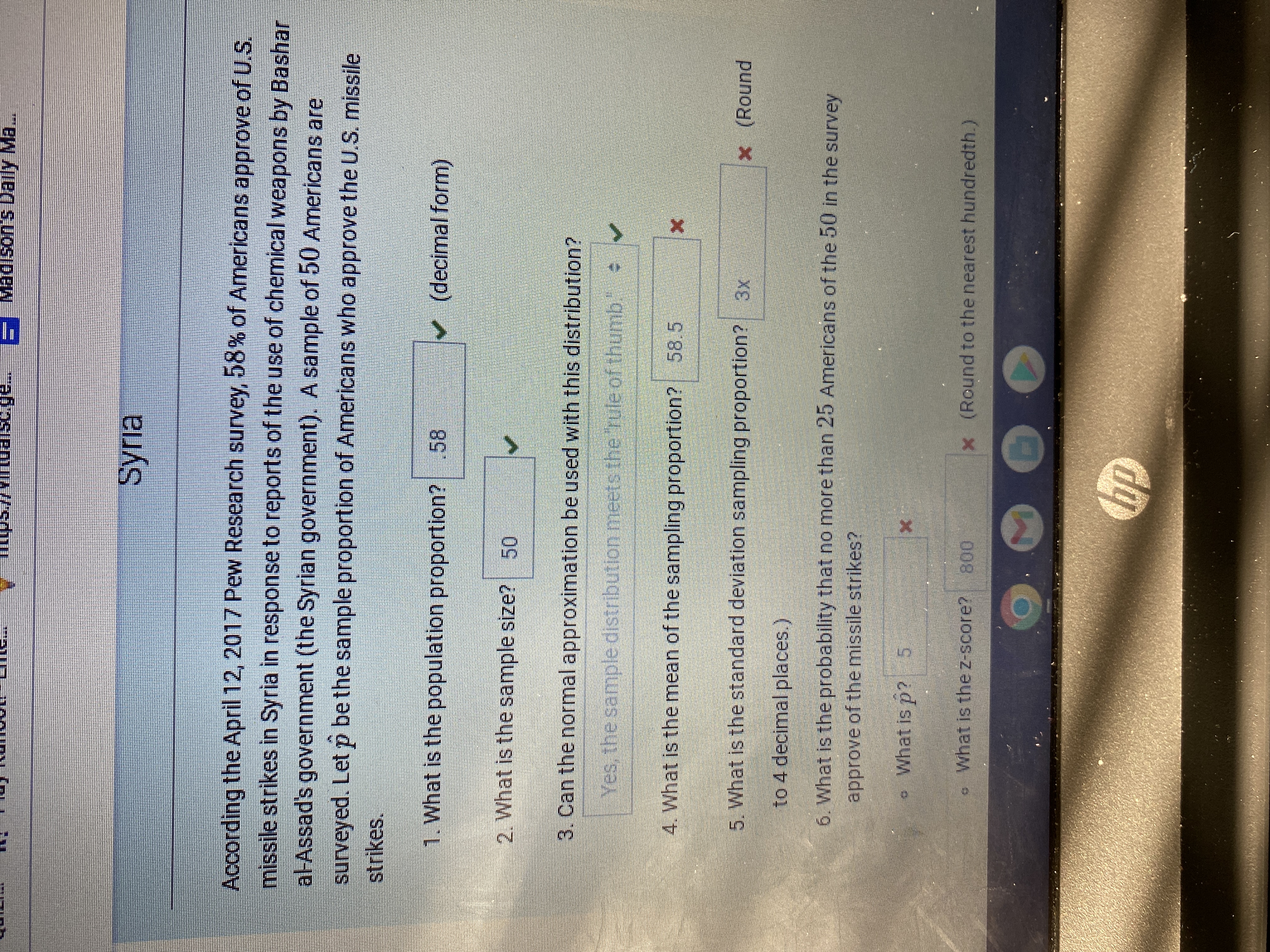
Transcribed Image Text:Syria
According the April 12, 2017 Pew Research survey, 58% of Americans approve of U.S.
missile strikes in Syria in response to reports of the use of chemical weapons by Bashar
al-Assad's government (the Syrian government). A sample of 50 Americans are
surveyed. Let p be the sample proportion of Americans who approve the U.S. missile
strikes.
1. What is the population proportion? .58
(decimal form)
2. What is the sample size?
3. Can the normal approximation be used with this distribution?
Yes, the sample distribution meets the "rule of thumb.
4. What is the mean of the sampling proportion? 58.5
5. What is the standard deviation sampling proportion? 3x
x (Round
to 4 decimal places.)
6. What is the probability that no more than 25 Americans of the 50 in the survey
approve of the missile strikes?
oWhat is p? 5
What is the z-score? 800
X (Round to the nearest hundredth.)
Solution
Recommended textbooks for you

A First Course in Probability (10th Edition)
Probability
ISBN:
9780134753119
Author:
Sheldon Ross
Publisher:
PEARSON


A First Course in Probability (10th Edition)
Probability
ISBN:
9780134753119
Author:
Sheldon Ross
Publisher:
PEARSON
