a. A bag contains 66 greengreen marbles, 99 blueblue marbles, and 88 yellowyellow marbles. If a marble is drawn from the bag, replaced, and another marble is drawn, what is the probability of drawing first a greengreen marble and then a yellowyellow marble? b. A bag contains 99 whitewhite marbles, 88 yellowyellow marbles, and 66 blueblue marbles. If two different marbles are drawn from the bag , what is the probability of drawing first a whitewhite marble and then a blueblue marble?
Round all answers to 4 decimal places.
a. A bag contains 66 greengreen marbles, 99 blueblue marbles, and 88 yellowyellow marbles. If a marble is drawn from the bag, replaced, and another marble is drawn, what is the
b. A bag contains 99 whitewhite marbles, 88 yellowyellow marbles, and 66 blueblue marbles. If two different marbles are drawn from the bag , what is the probability of drawing first a whitewhite marble and then a blueblue marble?
(a)
Obtain the probability of drawing first a green marble and then a yellow marble.
The probability of drawing first a green marble and then a yellow marble is obtained below as follows:
From the information, given that
The total number of outcomes =23(=6G+9B+8Y)
The total number of green variables=6
The total number of blue variables=9
The total number of yellow variables=8
Given that a marble is drawn and then replaced and again another marble is drawn.
Let E denote the event of drawing first a green marble and then a yellow marble.
The required probability is,
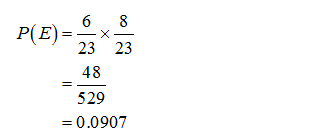
The probability of drawing first a green marble and then a yellow marble is 0.0907.
Step by step
Solved in 2 steps with 2 images









