A researcher wanted to examine whether type of dog food (freeze dried meat vs generic brand kibble) would affect dogs' attention span. The researcher hypothesized that dogs who ate freeze dried meat would not have the same attention span as those who ate generic kibble. The researcher used a single sample of 13 dogs, that took part in both treatment conditions. The mean was 7.0 and the SS for the difference in attention spans was 144. Alpha was set at the 0.05 level. Calculate the appropriate
A researcher wanted to examine whether type of dog food (freeze dried meat vs generic brand kibble) would affect dogs' attention span. The researcher hypothesized that dogs who ate freeze dried meat would not have the same attention span as those who ate generic kibble. The researcher used a single sample of 13 dogs, that took part in both treatment conditions. The mean was 7.0 and the SS for the difference in attention spans was 144. Alpha was set at the 0.05 level. Calculate the appropriate
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
Related questions
Question
A researcher wanted to examine whether type of dog food (freeze dried meat vs generic brand kibble) would affect dogs' attention span. The researcher hypothesized that dogs who ate freeze dried meat would not have the same attention span as those who ate generic kibble. The researcher used a single sample of 13 dogs, that took part in both treatment conditions. The mean was 7.0 and the SS for the difference in attention spans was 144. Alpha was set at the 0.05 level.
Calculate the appropriate t to the nearest thousandths and include the +/- value in front of the t. For example, -5.369.
What is the corresponding critical t?
What decision can you make about the null hypothesis?
Was there a treatment effect?
Transcribed Image Text:This image displays a t-Distribution table, commonly used in statistics to determine the critical t-value for a given degree of freedom (df) and cumulative probability. The table is structured in a grid format, with columns representing different t-values (t₀.₅₀, t₀.₂₅, etc.) and rows displaying the degrees of freedom, ranging from 2 to 1000.
### Table Headers:
- The table headers indicate cumulative probabilities for both one-tail and two-tail tests. Probabilities range from 0.50 to 0.0005 for one-tailed tests and 1.00 to 0.001 for two-tailed tests.
### Confidence Levels:
- A row at the bottom aligns the confidence levels with cumulative probability, ranging from 50% to 99.9%.
### Table Content:
- Each cell intersection between a degree of freedom and a cumulative probability column represents a t-value. These are critical values required for hypothesis testing, often used in constructing confidence intervals or conducting t-tests.
The table is shaded in alternating rows to enhance readability, helping users quickly locate the required t-value. The degrees of freedom (df) on the leftmost column range from 2 to 1000, with a bottom row for 'z' values, representing the standard normal distribution used in large sample sizes.
This table is an essential tool for students and professionals in statistics, allowing them to determine the critical points of the t-distribution for hypothesis testing and other statistical analyses.
Expert Solution
Step 1
Solution
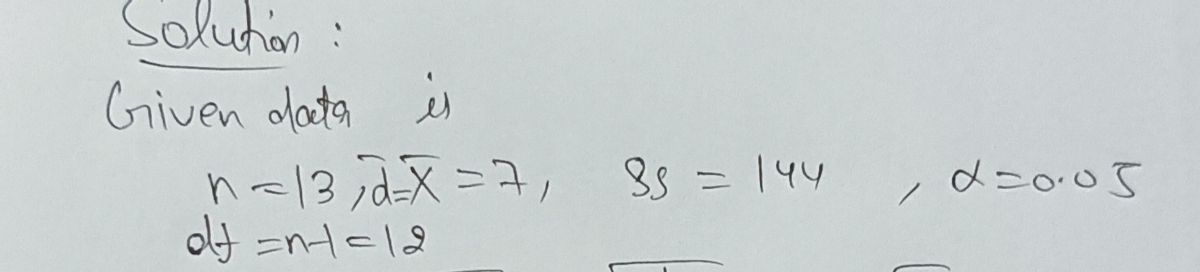
Step by step
Solved in 2 steps with 2 images
Recommended textbooks for you
MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc
Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning
Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning
MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc
Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning
Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning
Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON
The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman
Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman