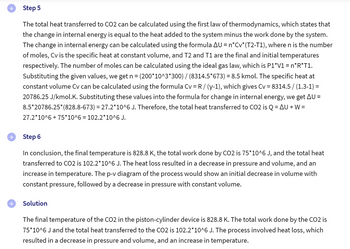
A piston-cylinder device initially contains CO2 at 200 kPa and 400°C and the total enclosed volume is 300 litres. Due to heat loss, the piston moves downwards to a position where it sits on a pair of stoppers and its final enclosed volume is 150 litres. Further heat loss leads to the pressure being reduced to 160kPa. Workout 1) the final temperature, 2) the total work done by CO2 and 3) the total heat transferred to CO2. Also make some comments on the result of heat. 4) Create a schematic p-v diagram of the process The following assumptions are to be made: CO2 is a perfect gas and its molecular mass is 44kg/kmol. The ratio of specific heat capacities, γ (gamma) is 1.3 The volume occupied by the stoppers is to be ignored. There is no leakage in the system There is no friction between the cylinder and piston walls and the piston moved slowly at the same speed therefore, no acceleration. For the conversion from Celsius to Kelvin, use 273 Use 8314.5 J/kmol.K for the universal gas constant
A piston-cylinder device initially contains CO2 at 200 kPa and 400°C and the total enclosed volume is 300 litres. Due to heat loss, the piston moves downwards to a position where it sits on a pair of stoppers and its final enclosed volume is 150 litres. Further heat loss leads to the pressure being reduced to 160kPa.
Workout 1) the final temperature, 2) the total work done by CO2 and 3) the total heat transferred to CO2. Also make some comments on the result of heat.
4) Create a schematic p-v diagram of the process
The following assumptions are to be made:
- CO2 is a perfect gas and its molecular mass is 44kg/kmol.
- The ratio of specific heat capacities, γ (gamma) is 1.3
- The volume occupied by the stoppers is to be ignored.
- There is no leakage in the system
- There is no friction between the cylinder and piston walls and the piston moved slowly at the same speed therefore, no acceleration.
- For the conversion from Celsius to Kelvin, use 273
- Use 8314.5 J/kmol.K for the universal gas constant
Step by step
Solved in 4 steps with 4 images

In the follow up question you have worked out the total work done ON CO2 and total heat transferred FROM CO2 but I asked to workout the total work done BY the CO2 and total heat transferred TO the CO2. Please clarify this, I am having to wait for so long with the answers to follow up questions, please reply quickly.

I asked bartleby to solve this question earlier, it gave me AI answer which is completely different to what's shown in this answer. I don't know which one is correct now. I have attached screenshots of the AI answer. Can you clarify which is correct. Also I previously asked about pv diagram and you said ' the curve in the explanation is correct', did you mean in your explanation or the one I showed as an example? Please advice

The p-v diagram doesn't make sense. Can you double check that it's correct? Does it not have any curves in it as the ones in the image attached?