Homework Help is Here – Start Your Trial Now!
Science
Advanced Physics
4. Show that the canonical ensemble probability 1 P.=ラ follows from maximizing S = -kEp, In p, subject to the constraints Ep, E, =U and Ep, =1 %3D

4. Show that the canonical ensemble probability 1 P.=ラ follows from maximizing S = -kEp, In p, subject to the constraints Ep, E, =U and Ep, =1 %3D

icon
Related questions
Question
4. Show that the canonical ensemble probability 1 P.=ラ follows from maximizing S = -kEp, In p, subject to the constraints Ep, E, =U and Ep, =1 %3D
Transcribed Image Text:4. Show that the canonical ensemble probability 1 P.=ラ follows from maximizing S = -kEp, In p, subject to the constraints Ep, E, =U and Ep, =1 %3D
Expert Solution
Step 1

The canonical partition function is given as:

Advanced Physics homework question answer, step 1, image 1

Step 2

The probability of canonical ensemble be given as:

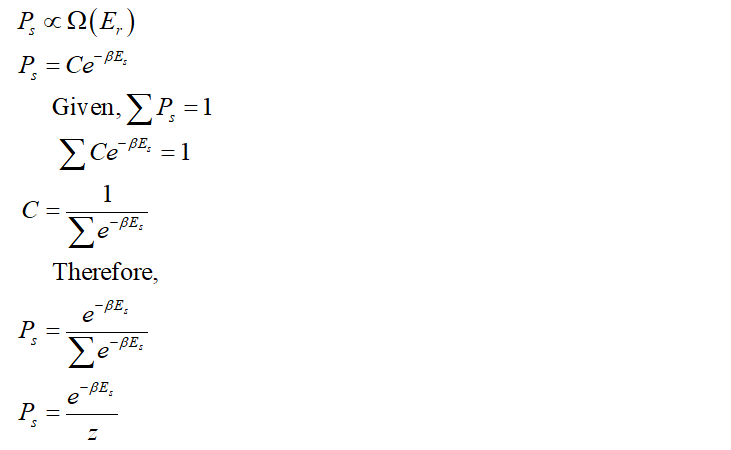
Advanced Physics homework question answer, step 2, image 1

steps

Step by step

Solved in 3 steps with 3 images

Blurred answer