3. The ground state and first excited state of the SHO for angular frequency w are Yo (x) = a exp[-½/2²] ¥₁ (x) = a 2¹/2² ₹ exp [ - ½ ₹² ] where α = (mw/Ãħ)¹/4 and ₹ = (mw/ħ)¹/² x. (a) Construct the second excited state 2 (x) by applying the raising operator. (b) Sketch yo (x), 4₁ (x), and 42 (X). (c) Check their orthogonality. (d) Compute directly and < V> for yo (x) and verify the answers agree with the known total energy E. [T means kinetic energy here].
3. The ground state and first excited state of the SHO for angular frequency w are Yo (x) = a exp[-½/2²] ¥₁ (x) = a 2¹/2² ₹ exp [ - ½ ₹² ] where α = (mw/Ãħ)¹/4 and ₹ = (mw/ħ)¹/² x. (a) Construct the second excited state 2 (x) by applying the raising operator. (b) Sketch yo (x), 4₁ (x), and 42 (X). (c) Check their orthogonality. (d) Compute directly and < V> for yo (x) and verify the answers agree with the known total energy E. [T means kinetic energy here].
Related questions
Question
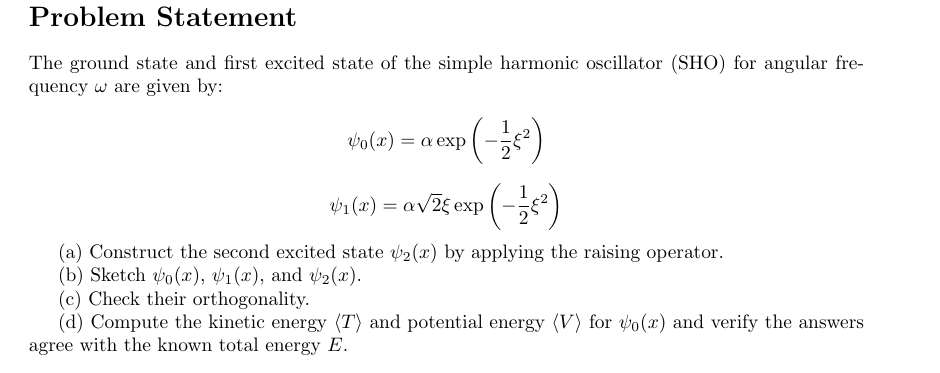
![**3. The ground state and first excited state of the Simple Harmonic Oscillator (SHO) for angular frequency ω are:**
\[ \psi_0(x) = \alpha \exp\left[-\frac{1}{2} \xi^2 \right] \]
\[ \psi_1(x) = \alpha \sqrt{2} \, \xi \exp\left[-\frac{1}{2} \xi^2 \right] \]
where \( \alpha = \left(\frac{m \omega}{\pi \hbar}\right)^{1/4} \) and \( \xi = \left(\frac{m \omega}{\hbar}\right)^{1/2} x \).
(a) Construct the second excited state \( \psi_2(x) \) by applying the raising operator.
(b) Sketch \( \psi_0(x) \), \( \psi_1(x) \), and \( \psi_2(x) \).
(c) Check their orthogonality.
(d) Compute directly \( \langle T \rangle \) and \( \langle V \rangle \) for \( \psi_0(x) \) and verify the answers agree with the known total energy \( E \). [\( T \) means kinetic energy here.]](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F62a79166-ba2c-43d7-9fa1-94c5c5be2063%2F96ccb689-4600-4f46-ac96-72f2379fc628%2Fpj3pexo_processed.png&w=3840&q=75)
Transcribed Image Text:**3. The ground state and first excited state of the Simple Harmonic Oscillator (SHO) for angular frequency ω are:**
\[ \psi_0(x) = \alpha \exp\left[-\frac{1}{2} \xi^2 \right] \]
\[ \psi_1(x) = \alpha \sqrt{2} \, \xi \exp\left[-\frac{1}{2} \xi^2 \right] \]
where \( \alpha = \left(\frac{m \omega}{\pi \hbar}\right)^{1/4} \) and \( \xi = \left(\frac{m \omega}{\hbar}\right)^{1/2} x \).
(a) Construct the second excited state \( \psi_2(x) \) by applying the raising operator.
(b) Sketch \( \psi_0(x) \), \( \psi_1(x) \), and \( \psi_2(x) \).
(c) Check their orthogonality.
(d) Compute directly \( \langle T \rangle \) and \( \langle V \rangle \) for \( \psi_0(x) \) and verify the answers agree with the known total energy \( E \). [\( T \) means kinetic energy here.]
Expert Solution
Step 1: Problem Statement
Step by step
Solved in 6 steps with 13 images
