What are Beams and Frames?

A beam is a horizontal structure or a member which is always subjected to transverse shear loads.

Beams are used in a variety of engineering applications, such as for the construction of buildings, structural applications, automobiles, excavators, and other construction applications.

Beams are generally implemented to carry or resist the lateral loads, that is, the loads that act perpendicular to the axis of the beam and parallel to the cross-section of the beam. Beams provide resistance to bending or deformation due to a property of their cross-section called the moment of inertia.

Construction of Beams and Frames

Construction of beams can be with different materials such as concrete, which is used for construction. Metallic beams are extensively used in structural applications such as for the construction of multi-storied buildings, stadiums, and electrical transmission structures. Beams are also used in mechanical applications for the construction of vehicle frames and vehicle chassis.

There is a difference between a beam and a column, and both should not be confused. A column is a vertical member subjected to compressive loads. Columns are extensively used to resist compressive loads.

Beams are of various kinds based on the loading conditions, and each of them requires a different kind of analysis methodology.

Types of beams

Depending on the loading condition and nature of the structure, beams are classified into three types,

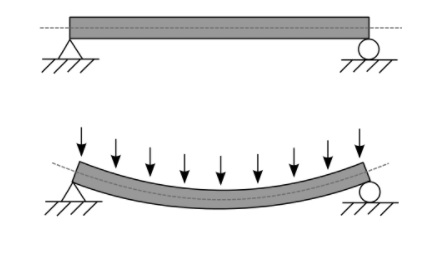
Simply supported beam

A simply supported beam is a horizontal member whose one end remains fixed and the other end is always roller supported. The load acts on the upper part of the beam.

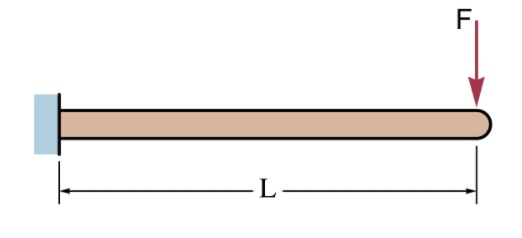
Cantilever beam

A cantilever beam is a horizontal member whose one end always remains fixed and the other remains free. This kind of beam is subjected to transverse shear loads and undergoes deflection.

Overhang beam

Overhang beams are the members whose supports are not situated at the ends of the beam, rather it remains shifted to form the ends. One of the ends or both the ends remain projected outwards.

Analysis of beams

In engineering applications, especially when analyzing structures, the corresponding dimensions of the beam are required to be calculated for the safety factor. In most cases, the unknown loads are required to be calculated.

The following steps are followed while analyzing beams:

1. Draw the free-body diagram of the beam, separate the beam from the structure, and isolate it from all the supports. Include the loads and reactions that are acting on the beam.
2. Calculate the unknown reactions. The unknown reactions are calculated using the three known equilibrium equations. It is generally a numerical approach that requires hand calculations or in complex cases, it is aided by the use of computer programs. The calculations are different for different loading conditions. The principle of superposition is followed during the analysis. The principle of superposition states that, on a linear elastic structure, the combined effects of several load acting simultaneously is the algebraic sum of each loads acting individually.
3. Draw the shear force diagram (SFD) and bending moment diagram (BMD) to get a clear insight into the solution.

Loading conditions on a beam

Based on the nature and application of forces on the beam, there are multiple loading conditions such as

• Shear loads
• Axial loads
• Uniformly distributed loads
• Uniformly varying loads

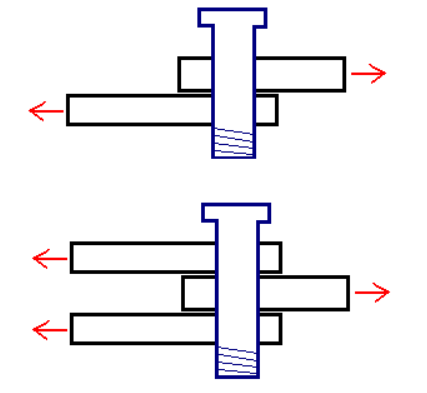
Shear loads

When the forces act perpendicular to the axis of the beam or parallel to the cross-section of the beam, the type of load is known as shear loads.

Axial loads

When the forces on the beam act perpendicular to the cross-section of the beam or parallel to the axis of the beam, the type of load are known as axial loads.

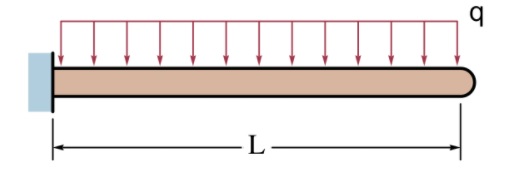
Uniformly distributed load

A uniformly distributed load acts across the whole span or length of the beam.

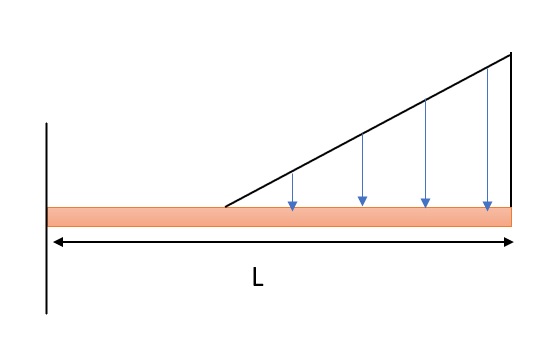
Uniformly varying load

Uniformly varying load acts along the whole length of the beam but varies linearly from one end to the other.

Analysis of frames

A framed structure is of the following three types:

1. Efficient or perfect
2. Deficient or imperfect
3. Redundant

There are certain assumptions to be followed while analyzing framed structures, such as

• All the members are pin jointed.
• The load only acts at the joints or the connection points between the members.
• The frame is perfect, that is, it is made up of structural members and the loads only act at the joints.
• The self-weight of the members is neglected.

The graphical method is usually considered while determining reactions in a beam.

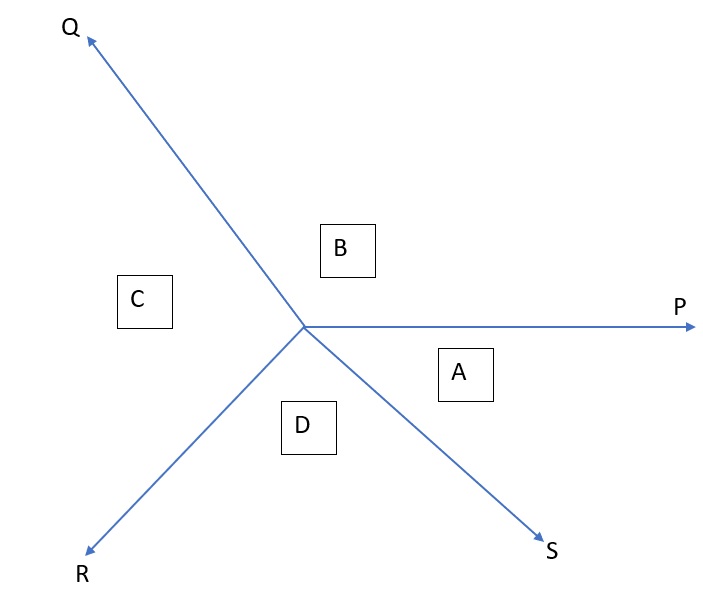
Bow's notation

The bow's notation is a method of designating a force by placing capital letters on either side of it. The only advantage of Bow's notation is that any force in the diagram can be represented by its magnitude and direction to some scale, by simply drawing a line parallel to the force.

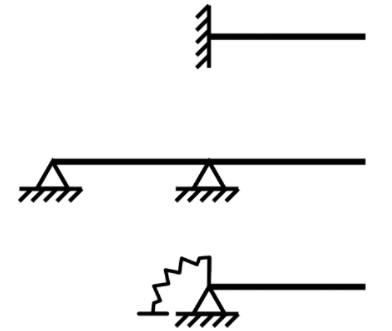
The supports which are usually used in Bow's notation are:

• Simple supports at both ends
• One end is a pin joint while the other is a roller support
• One end is a pin joint while the other is a smooth surface
• Both the joints are fixed

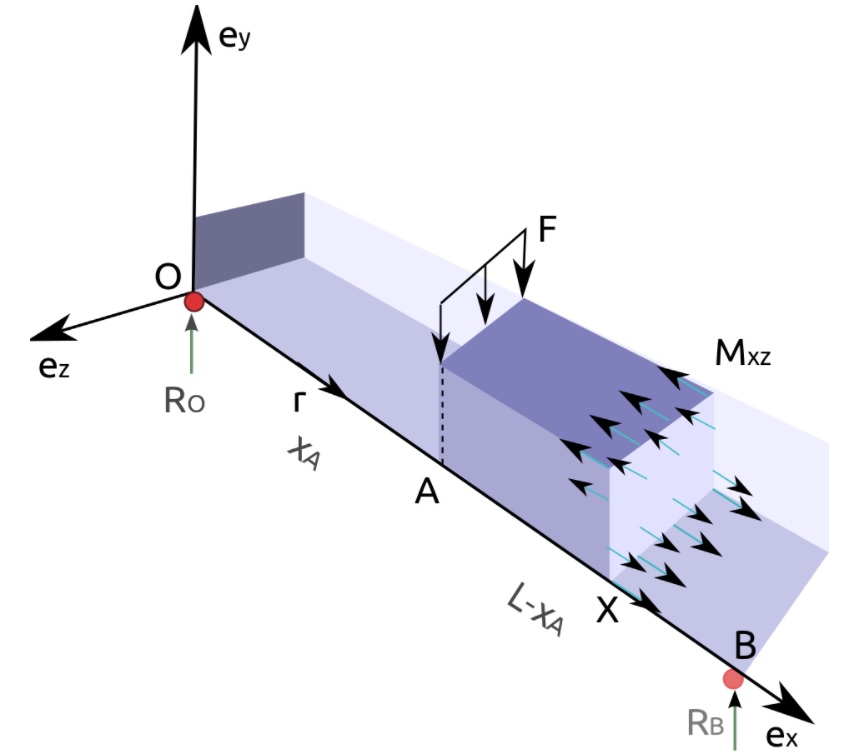
Internal forces in beams and frames

When any beam or frame is subjected to transverse loading condition, the following forces are induced in the member.

• Normal or axial force
• Shearing force
• Bending moment

Bending moment

Bending moment is a reactive moment induced inside the member when any external load tends to bend that member. The bending moment is the product of force and the distance of application of that force from the free or fixed end of the member.

Context and Applications

This topic is significant in the professional exams for both undergraduate and graduate courses, especially for

• Bachelor of science (Physics)
• Master of science (Physics)
• Bachelor in Technology (Mechanical engineering)

Practice Problems

1. Based on the loading conditions, which of the following options is true for a beam?

1. A beam is a member which is acted upon by a transverse load.
2. A beam is a member which is acted upon by a compressive load.
3. A beam is a member which is acted upon by both transverse and compressive load.
4. All of these

Correct option- a

Explanation: A beam is a structural member which is always acted upon by transverse shear loads.

2. What are the internal forces that are induced, when a beam or a frame is subjected to shear loads?

1. Bending moment only
2. Bending moment and shear forces
3. Shear forces only
4. Bending moment, axial forces, and shear forces

Correct option- d

Explanation: When a beam or a frame is acted upon by a shear force, internal forces consisting of a bending moment, shear forces, and axial forces are induced in it.

3. Which of the following defines a frame?

1. A frame is a single structural member which is only accompanied by shear forces acting anywhere in the member.
2. A frame is a combination of structural members joint together, where the load acts only on the joints.
3. A frame is a combination of structural members joint together, where the load acts on the joins and anywhere along with the members.
4. None of these

Correct option- b

Explanation: Frame is a structural member or a combination of different members which are pin joined together. The load on the frame always acts on the joints.

4. A heavy machinery needs to be fabricated on the floor of a workshop. The floor of the workshop often remains wet due to the water spillage because of the operations performed by other machinery. The machinery needs to be elevated from the floor to avoid corrosion of the components. Which of the following member would you choose for building the supports?

1. A column
2. A beam
3. A frame
4. A overhang beam

Correct option- a

Explanation: A column is a vertical member that is subjected to axial compressive loads. Columns are used where there is a need for resisting compressive loads. By using a column, the weight of the machinery can be easily supported.

5. Which of the following is true for an overhanging beam?

1. The supports are away from the ends and one or both the ends remain projected outwards.
2. The beam is supported by roller supports.
3. One end of the beam is fixed and the other is free.
4. Both the ends of the beam are fixed.

Correct option- a

Explanation: For an overhanging beam, the supports are away from its ends, and one of the ends or both ends remains projected outwards from its supports.