
Single Variable Calculus: Concepts and Contexts, Enhanced Edition
4th Edition
ISBN: 9781337687805
Author: James Stewart
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Question
Chapter H.1, Problem 36E
To determine
To find: the curve with the given polar equation
Expert Solution & Answer
Answer to Problem 36E
Thefollowing graph is drawn.
Explanation of Solution
Given:
Calculation:
Consider the following polar curve:
The objective is to sketch the curve with the given polar equation.
Find the values of r for some convenient values of
Compute the following table:
Sketch the following graph:
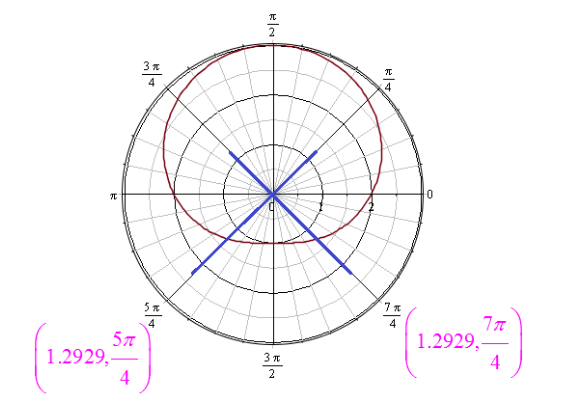
Conclusion:
Therefore, the graph is drawn.
Chapter H.1 Solutions
Single Variable Calculus: Concepts and Contexts, Enhanced Edition
Ch. H.1 - Prob. 1ECh. H.1 - Prob. 2ECh. H.1 - Prob. 3ECh. H.1 - Prob. 4ECh. H.1 - Prob. 5ECh. H.1 - Prob. 6ECh. H.1 - Prob. 7ECh. H.1 - Prob. 8ECh. H.1 - Prob. 9ECh. H.1 - Prob. 10E
Ch. H.1 - Prob. 11ECh. H.1 - Prob. 12ECh. H.1 - Prob. 13ECh. H.1 - Prob. 14ECh. H.1 - Prob. 15ECh. H.1 - Prob. 16ECh. H.1 - Prob. 17ECh. H.1 - Prob. 18ECh. H.1 - Prob. 19ECh. H.1 - Prob. 20ECh. H.1 - Prob. 21ECh. H.1 - Prob. 22ECh. H.1 - Prob. 23ECh. H.1 - Prob. 24ECh. H.1 - Prob. 25ECh. H.1 - Prob. 26ECh. H.1 - Prob. 27ECh. H.1 - Prob. 28ECh. H.1 - Prob. 29ECh. H.1 - Prob. 30ECh. H.1 - Prob. 31ECh. H.1 - Prob. 32ECh. H.1 - Prob. 33ECh. H.1 - Prob. 34ECh. H.1 - Prob. 35ECh. H.1 - Prob. 36ECh. H.1 - Prob. 37ECh. H.1 - Prob. 38ECh. H.1 - Prob. 39ECh. H.1 - Prob. 40ECh. H.1 - Prob. 41ECh. H.1 - Prob. 42ECh. H.1 - Prob. 43ECh. H.1 - Prob. 44ECh. H.1 - Prob. 45ECh. H.1 - Prob. 46ECh. H.1 - Prob. 48ECh. H.1 - Prob. 49ECh. H.1 - Prob. 50ECh. H.1 - Prob. 51ECh. H.1 - Prob. 52ECh. H.1 - Prob. 53ECh. H.1 - Prob. 54ECh. H.1 - Prob. 55ECh. H.1 - Prob. 56ECh. H.1 - Prob. 57ECh. H.1 - Prob. 58ECh. H.1 - Prob. 59ECh. H.1 - Prob. 60ECh. H.1 - Prob. 61ECh. H.1 - Prob. 62ECh. H.1 - Prob. 63ECh. H.1 - Prob. 64ECh. H.1 - Prob. 65ECh. H.1 - Prob. 66ECh. H.1 - Prob. 67ECh. H.1 - Prob. 69ECh. H.1 - Prob. 70E
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- Can you help explain what I did based on partial fractions decomposition?arrow_forwardSuppose that a particle moves along a straight line with velocity v (t) = 62t, where 0 < t <3 (v(t) in meters per second, t in seconds). Find the displacement d (t) at time t and the displacement up to t = 3. d(t) ds = ["v (s) da = { The displacement up to t = 3 is d(3)- meters.arrow_forwardLet f (x) = x², a 3, and b = = 4. Answer exactly. a. Find the average value fave of f between a and b. fave b. Find a point c where f (c) = fave. Enter only one of the possible values for c. c=arrow_forward
- please do Q3arrow_forwardUse the properties of logarithms, given that In(2) = 0.6931 and In(3) = 1.0986, to approximate the logarithm. Use a calculator to confirm your approximations. (Round your answers to four decimal places.) (a) In(0.75) (b) In(24) (c) In(18) 1 (d) In ≈ 2 72arrow_forwardFind the indefinite integral. (Remember the constant of integration.) √tan(8x) tan(8x) sec²(8x) dxarrow_forward
- Find the indefinite integral by making a change of variables. (Remember the constant of integration.) √(x+4) 4)√6-x dxarrow_forwarda -> f(x) = f(x) = [x] show that whether f is continuous function or not(by using theorem) Muslim_mathsarrow_forwardUse Green's Theorem to evaluate F. dr, where F = (√+4y, 2x + √√) and C consists of the arc of the curve y = 4x - x² from (0,0) to (4,0) and the line segment from (4,0) to (0,0).arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning

Calculus: Early Transcendentals
Calculus
ISBN:9781285741550
Author:James Stewart
Publisher:Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:9780134438986
Author:Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:9780134763644
Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:9781319050740
Author:Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:W. H. Freeman


Calculus: Early Transcendental Functions
Calculus
ISBN:9781337552516
Author:Ron Larson, Bruce H. Edwards
Publisher:Cengage Learning
Chain Rule dy:dx = dy:du*du:dx; Author: Robert Cappetta;https://www.youtube.com/watch?v=IUYniALwbHs;License: Standard YouTube License, CC-BY
CHAIN RULE Part 1; Author: Btech Maths Hub;https://www.youtube.com/watch?v=TIAw6AJ_5Po;License: Standard YouTube License, CC-BY