
Concept explainers
In Exercises 1-5, find a logic statement corresponding to the network. Determine the conditions under which current will flow from
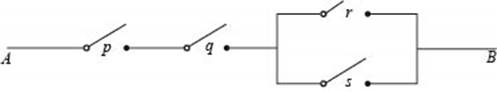
To find:
The logic statement corresponding to the network and write the conditions under which current can be flow from
Answer to Problem 1E
Solution:
The logic statement corresponding to the network is
Explanation of Solution
Given:
The logic statement is
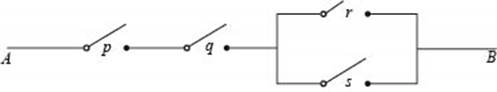
Figure(1)
Approach:
When the two circuits
When the two circuits
Calculation:
The current will pass from point
1.
2.
3. either
Therefore, the logic statement corresponding to the network is
Conclusion:
Hence, the logic statement corresponding to the network is
Want to see more full solutions like this?
Chapter A Solutions
Finite Mathematics for the Managerial, Life, and Social Sciences-Custom Edition
Additional Math Textbook Solutions
Precalculus: A Unit Circle Approach (3rd Edition)
Elementary Statistics: A Step By Step Approach
APPLIED STAT.IN BUS.+ECONOMICS
Elementary Statistics (13th Edition)
Calculus: Early Transcendentals (2nd Edition)
College Algebra (7th Edition)
- a) b) Negate and simplify the following statement in symbolic form. " [(x>0)^(< 0)) → (xy <0)] where x and y are all real numbers" Draw the switching network that represents the statement below: (r^q^p) [p^(qvr)] v [p^q^_r] Varrow_forwardb. Identify the systems from task (2a) that demonstrate closure, i.e., theresult of the operation always produces an element in the set.c. Identify the systems from task (2a) that have an identity element.arrow_forwardRewrite the following statement in the form "v All Java programs have at least 5 lines. X, if then 11arrow_forward
- (DO NOT use any other symbol except numbers in the answer slot ) Trump, Kim, Justin, Modi, Putin and Sefu are at a round table dinner.[The answers of the following questions are integers.Put the answer only.Don’t use space,decimal points,comma etc] Now, It is known that if any two of Trump, Kim and Putin sit beside each other (while sitting in a linear fashion), the dinner may end up in a disaster. In how many ways can they sit so that the dinner may NOT end in a disaster (i.e. how many ways can they sit so that none of Trump, Kim or Putin sits adjacent to each other)?arrow_forwardB) Solve the following linear equation using Grammar's rule 12x + 3y = 15 2x - 3y = 13arrow_forwardBelow is an axiomatic system on books and shelves. Suppose we have the following axioms: 21. – 30. A1: Every shelf is a collection of books. A2: Any two distinct shelves have one and only one book in common. A3: Every book belongs to two and only two shelves. A4: There are exactly four shelves. a. What is/are the undefined terms in the axiomatic system? b. Construct a model that will satisfy the axiomatic system. c. Prove that there are exactly six books using mathematical language. d. Is the system independent? Justify.arrow_forward
- Please do not give solution in image formate thanku. Items coming off an assembly line are inspected sequentially by two inspectors and declared to be defective (D) or non-defective (N). The two inspectors work in different rooms and cannot communicate with one another. State, with reasons, whether you think that the pairs of decisions made by the two inspectors on each item are dependent or independent. Please state the reasoning in a clear and concise way. HINT: Beware of giving "the obvious" answer! Think of the problem this way, by interpreting probability roughly as a proportion or relative frequency. Imagine that all the items that are declared defective by Inspector1 are put in a separate pile. Now imagine that all the items (all of them!) are put in a pile. Compare the proportion of items the Inspector2 declares defective from the first pile with the proportion of items that Inspector2 declares defective from the second pile. Relate your conclusion to the basic definition of…arrow_forwardExercise 7| We place ourselves on a language with a constant me (the person speaking) and three binary relations: friend(x, y) if x is the friend of y enemy(x, y) if x is the enemy ofy (x is my enemy then it corresponds to enemy(x, me)) wwwa - x = y if x and y are equal 1. Translate the following formulas into English: a) Vx, -friend (x, me) = enemy(x, me) b) 3x, Vy, friend(y, x) с) x, Эу, еnemy(y, х) w wmanarrow_forwardConstruct a closure table for the switching network. P Q [~P (NQ v P)] 1 1 ? v ? v ? v ? v 1 0 ? v ? v ? v ? v 0 1 ? v ? v ? v ? v ? v 0 0 ? v ? v ? v ? v ? v Use the closure table to determine the required conditions for the network to be closed. The network is closed if P and Q are both open. The network is closed if P is open and Q is closed. The network is closed if P is closed and Q is open. The network is always closed. O The network is never closed. Submit Answerarrow_forward
- How do the concepts of duality and the golden rectangle relate (Write at least 7 sentences)? Give at least two examplesarrow_forwardIn Exercises 6-10, complete each statement about the diagram. Then state the definition, postulate, or theorem that justifies your answer.arrow_forwardPls do ASAP ... I WILL GIVE LIKE FOR SURE Try to give solution in typed formarrow_forward
 Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elements Of Modern AlgebraAlgebraISBN:9781285463230Author:Gilbert, Linda, JimmiePublisher:Cengage Learning,
Elements Of Modern AlgebraAlgebraISBN:9781285463230Author:Gilbert, Linda, JimmiePublisher:Cengage Learning,


