
| In Exercises 1-6, do the following: | |
| a. | Determine the number of vertices edges, and loops in the given graph |
| b. | Draw another representation of the graph that looks significantly different. |
| c. | Find two adjacent edges. |
| d. | Find two adjacent vertices |
| e. | Find the degree of each vertex. |
| f. | Determine whether the graph is connected. |
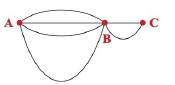 |
|
a)
The number of vertices, edges, and loops in the given graph.
Answer to Problem 1CR
Solution:
The number of vertices are
Explanation of Solution
Given:
The following figure shows the given diagram.
Figure
Approach:
A graph is the diagram that consists of points, known as vertices, and connecting lines are known as edges. The edge that connects a vertex with itself is called a loop.
Therefore, the number of vertices are
Conclusion:
Hence, the number of vertices are
b)
To draw:
The representation of the graph that looks significantly different.
Answer to Problem 1CR
Solution:
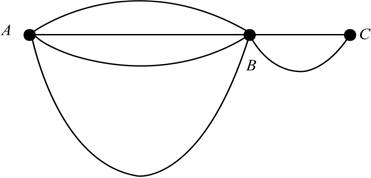
The representation of the graph that looks significantly different is shown in figure
Explanation of Solution
Approach:
The significantly different representation is as follows,
Take three vertices as A, B and C. Connect A and B with two edges, A and C with two edges. Make an edge between B and C. To form a loop make an edge that connects the vertex B with each other as shown below.
Figure
Therefore, the representation of the graph that looks significantly different is shown in figure
Conclusion:
Hence, the representation of the graph that looks significantly different is shown in figure
c)
To find:
The two adjacent edges of the given figure.
Answer to Problem 1CR
Solution:
The adjacent sides are AB, BC.
Explanation of Solution
Approach:
Adjacent edges are the edges which have a common vertex.
In the above given figure the adjacent sides are AB, BC.
Therefore, the adjacent sides are AB, BC.
Conclusion:
Hence, the adjacent sides are AB, BC.
d)
To find:
The two adjacent vertices.
Answer to Problem 1CR
Solution:
The vertices A and B, B and C are two adjacent vertices.
Explanation of Solution
Approach:
Two vertices will be adjacent if they are joined by an edge.
Thus in the above given figure Vertices A and B, B and C are two adjacent vertices.
Therefore, the vertices A and B, B and C are two adjacent vertices.
Conclusion:
Hence, the vertices A and B, B and C are two adjacent vertices.
e)
To find:
The degree of each vertex.
Answer to Problem 1CR
Solution:
The degree of each vertex is shown in the table (1).
Explanation of Solution
Approach:
The degree of vertex is defined as the total number of edges that are connected to it.
The degree of vertex is shown in the following table.
| Vertex | Degree |
| A | |
| B | |
| C |
Table (1)
Therefore, the degree of each vertex is shown in the table (1).
Conclusion:
Hence, the degree of each vertex is shown in the table (1).
f)
Whether the graph is connected or not.
Answer to Problem 1CR
Solution:
The given graph is said to be connected.
Explanation of Solution
Approach:
A graph will be connected if every pair of vertices in the graph is connected by a trail. A trail is sequence of adjacent vertices and the distinct edges that connect to them. Consider the above graph. There is a trail of
Therefore, the given graph is said to be connected.
Conclusion:
Hence, the given graph is said to be connected.
Want to see more full solutions like this?
Chapter 9 Solutions
Mathematics: A Practical Odyssey
- Spam filters are built on principles similar to those used in logistic regression. We fit a probability that each message is spam or not spam. We have several variables for each email. Here are a few: to_multiple=1 if there are multiple recipients, winner=1 if the word 'winner' appears in the subject line, format=1 if the email is poorly formatted, re_subj=1 if "re" appears in the subject line. A logistic model was fit to a dataset with the following output: Estimate SE Z Pr(>|Z|) (Intercept) -0.8161 0.086 -9.4895 0 to_multiple -2.5651 0.3052 -8.4047 0 winner 1.5801 0.3156 5.0067 0 format -0.1528 0.1136 -1.3451 0.1786 re_subj -2.8401 0.363 -7.824 0 (a) Write down the model using the coefficients from the model fit.log_odds(spam) = -0.8161 + -2.5651 + to_multiple + 1.5801 winner + -0.1528 format + -2.8401 re_subj(b) Suppose we have an observation where to_multiple=0, winner=1, format=0, and re_subj=0. What is the predicted probability that this message is spam?…arrow_forwardConsider an event X comprised of three outcomes whose probabilities are 9/18, 1/18,and 6/18. Compute the probability of the complement of the event. Question content area bottom Part 1 A.1/2 B.2/18 C.16/18 D.16/3arrow_forwardI need help making sure that I explain this part accutartly.arrow_forward
- Please help me with this question as I want to know how can I perform the partial fraction decompostion on this alebgric equation to find the time-domain of y(t)arrow_forwardJohn and Mike were offered mints. What is the probability that at least John or Mike would respond favorably? (Hint: Use the classical definition.) Question content area bottom Part 1 A.1/2 B.3/4 C.1/8 D.3/8arrow_forwardPlease help me with this question as I want to know how can I perform the partial fraction on this alebgric equation to find the time-domain of y(t)arrow_forward
- Evaluate F³ - dr where ♬ = (4z, -4y, x), and C' is given by (t) = (sin(t), t, cos(t)), 0≤t≤ñ .arrow_forwardThe details of the clock sales at a supermarket for the past 6 weeks are shown in the table below. The time series appears to be relatively stable, without trend, seasonal, or cyclical effects. The simple moving average value of k is set at 2. What is the simple moving average root mean square error? Round to two decimal places. Week Units sold 1 88 2 44 3 54 4 65 5 72 6 85 Question content area bottom Part 1 A. 207.13 B. 20.12 C. 14.39 D. 0.21arrow_forward5:00 PM Sat May 3 deltamath.com DeltaMath Given: ABBC and D is the midpoint of AC. Prove: ABD ≈ ACBD. ← Back to Home Deltamath Regents Review Week 3 Due: May 9 at 8:00 PM Grade: 97% Step Statement AB ≈ BC Reason 1 Given D is the midpoint of AC 2 BD BD 3 ADDC Calculating Volume (Mixed) Volume of Oblique Solids Volume, Density, and Unit 5 4 AABC is an isosceles triangle ZAZC Conversions (Level 1) Triangle Congruence Criteria try ZAD =/ DC Basic Triangle Proofs (Congruence Only - No CPCTC) Triangle Proofs (Reasons Only) Calculator Aseret Martinez Domi... Log Out Reflexive Property A midpoint divides a segment into two congruent segments The triangle has two congruent sides In a triangle, angles opposite of congruent sides are congruent An angle bisector divides an angle into two congruent angles B * A Ꭰ Note: the segment AC is a straight segment. 86%arrow_forward
- Evaluate the following expression and show your work to support your calculations. a). 6! b). 4! 3!0! 7! c). 5!2! d). 5!2! e). n! (n - 1)!arrow_forwardLANDMARKS Stonehenge is a British landmark made of huge stones arranged in a circular pattern that reflects the movements of Earth and the moon. The diagram shows that the angle formed by the north/south axis and the line aligned from the station stone to the northmost moonrise position measures 23.5°. a. Find measure of arc BC. b. Is arc ABC semicircle? Explain. c. If the circle measures about 100 feet across, approximately how far would you walk around the circle from point B to point sarsen circle B station stone trilithons horseshoe 71° 23.5° farthest north moonrise Sarrow_forwardMid-Term Review Find the formula for (f + g)(x). f(x) = x² - 10x + 25 and g(x) = x² - 10x + 24 (f + g) (x) = [ 2 ]x² X + DELL Skip Sarrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,  College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning



