
(a)
The coordinates in table form for the holes by using absolute programming.
Explanation of Solution
Given:
The length A is
The length B is
The length C is
The length D is
The length E is
The length F is
The length G is
The length H is
The length J is
The length K is
The length L is
The length M is
The length N is
Pitch diameter of circular pattern with holes is
Angle 1 is
Concept Used:
Draw the diagram for the location of holes as shown below:
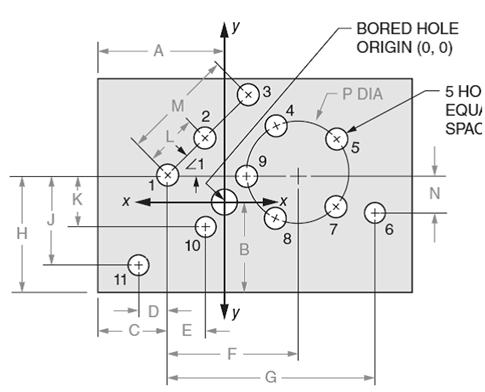
Write the expression for the location of first hole in absolute programming.
Here,
Write the expression for the location of second hole in absolute programming.
Here,
Write the expression for the location of third hole in absolute programming.
Here,
Write the expression for the location of fourth hole in absolute programming.
Here,
Write the expression for the location of fifth hole in absolute programming.
Here,
Write the expression for the location of sixth hole in absolute programming.
Here,
Write the expression for the location of seventh hole in absolute programming.
Here,
Write the expression for the location of eighth hole in absolute programming.
Here,
Write the expression for the location of ninth hole in absolute programming.
Here,
Write the expression for the location of tenth hole in absolute programming.
Here,
Write the expression for the location of eleventh hole in absolute programming.
Here,
Calculation:
Substitute
The coordinates of first hole in absolute programming is
Substitute
The coordinates of second hole in absolute programming is
Substitute
The coordinates of third hole in absolute programming is
Substitute
The coordinates of fourth hole in absolute programming is
Substitute
The coordinates of fifth hole in absolute programming is
Substitute
The coordinates of sixth hole in absolute programming is
Substitute
The coordinates of seventh hole in absolute programming is
Substitute
The coordinates of eighth hole in absolute programming is
Substitute
The coordinates of ninth hole in absolute programming is
Substitute
The coordinates of tenth hole in absolute programming is
Substitute
The coordinates of eleventh hole in absolute programming is
Conclusion:
The coordinate of each hole is shown in below table:
| Hole | X-coordinate (in mm) | Y-coordinate (in mm) |
| 1 | | |
| 2 | ||
| 3 | ||
| 4 | ||
| 5 | ||
| 6 | ||
| 7 | | |
| 8 | ||
| 9 | ||
| 10 | ||
| 11 |
(b)
The coordinates in table form for the holes by using incremental programming.
Explanation of Solution
Concept Used:
Write the expression for the location of first hole in incremental programming.
Here,
Write the expression for the location of second hole in incremental programming.
Here,
Write the expression for the location of third hole in incremental programming.
Here,
Write the expression for the location of fourth hole in incremental programming.
Here,
Write the expression for the location of fifth hole in incremental programming.
Here,
Write the expression for the location of sixth hole in incremental programming.
Here,
Write the expression for the location of seventh hole in incremental programming.
Here,
Write the expression for the location of eighth hole in incremental programming.
Here,
Write the expression for the location of ninth hole in incremental programming.
Here,
Write the expression for the location of tenth hole in incremental programming.
Here,
Write the expression for the location of eleventh hole in incremental programming.
Here,
Calculation:
Substitute
The coordinates of first hole in incremental programming is
Substitute
The coordinates of second hole in incremental programming is
Substitute
The coordinates of third hole in incremental programming is
Substitute
The coordinates of fourth hole in incremental programming is
Substitute
The coordinates of fifth hole in incremental programming is
Substitute
The coordinates of sixth hole in incremental programming is
Substitute
The coordinates of seventh hole in incremental programming is
Substitute
The coordinates of eighth hole in incremental programming is
Substitute
The coordinates of ninth hole in incremental programming is
Substitute
The coordinates of tenth hole in incremental programming is
Substitute
The coordinates of eleventh hole in incremental programming is
Conclusion:
The coordinate of each hole is shown in below table:
| Hole | X-coordinate (in mm) | Y-coordinate (in mm) |
| 1 | | |
| 2 | ||
| 3 | ||
| 4 | ||
| 5 | ||
| 6 | ||
| 7 | | |
| 8 | ||
| 9 | ||
| 10 | ||
| 11 |
Want to see more full solutions like this?
Chapter 80 Solutions
EBK MATHEMATICS FOR MACHINE TECHNOLOGY
- [) Hwk 25 → C Hwk 27 - (MA 244-03) (SP25) IN X Answered: [) Hwk 25 4. [-/4 Poir X + https://www.webassign.net/web/Student/Assignment-Responses/submit?dep=36606606&tags=autosave#question3706544_6 3. [-/2.85 Points] DETAILS MY NOTES LARLINALG8 7.1.021. Find the characteristic equation and the eigenvalues (and a basis for each of the corresponding eigenspaces) of the matrix. 2 -2 5 0 3 -2 0-1 2 (a) the characteristic equation (b) the eigenvalues (Enter your answers from smallest to largest.) (1, 2, 13) = ·( ) a basis for each of the corresponding eigenspaces X1 x2 = x3 = Need Help? Read It Watch It SUBMIT ANSWER 4. [-/2.85 Points] DETAILS MY NOTES LARLINALG8 7.1.041. Find the eigenvalues of the triangular or diagonal matrix. (Enter your answers as a comma-separated list.) λ= 1 0 1 045 002 Need Help? Read It ASK YOUR TEACHER PRACTICE ANOTHER ASK YOUR TEACHER PRACTICE ANOTHER illarrow_forward[) Hwk 25 4. [-/4 Points] Hwk 25 - (MA 244-03) (SP25) || X Answered: Homework#7 | bartle X + https://www.webassign.net/web/Student/Assignment-Responses/last?dep=36606604 DETAILS MY NOTES LARLINALG8 6.4.019. Use the matrix P to determine if the matrices A and A' are similar. -1 -1 12 9 '-[ ¯ ¯ ], ^ - [ _—2—2 _ ' ], ^' - [ ˜³ −10] P = 1 2 A = -20-11 A' -3-10 6 4 P-1 = Are they similar? Yes, they are similar. No, they are not similar. Need Help? Read It SUBMIT ANSWER P-1AP = 5. [-/4 Points] DETAILS MY NOTES LARLINALG8 6.4.023. Suppose A is the matrix for T: R³ - → R³ relative to the standard basis. Find the diagonal matrix A' for T relative to the basis B'. A' = -1 -2 0 A = -1 0 0 ' 0 02 B' = {(−1, 1, 0), (2, 1, 0), (0, 0, 1)} ☐☐☐ ↓ ↑ Need Help? Read It Update available →] - restart now ASK YOUR T Sync and save data { Sign In ill ↑ New tab HT New window N New private window +HP ASK YOUR T Bookmarks History Downloads > > HJ Passwords Add-ons and themes HA Print... HP Save page as... HS…arrow_forwardClarification: 1. f doesn’t have REAL roots2. f is a quadratic, so a≠0arrow_forward
- [J) Hwk 25 Hwk 25 - (MA 244-03) (SP25) || X Answered: Homework#7 | bartle X + https://www.webassign.net/web/Student/Assignment-Responses/last?dep=36606604 1. [-/4 Points] DETAILS MY NOTES Find the matrix A' for T relative to the basis B'. LARLINALG8 6.4.003. T: R² → R², T(x, y) = (x + y, 4y), B' = {(−4, 1), (1, −1)} A' = Need Help? Read It Watch It SUBMIT ANSWER 2. [-/4 Points] DETAILS MY NOTES LARLINALG8 6.4.007. Find the matrix A' for T relative to the basis B'. T: R³ → R³, T(x, y, z) = (x, y, z), B' = {(0, 1, 1), (1, 0, 1), (1, 1, 0)} A' = ↓ ↑ Need Help? Read It SUBMIT ANSWER 具⇧ ASK YOUR TEACHER PRACTICE ANOTHER ill ASK YOUR TEACHER PRACTICE ANOTHER 3. [-/4 Points] DETAILS MY NOTES LARLINALG8 6.4.013. ASK YOUR TEACHER PRACTICE ANOTHERarrow_forwardUse Laplace transforms to solve the following heat problem: U₁ = Urr x > 0, t> 0 u(x, 0) = 10c a -X u(0,t) = 0 lim u(x,t) = 0 I7Xarrow_forwarda/ Solve the equation Laplac transfors wt = wxx W (X10)=0 w (o,t) = f (t) lim wexit) = 0 *>° t>o , to t70 848arrow_forward
- / Solve the equation Laplac transfoms:- wt = wxx WCX10) = 0 w (o,t) = f(t) lim w(x,t) = 0 X∞arrow_forwardQ/Find the Fourier sine and cosine transforms of f(x) = Xe-Xarrow_forwardQ/solve the equation Laplac transforms:- x>0, to 43 1 иху u cx,α) = f(x) и a Cort ) = • lim u(x,t) = 0 8700 و X>0 , toarrow_forward
 Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal LittellAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal LittellAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning, Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,





