Videos
Positive-sequence components consist of three phasors _________ with magnitudes and _________ phase displacement in positive sequence; negative- sequence components consist of three phasors with ________ magnitudes and _________ phase displacement in negative sequence; and zero-sequence components consist of three phasors with __________ magnitudes and __________ phase displacement.
To fill: The appropriate words in the given blank spaces.
Answer to Problem 8.1MCQ
The appropriate words are: equal,
Explanation of Solution
The method of symmetrical components is used to solve the unbalanced system. This method is also termed as three-component method. The balanced set of components are positive sequence component, negative sequence component and zero-sequence component.
Positive Sequence components: The positive-sequence system is represented by a balanced system of phasors having the same phase sequence (and therefore positive phase rotation) as the original unbalanced system. The phasors of the positive-sequence system are equal in magnitude and displaced from each other by
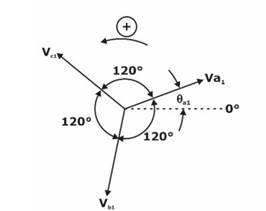
Negative Sequence components: The negative-sequence system is represented by a balanced system of phasors having the opposite phase sequence (and therefore negative phase rotation) to the original system. The phasors of the negative-sequence system are also equal in magnitude and displaced from each other by
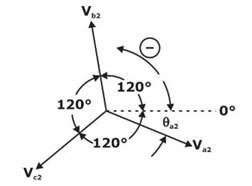
Zero Sequence components: The zero-sequence system is represented by three single phasors that are equal in magnitude and angular displacement between all the phasors is
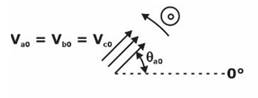
Therefore, in positive-sequence component, there are three phasors of equal magnitude but
Want to see more full solutions like this?
Chapter 8 Solutions
Power System Analysis & Design
- Consider the homogeneous RLC circuit (no voltage source) shown in the diagram below. Before the switch is closed, the capacitor has an initial charge go and the circuit has an initial current go. R w i(t) q(t) C н After the switches closes, current flows through the circuit and the capacitor begins to discharge. The equation that describes the total voltage in the loop comes from Kirchoff's voltage law: di(t) L + Ri(t) + (t) = 0, dt (1) where i(t) and q(t) are the current and capacitor charge as a function of time, L is the inductance, R is the resistance, and C is the capacitance. Using the fact that the current equals the rate of change of the capacitor charge, and dividing by L, we can write the following homogeneous (no input source) differential equation for the charge on the capacitor: ä(t)+2ag(t)+wg(t) = 0, (2) where R a 2L and w₁ = C LC The solution to this second order linear differential equation can be written as: where 81= q(t) = Ae³¹- Bel 82 = (3) (4) (5)arrow_forward2. 1. A. Simplify the models in the following block diagrams to open loop models (Y/R = G). U(s) o G₁ ROS G₂ 1-GG G4 X₁ Σ az 51- 515 G6 G₂ 5 G₂ M b₁ b₂ Σ o Y(s) X₁ byarrow_forwardNeed handwritten solution do not use chatgpt or AIarrow_forward
- B. Design a 2nd order Band Stop Filter (BSF) with overall gain=10, centre frequency-12kHz, and bandwidth=4KHz. (8 Marks)arrow_forwardDesign a fifth (5th) order HPF with 8 KHz cutoff frequency, and overall gain Av=35.57dB. Calculate the roll-off rate and draw its frequency response.arrow_forwardThe reverse recovery charge and the peak reverse current are QH-500 uC and I-250A respectively. Assume that the softness factor is SF=0.5, estimate (a) The reverse recovery time of the diode trr (b) The rate of fall of the diode current di/dtarrow_forward
- Q2: A 208V, Y-connected synchronous motor is drawing 40A at unity power factor from a 208V power system. The field current flowing under these conditions is 2.7A. Its synchronous reactance is 0.82 and its armature resistance is 0.2 2. Assume a linear open-circuit characteristic. 1- Find EA and the torque angle. 2- How much field current would be required to make the motor operate at 0.8 PF lagging. 3- How much field current would be required to make the motor operate at 0.8 PF leading. 4- How much field current would be required to make the motor operate at unity PF.arrow_forward6) For each case find the answer: 2 (a) If q (t) = 2+ + 6 + + 3 Coulombs Find i(t) at t = 4 seconds (b) If i(t) = 4 Amperes If Find q (t) for 25 = ≤6 seconds (c) If w(t) = 5t³ Joules Find p(t) at t = 3 seconds (d) If p(t 2t+3+4 Watts Find w(t) for 1st≤5 secondsarrow_forwardAs we will learn in Chapter 8, to maximize the transfer of power from an input circuit to a load ZL, it is necessary to choose ZL such that it is equal to the complex conjugate of the impedance of the input circuit. For the circuit in Fig. P7.50, such a condition translates into requiring ZL = Zth*. Determine ZL such that it satisfies this condition.arrow_forward
- 7.44 In the circuit of Fig. P7.44, what should the value of L be 104 rad/s so that i(t) is in-phase with u,(t)? at i(t) 50 Ω www Ds(f) z- 25 Ω 4μF L b Figure P7.44 Circuit for Problem 7.44.arrow_forward5) An orbiting satellite has both solar panels and a 48-volt battery on board. The instrumentation package has sent the following data regarding supplied Coulombs vs. minutes for the 48 volt battery on the Satellite Coulombs 3.5 3 25 2 15 05 O 0.5 1 1.5 2 2.5 3 3.5 4 4.5 5 5.5 6 6 minutes (a) what is the battery current at t=0-5 minute: (6) How much power is the battery supplying at 0.5 Minutes? (c) Between 2 and 3 minutes how much poner does the battery supply? (d) Between 3 and 4 minutes what current is produced by the battery?arrow_forward7.36 Find the input impedance Z of the circuit in Fig. P7.36 at 0 400 rad/s. 502 3 mH ww m Z→ 2 mF b 5025 ww ell Figure P7.36 Circuit for Problem 7.36. 9 mHarrow_forward
Power System Analysis and Design (MindTap Course ...Electrical EngineeringISBN:9781305632134Author:J. Duncan Glover, Thomas Overbye, Mulukutla S. SarmaPublisher:Cengage Learning