
Concept explainers
Draw the influence lines for the force in member AB, BG, DF, and FG.
Explanation of Solution
Calculation:
Find the support reactions.
Apply 1 k moving load from E to G in the top chord member.
Draw the free body diagram of the member as in Figure 1.
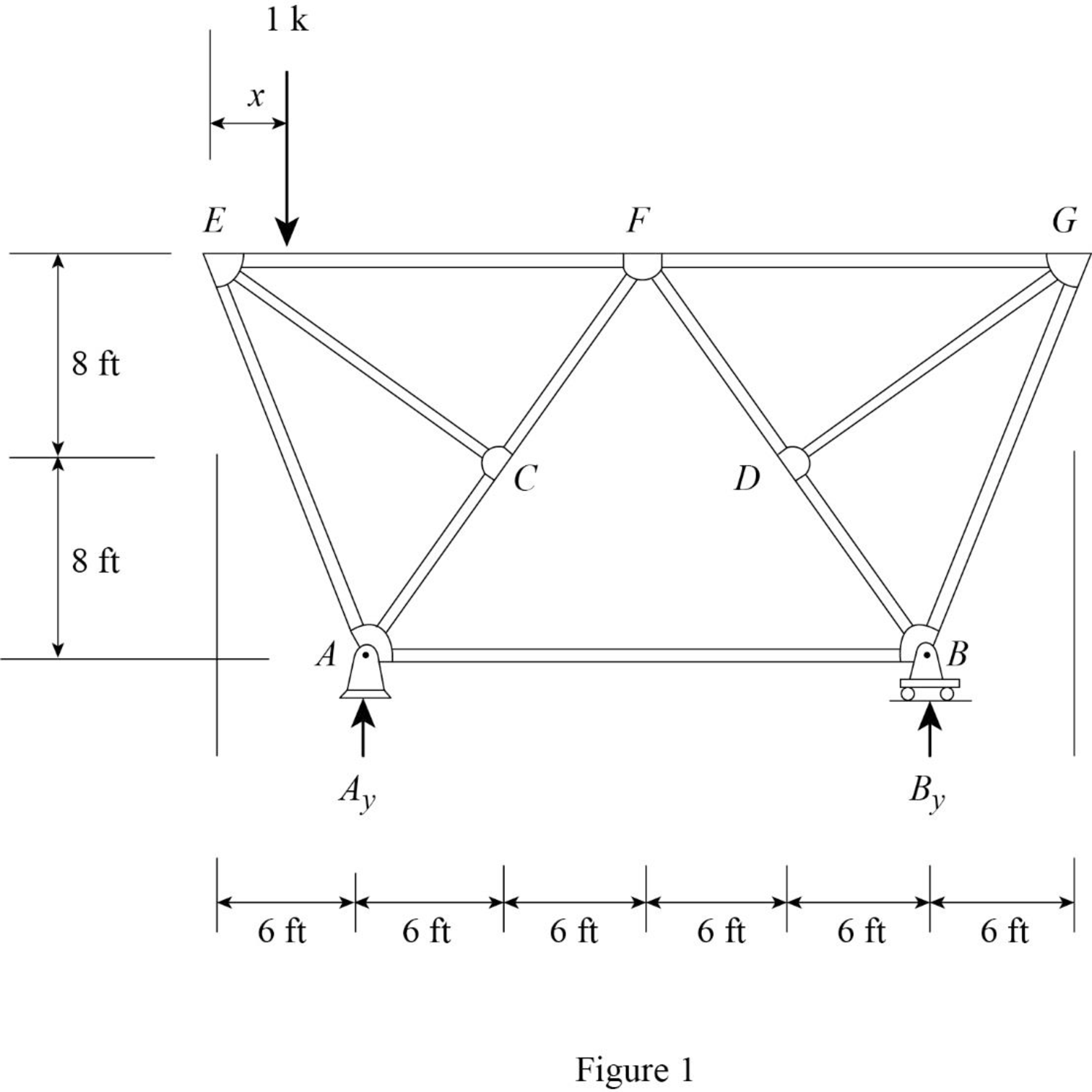
Find the reaction at A and B when 1 k load placed from E to G.
Apply moment equilibrium at A.
Apply force equilibrium equation along vertical.
Consider the upward force as positive
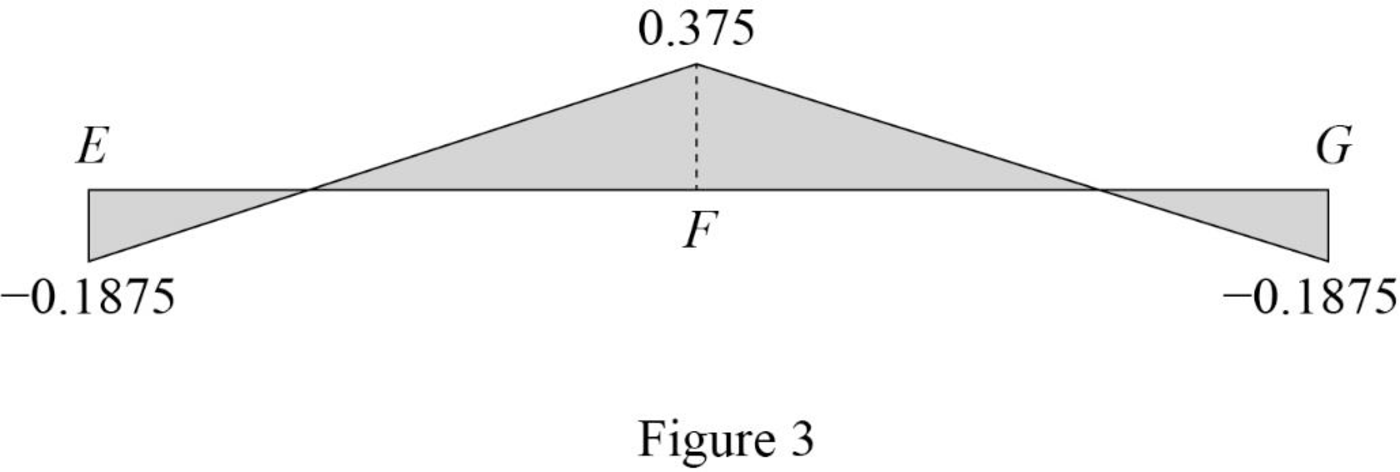
Influence line for the force in member AB.
The expressions for the member force
Draw the free body diagram of member with section aa as shown in Figure 2.
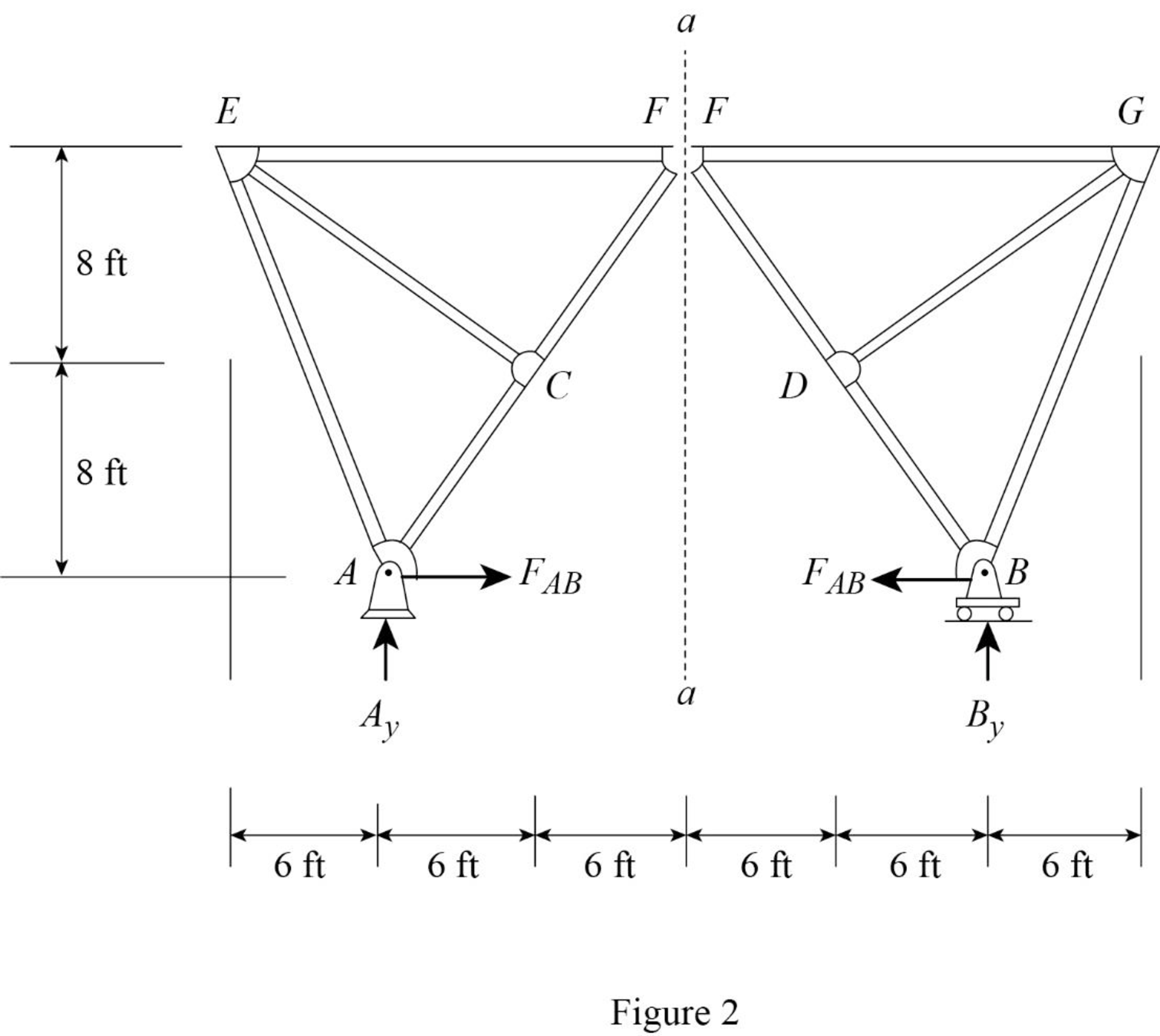
Refer Figure 2.
Find the equation of member force AB.
Apply a 1 k load at just left of F
Consider the right hand portion to section a-a.
Apply moment equilibrium equation at F.
Consider clockwise moment as positive and anticlockwise moment as negative.
Substitute
Apply a 1 k load at just right of F
Consider the left hand portion to section a-a.
Apply moment equilibrium equation at F.
Consider clockwise moment as positive and anticlockwise moment as negative.
Substitute
Thus, the equation of force in the member AB,
Find the force in member AB using the Equation (1) and (2) and then summarize the value in Table 1.
| x (ft) | Apply 1 k load | Force in member AB (k) | Influence line ordinate for the force in member AB (k/k) |
| 0 | E | ||
| 18 | F | 0.375 | |
| 36 | G |
Sketch the influence line diagram for ordinate for the force in member AB using Table 2 as shown in Figure 3.
Influence line for the force in member BG.
The expressions for the member force
Draw the free body diagram of section a-a as shown in Figure 4.
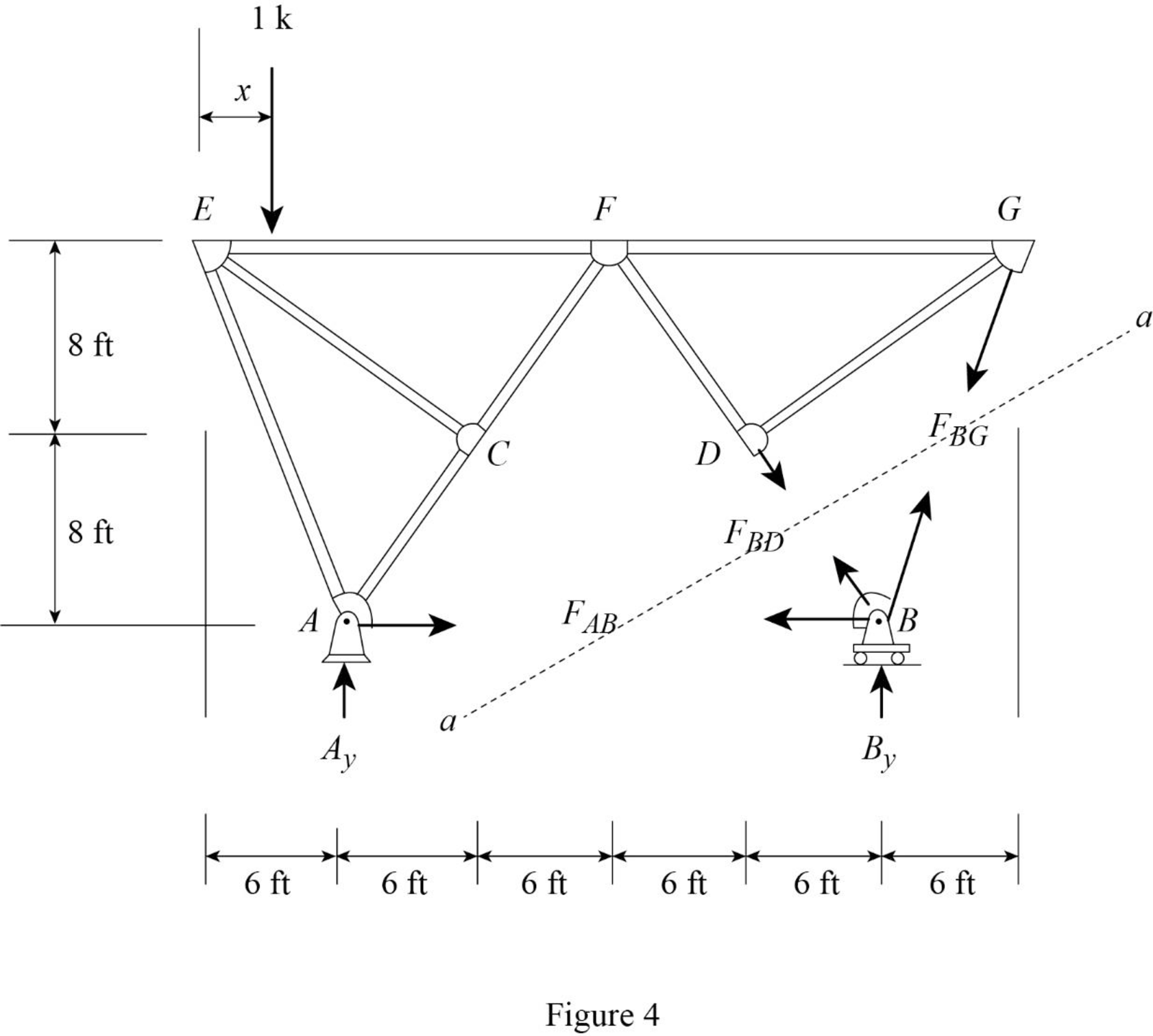
Refer Figure 4.
Find the force in member BG.
Apply 1 k load just left of F
Consider the section EF.
The member force of EF not affected when 1 k load applied from E to F. Therefore, the influence line ordinate of member force BG is 0 k/k from E to F.
Apply a 1 k load just the right of F
Apply moment equilibrium at F.
Consider the section right of line a-a.
Consider clockwise moment as positive and anticlockwise moment as negative.
Substitute
Thus, the equation of force in the member BG,
Find the force in member BG using the Equation (3) and (4) and then summarize the value in Table 2.
| x (ft) | Apply 1 k load | Force in member BG (k) | Influence line ordinate for the force in member BG (k/k) |
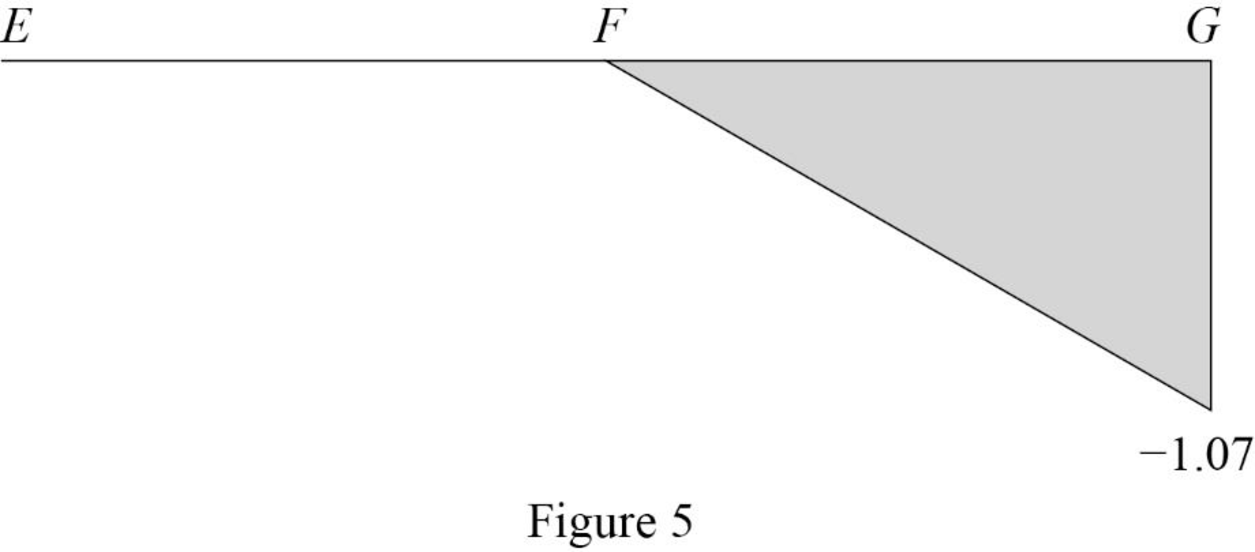
| 0 | E | 0 | |
| 18 | F | 0 | |
| 36 | G | ‑1.07 |
Sketch the influence line diagram for ordinate for the force in member BG using Table 2 as shown in Figure 5.
Influence line for the force in member DF.
The expressions for the member force
Draw the free body diagram of section a-a as shown in Figure 6.
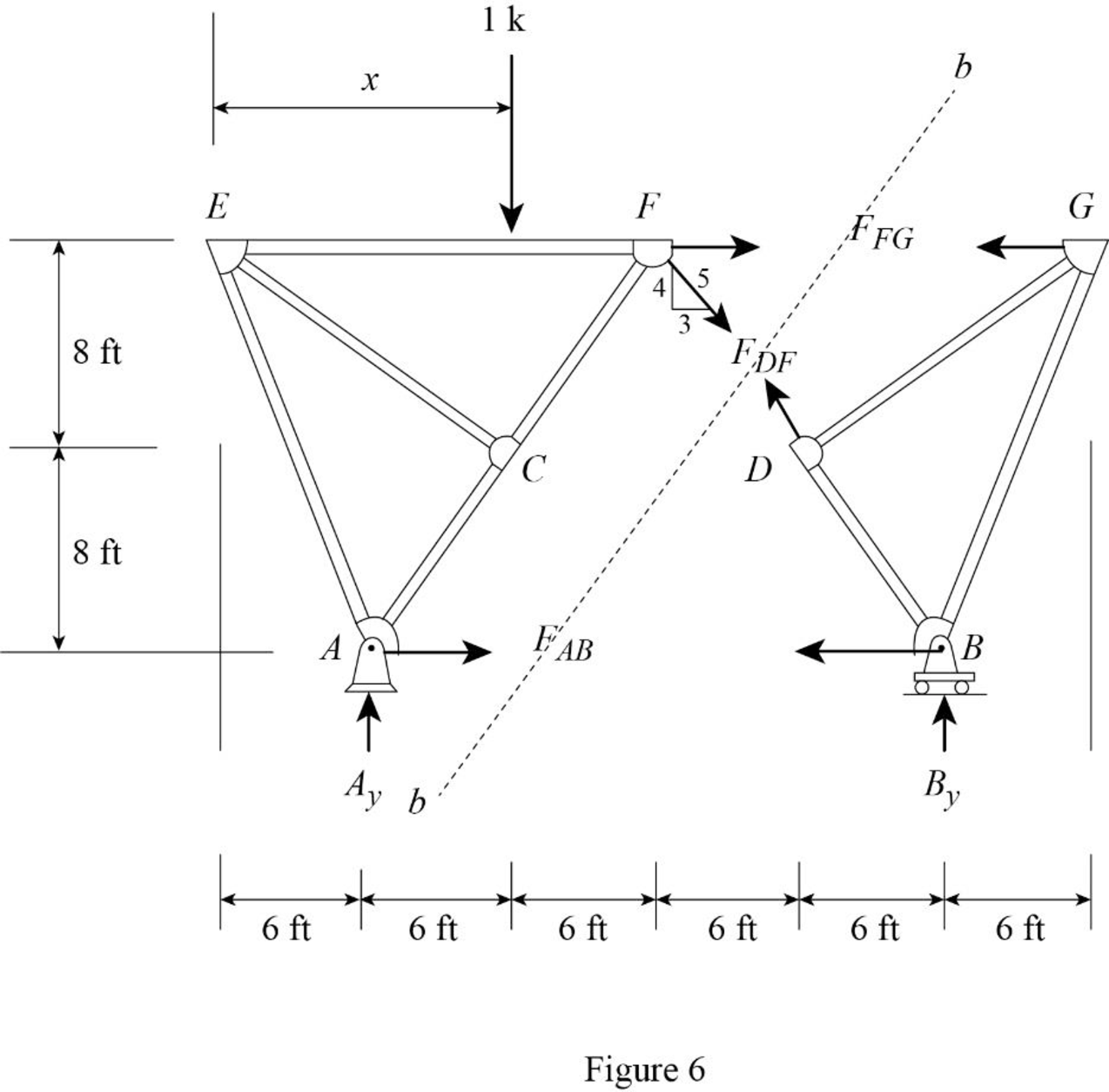
Refer Figure 6.
Find the force in member DF.
Apply 1 k load just left of F
Consider the section right of line bb.
Apply moment equilibrium at G.
Consider clockwise moment as positive and anticlockwise moment as negative.
Substitute
Apply 1 k load just right of F
Consider the section left of line bb.
Apply moment equilibrium at E.
Consider clockwise moment as positive and anticlockwise moment as negative.
Substitute
Thus, the equation of force in the member DF,
Find the force in member DF using the Equation (5) and (6) and then summarize the value in Table 3.
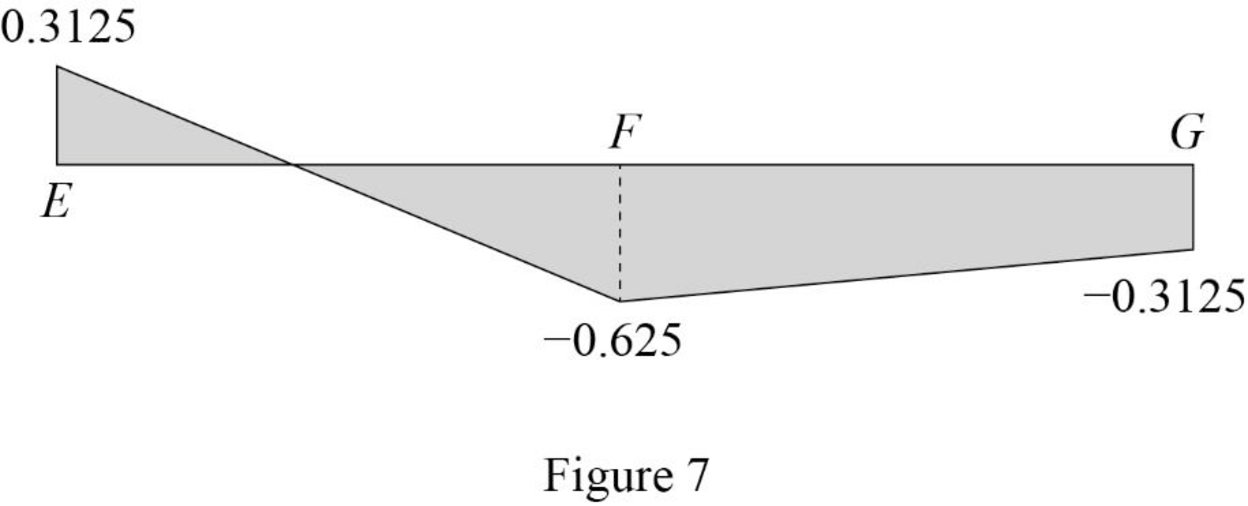
| x (ft) | Apply 1 k load | Force in member DF (k) | Influence line ordinate for the force in member DF (k/k) |
| 0 | E | 0.3125 | |
| 18 | F | ‑0.625 | ‑0.625 |
| 36 | G | ‑0.3125 | ‑0.3125 |
Sketch the influence line diagram for ordinate for the force in member DF using Table 3 as shown in Figure 7.
Influence line for the force in member FG.
Refer Figure 6.
Find the force in member FG.
Apply 1 k load just left of F
Consider the section right of line bb.
Apply moment equilibrium at B.
Consider clockwise moment as positive and anticlockwise moment as negative.
Apply 1 k load just right of F
Consider the section left of line bb.
Apply moment equilibrium at A.
Consider clockwise moment as positive and anticlockwise moment as negative.
Substitute
Thus, the equation of force in the member FG,
Find the force in member FG using the Equation (7) and (8) and then summarize the value in Table 4.
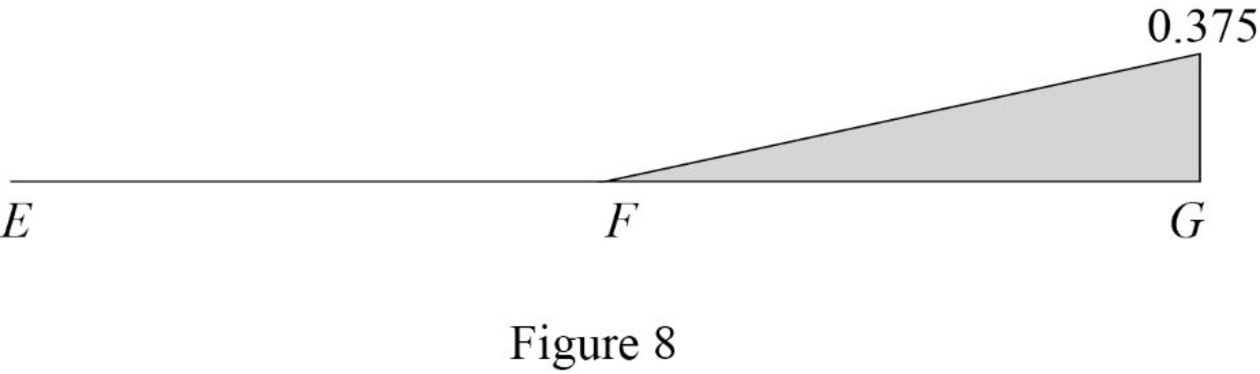
| x (ft) | Apply 1 k load | Force in member FG (k) | Influence line ordinate for the force in member FG (k/k) |
| 0 | E | 0.3125 | |
| 18 | F | ‑0.625 | ‑0.625 |
| 36 | G | ‑0.3125 | ‑0.3125 |
Sketch the influence line diagram for ordinate for the force in member FG using Table 4 as shown in Figure 8.
Want to see more full solutions like this?
Chapter 8 Solutions
Structural Analysis, 5th Edition
- 10.37 What is ffor the flow of water at 10°C through a 30-cm cast iron pipe with a mean velocity of 24 m/s?arrow_forward10.60 As shown, water (15°C) is draining from a tank through a galvanized iron pipe. The pipe length is L = 2 m, the tank depth is H = 1 m, and the pipe is a 0.5-inch NPS schedule 40. Calculate the velocity in the pipe. Neglect component head loss. H Pipe of diameter D L Problems 10.59 and 10.60arrow_forward10.53 Water is pumped through a vertical 10-cm new steel pipe to an elevated tank on the roof of a building. The pressure on the discharge side of the pump is 1.6 MPa. What pressure can be expected at a point in the pipe 110 m above the pump when the flow is 0.02 m³/s? Assume T = 20°C.arrow_forward
- 10.61 A pipeline is to be designed to carry crude oil (SG = 0.93, v = 10-5 m²/s) with a discharge of 0.10 m³/s and a head loss per kilometer of 50 m. What diameter of steel pipe is needed? What power output from a pump is required to maintain this flow? Available pipe diameters are 20, 22, and 24 cm.arrow_forwardCalculate the active earth pressure (exerted by the supported soil mass on the right) against the 10-meter-long, dense and smooth sheet pile wall shown in Figure E2:1. The ground surface is loaded with heavy construction machinery applying a pressure of q = 10.0 kPa. Other data is according to the figure.Assume the sheet pile moves sufficiently to the left to reach active failure conditions behind it, and passive failure conditions develop in the soil mass below the excavation bottom. Will the sheet pile wall hold without rain? (Calculate the forces.) Will the sheet pile wall hold if it rains? (Assume water-filled cracks.) If the sheet pile does not hold in any of the above cases – how deep would it need to be embedded in order to hold? Draw diagrams for active and passive earth pressure as well as the resultant earth pressure. gvy=grownd water levelarrow_forwardThe composite beam shown in the figure is subjected to a bending moment Mz=8 kNmMz=8kNm.The elastic moduli for the different parts are E1=30 GPa, E2=20 GPa, and E3=60GPa. a) Determine the reduced moment of inertia IredIred for the entire beam. b) Sketch the bending stress distribution in the beam.arrow_forward
- USING THE ATTACHED SKETCH , DETERMINE THE FOLLOWING: 1. INVERSE DISTANCE, NORTH AZIMUTH AND BEARING BETWEEN CP-102 AND THE SOUTHWEST BUILDING CORNER.2. DETERMINE THE INTERIOR ANGLE AT CP-101 - CP-102 AND THE SOUTHWEST BUILDING CORNER.3. WHAT ARE THE COORDINATES (N,E) AT POINT A AND POINT B IN THE ATTACHED SKETCH?arrow_forwardGiven the following Right Triangle, find the " Area by Coordinates" (Not B*H/2). Report to the nearest Sq. Ft. and to the nearest thousandth of an acre.arrow_forward1) 4,739,281 SQ.FT. = ______________________ ACRES? 2) S 90°00'00" W IS ALSO KNOW AS WHAT CARDINAL DIRECTION? 3) CALCULATE THE NORTH AZIMUTH (NAZ) OF THE FOLLOWING BEARINGS: N 31° 22' 22" E=___________________________NAZ? S 87° 29' 17" W=___________________________NAZ? S 27° 43' 27" E=___________________________NAZ? N 43° 17' 43" E=___________________________NAZ?arrow_forward
- 1) 187.25597°=_____________________________________(DEG-MIN-SEC FORMAT)? 2) CALCULATE THE BEARING AND DIRECTION IN DEG-MIN-SEC OF THE FOLLOWING: NAZ 142°49'18"=____________________________(BEARING/DIRECTION DEG-MIN-SEC)? NAZ 180°00'00"=____________________________(BEARING/DIRECTION DEG-MIN-SEC)? NAZ 270°00'00"=____________________________(BEARING/DIRECTION DEG-MIN-SEC)?arrow_forwardA traffic signal has a 60-second cycle length (Red time + Green time). For the travel direction of interest, the red and green times are 30 seconds each, the arrival rate is constant at 20 [veh/min] and the saturation flow (i.e., the departure rate) is 1 [veh/sec]. a. Calculate the average delay (for all vehicles) for the travel direction of interest. b. Assume a work zone on the street downstream of the intersection so that only 25 [veh/min] (in the direction of interest) can pass. Calculate the average delay caused by the work zone to a vehicle leaving the intersection. Assume that the queue at the work zone never backs- up into the intersection. c. Discuss qualitatively the implications of queue spillback from the work zone on the delay of the system. Traffic Direction (a) Traffic Direction (b)arrow_forwardCalculate the active earth pressure (exerted by the supported soil mass on the right) against the 10-meter-long, dense and smooth sheet pile wall shown in Figure E2:1. The ground surface is loaded with heavy construction machinery applying a pressure of q = 10.0 kPa. Other data is according to the figure.Assume the sheet pile moves sufficiently to the left to reach active failure conditions behind it, and passive failure conditions develop in the soil mass below the excavation bottom. Draw diagrams for active and passive earth pressure as well as the resultant earth pressure. Questions to Answer: Will the sheet pile wall hold without rain? (Calculate the forces.) Will the sheet pile wall hold if it rains? (Assume water-filled cracks.) If the sheet pile does not hold in any of the above cases – how deep would it need to be embedded in order to hold?arrow_forward
