Chapter 8, Problem 3SR

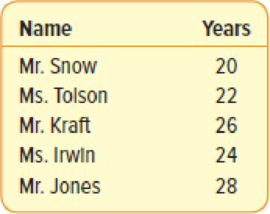
The years of service of the five executives employed by Standard Chemicals are:

1. (a) Using the combination formula, how many samples of size 2 are possible?
2. (b) List all possible samples of two executives from the population and compute their means.
3. (c) Organize the means into a sampling distribution.
4. (d) Compare the population mean and the mean of the sample means.
5. (e) Compare the dispersion in the population with that in the distribution of the sample mean.
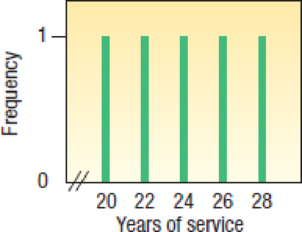
6. (f) A chart portraying the population values follows. Is the distribution of population values normally distributed (bell-shaped)?

1. (g) Is the distribution of the sample mean computed in part (c) starting to show some tendency toward being bell-shaped?

To determine

Find the possible number of samples of size 2 using combination formula.

Answer to Problem 3SR

The possible number of samples of size 2 using combination formula is 10.

Explanation of Solution

From the given information, the years of service of the five executives are 20, 22, 26, 24 and 28.

The possible number of different samples of size two is obtained by using the following formula:

${}_N{C}_n=\frac{N!}{\left(N-n\right)!n!}$

Substitute 5 for the N and 2 for the n

Then,

$\begin{array}{c}{}_5{C}_2=\frac{5!}{\left(5-2\right)!2!}\\ =\frac{5\times 4\times 3!}{3!2!}\\ =\frac{5\times 4}{2\times 1}\\ =10\end{array}$

Thus, the possible number of samples of size 2 using combination formula is 10.

To determine

Give the all possible samples of two executives.

Find the mean of each sample.

Answer to Problem 3SR

Thus, all possible samples of two executives are $\left(20,22\right)$, $\left(20,26\right)$, $\left(20,24\right)$, $\left(20,28\right)$, $\left(22,26\right)$, $\left(22,24\right)$, $\left(22,28\right)$, $\left(26,24\right)$, $\left(26,28\right)$ and $\left(24,28\right)$.

Thus, the mean of each sample is 21, 23, 22, 24, 24, 23, 25, 25, 27 and 26.

Explanation of Solution

The mean is calculated by using the following formula:

$\text{Mean}=\frac{\text{Sum}\text{of}\text{all}\text{the}\text{observations}}{\text{Number}\text{of}\text{observations}}$

Sample	Mean
$\left(20,22\right)$	$\frac{20+22}{2}=21$
$\left(20,26\right)$	$\frac{20+26}{2}=23$
$\left(20,24\right)$	$\frac{20+24}{2}=22$
$\left(20,28\right)$	$\frac{20+28}{2}=24$
$\left(22,26\right)$	$\frac{22+26}{2}=24$
$\left(22,24\right)$	$\frac{22+24}{2}=23$
$\left(22,28\right)$	$\frac{22+28}{2}=25$
$\left(26,24\right)$	$\frac{26+24}{2}=25$
$\left(26,28\right)$	$\frac{26+28}{2}=27$
$\left(24,28\right)$	$\frac{24+28}{2}=26$

Thus, all possible samples of size 2 are $\left(20,22\right)$, $\left(20,26\right)$, $\left(20,24\right)$, $\left(20,28\right)$, $\left(22,26\right)$, $\left(22,24\right)$, $\left(22,28\right)$, $\left(26,24\right)$, $\left(26,28\right)$ and $\left(24,28\right)$.

Thus, the mean of each sample is 21, 23, 22, 24, 24, 23, 25, 25, 27 and 26.

To determine

Construct the sampling distribution for the means.

Answer to Problem 3SR

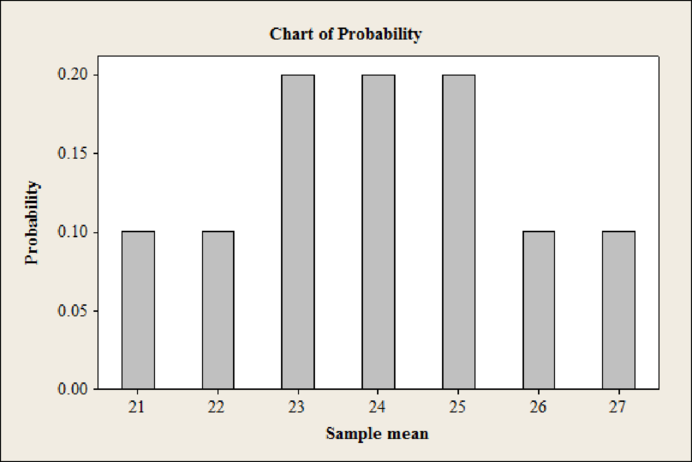
The sampling distribution for the means is

Explanation of Solution

The sampling distribution for the means is as follows:

Sample mean	Frequency	Probability
21	1	$\frac{1}{10}=0.1$
22	1	$\frac{1}{10}=0.1$
23	2	$\frac{2}{10}=0.2$
24	2	$\frac{2}{10}=0.2$
25	2	$\frac{2}{10}=0.2$
26	1	$\frac{1}{10}=0.1$
27	1	$\frac{1}{10}=0.1$
	$N=10$

Software procedure:

Step-by-step procedure to obtain the bar chart using MINITAB:

• Choose Stat > Graph > Bar chart.
• Under Bars represent, enter select Values from a table.
• Under One column of values select Simple.
• Click on OK.
• Under Graph variables enter probability and under categorical variable enter sample mean.
• Click OK.

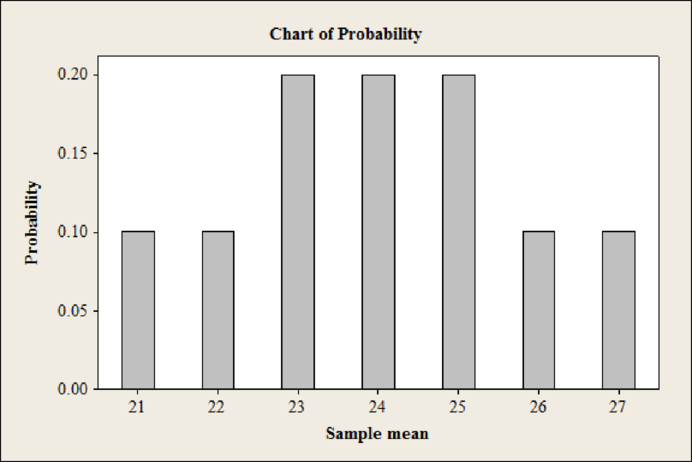
Output using MINITAB software is given below:

To determine

Give the comparison between the population mean and the mean of the sample means.

Answer to Problem 3SR

The mean of the distribution of the sample means is equal to the population mean.

Explanation of Solution

Population mean is calculated as follows:

$\begin{array}{c}\text{Mean}=\frac{\text{Sum}\text{of}\text{the}\text{the years of service of the five executives}}{\text{Number}\text{of}\text{executives}}\\ =\frac{20+22+26+24+28}{5}\\ =\frac{120}{5}\\ =24\end{array}$

The mean of the sample means is calculated as follows:

$\begin{array}{c}\text{Mean}=\frac{\text{Sum}\text{of}\text{all}\text{the}\text{sample}\text{means}}{\text{Number}\text{of}\text{samples}}\\ =\frac{21+22+23+23+24+24+25+25+26+27}{10}\\ =\frac{240}{10}\\ =24\end{array}$

Comparison:

The mean of the distribution of the sample mean is 24 and the population mean is 24. The two means are exactly same.

Thus, the mean of the distribution of the sample means is equal to the population mean.

To determine

Give the dispersion in the population with that in the distribution of the sample mean.

Answer to Problem 3SR

The dispersion in the population is greater than with that of the sample mean.

Explanation of Solution

The population values are 20, 22, 26, 24 and 28. From the part b, the mean of each sample is 21, 23, 22, 24, 24, 23, 25, 25, 27 and 26.

The population values are between 20 and 28. The sample mean values are between 21 and 27.

Thus, the dispersion in the population is greater than with that of the sample mean.

To determine

Check whether the distribution of population values is normally distributed or not.

Answer to Problem 3SR

The distribution of population values is not normally distributed.

Explanation of Solution

From the given chart, the frequency of all the years of service is at same level. Then, the distribution of the population values is uniform.

Thus, the distribution of population values is not normally distributed.

To determine

Check whether the distribution of the sample mean computed in part (c) starting to show some tendency toward being bell-shaped or not.

Answer to Problem 3SR

The distribution of the sample mean computed in part (c) starting to show some tendency toward being bell-shaped.

Explanation of Solution

From the part (c), it can be observed that the shape of the bar chart is bell shaped.

Thus, the distribution of the sample mean computed in part (c) starting to show some tendency toward being bell-shaped.