College Algebra By Openstax
17th Edition
ISBN: 9781506698229
Author: Jay Abramson;arizona State University
Publisher: XANEDU PUBLISHING
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 7.8, Problem 68SE
For the following exercises, use this scenario: A health-conscious company decides to make a trail mix Out Of almonds, dried cranberries, and chocolate-covered cashews. The nutritional information for these items is shown in Table 1.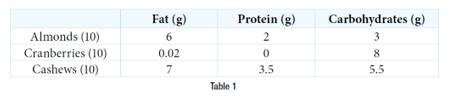 
68.For the "energy-booster" mix, there are 1,000 pieces in the mix, containing 145 g of protein and 625 g of carbohydrates. If the number of almonds and cashews summed together is equivalent to the amount of cranberries, how many of each item is in the trail mix?
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
During a typical school day, Sally recorded eating the following:For breakfast:½ cup of cooked porridge (made with 200mls of full fat cows milk)2 tablespoons of maple syrup on his porridge250mls of apple drinkSally’s lunch tray consisted of:150g high fat yoghurt Can of Sprite (330mls)Two servings of hotdogs1 apple (which she gives away)½ cup mixed vegetablesBarbecue sauceOn the way home from school Sally drank the following:Gatorade sports drink (500mls)For dinner Sally ate the following:One fillet of fish – battered and deep friedDeep fried fries (equivalent to one medium sized potato)One jam donutChocolate flavoured milk (full fat milk – 250mls)Snack before bed:Ham sandwich (2 slices of white bread and 1 slices of cheese)
Q1: How many servings of fruit and vegetables did Sally eat? How many servings of milk and milk products did Sally eat?
The weight of an adult wombat is N(25,2.2). You have 4 wombats. How much would your
wombats have to weigh in order to outweigh, on the average, 32% of all such groups of wombats?
"I am a healthy eater," Alex told his class. "I eat the same thing every day. For breakfast I eat 3/4 of a whey protein bar." The protein bar that Alex eats has a larger wrapper with nutritional information on it. A serving size is 1/2 of a bar. Each serving has 180 calories, 6 grams of fat, and 15 grams of protein.
"How is eating the same thing every day healthy?" asked Jennifer.
Alex ignored Jennifer. He prefers to brag. "Last year I only missed my healthy breakfast two time."
Last week Alex did NOT miss his healthy breakfast. How many grams of protein did Alex get from his breakfast last week? How many grams of fat did Alex get from his protein bar last year?
Chapter 7 Solutions
College Algebra By Openstax
Ch. 7.1 - Determine whether the ordered pair (8, 5) is a...Ch. 7.1 - Solve the following system of equations by...Ch. 7.1 - Solve the following system of equations by...Ch. 7.1 - Solve the system of equations by addition....Ch. 7.1 - Solve the system of equations by addition....Ch. 7.1 - Solve the following system of equations in two...Ch. 7.1 - Solve the following system of equations in two...Ch. 7.1 - Meal tickets at the circus cost $4.00 for children...Ch. 7.1 - Can a system of linear equations have exactly two...Ch. 7.1 - If you are performing a break-even analysis for a...
Ch. 7.1 - If you are solving a break-even analysis and get a...Ch. 7.1 - If you are solving a break-even analysis and there...Ch. 7.1 - Given a system of equations, explain at least two...Ch. 7.1 - For the following exercises, determine whether the...Ch. 7.1 - For the following exercises, determine whether the...Ch. 7.1 - For the following exercises, determine whether the...Ch. 7.1 - For the following exercises, determine whether the...Ch. 7.1 - For the following exercises, determine whether the...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, solve each system by...Ch. 7.1 - For the following exercises, graph the system of...Ch. 7.1 - For the following exercises, graph the system of...Ch. 7.1 - For the following exercises, graph the system of...Ch. 7.1 - For the following exercises, graph the system of...Ch. 7.1 - For the following exercises, graph the system of...Ch. 7.1 - For the following exercises, use the intersect...Ch. 7.1 - For the following exercises, use the intersect...Ch. 7.1 - For the following exercises, use the intersect...Ch. 7.1 - For the following exercises, use the intersect...Ch. 7.1 - For the following exercises, use the intersect...Ch. 7.1 - For the following exercises, solve each system in...Ch. 7.1 - For the following exercises, solve each system in...Ch. 7.1 - For the following exercises, solve each system in...Ch. 7.1 - For the following exercises, solve each system in...Ch. 7.1 - For the following exercises, solve each system in...Ch. 7.1 - For the following exercises, solve for the desired...Ch. 7.1 - For the following exercises, solve for the desired...Ch. 7.1 - For the following exercises, solve for the desired...Ch. 7.1 - For the following exercises, solve for the desired...Ch. 7.1 - For the following exercises, solve for the desired...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.1 - For the following exercises, use a system of...Ch. 7.2 - Solve the system of equations in three variables....Ch. 7.2 - Solve the system of equations in three variables....Ch. 7.2 - Solve the following system...Ch. 7.2 - Can a linear system of three equations have...Ch. 7.2 - If a given ordered triple solves the system of...Ch. 7.2 - If a given ordered triple does not solve the...Ch. 7.2 - Using the method of addition, is there only one...Ch. 7.2 - Can you explain whether there can be only one...Ch. 7.2 - For the Following exercises, determine whether the...Ch. 7.2 - For the Following exercises, determine whether the...Ch. 7.2 - For the Following exercises, determine whether the...Ch. 7.2 - For the Following exercises, determine whether the...Ch. 7.2 - For the Following exercises, determine whether the...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve each system by...Ch. 7.2 - For the following exercises, solve the system for...Ch. 7.2 - For the following exercises, solve the system for...Ch. 7.2 - For the following exercises, solve the system for...Ch. 7.2 - For the following exercises, solve the system for...Ch. 7.2 - For the following exercises, solve the system for...Ch. 7.2 - Three even numbers sum up to 108. The smaller is...Ch. 7.2 - Three numbers sum up to 147. The smallest number...Ch. 7.2 - At a family reunion, there were only blood...Ch. 7.2 - An animal shelter has a total of 350 animals...Ch. 7.2 - Your roommate, Sarah, offered to buy groceries for...Ch. 7.2 - Your roommate, John, offered to buy household...Ch. 7.2 - Three coworkers work for the same employer. Their...Ch. 7.2 - At a carnival, $2,914.25 in receipts were taken at...Ch. 7.2 - A local band sells out for their concert. They...Ch. 7.2 - In a bag, a child has 325 coins worth $19.50....Ch. 7.2 - Last year, at Haven's Pond Car Dealership, for a...Ch. 7.2 - A recent college graduate took advantage of his...Ch. 7.2 - You inherit one million dollars. You invest it all...Ch. 7.2 - You inherit one hundred thousand dollars. You...Ch. 7.2 - The top three countries in oil consumption in a...Ch. 7.2 - The top three countries in oil production in the...Ch. 7.2 - The top three sources of oil imports for the...Ch. 7.2 - The top three oil producers in the United States...Ch. 7.2 - At one time, in the United States, 398 species of...Ch. 7.2 - Meat consumption in the United States can be...Ch. 7.3 - Solve the given system of equations by...Ch. 7.3 - Solve the given system of equations by...Ch. 7.3 - Find the solution set for the given system of...Ch. 7.3 - Graph the given system of inequalities. yx21xy1Ch. 7.3 - Explain whether a system of two nonlinear...Ch. 7.3 - When graphing an inequality, explain why we only...Ch. 7.3 - When you graph a system of inequalities, will...Ch. 7.3 - If you graph a revenue and cost function, explain...Ch. 7.3 - If you perform your break-even analysis and there...Ch. 7.3 - For the following exercises, solve the system of...Ch. 7.3 - For the following exercises, solve the system of...Ch. 7.3 - For the following exercises, solve the system of...Ch. 7.3 - For the following exercises, solve the system of...Ch. 7.3 - For the following exercises, solve the system of...Ch. 7.3 - For the following exercises, solve the system of...Ch. 7.3 - For the following exercises, solve the system of...Ch. 7.3 - For the following exercises, solve the system of...Ch. 7.3 - For the following exercises, solve the system of...Ch. 7.3 - For the following exercises, solve the system of...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, use any method to...Ch. 7.3 - For the following exercises, graph the inequality....Ch. 7.3 - For the following exercises, graph the inequality....Ch. 7.3 - For the following exercises, graph the system of...Ch. 7.3 - For the following exercises, graph the system of...Ch. 7.3 - For the following exercises, graph the system of...Ch. 7.3 - For the following exercises, graph the system of...Ch. 7.3 - For the following exercises, graph the system of...Ch. 7.3 - For the following exercises, graph the inequality....Ch. 7.3 - For the following exercises, graph the inequality....Ch. 7.3 - For the following exercises, find the solutions to...Ch. 7.3 - For the following exercises, find the solutions to...Ch. 7.3 - For the following exercises, find the solutions to...Ch. 7.3 - For the following exercises, find the solutions to...Ch. 7.3 - For the following exercises, find the solutions to...Ch. 7.3 - For the following exercises, solve the system of...Ch. 7.3 - For the following exercises, solve the system of...Ch. 7.3 - For the following exercises, construct a system of...Ch. 7.3 - For the following exercises, construct a system of...Ch. 7.3 - For the following exercises, construct a system of...Ch. 7.3 - For the following exercises, construct a system of...Ch. 7.4 - Find the partial fraction decomposition of the...Ch. 7.4 - Find the partial fraction decomposition of the...Ch. 7.4 - Find the partial fraction decomposition of the...Ch. 7.4 - Find the partial fraction decomposition of the...Ch. 7.4 - Can any quotient of polynomials be decomposed into...Ch. 7.4 - Can you explain why a partial fraction...Ch. 7.4 - Can you explain how to verify a partial fraction...Ch. 7.4 - You are unsure if you correctly decomposed the...Ch. 7.4 - Once you have a system of equations generated by...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the...Ch. 7.4 - For the following exercises, find the partial...Ch. 7.4 - For the following exercises, find the partial...Ch. 7.4 - For the following exercises, perform the operation...Ch. 7.4 - For the following exercises, perform the operation...Ch. 7.4 - For the following exercises, perform the operation...Ch. 7.5 - Add matrix A and matrix B. A=[211603] and...Ch. 7.5 - Given matrix B, find - 2B where A=[4132]Ch. 7.5 - Can we add any two matrices together? If so,...Ch. 7.5 - Can we multiply any column matrix by any row...Ch. 7.5 - Can both the products AB and BA be defined? If so,...Ch. 7.5 - Can any two matrices of the same size be...Ch. 7.5 - Does matrix multiplication commute? That is, does...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrices...Ch. 7.5 - For the following exercises, use the matrix below...Ch. 7.5 - For the following exercises, use the matrix below...Ch. 7.5 - For the following exercises, use the matrix below...Ch. 7.5 - For the following exercises, use the matrix below...Ch. 7.5 - For the following exercises, use the matrix below...Ch. 7.6 - Write the augmented matrix of the given system of...Ch. 7.6 - Write the system of equations from the augmented...Ch. 7.6 - Solve the given system by Gaussian elimination....Ch. 7.6 - Write the system of equations in row-echelon form....Ch. 7.6 - Solve the system using matrices....Ch. 7.6 - A small shoe company took out a loan of $l,500,000...Ch. 7.6 - Can any system of linear equations be written as...Ch. 7.6 - Can any matrix be written as a system of linear...Ch. 7.6 - Is there only one correct method of using row...Ch. 7.6 - Can a matrix whose entry is 0 on the diagonal be...Ch. 7.6 - Can a matrix that has 0 entries for an entire row...Ch. 7.6 - For the following exercises, write the augmented...Ch. 7.6 - For the following exercises, write the augmented...Ch. 7.6 - For the following exercises, write the augmented...Ch. 7.6 - For the following exercises, write the augmented...Ch. 7.6 - For the following exercises, write the augmented...Ch. 7.6 - For the following exercises, write the linear...Ch. 7.6 - For the following exercises, write the linear...Ch. 7.6 - For the following exercises, write the linear...Ch. 7.6 - For the following exercises, write the linear...Ch. 7.6 - For the following exercises, write the linear...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, solve the system by...Ch. 7.6 - For the following exercises, Gaussian elimination...Ch. 7.6 - For the following exercises, Gaussian elimination...Ch. 7.6 - For the following exercises, Gaussian elimination...Ch. 7.6 - For the following exercises, Gaussian elimination...Ch. 7.6 - For the following exercises, Gaussian elimination...Ch. 7.6 - For the following exercises, set up the augmented...Ch. 7.6 - For the following exercises, set up the augmented...Ch. 7.6 - For the following exercises, set up the augmented...Ch. 7.6 - For the following exercises, set up the augmented...Ch. 7.6 - For the following exercises, set up the augmented...Ch. 7.6 - For the following exercises, set up the augmented...Ch. 7.6 - For the following exercises, set up the augmented...Ch. 7.6 - For the following exercises, set up the augmented...Ch. 7.6 - For the following exercises, set up the augmented...Ch. 7.6 - For the following exercises, set up the augmented...Ch. 7.7 - Show that the following two matrices are inverses...Ch. 7.7 - Use the formula to find the inverse of matrix A....Ch. 7.7 - Find the inverse of the 33 matrix....Ch. 7.7 - Solve the system using the inverse of the...Ch. 7.7 - In a previous section, we showed that matrix...Ch. 7.7 - Does every 22 matrix have an inverse? Explain why...Ch. 7.7 - Can you explain whether a 2×2 matrix with an...Ch. 7.7 - Can a matrix with an entire column of zeros have...Ch. 7.7 - Can a matrix with zeros on the diagonal have an...Ch. 7.7 - In the following exercises, show that matrix A is...Ch. 7.7 - In the following exercises, show that matrix A is...Ch. 7.7 - In the following exercises, show that matrix A is...Ch. 7.7 - In the following exercises, show that matrix A is...Ch. 7.7 - In the following exercises, show that matrix A is...Ch. 7.7 - In the following exercises, show that matrix A is...Ch. 7.7 - In the following exercises, show that matrix A is...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, find the...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, solve the system using...Ch. 7.7 - For the following exercise, use a calculator to...Ch. 7.7 - For the following exercise, use a calculator to...Ch. 7.7 - For the following exercise, use a calculator to...Ch. 7.7 - For the following exercise, use a calculator to...Ch. 7.7 - For the following exercises, find the inverse of...Ch. 7.7 - For the following exercises, find the inverse of...Ch. 7.7 - For the following exercises, find the inverse of...Ch. 7.7 - For the following exercises, find the inverse of...Ch. 7.7 - For the following exercises, find the inverse of...Ch. 7.7 - For the following exercises, write a system of...Ch. 7.7 - For the following exercises, write a system of...Ch. 7.7 - For the following exercises, write a system of...Ch. 7.7 - For the following exercises, write a system of...Ch. 7.7 - For the following exercises, write a system of...Ch. 7.7 - For the following exercises, write a system of...Ch. 7.7 - For the following exercises, write a system of...Ch. 7.7 - For the following exercises, write a system of...Ch. 7.7 - For the following exercises, write a system of...Ch. 7.7 - For the following exercises, write a system of...Ch. 7.8 - Use Cramer's Rule to solve the 22 system of...Ch. 7.8 - Find the determinant of the 33 matrix....Ch. 7.8 - Use Cramer's Rule to solve the 33 matrix....Ch. 7.8 - Explain why we can always evaluate the determinant...Ch. 7.8 - Examining Cramer's Rule, explain why there is no...Ch. 7.8 - Explain what it means in terms of an inverse for a...Ch. 7.8 - The determinant of 22 matrix A is 3. If you switch...Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, find the determinant....Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, solve the system of...Ch. 7.8 - For the following exercises, use the determinant...Ch. 7.8 - For the following exercises, use the determinant...Ch. 7.8 - For the following exercises, use the determinant...Ch. 7.8 - For the following exercises, use the determinant...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercise, create a system of...Ch. 7.8 - For the following exercises, use this scenario: A...Ch. 7.8 - For the following exercises, use this scenario: A...Ch. 7.8 - For the following exercises, use this scenario: A...Ch. 7 - For the following exercise, determine whether the...Ch. 7 - For the following exercise, determine whether the...Ch. 7 - For the following exercises, use substitution to...Ch. 7 - For the following exercises, use substitution to...Ch. 7 - For the following exercises, use substitution to...Ch. 7 - For the following exercises, use addition to solve...Ch. 7 - For the following exercises, use addition to solve...Ch. 7 - For the following exercises, use addition to solve...Ch. 7 - For the following exercises, write a system of...Ch. 7 - For the following exercises, write a system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, perform the given...Ch. 7 - For the following exercises, write a system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, graph the inequality....Ch. 7 - For the following exercises, graph the system of...Ch. 7 - For the following exercises, graph the system of...Ch. 7 - For the following exercise, graph the system of...Ch. 7 - For the following exercises, graph the system of...Ch. 7 - For the following exercises decompose into partial...Ch. 7 - For the following exercises decompose into partial...Ch. 7 - For the following exercises decompose into partial...Ch. 7 - For the following exercises decompose into partial...Ch. 7 - For the following exercises decompose into partial...Ch. 7 - For the following exercises decompose into partial...Ch. 7 - For the following exercises decompose into partial...Ch. 7 - For the following exercises decompose into partial...Ch. 7 - For the following exercises, perform the requested...Ch. 7 - For the following exercises, perform the requested...Ch. 7 - For the following exercises, perform the requested...Ch. 7 - For the following exercises, perform the requested...Ch. 7 - For the following exercises, perform the requested...Ch. 7 - For the following exercises, perform the requested...Ch. 7 - For the following exercises, perform the requested...Ch. 7 - For the following exercises, perform the requested...Ch. 7 - For the following exercises, perform the requested...Ch. 7 - For the following exercises, perform the requested...Ch. 7 - For the following exercises, perform the requested...Ch. 7 - For the following exercises, perform the requested...Ch. 7 - For the following exercises, write the system of...Ch. 7 - For the following exercises, write the system of...Ch. 7 - For the following exercises, write the augmented...Ch. 7 - For the following exercises, write the augmented...Ch. 7 - For the following exercises, write the augmented...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, find the inverse of...Ch. 7 - For the following exercises, find the inverse of...Ch. 7 - For the following exercises, find the inverse of...Ch. 7 - For the following exercises, find the inverse of...Ch. 7 - For the following exercises, find the solutions by...Ch. 7 - For the following exercises, find the solutions by...Ch. 7 - For the following exercises, find the solutions by...Ch. 7 - For the following exercises, find the solutions by...Ch. 7 - For the following exercises, write a system of...Ch. 7 - For the following exercises, write a system of...Ch. 7 - For the following exercises, find the determinant....Ch. 7 - For the following exercises, find the determinant....Ch. 7 - For the following exercises, find the determinant....Ch. 7 - For the following exercises, find the determinant....Ch. 7 - For the following exercises, use Cramer’s rule to...Ch. 7 - For the following exercises, use Cramer’s rule to...Ch. 7 - For the following exercises, use Cramer’s Rule to...Ch. 7 - For the following exercises, use Cramer’s rule to...Ch. 7 - For the following exercises, use Cramer’s rule to...Ch. 7 - For the following exercises, use Cramer’s rule to...Ch. 7 - Is the following ordered pair a solution to the...Ch. 7 - For the following exercises, solve the systems of...Ch. 7 - ]For the following exercises, solve the systems of...Ch. 7 - For the following exercises, solve the systems of...Ch. 7 - For the following exercises, solve the systems of...Ch. 7 - For the following exercises, solve the systems of...Ch. 7 - For the following exercises, solve the systems of...Ch. 7 - For the following exercises, solve the systems of...Ch. 7 - For the following exercises, solve the systems of...Ch. 7 - For the following exercises, graph the following...Ch. 7 - For the following exercises, graph the following...Ch. 7 - For the following exercises, write the partial...Ch. 7 - For the following exercises, write the partial...Ch. 7 - For the following exercises, write the partial...Ch. 7 - For the following exercises, perform the given...Ch. 7 - For the following exercises, perform the given...Ch. 7 - For the following exercises, perform the given...Ch. 7 - For the following exercises, perform the given...Ch. 7 - For the following exercises, perform the given...Ch. 7 - For the following exercises, perform the given...Ch. 7 - For the following exercises, perform the given...Ch. 7 - For the following exercises, perform the given...Ch. 7 - For the following exercises, solve the system of...Ch. 7 - For the following exercises, use Gaussian...Ch. 7 - For the following exercises, use the inverse of a...Ch. 7 - For the following exercises, use the inverse of a...Ch. 7 - For the following exercises, use Cramer’s Rule to...Ch. 7 - For the following exercises, use Cramer’s Rule to...Ch. 7 - For the following exercises, solve using a system...Ch. 7 - For the following exercises, solve using a system...
Additional Math Textbook Solutions
Find more solutions based on key concepts
Identify f as being linear, quadratic, or neither. If f is quadratic, identify the leading coefficient a and ...
College Algebra with Modeling & Visualization (5th Edition)
Exercise 117–120: For each function findand.
117.
College Algebra with Modeling & Visualization (6th Edition)
In the following exercises, find three solutions to each equation and then graph each line. y=3x
PREALGEBRA
Find a polynomial equation with real coefficients that has the given roots. 1, 2 – 3i
College Algebra (6th Edition)
Solve each formula for the given letter . [2.3] What percent of 60 is 42? [2.4]
Elementary and Intermediate Algebra
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- 3 quarts of diced onions are needed in a recipe. What is the cost? The yield percent for onions is 86%; onions cost $1.39 per pound; 1 cup of diced onions weighs 5.5 oz.; each onion weighs 9 oz.arrow_forwardA steakhouse forecasts 150 customers and typically 18% of the guests order the chicken. The yield is 73% and each portion is 6oz. The chef has 3# in the inventory, how many pounds of chicken should the chef order and round up the nearest pound as a safety net. Select one: a. 12# b. 11# с. 10# d. 14#arrow_forwardHow much water would you expect the people in a city with a population of 100,000 to use in 1 day? Use the following information to estimate the total water usage: a. Many showers have a water flow of 10 liters per minute. Let's say that a person showers each day for 12 minutes. b. Many toilets use about 4.2 liters of water per flush. Let's say that a person flushes toilets 6 times each day. C. Let's say that a person drinks 2 liters of water each day. d. Let's say that a person uses 18 liters to prepare meals and to wash dishes and laundry each day.arrow_forward
- The following is the nutrition label from a 5 oz. container of a three cheese blend of shredded cheese. The container costs $3.99. What is the cost per serving? Nutrition Amountserving Facts about 28 servings per container Serving size 1 Tosp (59) Calories % DV Amount/serving 2% Total Carb. Og 5% Fiber 0g Total Sugars 0g 1% Incl. Og Added Sugars 1% Protein 1g % DV Total Fat 1.5g Sat. Fat 1g 0% 0% Trans Fat 0g Cholest. <5mg Sodium 30mg 0% per serving 20 Vit. D 0% • Calcium 4% • Iron 0% • Potas. 0% O $0.143 O $1.33 O $0.798 O $0.266 --- |T||arrow_forwardPlanter's Peanut Company wants to mix 20 pounds of peanuts worth $3 a pound with cashews worth $5 a pound in order to make an experimental mix worth $3.50 a pound. How many pounds of cashews should be added to the peanuts? Number of Cost per Роunds Value Рound $3 per lb Peanuts $5 per lb Cashews $3.50 per lb Mixture Wantedarrow_forwardIf Sue eats 1/8 of a large cake, and Bill eats 2/4 of a small cake, who eats more cake?arrow_forward
- A health clinic uses a solution of bleach to sterilize petri dishes in which cultures are grown. The sterilization tank contains 60 gal of a solution of 4% ordinary household bleach mixed with pure distilled water. New research indicates that the concentration of bleach should be 6% for complete sterilization. How much of the solution should be drained and replaced with bleach to increase the bleach content to the recommended level? gal?arrow_forwardWhich is more economical: purchasing the economy size of a detergent at 6kilograms for $6.15 or purchasing the regular size at 910 grams for 60¢?arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
What is a Linear Equation in One Variable?; Author: Don't Memorise;https://www.youtube.com/watch?v=lDOYdBgtnjY;License: Standard YouTube License, CC-BY
Linear Equation | Solving Linear Equations | What is Linear Equation in one variable ?; Author: Najam Academy;https://www.youtube.com/watch?v=tHm3X_Ta_iE;License: Standard YouTube License, CC-BY