
a.
Construct the histogram for body mass of the 50 difference bird species based on the class intervals.
Identify whether transformation of the data is desirable or not.
a.
Answer to Problem 107E
The histogram for body mass of the 50 difference bird species based on the class intervals is given below:
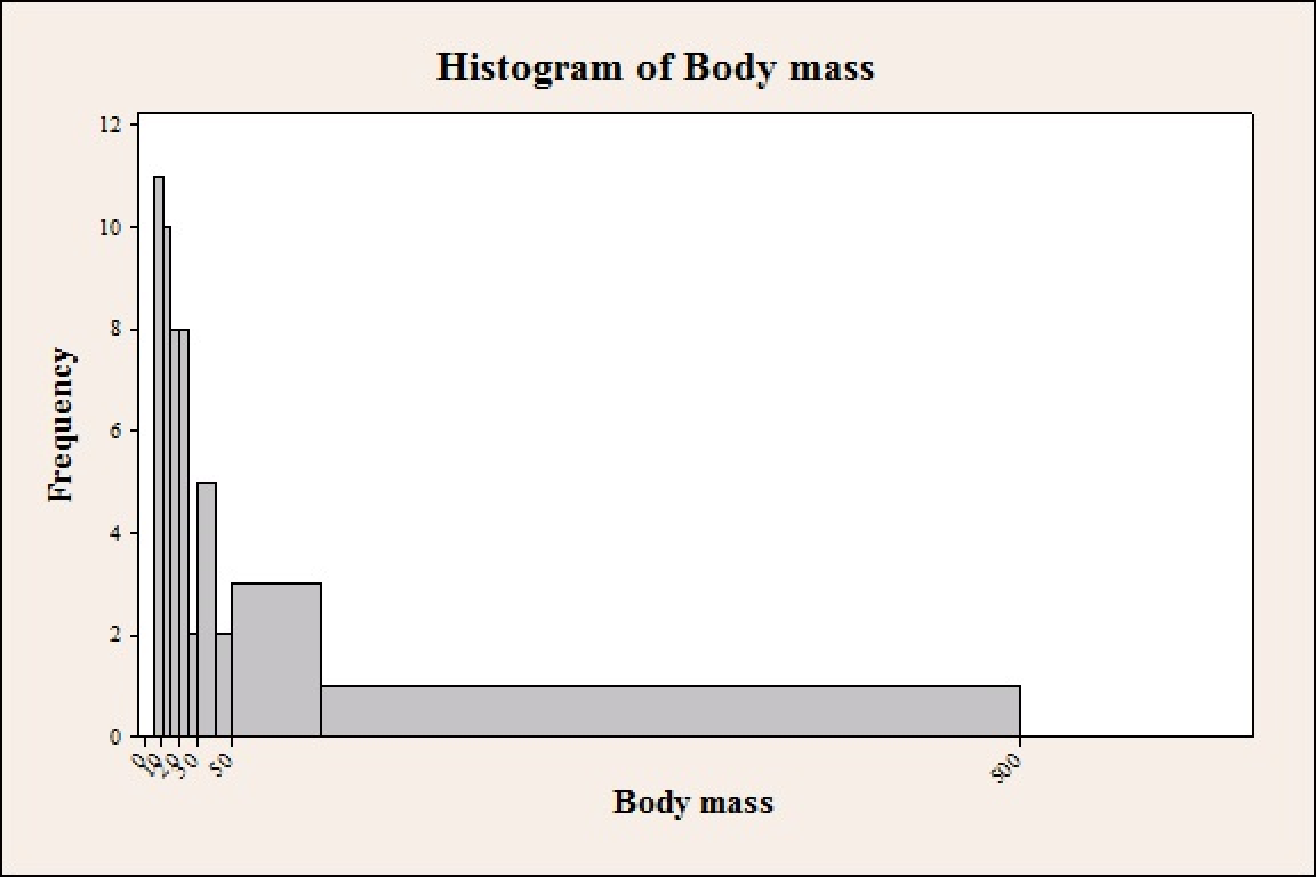
The transformation of the data is desirable.
Explanation of Solution
Calculation:
It is given that the body mass of the 50 different bird species.
Software procedure:
Step-by-step procedure to construct the histogram using the MINITAB software:
- Choose Graph > Histogram.
- Select Simple, and then click OK.
- In Graph variables, enter the corresponding column of Body mass.
- Click OK.
- Double click on x axis and select Binning.
- Choose Cutpoint and enter Number of intervals as 9.
- Enter 5 10 15 20 25 30 40 50 100 500 under Midpoint/Cutpoint positions.
- Click Ok.
From the histogram, it can be observed that most of the data values fall towards the left of the distribution extending the tail towards the right indicating that the distribution of body mass is positively skewed. Thus, for making the data symmetric a transformation is needed.
Hence, the transformation of the data is desirable.
b.
Construct the histogram by transforming the data using logarithms.
Identify whether the log transformation was successful in producing a more symmetric distribution or not.
b.
Answer to Problem 107E
The histogram by transforming the data using logarithms is given below:
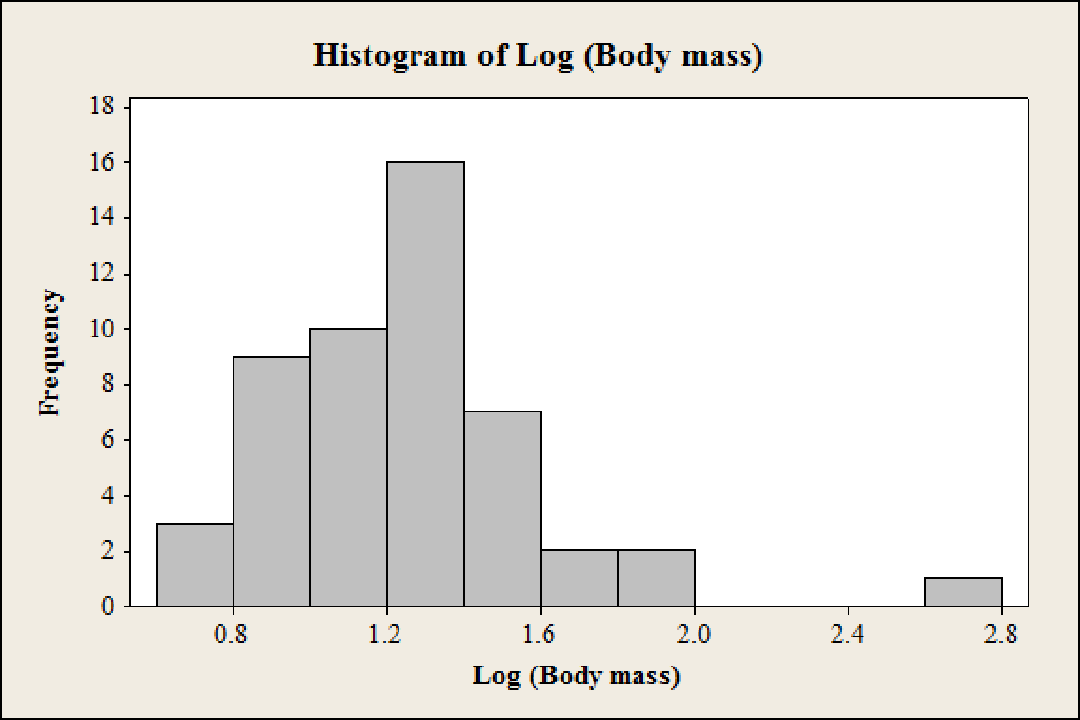
Yes, the log transformation was successful in producing a more symmetric distribution.
Explanation of Solution
Calculation:
It is given that the body mass of the 50 different bird species. The log for the data values is obtained as shown below:
| Body Mass | Log (Body Mass) | Transformed values |
| 7.7 | log (7.7) | 0.88649 |
| 10.1 | log (10.1) | 1.00432 |
| 21.6 | log (21.6) | 1.33445 |
| 8.6 | log (8.6) | 0.93450 |
| 12 | log (12) | 1.07918 |
| 11.4 | log (11.4) | 1.05690 |
| 16.6 | log (16.6) | 1.22011 |
| 9.4 | log (9.4) | 0.97313 |
| 11.5 | log (11.5) | 1.06070 |
| 9 | log (9) | 0.95424 |
| 8.2 | log (8.2) | 0.91381 |
| 20.2 | log (20.2) | 1.30535 |
| 48.5 | log (48.5) | 1.68574 |
| 21.6 | log (21.6) | 1.33445 |
| 26.1 | log (26.1) | 1.41664 |
| 6.2 | log (6.2) | 0.79239 |
| 19.1 | log (19.1) | 1.28103 |
| 21 | log (21) | 1.32222 |
| 28.1 | log (28.1) | 1.44871 |
| 10.6 | log (10.6) | 1.02531 |
| 31.6 | log (31.6) | 1.49969 |
| 6.7 | log (6.7) | 0.82607 |
| 5 | log (5) | 0.69897 |
| 68.8 | log (68.8) | 1.83759 |
| 23.9 | log (23.9) | 1.37840 |
| 19.8 | log (19.8) | 1.29667 |
| 20.1 | log (20.1) | 1.30320 |
| 6 | log (6) | 0.77815 |
| 99.6 | log (99.6) | 1.99826 |
| 19.8 | log (19.8) | 1.29667 |
| 16.5 | log (16.5) | 1.21748 |
| 9 | log (9) | 0.95424 |
| 448 | log (448) | 2.65128 |
| 21.3 | log (21.3) | 1.32838 |
| 17.4 | log (17.4) | 1.24055 |
| 36.9 | log (36.9) | 1.56703 |
| 34 | log (34) | 1.53148 |
| 41 | log (41) | 1.61278 |
| 15.9 | log (15.9) | 1.20140 |
| 12.5 | log (12.5) | 1.09691 |
| 10.2 | log (10.2) | 1.00860 |
| 31 | log (31) | 1.49136 |
| 21.5 | log (21.5) | 1.33244 |
| 11.9 | log (11.9) | 1.07555 |
| 32.5 | log (32.5) | 1.51188 |
| 9.8 | log (9.8) | 0.99123 |
| 93.9 | log (93.9) | 0.97267 |
| 10.9 | log (10.9) | 1.03743 |
| 19.6 | log (19.6) | 1.29226 |
| 14.5 | log (14.5) | 1.16137 |
Software procedure:
Step-by-step procedure to obtain histogram using MINITAB:
- Choose Graph > Histogram.
- Choose Simple, and then click OK.
- In Graph variables, enter the corresponding column of Logarithm of Body mass.
- Click OK.
- On graph right click on X axis, select edit X scale.
- Select Binning.
- Under Interval Type, Choose cut point.
- Click OK.
Justification: It can be observed that the histogram of the transformed data (using logarithms) is more near to symmetrical shape when compared to the untransformed data. This shows that using the transformation of logarithms produce a more symmetrical distribution for body mass.
Hence, the log transformation was successful in producing a more symmetric distribution.
c.
Construct the histogram by transforming the data using
Identify whether the transformation
c.
Answer to Problem 107E
The histogram by transforming the data using
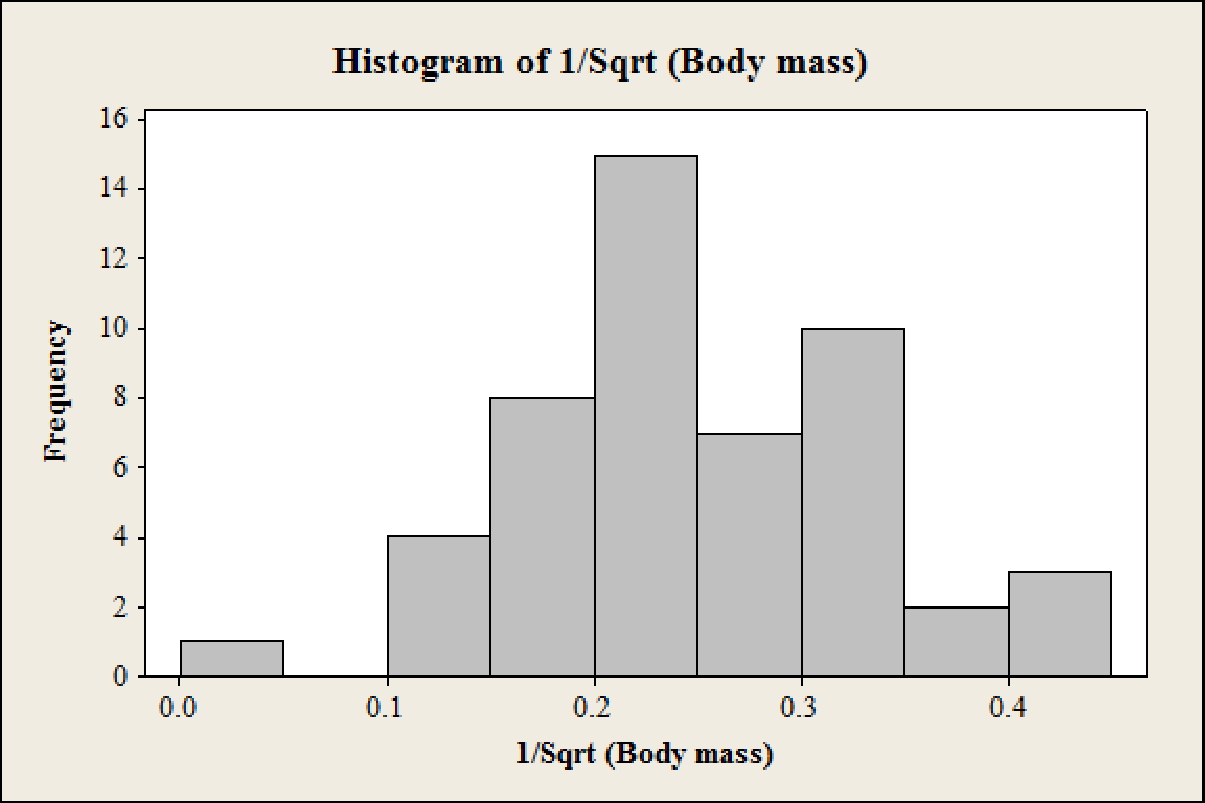
Yes, the transformation
Explanation of Solution
Calculation:
The transformation for the data values using
| Body Mass | Transformed values | |
| 7.7 | 0.360375 | |
| 10.1 | 0.314658 | |
| 21.6 | 0.215166 | |
| 8.6 | 0.340997 | |
| 12 | 0.288675 | |
| 11.4 | 0.296174 | |
| 16.6 | 0.245440 | |
| 9.4 | 0.326164 | |
| 11.5 | 0.294884 | |
| 9 | 0.333333 | |
| 8.2 | 0.349215 | |
| 20.2 | 0.222497 | |
| 48.5 | 0.143592 | |
| 21.6 | 0.215166 | |
| 26.1 | 0.195740 | |
| 6.2 | 0.401610 | |
| 19.1 | 0.228814 | |
| 21 | 0.218218 | |
| 28.1 | 0.188646 | |
| 10.6 | 0.307148 | |
| 31.6 | 0.177892 | |
| 6.7 | 0.386334 | |
| 5 | 0.447214 | |
| 68.8 | 0.120561 | |
| 23.9 | 0.204551 | |
| 19.8 | 0.224733 | |
| 20.1 | 0.223050 | |
| 6 | 0.408248 | |
| 99.6 | 0.100201 | |
| 19.8 | 0.224733 | |
| 16.5 | 0.246183 | |
| 9 | 0.333333 | |
| 448 | 0.017546 | |
| 21.3 | 0.216676 | |
| 17.4 | 0.239732 | |
| 36.9 | 0.164622 | |
| 34 | 0.171499 | |
| 41 | 0.156174 | |
| 15.9 | 0.250785 | |
| 12.5 | 0.282843 | |
| 10.2 | 0.313112 | |
| 31 | 0.179605 | |
| 21.5 | 0.215666 | |
| 11.9 | 0.289886 | |
| 32.5 | 0.175412 | |
| 9.8 | 0.319438 | |
| 93.9 | 0.103197 | |
| 10.9 | 0.302891 | |
| 19.6 | 0.225877 | |
| 14.5 | 0.262613 |
Software procedure:
Step-by-step procedure to obtain histogram using MINITAB:
- Choose Graph > Histogram.
- Choose Simple, and then click OK.
- In Graph variables, enter the corresponding column of 1/sqrt(Body mass).
- Click OK.
- On graph right click on X axis, select edit X scale.
- Select Binning.
- Under Interval Type, Choose cut point.
- Click OK.
Justification: It can be observed that the histogram of the transformed data (using
Hence, the transformation
Want to see more full solutions like this?
Chapter 7 Solutions
Introduction To Statistics And Data Analysis
- Discuss and explain in the picturearrow_forwardBob and Teresa each collect their own samples to test the same hypothesis. Bob’s p-value turns out to be 0.05, and Teresa’s turns out to be 0.01. Why don’t Bob and Teresa get the same p-values? Who has stronger evidence against the null hypothesis: Bob or Teresa?arrow_forwardReview a classmate's Main Post. 1. State if you agree or disagree with the choices made for additional analysis that can be done beyond the frequency table. 2. Choose a measure of central tendency (mean, median, mode) that you would like to compute with the data beyond the frequency table. Complete either a or b below. a. Explain how that analysis can help you understand the data better. b. If you are currently unable to do that analysis, what do you think you could do to make it possible? If you do not think you can do anything, explain why it is not possible.arrow_forward
- 0|0|0|0 - Consider the time series X₁ and Y₁ = (I – B)² (I – B³)Xt. What transformations were performed on Xt to obtain Yt? seasonal difference of order 2 simple difference of order 5 seasonal difference of order 1 seasonal difference of order 5 simple difference of order 2arrow_forwardCalculate the 90% confidence interval for the population mean difference using the data in the attached image. I need to see where I went wrong.arrow_forwardMicrosoft Excel snapshot for random sampling: Also note the formula used for the last column 02 x✓ fx =INDEX(5852:58551, RANK(C2, $C$2:$C$51)) A B 1 No. States 2 1 ALABAMA Rand No. 0.925957526 3 2 ALASKA 0.372999976 4 3 ARIZONA 0.941323044 5 4 ARKANSAS 0.071266381 Random Sample CALIFORNIA NORTH CAROLINA ARKANSAS WASHINGTON G7 Microsoft Excel snapshot for systematic sampling: xfx INDEX(SD52:50551, F7) A B E F G 1 No. States Rand No. Random Sample population 50 2 1 ALABAMA 0.5296685 NEW HAMPSHIRE sample 10 3 2 ALASKA 0.4493186 OKLAHOMA k 5 4 3 ARIZONA 0.707914 KANSAS 5 4 ARKANSAS 0.4831379 NORTH DAKOTA 6 5 CALIFORNIA 0.7277162 INDIANA Random Sample Sample Name 7 6 COLORADO 0.5865002 MISSISSIPPI 8 7:ONNECTICU 0.7640596 ILLINOIS 9 8 DELAWARE 0.5783029 MISSOURI 525 10 15 INDIANA MARYLAND COLORADOarrow_forward
- Suppose the Internal Revenue Service reported that the mean tax refund for the year 2022 was $3401. Assume the standard deviation is $82.5 and that the amounts refunded follow a normal probability distribution. Solve the following three parts? (For the answer to question 14, 15, and 16, start with making a bell curve. Identify on the bell curve where is mean, X, and area(s) to be determined. 1.What percent of the refunds are more than $3,500? 2. What percent of the refunds are more than $3500 but less than $3579? 3. What percent of the refunds are more than $3325 but less than $3579?arrow_forwardA normal distribution has a mean of 50 and a standard deviation of 4. Solve the following three parts? 1. Compute the probability of a value between 44.0 and 55.0. (The question requires finding probability value between 44 and 55. Solve it in 3 steps. In the first step, use the above formula and x = 44, calculate probability value. In the second step repeat the first step with the only difference that x=55. In the third step, subtract the answer of the first part from the answer of the second part.) 2. Compute the probability of a value greater than 55.0. Use the same formula, x=55 and subtract the answer from 1. 3. Compute the probability of a value between 52.0 and 55.0. (The question requires finding probability value between 52 and 55. Solve it in 3 steps. In the first step, use the above formula and x = 52, calculate probability value. In the second step repeat the first step with the only difference that x=55. In the third step, subtract the answer of the first part from the…arrow_forwardIf a uniform distribution is defined over the interval from 6 to 10, then answer the followings: What is the mean of this uniform distribution? Show that the probability of any value between 6 and 10 is equal to 1.0 Find the probability of a value more than 7. Find the probability of a value between 7 and 9. The closing price of Schnur Sporting Goods Inc. common stock is uniformly distributed between $20 and $30 per share. What is the probability that the stock price will be: More than $27? Less than or equal to $24? The April rainfall in Flagstaff, Arizona, follows a uniform distribution between 0.5 and 3.00 inches. What is the mean amount of rainfall for the month? What is the probability of less than an inch of rain for the month? What is the probability of exactly 1.00 inch of rain? What is the probability of more than 1.50 inches of rain for the month? The best way to solve this problem is begin by a step by step creating a chart. Clearly mark the range, identifying the…arrow_forward
- Client 1 Weight before diet (pounds) Weight after diet (pounds) 128 120 2 131 123 3 140 141 4 178 170 5 121 118 6 136 136 7 118 121 8 136 127arrow_forwardClient 1 Weight before diet (pounds) Weight after diet (pounds) 128 120 2 131 123 3 140 141 4 178 170 5 121 118 6 136 136 7 118 121 8 136 127 a) Determine the mean change in patient weight from before to after the diet (after – before). What is the 95% confidence interval of this mean difference?arrow_forwardIn order to find probability, you can use this formula in Microsoft Excel: The best way to understand and solve these problems is by first drawing a bell curve and marking key points such as x, the mean, and the areas of interest. Once marked on the bell curve, figure out what calculations are needed to find the area of interest. =NORM.DIST(x, Mean, Standard Dev., TRUE). When the question mentions “greater than” you may have to subtract your answer from 1. When the question mentions “between (two values)”, you need to do separate calculation for both values and then subtract their results to get the answer. 1. Compute the probability of a value between 44.0 and 55.0. (The question requires finding probability value between 44 and 55. Solve it in 3 steps. In the first step, use the above formula and x = 44, calculate probability value. In the second step repeat the first step with the only difference that x=55. In the third step, subtract the answer of the first part from the…arrow_forward
 Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL

