EBK THINKING MATHEMATICALLY
EBK THINKING MATHEMATICALLY
6th Edition
ISBN: 9780100802728
Author: Blitzer
Publisher: YUZU
bartleby

Concept explainers

Unitary Method
The word “unitary” comes from the word “unit”, which means a single and complete entity. In this method, we find the value of a unit product from the given number of products, and then we solve for the other number of products.
Speed, Time, and Distance
Imagine you and 3 of your friends are planning to go to the playground at 6 in the evening. Your house is one mile away from the playground and one of your friends named Jim must start at 5 pm to reach the playground by walk. The other two friends are 3 …
Profit and Loss
The amount earned or lost on the sale of one or more items is referred to as the profit or loss on that item.
Units and Measurements
Measurements and comparisons are the foundation of science and engineering. We, therefore, need rules that tell us how things are measured and compared. For these measurements and comparisons, we perform certain experiments, and we will need the experime…
bartleby

Videos

Textbook Question
Book Icon
Chapter 7.3, Problem 61E

An important application of systems of equations arises in connection with supply and demand. As the price of a product increases, the demand for that product decreases. However, at higher prices, suppliers are willing to produce greater quantities of the product. The price at which supply and demand are equal is called the equilibrium price. The quantity supplied and demanded at that price is called the equilibrium quantity. Exercises 61-62 involve supply and demand.

The following models describe wages for low-skilled labor.

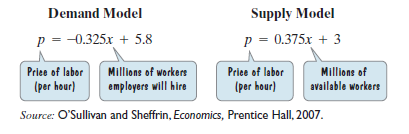
Chapter 7.3, Problem 61E, An important application of systems of equations arises in connection with supply and demand. As the

a. Solve the system and find the equilibrium number of workers, in millions, and the equilibrium hourly wage.

b. Use your answer from part (a) to complete this statement:

If workers are paid ____ per hour, there will be ____ million available workers and ____ million workers will be hired.

c. In 2007, the federal minimum wage was set at $5.15 per hour. Substitute 5.15 for p in the demand model, p = − 0.325 x + 5.8 , and determine the millions of workers employers will hire at this price.

d. At a minimum wage of $5.15 per hour, use the supply model, p = 0.375 x + 3 , to determine the millions of available workers. Round to one decimal place.

e. At a minimum wage of $5.15 per hour, use your answers from parts (c) and (d) to determine how many more people are looking for work than employers are willing to hire.

Expert Solution & Answer
Check Mark

Want to see the full answer?

Check out a sample textbook solution
Blurred answer
Previous
Chapter 7.3, Problem 60E
Next
Chapter 7.3, Problem 62E
Students have asked these similar questions
a) Find the scalars p, q, r, s, k1, and k2.  b) Is there a different linearly independent eigenvector associated to either k1 or k2? If yes,find it. If no, briefly explain.
Plz no chatgpt answer Plz  Will upvote
1/ Solve the following: 1 x + X + cos(3X) -75 -1 2 2 (5+1) e 5² + 5 + 1 3 L -1 1 5² (5²+1) 1 5(5-5)

Chapter 7 Solutions

EBK THINKING MATHEMATICALLY

Ch. 7.1 - CHECK POINT 1 Plot the points: A ( − 2 , 4 ) , B (...Ch. 7.1 - CHECK POINT 2 Graph y = 4 − x . Select integers...Ch. 7.1 - CHECK POINT 3 The toll to a bridge costs $2. If...Ch. 7.1 - CHECK POINT 4 Find each of the following: a. f(6)...Ch. 7.1 - CHECK POINT 5 a. Use the line graph in Figure 7.7...Ch. 7.1 - CHECK POINT 6 Graph the functions f(x) = 2x and...Ch. 7.1 - Prob. 7CPCh. 7.1 - Prob. 8CPCh. 7.1 - Fill in each blank so that the resulting statement...Ch. 7.1 - Prob. 2CVC
Ch. 7.1 - Prob. 3CVCCh. 7.1 - Prob. 4CVCCh. 7.1 - Prob. 5CVCCh. 7.1 - Prob. 6CVCCh. 7.1 - If an equation in two variables (x and y) yields...Ch. 7.1 - Prob. 8CVCCh. 7.1 - Fill in each blank so that the resulting statement...Ch. 7.1 - In Exercises 1-20, plot the given point in a...Ch. 7.1 - In Exercises 1-20, plot the given point in a...Ch. 7.1 - Prob. 3ECh. 7.1 - Prob. 4ECh. 7.1 - Prob. 5ECh. 7.1 - In Exercises 1-20, plot the given point in a...Ch. 7.1 - In Exercises 1-20, plot the given point in a...Ch. 7.1 - Prob. 8ECh. 7.1 - Prob. 9ECh. 7.1 - In Exercises 1-20, plot the given point in a...Ch. 7.1 - Prob. 11ECh. 7.1 - In Exercises 1-20, plot the given point in a...Ch. 7.1 - Prob. 13ECh. 7.1 - Prob. 14ECh. 7.1 - In Exercises 1-20, plot the given point in a...Ch. 7.1 - In Exercises 1-20, plot the given point in a...Ch. 7.1 - Prob. 17ECh. 7.1 - Prob. 18ECh. 7.1 - Prob. 19ECh. 7.1 - Prob. 20ECh. 7.1 - Graph each equation in Exercises 21-32. Select...Ch. 7.1 - Graph each equation in Exercises 21-32. Select...Ch. 7.1 - Graph each equation in Exercises 21-32. Select...Ch. 7.1 - Prob. 24ECh. 7.1 - Graph each equation in Exercises 21-32. Select...Ch. 7.1 - Graph each equation in Exercises 21-32. Select...Ch. 7.1 - Prob. 27ECh. 7.1 - Graph each equation in Exercises 21-32. Select...Ch. 7.1 - Prob. 29ECh. 7.1 - Graph each equation in Exercises 21-32. Select...Ch. 7.1 - Prob. 31ECh. 7.1 - Prob. 32ECh. 7.1 - In Exercises 33-46, evaluate each function at the...Ch. 7.1 - In Exercises 33-46, evaluate each function at the...Ch. 7.1 - In Exercises 33-46, evaluate each function at the...Ch. 7.1 - In Exercises 33-46, evaluate each function at the...Ch. 7.1 - In Exercises 33-46, evaluate each function at the...Ch. 7.1 - In Exercises 33-46, evaluate each function at the...Ch. 7.1 - In Exercises 33-46, evaluate each function at the...Ch. 7.1 - In Exercises 33-46, evaluate each function at the...Ch. 7.1 - In Exercises 33-46, evaluate each function at the...Ch. 7.1 - Prob. 42ECh. 7.1 - In Exercises 33-46, evaluate each function at the...Ch. 7.1 - In Exercises 33-46, evaluate each function at the...Ch. 7.1 - In Exercises 33-46, evaluate each function at the...Ch. 7.1 - Prob. 46ECh. 7.1 - In Exercises 47-54, evaluate f(x) for the given...Ch. 7.1 - In Exercises 47-54, evaluate f(x) for the given...Ch. 7.1 - In Exercises 47-54, evaluate f(x) for the given...Ch. 7.1 - In Exercises 47-54, evaluate f(x) for the given...Ch. 7.1 - In Exercises 47-54, evaluate f(x) for the given...Ch. 7.1 - Prob. 52ECh. 7.1 - In Exercises 47-54, evaluate f(x) for the given...Ch. 7.1 - Prob. 54ECh. 7.1 - For Exercises 55-62, use the vertical line test to...Ch. 7.1 - For Exercises 55-62, use the vertical line test to...Ch. 7.1 - For Exercises 55-62, use the vertical line test to...Ch. 7.1 - Prob. 58ECh. 7.1 - For Exercises 55-62, use the vertical line test to...Ch. 7.1 - Prob. 60ECh. 7.1 - For Exercises 55-62, use the vertical line test to...Ch. 7.1 - For Exercises 55-62, use the vertical line test to...Ch. 7.1 - Prob. 63ECh. 7.1 - Prob. 64ECh. 7.1 - Prob. 65ECh. 7.1 - Prob. 66ECh. 7.1 - Prob. 67ECh. 7.1 - Prob. 68ECh. 7.1 - Prob. 69ECh. 7.1 - Prob. 70ECh. 7.1 - A football is thrown by a quarterback to a...Ch. 7.1 - Prob. 72ECh. 7.1 - Prob. 73ECh. 7.1 - Prob. 74ECh. 7.1 - Prob. 75ECh. 7.1 - Prob. 76ECh. 7.1 - The function G ( x ) = − 0.01 x 2 + x + 60 models...Ch. 7.1 - Prob. 78ECh. 7.1 - The function f ( x ) = 0.4 x 2 − 36 x + 1000...Ch. 7.1 - Prob. 80ECh. 7.1 - The function f ( x ) = 0.4 x 2 − 36 x + 1000...Ch. 7.1 - Prob. 82ECh. 7.1 - What is the rectangular coordinate system?Ch. 7.1 - Prob. 84ECh. 7.1 - Prob. 85ECh. 7.1 - Prob. 86ECh. 7.1 - Prob. 87ECh. 7.1 - Prob. 88ECh. 7.1 - Prob. 89ECh. 7.1 - Prob. 90ECh. 7.1 - Prob. 91ECh. 7.1 - Make Sense? In Exercises 89-92, determine whether...Ch. 7.1 - Prob. 93ECh. 7.1 - Prob. 94ECh. 7.1 - Prob. 95ECh. 7.1 - Prob. 96ECh. 7.1 - Use a graphing calculator to verify the graphs...Ch. 7.2 - CHECK POINT 1 Graph: 2 x + 3 y = 6.Ch. 7.2 - CHECK POINT 2 Find the slope of the line passing...Ch. 7.2 - Prob. 3CPCh. 7.2 - CHECK POINT 4 Graph the linear function by using...Ch. 7.2 - Prob. 5CPCh. 7.2 - CHECK POINT 6 Graph in the rectangular coordinate...Ch. 7.2 - CHECK POINT 7 Find the slope of the line segment...Ch. 7.2 - Prob. 8CPCh. 7.2 - The x-coordinate of a point where a graph crosses...Ch. 7.2 - The point (0, 3) lies along a line, so 3 is a/an...Ch. 7.2 - The slope of the line through the distinct points...Ch. 7.2 - Prob. 4CVCCh. 7.2 - The slope of the linear function whose equation is...Ch. 7.2 - 6. In order to graph the line whose equation is...Ch. 7.2 - Prob. 7CVCCh. 7.2 - Prob. 8CVCCh. 7.2 - In Exercises 1-8, use the x- and y-intercepts to...Ch. 7.2 - In Exercises 1-8, use the x- and y-intercepts to...Ch. 7.2 - In Exercises 1-8, use the x- and y-intercepts to...Ch. 7.2 - Prob. 4ECh. 7.2 - In Exercises 1-8, use the x- and y-intercepts to...Ch. 7.2 - In Exercises 1-8, use the x- and y-intercepts to...Ch. 7.2 - Prob. 7ECh. 7.2 - In Exercises 1-8, use the x- and y-intercepts to...Ch. 7.2 - In Exercises 9-20, calculate the slope of the line...Ch. 7.2 - Prob. 10ECh. 7.2 - In Exercises 9-20, calculate the slope of the line...Ch. 7.2 - Prob. 12ECh. 7.2 - In Exercises 9-20, calculate the slope of the line...Ch. 7.2 - Prob. 14ECh. 7.2 - In Exercises 9-20, calculate the slope of the line...Ch. 7.2 - Prob. 16ECh. 7.2 - Prob. 17ECh. 7.2 - Prob. 18ECh. 7.2 - In Exercises 9-20, calculate the slope of the line...Ch. 7.2 - Prob. 20ECh. 7.2 - Prob. 21ECh. 7.2 - In Exercises 21-32, graph each linear function...Ch. 7.2 - In Exercises 21-32, graph each linear function...Ch. 7.2 - Prob. 24ECh. 7.2 - Prob. 25ECh. 7.2 - Prob. 26ECh. 7.2 - Prob. 27ECh. 7.2 - In Exercises 21-32, graph each linear function...Ch. 7.2 - In Exercises 21-32, graph each linear function...Ch. 7.2 - In Exercises 21-32, graph each linear function...Ch. 7.2 - Prob. 31ECh. 7.2 - Prob. 32ECh. 7.2 - Prob. 33ECh. 7.2 - In Exercises 33-40, a. Put the equation in...Ch. 7.2 - In Exercises 33-40, a. Put the equation in...Ch. 7.2 - In Exercises 33-40, a. Put the equation in...Ch. 7.2 - In Exercises 33-40, a. Put the equation in...Ch. 7.2 - Prob. 38ECh. 7.2 - In Exercises 33-40, a. Put the equation in...Ch. 7.2 - Prob. 40ECh. 7.2 - Prob. 41ECh. 7.2 - In Exercises 41-48, graph each horizontal or...Ch. 7.2 - In Exercises 41-48, graph each horizontal or...Ch. 7.2 - In Exercises 41-48, graph each horizontal or...Ch. 7.2 - In Exercises 41-48, graph each horizontal or...Ch. 7.2 - Prob. 46ECh. 7.2 - In Exercises 41-48, graph each horizontal or...Ch. 7.2 - In Exercises 41-48, graph each horizontal or...Ch. 7.2 - Prob. 49ECh. 7.2 - Prob. 50ECh. 7.2 - Prob. 51ECh. 7.2 - Prob. 52ECh. 7.2 - Prob. 53ECh. 7.2 - Prob. 54ECh. 7.2 - Prob. 55ECh. 7.2 - Prob. 56ECh. 7.2 - Prob. 57ECh. 7.2 - Prob. 58ECh. 7.2 - 59. Older. Calmer. As we age, daily stress and...Ch. 7.2 - Prob. 60ECh. 7.2 - Prob. 61ECh. 7.2 - Prob. 62ECh. 7.2 - Prob. 63ECh. 7.2 - Prob. 64ECh. 7.2 - Describe how to find the x-intercept of a linear...Ch. 7.2 - Prob. 66ECh. 7.2 - What is the slope of a line?Ch. 7.2 - Prob. 68ECh. 7.2 - Describe how to graph a line using the slope and...Ch. 7.2 - Prob. 70ECh. 7.2 - What does it mean if the slope of a line is...Ch. 7.2 - Prob. 72ECh. 7.2 - Prob. 73ECh. 7.2 - Prob. 74ECh. 7.2 - Make Sense? In Exercises 74-77, determine whether...Ch. 7.2 - Prob. 76ECh. 7.2 - Make Sense? In Exercises 74-77, determine whether...Ch. 7.2 - In Exercises 78-81, determine whether each...Ch. 7.2 - In Exercises 78-81, determine whether each...Ch. 7.2 - Prob. 80ECh. 7.2 - In Exercises 78-81, determine whether each...Ch. 7.2 - Prob. 82ECh. 7.2 - Prob. 83ECh. 7.2 - Use a graphing utility to verify any three of your...Ch. 7.3 - CHECK POINT 1 Determine whether ( − 4 , 3 ) is a...Ch. 7.3 - CHECK POINT 2 Solve by graphing: { 2 x + 3 y = 6 2...Ch. 7.3 - CHECK POINT 3 Solve by the substitution method: {...Ch. 7.3 - CHECK POINT 4 Solve by the substitution method: Ch. 7.3 - CHECK POINT 5 Solve by the addition method: { 4 x...Ch. 7.3 - CHECK POINT 6 Solve by the addition method: { 3 x...Ch. 7.3 - CHECK POINT 7 Solve the system: {     x + 2 y =  ...Ch. 7.3 - CHECK POINT 8 Solve the system: Ch. 7.3 - CHECK POINT 9 A company that manufactures running...Ch. 7.3 - Fill in each blank so that the resulting statement...Ch. 7.3 - Fill in each blank so that the resulting statement...Ch. 7.3 - Fill in each blank so that the resulting statement...Ch. 7.3 - Fill in each blank so that the resulting statement...Ch. 7.3 - Prob. 5CVCCh. 7.3 - Prob. 6CVCCh. 7.3 - Prob. 7CVCCh. 7.3 - Fill in each blank so that the resulting statement...Ch. 7.3 - Fill in each blank so that the resulting statement...Ch. 7.3 - In Exercises 1-4, determine whether the given...Ch. 7.3 - In Exercises 1-4, determine whether the given...Ch. 7.3 - In Exercises 1-4, determine whether the given...Ch. 7.3 - In Exercises 1-4, determine whether the given...Ch. 7.3 - In Exercises 5-12, solve each system by graphing....Ch. 7.3 - In Exercises 5-12, solve each system by graphing....Ch. 7.3 - In Exercises 5-12, solve each system by graphing....Ch. 7.3 - Prob. 8ECh. 7.3 - In Exercises 5-12, solve each system by graphing....Ch. 7.3 - In Exercises 5-12, solve each system by graphing....Ch. 7.3 - In Exercises 5-12, solve each system by graphing....Ch. 7.3 - In Exercises 5-12, solve each system by graphing....Ch. 7.3 - In Exercises 13-24, solve each system by the...Ch. 7.3 - Prob. 14ECh. 7.3 - In Exercises 13-24, solve each system by the...Ch. 7.3 - Prob. 16ECh. 7.3 - In Exercises 13-24, solve each system by the...Ch. 7.3 - Prob. 18ECh. 7.3 - In Exercises 13-24, solve each system by the...Ch. 7.3 - In Exercises 13-24, solve each system by the...Ch. 7.3 - In Exercises 13-24, solve each system by the...Ch. 7.3 - In Exercises 13-24, solve each system by the...Ch. 7.3 - In Exercises 13-24, solve each system by the...Ch. 7.3 - In Exercises 13-24, solve each system by the...Ch. 7.3 - In Exercises 25-36, solve each system by the...Ch. 7.3 - In Exercises 25-36, solve each system by the...Ch. 7.3 - In Exercises 25-36, solve each system by the...Ch. 7.3 - In Exercises 25-36, solve each system by the...Ch. 7.3 - In Exercises 25-36, solve each system by the...Ch. 7.3 - In Exercises 25-36, solve each system by the...Ch. 7.3 - In Exercises 25-36, solve each system by the...Ch. 7.3 - In Exercises 25-36, solve each system by the...Ch. 7.3 - In Exercises 25-36, solve each system by the...Ch. 7.3 - In Exercises 25-36, solve each system by the...Ch. 7.3 - In Exercises 25-36, solve each system by the...Ch. 7.3 - Prob. 36ECh. 7.3 - In Exercises 37-44, solve by the method of your...Ch. 7.3 - In Exercises 37-44, solve by the method of your...Ch. 7.3 - In Exercises 37-44, solve by the method of your...Ch. 7.3 - Prob. 40ECh. 7.3 - In Exercises 37-44, solve by the method of your...Ch. 7.3 - In Exercises 37-44, solve by the method of your...Ch. 7.3 - In Exercises 37-44, solve by the method of your...Ch. 7.3 - Prob. 44ECh. 7.3 - Use the graphs of the linear functions to solve...Ch. 7.3 - Prob. 46ECh. 7.3 - Prob. 47ECh. 7.3 - In Exercises 47-48, solve each system for x and y,...Ch. 7.3 - Prob. 49ECh. 7.3 - Prob. 50ECh. 7.3 - The figure shows the graphs of the cost and...Ch. 7.3 - Prob. 52ECh. 7.3 - The figure shows the graphs of the cost and...Ch. 7.3 - The figure shows the graphs of the cost and...Ch. 7.3 - The figure shows the graphs of the cost and...Ch. 7.3 - Prob. 56ECh. 7.3 - Exercises 57-60 describe a number of business...Ch. 7.3 - Prob. 58ECh. 7.3 - Exercises 57-60 describe a number of business...Ch. 7.3 - Prob. 60ECh. 7.3 - An important application of systems of equations...Ch. 7.3 - Prob. 62ECh. 7.3 - We opened this section with a study showing that...Ch. 7.3 - Prob. 64ECh. 7.3 - 65. What is a system of linear equations? Provide...Ch. 7.3 - What is the solution to a system of linear...Ch. 7.3 - Prob. 67ECh. 7.3 - Prob. 68ECh. 7.3 - Prob. 69ECh. 7.3 - Prob. 70ECh. 7.3 - When is it easier to use the addition method...Ch. 7.3 - Prob. 72ECh. 7.3 - 73. When using the addition or substitution...Ch. 7.3 - Prob. 74ECh. 7.3 - Prob. 75ECh. 7.3 - Prob. 76ECh. 7.3 - Prob. 77ECh. 7.3 - Prob. 78ECh. 7.3 - Prob. 79ECh. 7.3 - Prob. 80ECh. 7.3 - Prob. 81ECh. 7.4 - CHECK POINT 1 Graph: 2 x − 4 y ≥ 8.Ch. 7.4 - CHECK POINT 2 Graph: y > − 3 4 x .Ch. 7.4 - Prob. 3CPCh. 7.4 - Prob. 4CPCh. 7.4 - CHECK POINT 5 Graph the solution set of the...Ch. 7.4 - CHECK POINT 6 Graph the solution set of the...Ch. 7.4 - Prob. 1CVCCh. 7.4 - Fill in each blank so that the resulting statement...Ch. 7.4 - Prob. 3CVCCh. 7.4 - Prob. 4CVCCh. 7.4 - Prob. 5CVCCh. 7.4 - Prob. 6CVCCh. 7.4 - Prob. 7CVCCh. 7.4 - Prob. 8CVCCh. 7.4 - In Exercises 1-22, graph each linear inequality. x...Ch. 7.4 - Prob. 2ECh. 7.4 - In Exercises 1-22, graph each linear inequality. 3...Ch. 7.4 - Prob. 4ECh. 7.4 - In Exercises 1-22, graph each linear inequality. 2...Ch. 7.4 - Prob. 6ECh. 7.4 - In Exercises 1-22, graph each linear inequality. 5...Ch. 7.4 - Prob. 8ECh. 7.4 - In Exercises 1-22, graph each linear inequality. 2...Ch. 7.4 - Prob. 10ECh. 7.4 - In Exercises 1-22, graph each linear...Ch. 7.4 - Prob. 12ECh. 7.4 - In Exercises 1-22, graph each linear inequality. y...Ch. 7.4 - Prob. 14ECh. 7.4 - In Exercises 1-22, graph each linear...Ch. 7.4 - Prob. 16ECh. 7.4 - In Exercises 1-22, graph each linear...Ch. 7.4 - Prob. 18ECh. 7.4 - In Exercises 1-22, graph each linear inequality. y...Ch. 7.4 - Prob. 20ECh. 7.4 - In Exercises 1-22, graph each linear inequality. y...Ch. 7.4 - Prob. 22ECh. 7.4 - In Exercises 23-38, graph the solution set of each...Ch. 7.4 - In Exercises 23-38, graph the solution set of each...Ch. 7.4 - In Exercises 23-38, graph the solution set of each...Ch. 7.4 - Prob. 26ECh. 7.4 - In Exercises 23-38, graph the solution set of each...Ch. 7.4 - In Exercises 23-38, graph the solution set of each...Ch. 7.4 - In Exercises 23-38, graph the solution set of each...Ch. 7.4 - Prob. 30ECh. 7.4 - In Exercises 23-38, graph the solution set of each...Ch. 7.4 - Prob. 32ECh. 7.4 - In Exercises 23-38, graph the solution set of each...Ch. 7.4 - Prob. 34ECh. 7.4 - In Exercises 23-38, graph the solution set of each...Ch. 7.4 - In Exercises 23-38, graph the solution set of each...Ch. 7.4 - In Exercises 23-38, graph the solution set of each...Ch. 7.4 - In Exercises 23-38, graph the solution set of each...Ch. 7.4 - Prob. 39ECh. 7.4 - Prob. 40ECh. 7.4 - Prob. 41ECh. 7.4 - Prob. 42ECh. 7.4 - Prob. 43ECh. 7.4 - Prob. 44ECh. 7.4 - The figure shows the healthy weight region for...Ch. 7.4 - The figure shows the healthy weight region for...Ch. 7.4 - The figure shows the healthy weight region for...Ch. 7.4 - The figure shows the healthy weight region for...Ch. 7.4 - 49. Many elevators have a capacity of 2000...Ch. 7.4 - Prob. 50ECh. 7.4 - In these graphs, each horizontal axis shows a...Ch. 7.4 - In these graphs, each horizontal axis shows a...Ch. 7.4 - What is a half-plane?Ch. 7.4 - What does a dashed line mean in the graph of an...Ch. 7.4 - Prob. 55ECh. 7.4 - Prob. 56ECh. 7.4 - Prob. 57ECh. 7.4 - Make Sense? In Exercises 58-61, determine whether...Ch. 7.4 - Make Sense? In Exercises 58-61, determine whether...Ch. 7.4 - Make Sense? In Exercises 58-61, determine whether...Ch. 7.4 - Make Sense? In Exercises 58-61, determine whether...Ch. 7.4 - Prob. 62ECh. 7.4 - In Exercises 62-63, write a system of inequalities...Ch. 7.4 - Prob. 64ECh. 7.4 - Without graphing, in Exercises 64-67, determine if...Ch. 7.4 - Prob. 66ECh. 7.4 - Prob. 67ECh. 7.5 - CHECK POINT 1 A company manufactures bookshelves...Ch. 7.5 - CHECK POINT 2 To maintain high quality, the...Ch. 7.5 - CHECK POINT 3 To meet customer demand, the company...Ch. 7.5 - CHECK POINT 4 For the company in Check Points 1-3,...Ch. 7.5 - 1. A method for finding the maximum or minimum...Ch. 7.5 - 2. An algebraic expression in two or more...Ch. 7.5 - A system of linear inequalities is used to...Ch. 7.5 - In Exercises 1-4, find the value of the objective...Ch. 7.5 - Prob. 2ECh. 7.5 - In Exercises 1-4, find the value of the objective...Ch. 7.5 - Prob. 4ECh. 7.5 - In Exercises 5-8, an objective function and a...Ch. 7.5 - Prob. 6ECh. 7.5 - In Exercises 5-8, an objective function and a...Ch. 7.5 - In Exercises 5-8, an objective function and a...Ch. 7.5 - Use the directions for Exercises 5-8 to solve...Ch. 7.5 - Use the directions for Exercises 5-8 to solve...Ch. 7.5 - Use the directions for Exercises 5-8 to solve...Ch. 7.5 - Use the directions for Exercises 5-8 to solve...Ch. 7.5 - Prob. 13ECh. 7.5 - Prob. 14ECh. 7.5 - 15. Food and clothing are shipped to survivors of...Ch. 7.5 - Prob. 16ECh. 7.5 - 17. A theater is presenting a program on drinking...Ch. 7.5 - Prob. 18ECh. 7.5 - What kinds of problems are solved using the linear...Ch. 7.5 - Prob. 20ECh. 7.5 - Prob. 21ECh. 7.5 - Prob. 22ECh. 7.5 - Describe a situation in your life in which you...Ch. 7.5 - Prob. 24ECh. 7.5 - Make Sense? In Exercises 24-27, determine whether...Ch. 7.5 - Prob. 26ECh. 7.5 - Make Sense? In Exercises 24-27, determine whether...Ch. 7.5 - Prob. 28ECh. 7.5 - Group members should choose a particular field of...Ch. 7.5 - Members of the group should interview a business...Ch. 7.6 - CHECK POINT 1 Graph: f ( x ) = 3 x .Ch. 7.6 - CHECK POINT 2 Use the models f ( x ) = 0.074 x +...Ch. 7.6 - Prob. 3CPCh. 7.6 - Prob. 4CPCh. 7.6 - Prob. 5CPCh. 7.6 - Prob. 6CPCh. 7.6 - Prob. 7CPCh. 7.6 - Prob. 1CVCCh. 7.6 - Prob. 2CVCCh. 7.6 - Prob. 3CVCCh. 7.6 - For each set of points in Exercises 2-5, determine...Ch. 7.6 - Prob. 5CVCCh. 7.6 - 6. The exponential function with base b is defined...Ch. 7.6 - Prob. 7CVCCh. 7.6 - Prob. 8CVCCh. 7.6 - 9. is usually expressed as ______ and is called...Ch. 7.6 - Prob. 10CVCCh. 7.6 - Prob. 11CVCCh. 7.6 - In Exercises 1-6, use a table of coordinates to...Ch. 7.6 - Prob. 2ECh. 7.6 - Prob. 3ECh. 7.6 - In Exercises 1-6, use a table of coordinates to...Ch. 7.6 - Prob. 5ECh. 7.6 - Prob. 6ECh. 7.6 - Prob. 7ECh. 7.6 - Prob. 8ECh. 7.6 - In Exercises 9-14, a. Determine if the parabola...Ch. 7.6 - In Exercises 9-14, a. Determine if the parabola...Ch. 7.6 - In Exercises 9-14, a. Determine if the parabola...Ch. 7.6 - In Exercises 9-14, a. Determine if the parabola...Ch. 7.6 - In Exercises 9-14, a. Determine if the parabola...Ch. 7.6 - In Exercises 9-14, a. Determine if the parabola...Ch. 7.6 - In Exercises 15-22, a. Create a scatter plot for...Ch. 7.6 - Prob. 16ECh. 7.6 - In Exercises 15-22, a. Create a scatter plot for...Ch. 7.6 - In Exercises 15-22, a. Create a scatter plot for...Ch. 7.6 - In Exercises 15-22, a. Create a scatter plot for...Ch. 7.6 - Prob. 20ECh. 7.6 - In Exercises 15-22, a. Create a scatter plot for...Ch. 7.6 - Prob. 22ECh. 7.6 - In Exercises 23-24, use a table of coordinates to...Ch. 7.6 - Prob. 24ECh. 7.6 - Prob. 25ECh. 7.6 - Prob. 26ECh. 7.6 - Prob. 27ECh. 7.6 - Prob. 28ECh. 7.6 - In Exercises 29-30, find the vertex for the...Ch. 7.6 - Prob. 30ECh. 7.6 - The graphing calculator screen displays an...Ch. 7.6 - The graphing calculator screen displays an...Ch. 7.6 - Prob. 33ECh. 7.6 - Prob. 34ECh. 7.6 - The graphing calculator screen displays the...Ch. 7.6 - Prob. 36ECh. 7.6 - The percentage of adult height attained by a girl...Ch. 7.6 - The percentage of adult height attained by a girl...Ch. 7.6 - Prob. 39ECh. 7.6 - Prob. 40ECh. 7.6 - What is a scatter plot?Ch. 7.6 - Prob. 42ECh. 7.6 - Prob. 43ECh. 7.6 - Prob. 44ECh. 7.6 - Prob. 45ECh. 7.6 - Prob. 46ECh. 7.6 - Prob. 47ECh. 7.6 - Prob. 48ECh. 7.6 - Prob. 49ECh. 7.6 - Prob. 50ECh. 7.6 - In Exercises 51-53, the value of a in y = a x 2 +...Ch. 7.6 - Prob. 52ECh. 7.6 - Prob. 53ECh. 7.6 - Prob. 54ECh. 7.6 - Prob. 55ECh. 7.6 - Each group member should consult an almanac,...Ch. 7 - Prob. 1TCh. 7 - Prob. 2TCh. 7 - Prob. 3TCh. 7 - Prob. 4TCh. 7 - Prob. 5TCh. 7 - Prob. 6TCh. 7 - Prob. 7TCh. 7 - Prob. 8TCh. 7 - Prob. 9TCh. 7 - Prob. 10TCh. 7 - Prob. 11TCh. 7 - Prob. 12TCh. 7 - Prob. 13TCh. 7 - Prob. 14TCh. 7 - Prob. 15TCh. 7 - Prob. 16TCh. 7 - Prob. 17TCh. 7 - Prob. 18TCh. 7 - Prob. 19TCh. 7 - Prob. 20TCh. 7 - Prob. 21TCh. 7 - Prob. 22TCh. 7 - Prob. 23TCh. 7 - Prob. 24TCh. 7 - Prob. 25TCh. 7 - Prob. 26TCh. 7 - Prob. 27TCh. 7 - Prob. 28TCh. 7 - Prob. 29TCh. 7 - Prob. 30TCh. 7 - In Exercises 1-4, plot the given point in a...Ch. 7 - In Exercises 1-4, plot the given point in a...Ch. 7 - Prob. 3RECh. 7 - In Exercises 1-4, plot the given point in a...Ch. 7 - Prob. 5RECh. 7 - Prob. 6RECh. 7 - Prob. 7RECh. 7 - Prob. 8RECh. 7 - Prob. 9RECh. 7 - Prob. 10RECh. 7 - Prob. 11RECh. 7 - Prob. 12RECh. 7 - Prob. 13RECh. 7 - In Exercises 14-15, use the vertical line test to...Ch. 7 - In Exercises 14-15, use the vertical line test to...Ch. 7 - Prob. 16RECh. 7 - Prob. 17RECh. 7 - Prob. 18RECh. 7 - Prob. 19RECh. 7 - In Exercises 20-23, calculate the slope of the...Ch. 7 - Prob. 21RECh. 7 - Prob. 22RECh. 7 - Prob. 23RECh. 7 - Prob. 24RECh. 7 - Prob. 25RECh. 7 - Prob. 26RECh. 7 - Prob. 27RECh. 7 - Prob. 28RECh. 7 - Prob. 29RECh. 7 - Prob. 30RECh. 7 - Prob. 31RECh. 7 - Prob. 32RECh. 7 - Prob. 33RECh. 7 - 34. Shown, again, is the scatter plot that...Ch. 7 - Prob. 35RECh. 7 - Prob. 36RECh. 7 - Prob. 37RECh. 7 - Prob. 38RECh. 7 - Prob. 39RECh. 7 - Prob. 40RECh. 7 - Prob. 41RECh. 7 - Prob. 42RECh. 7 - Prob. 43RECh. 7 - In Exercises 44-46, solve by the method of your...Ch. 7 - Prob. 45RECh. 7 - Prob. 46RECh. 7 - Prob. 47RECh. 7 - Prob. 48RECh. 7 - Prob. 49RECh. 7 - Prob. 50RECh. 7 - Prob. 51RECh. 7 - Prob. 52RECh. 7 - Prob. 53RECh. 7 - Prob. 54RECh. 7 - Prob. 55RECh. 7 - Prob. 56RECh. 7 - Prob. 57RECh. 7 - Prob. 58RECh. 7 - Prob. 59RECh. 7 - Prob. 60RECh. 7 - Prob. 61RECh. 7 - Prob. 62RECh. 7 - Prob. 63RECh. 7 - Prob. 64RECh. 7 - Prob. 65RECh. 7 - Prob. 66RECh. 7 - Prob. 67RECh. 7 - Prob. 68RECh. 7 - Prob. 69RECh. 7 - Prob. 70RECh. 7 - Prob. 71RECh. 7 - Prob. 72RECh. 7 - Prob. 73RECh. 7 - Prob. 74RE
Knowledge Booster
Background pattern image
Math
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
Text book image
College Algebra
Algebra
ISBN:9781337282291
Author:Ron Larson
Publisher:Cengage Learning
Text book image
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Text book image
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
SEE MORE TEXTBOOKS
Use of ALGEBRA in REAL LIFE; Author: Fast and Easy Maths !;https://www.youtube.com/watch?v=9_PbWFpvkDc;License: Standard YouTube License, CC-BY
Compound Interest Formula Explained, Investment, Monthly & Continuously, Word Problems, Algebra; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=P182Abv3fOk;License: Standard YouTube License, CC-BY
Applications of Algebra (Digit, Age, Work, Clock, Mixture and Rate Problems); Author: EngineerProf PH;https://www.youtube.com/watch?v=Y8aJ_wYCS2g;License: Standard YouTube License, CC-BY