Calculus: Early Transcendental Functions
Calculus: Early Transcendental Functions
6th Edition
ISBN: 9781305005303
Author: Ron Larson, Bruce Edwards
Publisher: CENGAGE C
bartleby

Videos

Textbook Question
Book Icon
Chapter 7.3, Problem 58E

Modeling Data A pond is approximately circular, with a diameter of 400 feet. Starting at the center, the depth of the water is measured every 25 feet and recorded in the table (see figure).

x

0

25

50

Depth

20

19

19

x

75

100

125

Depth

17

15

14

x

150

175

200

Depth

10

6

0

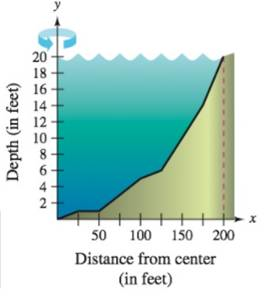
Chapter 7.3, Problem 58E, Modeling Data A pond is approximately circular, with a diameter of 400 feet. Starting at the center,

(a) Use the regression capabilities of a graphing utility to find a quadratic model for the depths recorded in the table. Use the graphing utility to plot the depths and graph the model.

(b) Use the integration capabilities of a graphing utility and the model in part (a) to approximate the volume of water in the pond.

(c) Use the result of part (b) to approximate the number of gallons of water in the pond. (Hint: 1 cubic foot of water is approximately 7.48 gallons.)

Expert Solution & Answer
Check Mark
bartleby

Trending nowThis is a popular solution!

See solution
Check out sample textbook solution
Blurred answer
Previous
Chapter 7.3, Problem 57E
Next
Chapter 7.3, Problem 59E
Students have asked these similar questions
été + Find the area of the surface generated by revolving the curve x= the interval 0 sys In 7 about the y-axis. in 2 2 -In 7
Find area of the sector
Number 18 please show work

Chapter 7 Solutions

Calculus: Early Transcendental Functions

Ch. 7.1 - Writing a Definite Integral In Exercises 5-10,...Ch. 7.1 - Writing a Definite Integral In Exercises 5-10,...Ch. 7.1 - Writing a Definite Integral In Exercises 5-10,...Ch. 7.1 - Writing a Definite Integral In Exercises 5-10,...Ch. 7.1 - Writing a Definite Integral In Exercises 5-10,...Ch. 7.1 - Writing a Definite Integral In Exercises 5-10,...Ch. 7.1 - Finding a Region In Exercises 11-14, the integrand...Ch. 7.1 - Prob. 8ECh. 7.1 - Finding a Region In Exercises 11-14, the integrand...Ch. 7.1 - Prob. 10E
Ch. 7.1 - Finding a Region In Exercises 11-14, the integrand...Ch. 7.1 - Finding a Region In Exercises 11-14, the integrand...Ch. 7.1 - Prob. 13ECh. 7.1 - Prob. 14ECh. 7.1 - Finding the Area of a Region In Exercises 1S-2B,...Ch. 7.1 - Finding the area of a Region In Exercises 15-28....Ch. 7.1 - Finding the area of a Region In Exercises 15-28....Ch. 7.1 - Finding the area of a Region In Exercises 15-28....Ch. 7.1 - Finding the Area of a Region In Exercises 1730,...Ch. 7.1 - Prob. 22ECh. 7.1 - Prob. 23ECh. 7.1 - Finding the area of a Region In Exercises 15-28....Ch. 7.1 - Finding the area of a Region In Exercises 15-28....Ch. 7.1 - Finding the area of a Region In Exercises 15-28....Ch. 7.1 - Finding the area of a Region In Exercises 15-28....Ch. 7.1 - Finding the area of a Region In Exercises 15-28....Ch. 7.1 - Finding the area of a Region In Exercises 15-28....Ch. 7.1 - Finding the area of a Region In Exercises 15-28....Ch. 7.1 - Comparing Methods In Exercises 29 and 30, find the...Ch. 7.1 - Comparing Methods In Exercises 29 and 30, find the...Ch. 7.1 - Finding the Area of a Region In Exercises 31-36,...Ch. 7.1 - Prob. 32ECh. 7.1 - Finding the Area of a Region In Exercises 31-36,...Ch. 7.1 - Finding the Area of a Region In Exercises 31-36,...Ch. 7.1 - Finding the Area of a Region In Exercises 31-36,...Ch. 7.1 - Finding the Area of a Region In Exercises 31-36,...Ch. 7.1 - Finding the Area of a Region In Exercises 37-42,...Ch. 7.1 - Finding the Area of a Region In Exercises 37-42,...Ch. 7.1 - Finding the Area of a Region In Exercises 37-42,...Ch. 7.1 - Prob. 40ECh. 7.1 - Finding the Area of a Region In Exercises 37-42,...Ch. 7.1 - Finding the Area of a Region In Exercises 37-42,...Ch. 7.1 - Prob. 43ECh. 7.1 - Prob. 44ECh. 7.1 - Prob. 45ECh. 7.1 - Prob. 46ECh. 7.1 - Prob. 47ECh. 7.1 - Prob. 48ECh. 7.1 - Prob. 49ECh. 7.1 - Prob. 50ECh. 7.1 - Prob. 51ECh. 7.1 - Integration as an Accumulation Process In...Ch. 7.1 - Prob. 53ECh. 7.1 - Prob. 54ECh. 7.1 - Prob. 55ECh. 7.1 - Prob. 56ECh. 7.1 - Prob. 57ECh. 7.1 - Finding the Area of a Figure In Exercises 57-60,...Ch. 7.1 - Numerical Integration Estimate the surface area of...Ch. 7.1 - Numerical Integration Estimate the surface area of...Ch. 7.1 - Prob. 61ECh. 7.1 - Prob. 62ECh. 7.1 - Using a Tangent Line In Exercises 61-64, write and...Ch. 7.1 - Prob. 64ECh. 7.1 - Prob. 65ECh. 7.1 - Prob. 66ECh. 7.1 - Prob. 67ECh. 7.1 - Prob. 68ECh. 7.1 - Prob. 69ECh. 7.1 - Prob. 70ECh. 7.1 - Prob. 71ECh. 7.1 - Prob. 72ECh. 7.1 - Prob. 73ECh. 7.1 - Prob. 74ECh. 7.1 - Revenue In Exercises 75 and 76. two models R 1,...Ch. 7.1 - Prob. 76ECh. 7.1 - Prob. 77ECh. 7.1 - Profit The chief financial officer of a company...Ch. 7.1 - Building Design Concrete sections for a new...Ch. 7.1 - Prob. 80ECh. 7.1 - Area Find the area between the graph of y=sinx and...Ch. 7.1 - Prob. 82ECh. 7.1 - Prob. 83ECh. 7.1 - Prob. 84ECh. 7.1 - Prob. 85ECh. 7.1 - Prob. 86ECh. 7.1 - Prob. 87ECh. 7.2 - Finding the Volume of a Solid In Exercises 5-8,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 5-8,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 16, set...Ch. 7.2 - Prob. 4ECh. 7.2 - Finding the Volume of a Solid In Exercises 5-8,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 5-8,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 9-12,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 9-12,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 9-12,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 9-12,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 13-16,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 13-16,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 13-16,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 13-16,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 17-20,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 17-20,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 1518,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 1518,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 21-24,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 1922,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 21-24,...Ch. 7.2 - Prob. 22ECh. 7.2 - Finding the Volume of a Solid In Exercises 2330....Ch. 7.2 - Finding the Volume of a Solid In Exercises 25-32,...Ch. 7.2 - Prob. 25ECh. 7.2 - Finding the Volume of a Solid In Exercises 25-32,...Ch. 7.2 - Prob. 27ECh. 7.2 - Finding the Volume of a Solid In Exercises 25-32,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 25-32,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 25-32,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 33-36,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 33-36,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 37-40,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 37-40,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 37-40,...Ch. 7.2 - Finding the Volume of a Solid In Exercises 37-40,...Ch. 7.2 - Prob. 41ECh. 7.2 - Finding the Volume of a Solid In Exercises 41-48,...Ch. 7.2 - Prob. 43ECh. 7.2 - Prob. 44ECh. 7.2 - Prob. 45ECh. 7.2 - Prob. 46ECh. 7.2 - Prob. 47ECh. 7.2 - Finding the Volume of a Solid In Exercises 4148,...Ch. 7.2 - Finding the Volume of a Solid Using Technology In...Ch. 7.2 - Prob. 38ECh. 7.2 - Finding the Volume of a Solid Using Technology In...Ch. 7.2 - Prob. 49ECh. 7.2 - Prob. 50ECh. 7.2 - Prob. 53ECh. 7.2 - Dividing a Solid In Exercises 55 and 56, consider...Ch. 7.2 - Prob. 56ECh. 7.2 - Manufacturing For the metal sphere in Exercise 59,...Ch. 7.2 - Prob. 63ECh. 7.2 - Prob. 64ECh. 7.2 - Finding Volumes of Solids Find the volumes of the...Ch. 7.2 - Water Tower A tank on a water tower is a sphere of...Ch. 7.2 - Minimum Volume The function y=4(x2/4) on the...Ch. 7.2 - Prob. 68ECh. 7.2 - Prob. 70ECh. 7.3 - Finding the Volume of a Solid In Exercises 3-12,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 3-12,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 3-12,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 3-12,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 3-12,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 3-12,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 3-12,...Ch. 7.3 - Prob. 8ECh. 7.3 - Prob. 9ECh. 7.3 - Finding the Volume of a Solid In Exercises 3-12,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 3-12,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 3-12,...Ch. 7.3 - Prob. 13ECh. 7.3 - Prob. 14ECh. 7.3 - Finding the Volume of a Solid In Exercises 13-22,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 13-22,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 13-22,...Ch. 7.3 - Prob. 18ECh. 7.3 - Finding the Volume of a Solid In Exercises 13-22,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 13-22,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 13-22,...Ch. 7.3 - Prob. 22ECh. 7.3 - Finding the Volume of a Solid In Exercises 23-26,...Ch. 7.3 - Finding the Volume of a Solid In Exercises 23-26,...Ch. 7.3 - Prob. 25ECh. 7.3 - Finding the Volume of a Solid In Exercises 23-26,...Ch. 7.3 - Choosing a Method In Exercises 27 and 28, decide...Ch. 7.3 - Prob. 28ECh. 7.3 - Choosing a Method In Exercises 29-32, use the disk...Ch. 7.3 - Prob. 30ECh. 7.3 - Prob. 31ECh. 7.3 - Prob. 32ECh. 7.3 - Finding the Volume of a Solid Using Technology In...Ch. 7.3 - Prob. 34ECh. 7.3 - Prob. 35ECh. 7.3 - Prob. 36ECh. 7.3 - Prob. 37ECh. 7.3 - Prob. 38ECh. 7.3 - Prob. 39ECh. 7.3 - Prob. 40ECh. 7.3 - Comparing Volumes The region in the figure is...Ch. 7.3 - Prob. 42ECh. 7.3 - Prob. 43ECh. 7.3 - Prob. 44ECh. 7.3 - Prob. 45ECh. 7.3 - Prob. 46ECh. 7.3 - Prob. 47ECh. 7.3 - Machine Part A solid is generated by revolving the...Ch. 7.3 - Volume of a Torus A torus is formed by revolving...Ch. 7.3 - Prob. 50ECh. 7.3 - Prob. 51ECh. 7.3 - Prob. 52ECh. 7.3 - Volume of a Segment of a Sphere Let a sphere of...Ch. 7.3 - Prob. 54ECh. 7.3 - Prob. 55ECh. 7.3 - Prob. 56ECh. 7.3 - Volume of a Storage Shed A storage shed has a...Ch. 7.3 - Modeling Data A pond is approximately circular,...Ch. 7.3 - Equal Volumes Let V1 and V2 be the volumes of the...Ch. 7.3 - Prob. 60ECh. 7.3 - Finding Volumes of Solids Consider the graph of...Ch. 7.4 - Prob. 1ECh. 7.4 - Prob. 2ECh. 7.4 - Prob. 3ECh. 7.4 - Finding Arc Length In Exercises 7-20, find the arc...Ch. 7.4 - Prob. 5ECh. 7.4 - Prob. 6ECh. 7.4 - Finding Arc Length In Exercises 7-20, find the arc...Ch. 7.4 - Prob. 8ECh. 7.4 - Finding Arc Length In Exercises 7-20, find the arc...Ch. 7.4 - Finding Arc Length In Exercises 7-20, find the arc...Ch. 7.4 - Finding Arc Length In Exercises 7-20, find the arc...Ch. 7.4 - Finding Arc Length In Exercises 7-20, find the arc...Ch. 7.4 - Finding Arc Length In Exercises 7-20, find the arc...Ch. 7.4 - Prob. 14ECh. 7.4 - Prob. 15ECh. 7.4 - Prob. 16ECh. 7.4 - Prob. 17ECh. 7.4 - Prob. 18ECh. 7.4 - Prob. 19ECh. 7.4 - Prob. 20ECh. 7.4 - Prob. 21ECh. 7.4 - Prob. 22ECh. 7.4 - Finding Arc LengthIn Exercises1726, (a) sketch the...Ch. 7.4 - Prob. 24ECh. 7.4 - Prob. 25ECh. 7.4 - Prob. 26ECh. 7.4 - ApproximationIn Exercises 27 and 28, determine...Ch. 7.4 - ApproximationIn Exercises 27 and 28, determine...Ch. 7.4 - Prob. 29ECh. 7.4 - Prob. 30ECh. 7.4 - Length of a Cable An electric cable is hung...Ch. 7.4 - Roof Area A bam is 100 feet long and 40 feet wide...Ch. 7.4 - Length of Gateway Arch The Gateway Arch in St....Ch. 7.4 - Astroid Find the total length of the graph of the...Ch. 7.4 - Prob. 35ECh. 7.4 - Prob. 36ECh. 7.4 - Finding the Area of a Surface of Revolution In...Ch. 7.4 - Finding the Area of a Surface of Revolution In...Ch. 7.4 - Finding the Area of a Surface of Revolution In...Ch. 7.4 - Finding the Area of a Surface of Revolution In...Ch. 7.4 - Finding the Area of a Surface of Revolution In...Ch. 7.4 - Finding the Area of a Surface of Revolution In...Ch. 7.4 - Finding the Area of a Surface of RevolutionIn...Ch. 7.4 - Prob. 44ECh. 7.4 - Finding the Area of a Surface of Revolution In...Ch. 7.4 - Finding the Area of a Surface of Revolution In...Ch. 7.4 - Finding the Area of a Surface of Revolution Using...Ch. 7.4 - Finding the Area of a Surface of Revolution Using...Ch. 7.4 - Prob. 49ECh. 7.4 - Prob. 50ECh. 7.4 - Prob. 51ECh. 7.4 - Prob. 52ECh. 7.4 - Prob. 53ECh. 7.4 - Verifying a Formula (a) Given a circular sector...Ch. 7.4 - Prob. 55ECh. 7.4 - Prob. 56ECh. 7.4 - Prob. 57ECh. 7.4 - Prob. 58ECh. 7.4 - Prob. 59ECh. 7.4 - Modeling Data Property bounded by two...Ch. 7.4 - Prob. 61ECh. 7.4 - Prob. 62ECh. 7.4 - Approximating Arc Length or Surface Area In...Ch. 7.4 - Approximating Arc Length or Surface Area In...Ch. 7.4 - Prob. 65ECh. 7.4 - Prob. 66ECh. 7.4 - Suspension Bridge A cable for a suspension bridge...Ch. 7.4 - Prob. 68ECh. 7.4 - Prob. 69ECh. 7.4 - Prob. 70ECh. 7.5 - Constant Force In Exercises 5-8, determine the...Ch. 7.5 - Constant ForceIn Exercises 14, determine the work...Ch. 7.5 - Constant Force In Exercises 5-8, determine the...Ch. 7.5 - Prob. 4ECh. 7.5 - Hooke's Law In Exercises 9-14, use Hookes Law to...Ch. 7.5 - Hooke's Law In Exercises 9-14, use Hookes Law to...Ch. 7.5 - Hooke's Law In Exercises 9-14, use Hookes Law to...Ch. 7.5 - Hooke's Law In Exercises 9-14, use Hookes Law to...Ch. 7.5 - Hooke's Law In Exercises 9-14, use Hookes Law to...Ch. 7.5 - Seven and one-half foot-pounds of work is required...Ch. 7.5 - Propulsion Neglecting air resistance and the...Ch. 7.5 - PropulsionUse the information in Exercise 11 to...Ch. 7.5 - Propulsion Neglecting air resistance and the...Ch. 7.5 - Propulsion A lunar module weighs 12 metric tons on...Ch. 7.5 - Pumping Water A rectangular tank with a base 4...Ch. 7.5 - Prob. 16ECh. 7.5 - Pumping Water A cylindrical water tank 4 meters...Ch. 7.5 - Prob. 18ECh. 7.5 - Prob. 19ECh. 7.5 - Prob. 20ECh. 7.5 - Pumping Water A hemispherical tank of radius 6...Ch. 7.5 - Prob. 22ECh. 7.5 - Pumping Gasoline In Exercises 27 and 28, find the...Ch. 7.5 - Pumping Gasoline In Exercises 27 and 28, find the...Ch. 7.5 - Winding a Chain In Exercises 29-32, consider a...Ch. 7.5 - Winding a Chain In Exercises 29-32, consider a...Ch. 7.5 - Winding a Chain In Exercises 29-32, consider a...Ch. 7.5 - Winding a Chain In Exercises 29-32, consider a...Ch. 7.5 - Lifting a Chain In Exercises 33 and 34, consider a...Ch. 7.5 - Prob. 30ECh. 7.5 - Prob. 31ECh. 7.5 - Prob. 32ECh. 7.5 - Prob. 33ECh. 7.5 - HOW DO YOU SEE IT? The graphs show the force Fi...Ch. 7.5 - Prob. 35ECh. 7.5 - Prob. 36ECh. 7.5 - Prob. 37ECh. 7.5 - Prob. 38ECh. 7.5 - Hydraulic Press In Exercises 45-48, use the...Ch. 7.5 - Hydraulic Press In Exercises 45-48, use the...Ch. 7.5 - Hydraulic Press In Exercises 45-48, use the...Ch. 7.5 - Hydraulic Press In Exercises 45-48, use the...Ch. 7.5 - Modeling Data The hydraulic cylinder on a...Ch. 7.6 - Prob. 1ECh. 7.6 - Prob. 2ECh. 7.6 - Center of Mass of a Linear System In Exercises...Ch. 7.6 - Prob. 4ECh. 7.6 - Prob. 5ECh. 7.6 - Prob. 6ECh. 7.6 - Equilibrium of a Linear System In Exercises 9 and...Ch. 7.6 - Equilibrium of a Linear System In Exercises 9 and...Ch. 7.6 - Center of Mass of a Two-Dimensional System In...Ch. 7.6 - Prob. 10ECh. 7.6 - Prob. 11ECh. 7.6 - Center of Mass of a Two-Dimensional System In...Ch. 7.6 - Center of Mass of a Planar Lamina In Exercises...Ch. 7.6 - Prob. 14ECh. 7.6 - Prob. 15ECh. 7.6 - Center of Mass of a Planar Lamina In Exercises...Ch. 7.6 - Prob. 17ECh. 7.6 - Prob. 18ECh. 7.6 - Center of Mass of a Planar Lamina In Exercises...Ch. 7.6 - Center of Mass of a Planar Lamina In Exercises...Ch. 7.6 - Center of Mass of a Planar Lamina In Exercises...Ch. 7.6 - Center of Mass of a Planar Lamina In Exercises...Ch. 7.6 - Center of Mass of a Planar Lamina In Exercises...Ch. 7.6 - Center of Mass of a Planar Lamina In Exercises...Ch. 7.6 - Prob. 25ECh. 7.6 - Prob. 26ECh. 7.6 - Prob. 27ECh. 7.6 - Prob. 28ECh. 7.6 - Prob. 29ECh. 7.6 - Prob. 30ECh. 7.6 - Finding the Center of Mass In Exercises 31-34,...Ch. 7.6 - Prob. 32ECh. 7.6 - Finding the Center of Mass In Exercises 31-34,...Ch. 7.6 - Prob. 34ECh. 7.6 - Finding the Center of Mass Find the center of mass...Ch. 7.6 - Prob. 36ECh. 7.6 - Prob. 37ECh. 7.6 - Prob. 38ECh. 7.6 - Finding Volume by the Theorem of Pappus In...Ch. 7.6 - Prob. 40ECh. 7.6 - Prob. 41ECh. 7.6 - Prob. 42ECh. 7.6 - WRITING ABOUT CONCEPTS Theorem of Pappus State the...Ch. 7.6 - Prob. 44ECh. 7.6 - Prob. 45ECh. 7.6 - Centroid of a Common Region In Exercises 45-50,...Ch. 7.6 - Centroid of a Common Region In Exercises 45-50,...Ch. 7.6 - Centroid of a Common Region In Exercises 45-50,...Ch. 7.6 - Prob. 49ECh. 7.6 - Prob. 50ECh. 7.6 - Prob. 51ECh. 7.6 - Prob. 52ECh. 7.6 - Prob. 53ECh. 7.6 - Modeling Data The manufacturer of a boat needs...Ch. 7.6 - Prob. 55ECh. 7.6 - Prob. 56ECh. 7.6 - Finding a Centroid Let n1 be constant, and...Ch. 7.6 - PUTNAM EXAM CHALLENGE Let V be the region in the...Ch. 7.7 - Prob. 1ECh. 7.7 - Prob. 2ECh. 7.7 - Prob. 3ECh. 7.7 - Force on a Submerged Sheet In Exercises 3-6, the...Ch. 7.7 - Buoyant ForceIn Exercises 5 and 6, find the...Ch. 7.7 - Prob. 6ECh. 7.7 - Fluid Force on a Tank Wall In Exercises 9-14, find...Ch. 7.7 - Fluid Force on a Tank Wall In Exercises 9-14, find...Ch. 7.7 - Fluid Force on a Tank Wall In Exercises 9-14, find...Ch. 7.7 - Fluid Force on a Tank Wall In Exercises 9-14, find...Ch. 7.7 - Fluid Force on a Tank Wall In Exercises 9-14, find...Ch. 7.7 - Fluid Force on a Tank Wall In Exercises 9-14, find...Ch. 7.7 - Fluid Force of Water In Exercises 15-18, Find the...Ch. 7.7 - Fluid Force of Water In Exercises 15-18, Find the...Ch. 7.7 - Fluid Force of Water In Exercises 15-18, Find the...Ch. 7.7 - Fluid Force of Water In Exercises 15-18, Find the...Ch. 7.7 - Prob. 17ECh. 7.7 - Force on a Concrete Form In Exercises 19-22, the...Ch. 7.7 - Prob. 19ECh. 7.7 - Prob. 20ECh. 7.7 - Fluid Force of Gasoline A cylindrical gasoline...Ch. 7.7 - Prob. 22ECh. 7.7 - EXPLORING CONCEPTS Fluid Pressure Explain why...Ch. 7.7 - Prob. 34ECh. 7.7 - Fluid Force on a Circular Plate A circular plate...Ch. 7.7 - Fluid Force on a Circular Plate Use the result of...Ch. 7.7 - Fluid Force on a Rectangular Plate A rectangular...Ch. 7.7 - Fluid Force on a Rectangular Plate Use the result...Ch. 7.7 - Submarine Porthole A square porthole on a vertical...Ch. 7.7 - Prob. 28ECh. 7.7 - Modeling Data The vertical stem of a boat...Ch. 7.7 - Prob. 30ECh. 7.7 - Prob. 31ECh. 7.7 - Prob. 32ECh. 7 - Finding the Area of a Region In Exercises 1-10,...Ch. 7 - Finding the Area of a Region In Exercises 1-10,...Ch. 7 - Prob. 3RECh. 7 - Prob. 4RECh. 7 - Prob. 5RECh. 7 - Prob. 6RECh. 7 - Prob. 7RECh. 7 - Prob. 8RECh. 7 - Prob. 9RECh. 7 - Prob. 10RECh. 7 - Prob. 11RECh. 7 - Prob. 12RECh. 7 - Prob. 13RECh. 7 - Finding the Area of a Region In Exercises 11-14,...Ch. 7 - Prob. 15RECh. 7 - Prob. 16RECh. 7 - Prob. 20RECh. 7 - Finding the Volume of a Solid In Exercises 19 and...Ch. 7 - Prob. 21RECh. 7 - Prob. 19RECh. 7 - Finding the Volume of a Solid In Exercises 23 and...Ch. 7 - Finding the Volume of a Solid In Exercises 23 and...Ch. 7 - Gasoline Tank A gasoline tank is an oblate...Ch. 7 - Prob. 24RECh. 7 - Prob. 25RECh. 7 - Prob. 26RECh. 7 - Length of a Catenary A cable of a suspension...Ch. 7 - Prob. 28RECh. 7 - Prob. 29RECh. 7 - Prob. 30RECh. 7 - Prob. 31RECh. 7 - Hooke's Law A force of 50 pounds stretches a...Ch. 7 - Pumping Water A water well has an 8-inch diameter...Ch. 7 - Prob. 34RECh. 7 - Winding a Chain A chain 10 feet long weighs 4...Ch. 7 - Prob. 36RECh. 7 - Prob. 37RECh. 7 - Prob. 38RECh. 7 - Center of Mass of a Linear System Find the center...Ch. 7 - Prob. 40RECh. 7 - Prob. 41RECh. 7 - Prob. 42RECh. 7 - Prob. 43RECh. 7 - Finding Volume Use the Theorem of Pappus to find...Ch. 7 - Fluid Force of Seawater Find the fluid force on...Ch. 7 - Force on a Concrete Form The vertical side of a...Ch. 7 - Prob. 47RECh. 7 - Finding a Limit Let R be the area of the region in...Ch. 7 - Prob. 2PSCh. 7 - Dividing a Region Let R be the region bounded by...Ch. 7 - Prob. 5PSCh. 7 - Volume A hole is cut through the center of a...Ch. 7 - Toms (a) A torus is formed by revolving the region...Ch. 7 - Prob. 7PSCh. 7 - Comparing Areas of Regions (a) The tangent line to...Ch. 7 - Prob. 9PSCh. 7 - Prob. 10PSCh. 7 - Archimedes' Principle Archimedes Principle states...Ch. 7 - Prob. 12PSCh. 7 - Prob. 13PSCh. 7 - Prob. 14PSCh. 7 - Prob. 15PSCh. 7 - Prob. 16PSCh. 7 - Prob. 17PS
Knowledge Booster
Background pattern image
Calculus
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
Text book image
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Text book image
College Algebra
Algebra
ISBN:9781337282291
Author:Ron Larson
Publisher:Cengage Learning
Text book image
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
SEE MORE TEXTBOOKS
UG/ linear equation in linear algebra; Author: The Gate Academy;https://www.youtube.com/watch?v=aN5ezoOXX5A;License: Standard YouTube License, CC-BY
System of Linear Equations-I; Author: IIT Roorkee July 2018;https://www.youtube.com/watch?v=HOXWRNuH3BE;License: Standard YouTube License, CC-BY